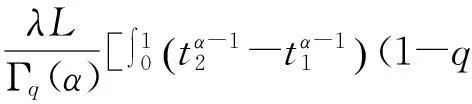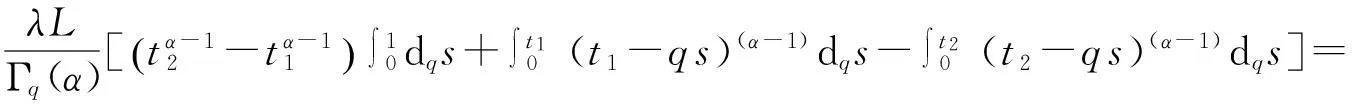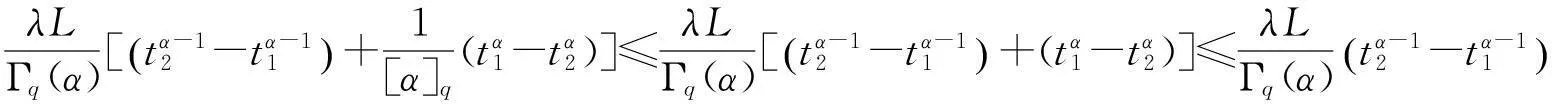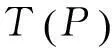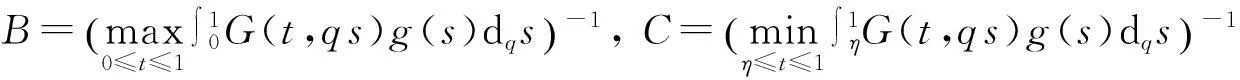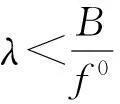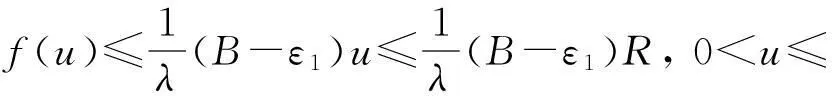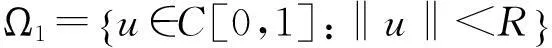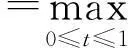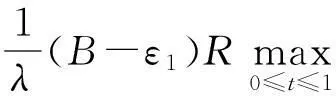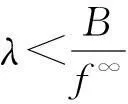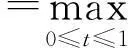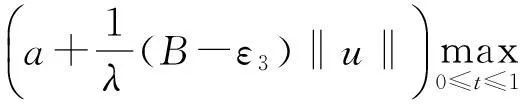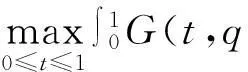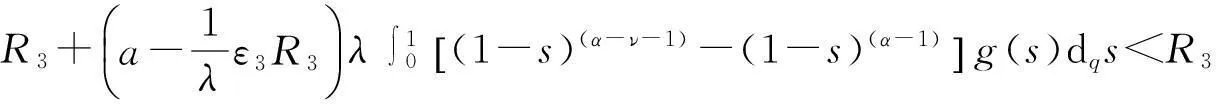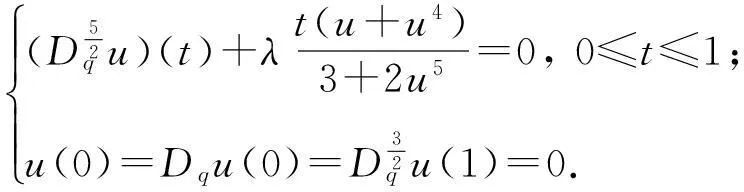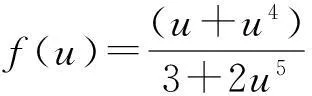一类带有参数的q-差分方程边值问题正解的存在性

一类带有参数的q-差分方程边值问题正解的存在性

孙明哲，侯成敏

( 延边大学理学院 数学系, 吉林 延吉 133002 )

摘要：研究了一类带有参数的q-差分方程正解的存在性.首先给出了该问题解的表达式，并分析了格林函数的性质，然后利用Banach空间锥上的不动点指数定理得到了当参数λ属于不同范围时正解存在性的充分条件，最后用具体实例验证了文中的结论.

关键词：q-差分方程； 边值问题； 参数; 不动点定理

收稿日期：2015-02-20

基金项目：吉林省教育厅“十二五”科学技术研究项目(吉教科合字[2014]第20号);国家自然科学基金资助项目(11161049)

文章编号：1004-4353(2015)02-0124-05

中图分类号：O175.8

Existence of positive solutions of q-differences equations with parameter

SUN Mingzhe,HOU Chengmin

(DepartmentofMathematics，CollegeofScience，YanbianUniversity，Yanji133002，China)

Abstract：We study the existence of positive solutions for the q-differences equation with parameter. Firstly, the expression of solutions is presented, and some characteristics of the Green function were analyzed. Secondly, by applying fixed point index theorem of Banach spaces cone, we obtained sufficient conditions for the existence of the positive solutions when parameters belong to different ranges. Finally, the main results were illustrated with some examples.

Key words： q-differenceequation; boundary value problem; parameter; fixed point theorem

1910年，Jackson引入了q-微积分概念[1]，之后由Al-Salam和Agarwal给出了分数阶q-微积分的基本概念和基本性质[2].近年来，分数阶q-差分边值问题作为新的研究方向受到国内外学者的关注,并取得了一些研究成果[3-8]，但对于含有分数阶边值条件的边值问题报道的很少.本文将探讨带有参数和分数阶边值条件的q-差分边值问题

本文假设下列条件成立：

(H1) f∶[0,1]→[0,+∞)为连续函数;

(H2) g∶[0,1]→[0,+∞)为连续函数，且在[0,1]的任意子区间上不恒为零;

1主要结果及其证明

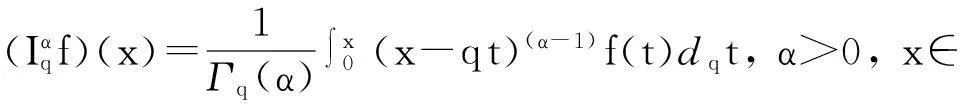
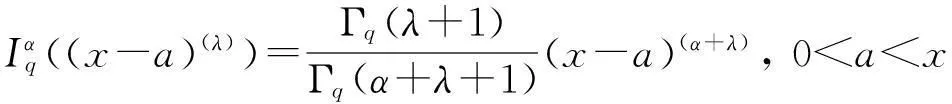
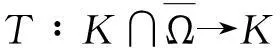
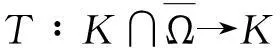
定理1设2<α<3, 1<ν<2,u(t)是问题(1)和(2)的解的充要条件是它具有以下形式:

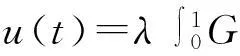
(3)

证明假设u(t)是问题(1)的解,则有

(4)

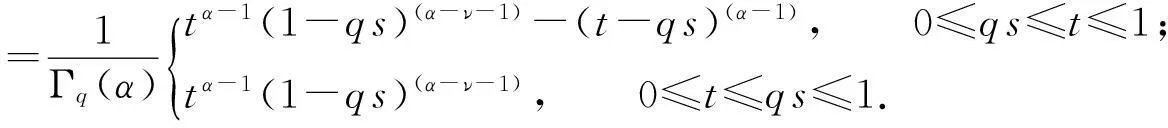
反之,如果u(t)满足式(3),不难推出u(t)是问题(1)和(2)的解.证毕.

注1[7]若α>0,a≤b≤t,则(t-a)(α)≥(t-b)(α).

定理2格林函数G(t,qs)具有以下性质：

① 0≤G(t,qs)≤G(1,qs),对一切s,t∈[0,1];

②G(t,qs)≥k(t)G(1,qs),对一切s,t∈[0,1],这里k(t)=tα-1.

证明记g1(t,qs)=(1-qs)(α-ν-1)tα-1-(t-qs)(α-1), 0≤qs≤t≤1,g2(t,qs)=(1-qs)(α-ν-1)tα-1, 0≤t≤qs≤1.

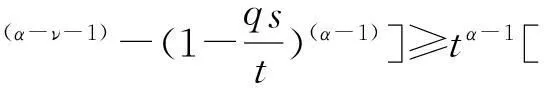
2) 当0≤qs≤t≤1时,

当0≤t≤qs≤1时,

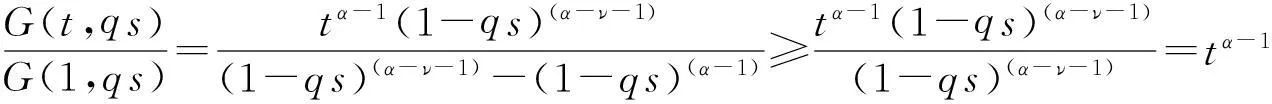
从而可得,当t,s∈[0,1]时,有G(t,qs)≥tα-1G(1,qs).证毕.

(5)

由定理1知,问题(1)和(2)有解当且仅当算子T有不动点.

定理3算子T∶P→P是完全连续的.

证明由函数G,f,g的连续性可知算子T∶P→P是连续的，再由定理2得

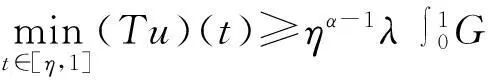
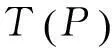
对于任意t1,t2∈[0,1]且t1

为了方便,定义如下记号：

(6)

因此,对于任意u∈P∩∂Ω1, 0<μ≤1,当‖u‖=R时,μTu≠u成立.于是算子T在u∈P∩∂Ω1上满足引理1的条件,即

i(T,P∩Ω1,P)=1.

(7)

i(T,P∩Ω2,P)=0.

(8)

因此,对于任意u∈P∩∂Ω3, 0<μ≤1,当‖u‖=R3时,μTu≠u.于是算子T在P∩∂Ω3上满足引理1的条件,即

i(T,P∩Ω3,P)=1.

(9)

i(T,P∩Ω4,P)=0.

(10)

2应用举例

例1考虑边值问题

(11)

参考文献：

[1]JacksonFH.q-differenceequationsAmer[J].JMath, 1910,32(4):305-314.

[2]Al-SalamWA.Somefractionalq-integralsandq-derivatives[J].ProcEdinbMathSoc, 1966,15(2):135-140.

[3]YangW.Positivesolutionsforboundaryvalueproblemsinvolvingnonlinearfrationalq-differenceequations[J].DifferEquAppl, 2013,5:205-219.

[4]ZhangQ.Existenceandmultiplicityofpositivesolutionsfornonhomogeneousboundaryvalueproblemswithfractionalq-derivative[J].BoundValueProbl, 2013,2013(1):1-16.

[5]AgarwalRP,AhmadB,AlsaediA,etal.Onnonlinearfractionalq-differenceequationsinvolvingtwofractionalorderswiththree-pointnonlocalboundaryconditions[J].DynContinDiscreteImpulsSystSerAMathAnal, 2014,21:135-151.

[6]EL-ShahedM.Nontrivialsolutionsforfractionalq-differenceboundaryvalueproblems[J].ElectronJQualTheoryDifferEqu, 2010,70(10):1-10.

[7]孙明哲,侯成敏.一类反周期分数阶q-差分边值问题解的存在性[J].吉林大学学报:理学版,2014,52(6):1215-1218.

[8]LiXinhui,HanZhenlai,SunShurong.Existenceofpositivesolutionsofnonlinearfractionalq-differenceequationwithparameter[J].AdvancesinDifferenceEquationsAugust, 2013,2013(1):1-13.

[9]GuoD,SunJ.Calculationandapplicationoftoplogicaldegree[J].JournalofMathmaticalResearchandExposition, 1988,8(3):469-480.