Blow-up流形的Gromov-Witten不变量的一个为零定理*
2012-05-10戚晓霞
戚晓霞
(中山大学数学与计算科学学院,广东 广州510275)

1 Gromov-Witten不变量及其退化公式
1.1 GW-不变量
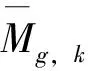


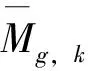
c1(A)+(n-3)(1-g)+k
(1)
其中c1是X的第一陈类。
给定α1,…,αk∈H*(X),定义GW-不变量为
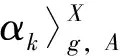
其中{αi}应满足维数条件:∑degαi=2c1(A)+2(n-3)(1-g)+2k,否则定义不变量为0。
1.2 相对GW-不变量
设D⊂X为X中一个光滑除子,即一个余维-2的辛子流形,且D是J-全纯的,即J(TD)⊂TD。给定A∈H2(X)满足A·D≥0,则相对J-全纯曲线的模空间为
u*[Σ]=A,Σμi=A·D,
u-1(D)=Σμiyi,μ=(μ1,…,μr)}
c1(A)+(n-3)(1-g)+k+r-A·D
(2)
给定α1,…,αk∈H*(X),β1,…,βr∈H*(D),定义相对GW-不变量为

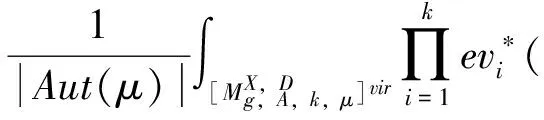

1.3 辛切割及退化公式

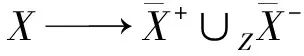
使得π|XH-1(0)=id,并且π|H-1(0)是商映射H-1(0)→Z。考虑
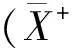
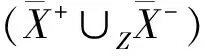

命题1[11-12]


(3)

命题2[11]
dimCM++dimCM-=dimCM+(n-1)l(μ)
(4)
2 主要结果及其证明
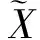


其中n≥3,r>0,p!=PD∘P*∘PD。
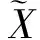
⊕O),≅
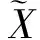



(5)
其中μ=(μ1,…,μl(μ))是E·(p!(A)+re)的一个划分,{βj}是H*(E)的任一组自对偶基底。这里E+应该被看作是P1-丛PE(O(-1)⊕O)的无穷截面,而E+≅E-≅E。
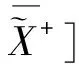
(p!(A)+re)+=aL+be,a≥0,b≥0

∑μi=H·(p!(A)+re)+=a
(6)
因为E+·(p!(A)+re)+=-r,于是
-r=E·(p!(A)+re)+=a-b
(7)
因此由(6)-(7)式得,b=∑μi+r,所以(p!(A)+re)+=∑μiL+(∑μi+r)e。
(1-g+)+k+l(μ)-∑μi=
[(n+1)H-(n-1)E]·[∑μiL+(∑μi+r)e]+
(n-3)(1-g+)+k+l(μ)-∑μi=
n∑μi+(n-1)r+(n-3)(1-g+)+k+l(μ)
不妨假设
∑
如果0

另一方面,由维数关系(4)得
(n-2)l(μ)-n∑μi=
n(l(μ)-∑μi)-2l(μ)<
由GW-不变量的定义,(5)式右边每一项的第二个不变量都为零,因此(5)式为零。 □
注1 我们同时证明了n=2的情况,即当W(α)满足0 注2 定理1是对文[10](Proposition3.1)的推广,我们这里证明了对任意辛流形以及g≥0的结果,并且当g=0时,扩展了W(α)的取值范围。 参考文献: [1]RUAN Y.Topological sigma model and Donaldson-type invariants in Gromov theory[J].Duke Math J,1996,83 (2): 461-500. [2]RUAN Y,TIAN G.A mathematical theory of quantum cohomology[J].J Diff Geom,1995,42(2):259-367. [3]BEHREND K.Gromov-Witten invariants in algebraic geometry[J].Invent Math,1997,127 (3): 601-617. [4]FUKAYA K,ONO K.Arnold conjecture and Gromov-Witten invariant[J].Topology,1999,38 (5): 933-1048. [5]LI J,TIAN G.Virtual moduli cycles and Gromov-Witten invariants of algebraic varieties[J].J Amer Math Soc,1998,11 (1): 119-174. [6]LI J,TIAN G.Virtual moduli cycles and Gromov-Witten invariants of general symplectic manifolds [G].Topics in Symplectic 4-manifolds (Irvine,CA,1996),First Int Press Lect Ser,I,Int Press,Cambridge,MA,1998: 47-84. [7]RUAN Y.Virtual neighborhoods and pseudo-holomorphic curves[J].Tr J Mathematics,1999,23: 161-231. [8]LERMAN E.Symplectic cuts[J].Math Res Lett,1995,2: 247-258. [9]HU J.Gromov-Witten invariants of blowups along points and curves[J].Math Z,2000,233: 709-739. [10]GATHMANN A.Gromov-Witten invariants of blow-ups[J].J Alg Geom,2001,10: 399-432.arXiv:math/9804043v2 [11]LI A,RUAN Y.Symplectic surgery and Gromov-Witten invariants of Calabi 3-folds[J].Invent Math,2001,145 (1): 151-218. [12]HU J,LI T,RUAN Y.Birational cobordism invariance of uniruled symplectic manifolds[J].Invent math,2008,172: 231-275.
