Simplified permeable surface correction for frequency-domain Ffowcs Williams and Hawkings integrals

Zhiteng Zhou ,Hongping Wang ,Shizhao Wang ,∗

a The State Key Laboratory of Nonlinear Mechanics, Institute of Mechanics, Chinese Academy of Sciences, Beijing 100190, China

b School of Engineering Sciences, University of Chinese Academy of Sciences, Beijing 10 0 049, China

ABSTRACT A simplified surface correction formulation is proposed to diminish the far-field spurious sound generated by the quadrupole source term in Ffowcs Williams and Hawkings (FW-H) integrals.The proposed formulation utilizes the far-field asymptotics of the Green’s function to simplify the computation of its high-order derivatives,which circumvents the difficulties reported in the original frequency-domain surface correction formulation.The proposed formulation has been validated by investigating the benchmark case of sound generated by a convecting vortex.The results show that the proposed formulation successfully eliminates the spurious sound.The applications of the proposed formulation to flows with some special parameters are also discussed.

Keywords:FW-H integral Surface correction Quadrupole source Aeroacoustics

The Ffowcs Williams and Hawkings (FW-H) equation extends Lighthill’s acoustic analogy method to flows with moving solid/permeable boundaries,resulting in an inhomogeneous wave equation that includes monopole,dipole and quadrupole sources on the right hand side.The solution to the FW-H equation can be expressed as integrals of the sources (hereinafter referred to as the FW-H integrals) by using the Green’s function.The integrals of monopole and dipole sources are surface integrals and that of quadrupole sources is a volume integral [1] .

The computation of the volume integral is much more challenging than that of surface integrals [2,3].In computing the far-field sound generated by low Mach number flows,the volume integral is usually ignored with the assumption that the sound is dominated by the monopole and dipole sources.However,recent researches find that the ignoration of the quadrupole sources may generate spurious sound even though the flow is at low Mach numbers [4–8] .

Different methods have been proposed to eliminate the spurious sound associated with the quadrupole sources [5,9,10].In particular,Wang et al.[11] found that the spurious sound is caused by the eddy crossing the surface of the integral domain.Then a new surface integral (hereinafter referred to as surface correction) is proposed to correct the contribution of quadrupole sources to the far-field sound.The surface correction formulation is constructed with the assumption that the eddy is frozen when it is convected across the integral surface.The convection velocity is usually computed by scaling the freestream velocity [11] .The spurious sound can be correctly eliminated as long as the eddy leaves the integral surfaces with relatively uniform velocity [11] .The surface correction formulation is then improved by Nitzkorski et al.[12],Rahier et al.[8] and Ikeda et al.[4] by taking into account the effects of non-uniform convection velocity.The above surface correction formulation is derived in a convective frame of reference and employed in the time-domain method for the FW-H integrals.The application of the above surface correction to the frequencydomain method is usually not straight forward,because most of the frequency-domain methods are derived in a laboratory frame of Ref.[11] .

Ikeda et al.[13] examined the far-field approximation for the surface correction between the laboratory frame of reference and convective frame of reference and derived a frequency-domain formulation via a Fourier transform of the time-domain formulation.Lockard and Casper [12] proposed an alternative method to derive the surface correction formulation in the frequency domain.This alternative method converts the volume integral into a series of surface integrals by repeatedly using the formulation of integration by parts.The proposed surface correction is validated by computing the sound generated by a 2D convecting vortex.The advantage of this method is that the surface correction formulation is directly derived in the frequency domain without referring to the surface correction formulation in the time domain.The disadvantage is that the surface correction formulation involves high-order derivatives of the Green’s function.The computation of the highorder derivatives of the Green’s function are quite complicated and nontrivial [14].

The objective of this letter is to propose a simplified surface correction formulation based on the work of Lockard and Casper[14].We simplify the computation of the high-order derivatives of the Green’s function based on its far-field approximations and validate the proposed formulation by using the 2D convecting vortex.

The solution to the FW-H equation in the frequency domain can be given by the FW-H integrals as follows

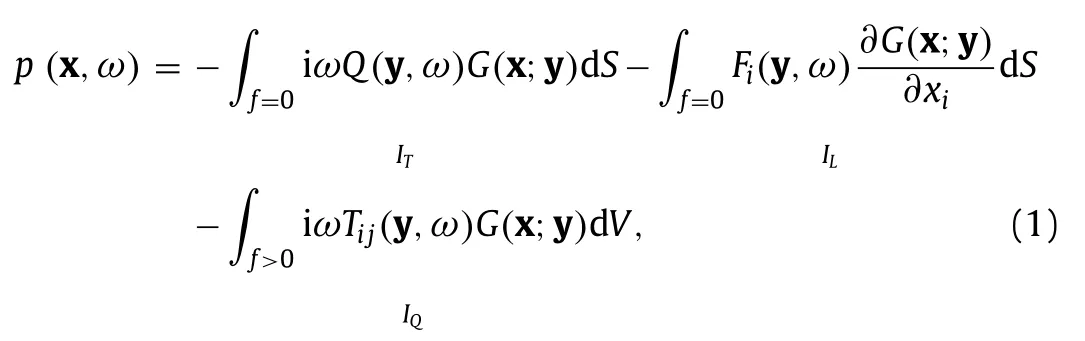
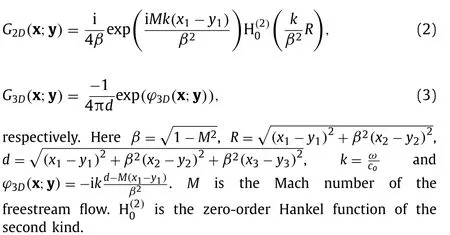
where theITandILterms are the surface integrals of monopole sources and dipole sources,respectively,IQterm is the volume integral of quadrupole sources.is theith component of the velocity of the fluid,Uiis theith component of the freestream velocity,Pij=(p−p0)δij−τijis the compressive stress tensor andτijis viscous stress tensor.Here,we ignore the viscous part according to the work of Lockard and Casper[14].fis a level set function wheref=0 indicates the FW-H surface andf>0 is the region outside the FW-H surface.njis the unit normal of the FW-H surface.ρo,coandporepresent the density,speed of sound and pressure in the background flow,respectively.ρ′=ρ−ρoandp=p−poare perturbations of density and pressure,respectively.In accordance with the work of Lockard and Casper [14],we set theo−y1direction as the freestream flow direction.Hence,the Green’s functionsGin Eq.(1) for 2D and 3D flows are

The termsITandILin Eq.(1) are surface integrals.IQis the volume integral over the regionf>0.The computation ofIQis much more challenging thanITandIL,because the Lighthill stress tensor at all grid points within the regionf>0 is needed to be experimentally measured or numerically simulated.Usually,the domainf>0 extends to far downstream of the wake for high Reynolds number flows.It is very difficult to measure or compute the large domain off>0 in flows of practical interest.To compute the contribution of the quadrupole term efficiently,many efforts have been devoted to transforming the quadrupole term from a volume integral to surface integrals [10,11,13].The frequency-domain surface correction proposed by Lockard and Casper [14] is as follows,

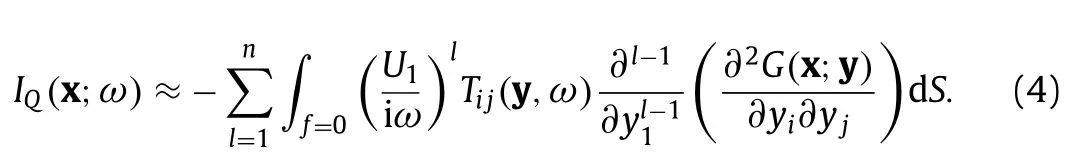
To ensure the accuracy of the series,n≥2 is necessary [14].However,as pointed out by Lockard and Casper [14],the computation of high-order derivatives of the Green’s function is quite complicated.They refer to the work of Gloerfelt et al.[15] to compute the second derivatives of the Green’s function in two dimension and use a symbolic algebra package to obtain the higher-order derivatives.

We propose a simplified surface correction formulation as follows to diminish the spurious far-field sound generated by the eddy crossing the FW-H integral surface,

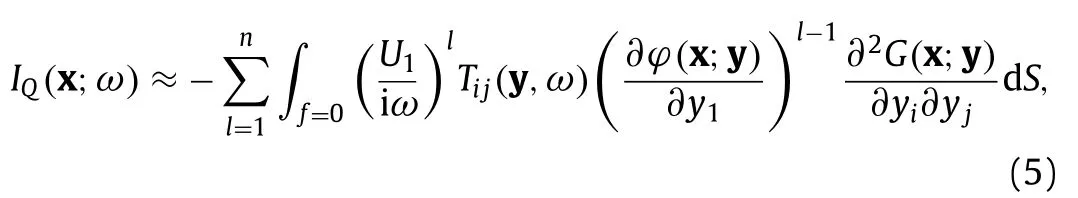
whereG=G3D,ϕ=ϕ3Dfor 3D flows andG=G2D,ϕ=for 2D flows.G2Dis the asymptotic Green’s function in 2D space

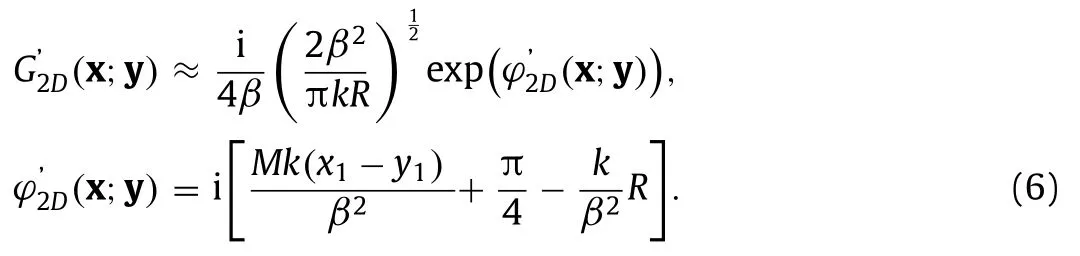
Equation (5) employs the approximations as follows to simplify the computation of the high-order derivatives of the Green’s function at the far-field

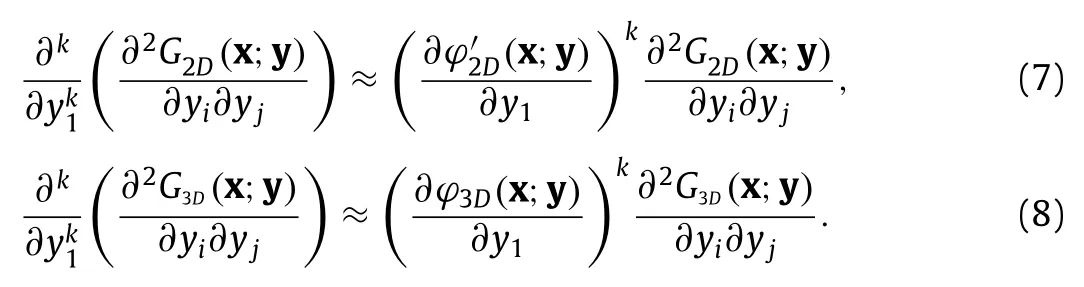
The proof of Eq.(7) is briefly reported as follows.Thekth-order derivative ofG2Dwith respect toy1is

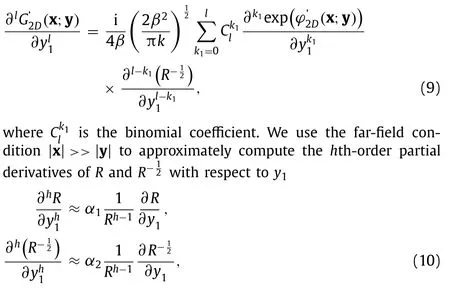
whereh≥1.In the far field,α1andα2areO(1)and independent ofR[16].By using the first line of Eq.(10),thes1-order derivative of exp(ϕ(x ;y))is approximated as follows

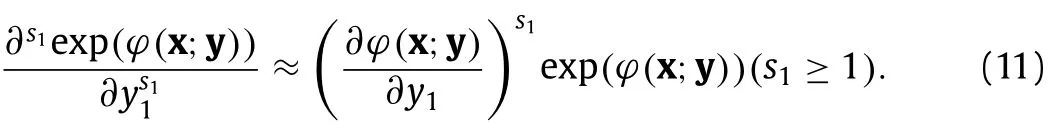
Equation (7) is obtained by ignoring the small terms in Eq.(9) according to Eqs.(10) and (11).More details of the proof can be found in the supplementary material [14].Thus,Eq.(7) is proved.Eq.(8) can be proved by using the similar method.Compared with Eq.(4),the computation of the high-order derivativesare replaced by.The computation of high-order derivatives of the Green’s function is circumvented.

Fig.1.Schematics of the FW-H surface position and pressure contours of the vortex.

In accordance with the work of Lockard and Casper [14],we validate the simplified surface correction formulation (Eq.(5)) by using the benchmark case of spurious sound generated by a 2D convecting vortex crossing the FW-H integral surface.The normalized pressure and velocity of the 2D convecting vortex is given by[12]

A permeable square with each side of 10 unit lengths centered at origin is used as the FW-H integral surface,as shown in Fig.1.The convecting vortex moves at the Mach number ofMa=0.2.The observer location is at(100,0)in the downstream of the convecting vortex.A spurious sound is generated when the vortex crosses through the FW-H surface.The spurious sound can be computed by usingIS=IT+ILfor this special flow,because sound pressure approaches to zero exponentially in the far-field.The negative of the spurious sound pressure generated by the vortex crossing the FW-H interface is plotted in Fig.2.The surface corrections computed by using Eq.(5) withn=1,2 and 3 are also plotted in Fig.2.The spurious sound pressure is correctly diminished by the proposed simplified surface correction withn≥2.The result is consistent with that of Lockard and Casper [14].

We also compared the details of the simplified surface correction (Eq.(5)) with the original surface correction (Eq.(4)) proposed by Lockard and Casper [14].The surface corrections computed by using these two equations are plotted in Fig.3.The results show that the surface corrections computed by using the simplified surface correction (Eq.(5)) are in good agreement with the corresponding results of the original surface correction (Eq.(4)) withn=1,2 and 3 at the downstream observer (100,0).Figure 4 plots the surface correction computed by Eq.(5) withn=2 and 3 at different downstream observers when the non-dimensional time ist=120.The distance between the downstream observer and the initial vortex center is denoted byR.It is observed that the surface correction computed by using Eq.(5) withn=2 and 3 can correctly diminish the spurious sound whenR>60.The results show that the simplified surface correction proposed by this work is valid for computing the far-field sound.

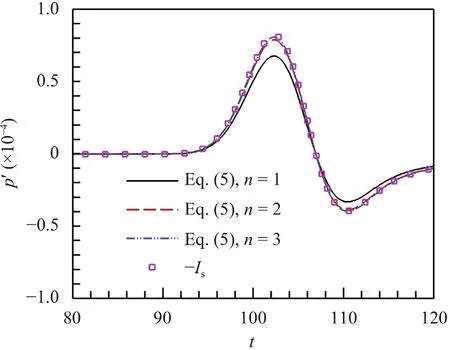
Fig.2.Negative of the spurious sound pressure and the correction computed by using the proposed simplified surface correction for the sound generated by a convecting vortex crossing the FW-H integral surface.

The simplified surface correction formulation (Eq.(5)) not only provides a simplified surface correction but also helps to identify the limitations of the previous surface correction.As pointed out by Lockard and Casper [14],the series of surface integrals of Eq.(4) is divergent when the convecting vortex moves atMa=0.6.The divergence of the series can be clearly inferred from Eq.(5),since Eq.(5) is the approximation of Eq.(4) at the far field.We note that Eq.(5) is a geometric series with a ratio of.The series is divergent when>1.Therefore,the divergence of Eq.(4) may occur when the convection velocity is larger than the phase velocity

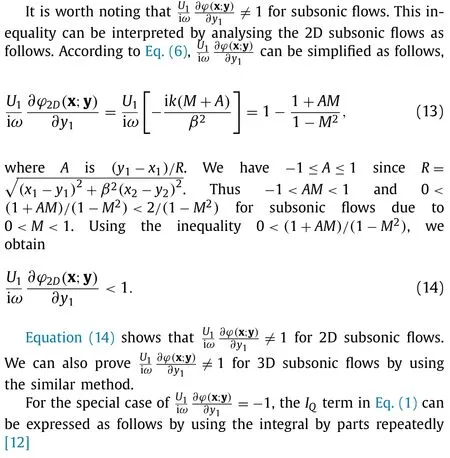
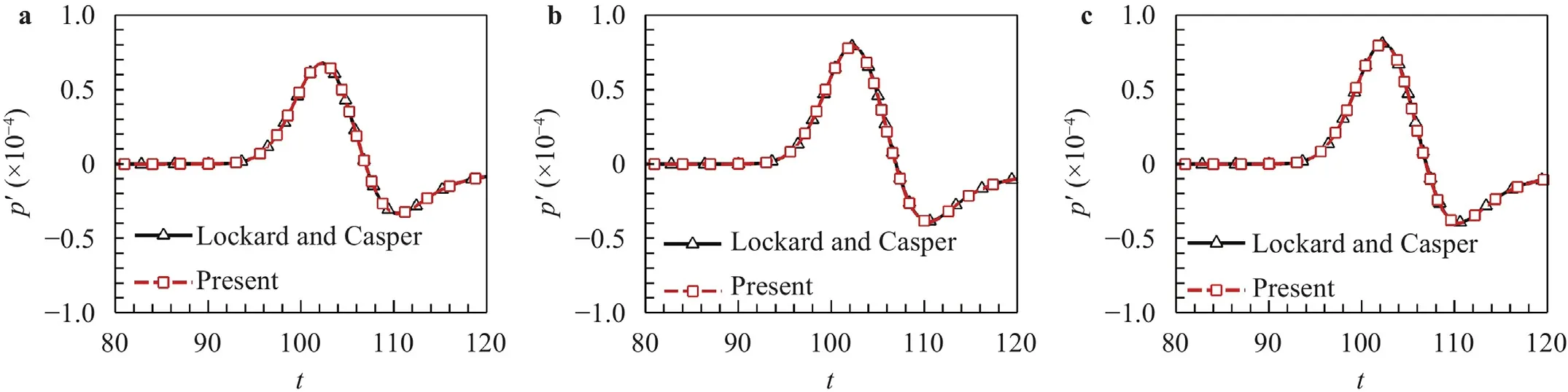
Fig.3.Comparison between the simplified surface correction proposed in the present work (Eq.(5)) and the surface correction proposed by Lockard and Casper [12] (Eq.(4))with a n=1, b n=2, c n=3.

Fig.4.The instantaneous surface correction at the downstream observer at t=120.

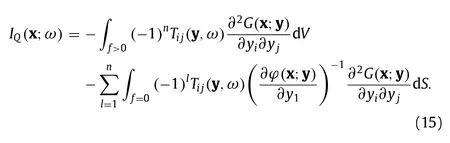
Whennis even,Eq.(15) reduces to the identityIQ=IQ.Whennis odd,Eq.(15) reduces to

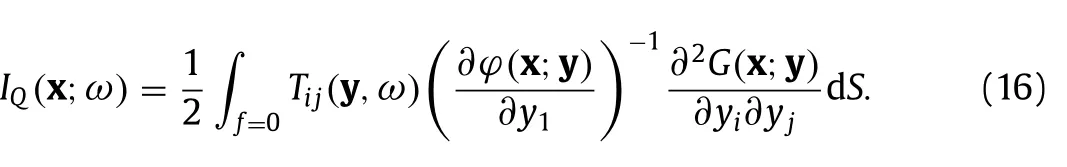
Equation (16) is much simpler than Eq.(5) for the surface correction,because Eq.(16) consists of only one surface integral instead of the series of surface integrals in Eq.(5).Equation (16) can be utilized for surface correction for the special case of=−1.

It is also worth noting that another much simpler surface correction formula consisted of only one surface integral for surface correction can be derived for the special case of nearly uniform Lighthill stress distributionnear the FW-H integral surface.The simplified surface correction for this special case is

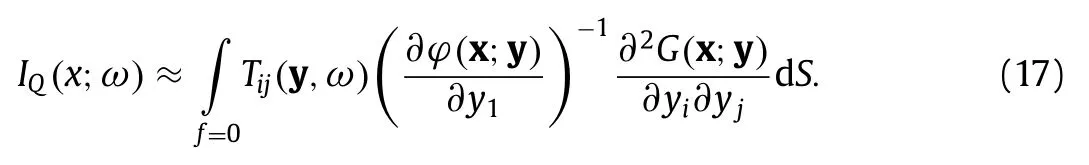
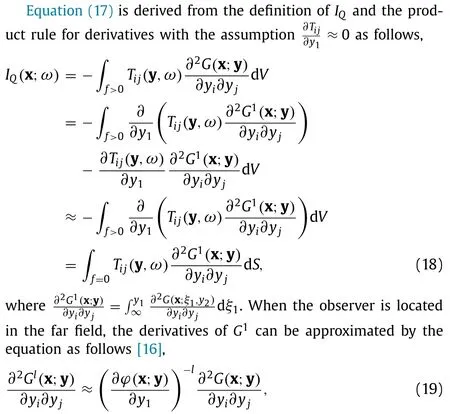
withl=1.More detailed derivation of Eq.(19) can be found in the supplementary material [14].Combination of Eq.(19) with Eq.(18) results in the simplified surface correction Eq.(17).

We have proposed a simplified surface correction formulation for the quadrupole source term of the Ffowcs Williams and Hawkings integrals in frequency domain.The proposed surface correction consists of a series of surface integrals and is applicable to eliminating the spurious sound at far field.The simplified formulation improves the original surface correction by circumventing the difficulties in computing the high-order derivatives of the Green’s function.An easy-to-use expression is derived to compute the high-order derivatives by referring tothe far-field asymptotic of the Green’s function.The spurious sound generated by a convecting vortex crossing the FW-H integral surface is investigated to validate the proposed surface correction formulation.The results show that the proposed formulation can successfully eliminate the spurious sound generated by the quadrupole term.The terms of the simplified surface correction formulation are consistent with these of the original surface correction formulation at the far field.The proposed formulation also helps to analyse the failure of the original surface correction formulation and further simplify the surface correction to a formulation with only one surface integral when the magnitude of the convection velocity equals to the phase velocityor the flow with nearly uniform Lighthill stress distribution(≈0)near the FW-H integral surface.

Declaration of Competing Interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Acknowledgment

This work was supported by the National Natural Science Foundation of China Basic Science Center Program for "Multiscale Problems in Nonlinear Mechanics" (Grant 11988102),the National Natural Science Foundation of China (Grants 11922214 and 91952301),and the National Numerical Windtunnel project.