The singularity in the state-based peridynamic solution of uniaxial tension
2018-11-24ZaixingHuang
Zaixing Huang
State Key Laboratory of Mechanics and Control of Mechanical Structures, Nanjing University of Aeronautics and Astronautics, Nanjing 210016, China
Keywords:Peridynmics Non-ordinary state-based peridynamics Singularity Local uniaxial tension Analytical solution
ABSTRACT We solve the local uniaxial tension of an infinite rod in the framework of non-ordinary state-based peridynamics. The singular solutions of stress and displacement are acquired. When the influencing range of the window function approaches zero, these two solutions will return to the solutions of the classical elasticity. The analysis shows that the singularities of the solutions stem from such a feature of the window function that must be represented by a rapidly decreasing function in physics. Contrary to the classical elasticity, the stress solution of peridynamics is smoother than the displacement solution. In addition, a criterion used to select the window function is proposed in this paper.
Peridynamics [1, 2] is a new continuum mechanics theory. In its framework, the gradient of displacement and relevant quantities are no longer concerned. Therefore, peridynamics can be used to conveniently and effectively analyze deformation companied with evolution of discontinuities such as damage, fracture and impact breakage [2-4]. However, when peridynamics is applied to analyze the static equilibrium problem with infinite domain, it will cause the singularity of solutions [5, 6]. So far,these solutions are all solved based on the bond-based peridynamics. Then, will similar singularity occur in the solution of state-based peridynamics? To answer this question, we analyze the local uniaxial tension based on non-ordinary state-based peridynamics.
In peridynamics, most of problems are solved based on numerical algorithm. So far, only a few analytical solutions are acquired for some simple benchmark problems such as uniaxial tension and longitudinal vibration of rod. Silling et al. [7] analyzed the deformation of an infinite rod subjected a simple-point load and gave a divergent formal solution. Weckner et al. [8, 9]found the Green's functions of 1- and 3-dimensional peridynamics with help of the Laplace and Fourier transforms. By these Green's functions, they gave an integral representation of 3-dimensional peridynamic solution. Using the similar approach,Mikata [5] investigated the peristatic and peridynamic analytical solutions of a 1-dimensional infinite rod. These solutions are represented as the sum of the Dirac delta functions and a convergent integral. Further, Wang et al. [6] proved that the Green's functions are uniformly expressed as a conventional solution plus a Dirac function and a convergent nonlocal integral. In addition, Nishawala and Ostoja-Starzewski [10] developed the inverse method to solve the 1- and 2-dimensional static equilibrium problems in peridynamics.
Recently, Bazant et al. [11] discussed improvable physical aspects of peridynamics through analyzing the dispersion of wave.As a response, Butt et al. [12] proposed that the strong disper-sion of peridynamic wave can be minimized by choosing a combination of the size of the peridynamic horizon and the shape of the influence function in a way that the peridynamic solution approaches the solution of classical elasticity.
Up to present, the analytical solutions of static equilibrium problems are all acquired form the bond-based peridynamics. In the framework of the non-ordinary state-based peridynamics,the study on the relevant problems is still lacking. So we will firstly solve the local uniaxial tension based on non-ordinary state-based peridynamics.
The outline of the paper is as follows. We introduce the basic equations of 1-dimensional non-ordinary state-based peridynamics. A criterion used to chose the window function is proposed. Then we solve the local uniaxial tension of an infinite rod based on non-ordinary state-based peridynamics. The analytical solutions of stress and displacement are acquired, and the origin of the solution singularity is analyzed. Finally, we close this paper with a brief summary.
Consider the case of small displacement, the governing equation of one dimensional non-ordinary state-based peridynamics can be simplified as [3, 4]


In the non-ordinary state-based peridynamics, the constitutive relation between stress and deformation is the same as that of the classical continuum in mathematical form, but the deformation tensor or strain tensor involved in peridynamic constitutive relation must be replaced with the peridynamic deformation gradient. The 1-dimensional form of the peridynamic deformation gradient can be written as

In terms of the Hooke's law, so the 1-dimensional linear elastic peridynamic constitutive equation is represented as follows
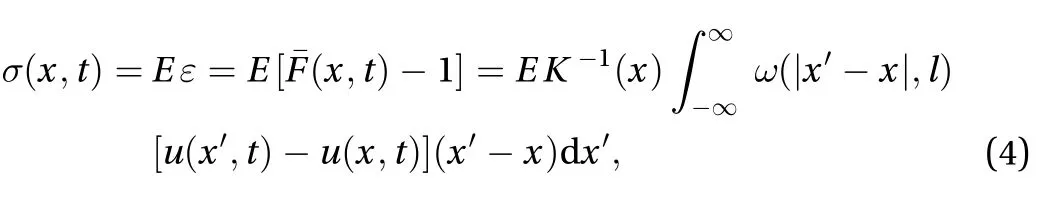

The local uniaxial tension of infinite rod is defined as the tension of a finite segment between two points in the infinite rod, as shown in Fig. 1.
In terms of the criterion represented by Eq. (5), the window function can be given as follows


Fig. 1. Local uniaxial tension of an infinite rod.

By the inverse Fourier transform of Eqs. (12) and (14), the general solutions of stress and displacement in the local uniaxial tension can be represented as


Equations (20) and (21) represent the stress solution and the displacement solution of the local uniaxial tension problem. Unfortunately, they are divergent, and this divergence is inevitable if only the window function is a rapidly decreasing function. The reason that the window function is represented by a rapidly decreasing function consists in: a rapidly decreasing function can be used to correctly describe the physical fact that the longrange interaction approaches zero with the distance increasing;meanwhile, Eq. (5) also requires that the window function must be a rapidly decreasing function. However, the Fourier transform of a rapidly decreasing function is a smooth and bounded function [13]. This results in the divergence of the peridynamic solutions.
In terms of the procedure in Refs. [8, 9], the divergent integral in Eqs. (20) and (21) can be decomposed into a singular function or a function with singular value plus a convergent integral representation. That is

Comparing Eq. (22) with Eq. (23), we find that the stress solution is smoother than the displacement solution. This is an anomalous result relative to the classical elasticity. The reason consists in Eq. (3), which shows that the stress is determined by a weight integral of relative displacement. On the contrary, in the classical elasticity the stress is governed by the gradient of displacement.
Whether in the bond-based peridynamics or in the statebased peridynamics, there exist the singularities of the stress solution and the displacement solution in the static equilibrium problem with infinite domain. The occurrence of these singularities is inevitable if only the window function is represented by a rapidly decreasing function. Contrary to the classical elasticity,the stress solution of peridynamics is smoother than the displacement solution.
Acknowledgements
The author is grateful for the support of this work by the National Natural Science Foundation of China (11672129) and the Research Fund of State Key Laboratory of Mechanics and Control of Mechanical Structures (Nanjing University of Aeronautics and Astronautics, MCMS-I-0218G01).
杂志排行
Theoretical & Applied Mechanics Letters的其它文章
- Continuum percolation of porous media via random packing of overlapping cube-like particles
- Traffic flow and safety analysis
- Air entrainment in a vertical dropshaft with limited air supply
- Intrinsic relationship of vorticity between modes A and B in the wake of a bluff body
- An improved lattice Boltzmann model for high gas and liquid density ratio in composite grids
- A visualized study of interfacial behavior of air–water two-phase flow in a rectangular Venturi channel
