解析Besov型空间在Bloch空间的闭包
2018-01-29钱睿深
钱 睿 深
(岭南师范学院 数学与统计学院,广东 湛江 524048)
1 引 言
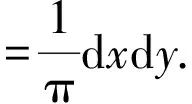
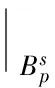

令Bloch空间B表示满足下列条件的全体函数f∈H(D):


2 主要结果
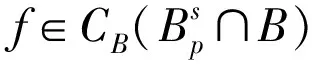

(1-|z|2)|f′(z)|≤(1-|z|2)|f′(z)-g′(z)|+(1-|z|2)|g′(z)|,
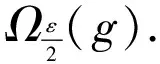
另一方面,设f∈B.由文献[11,引理4.2]知,
令
以及
那么f(z)=f1(z)+f2(z).现在将证明f1∈B.因为f2(z)=f(z)-f1(z),所以
即f2∈B,其中,C为正常数.从而,易知f1∈B.

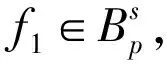
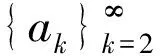
称θ(z)为Blaschke乘积.




所以对于任意的ε>0,可以推出

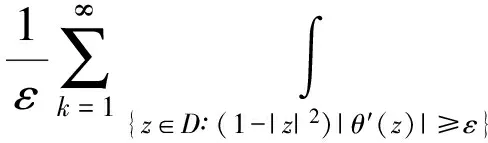
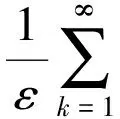
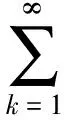


因此,

由文献[12,69页],有
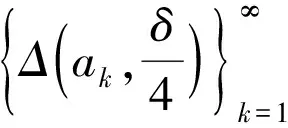
证毕.
[1] N. Arcozzi, D. Blasi and J. Pau, Interpolating sequences on analytic Besov type spaces[J].Indiana Univ Math J,2009,58:1281-1318.
[2] J Anderson, J Clunie , Ch Pommerenke, On Bloch functions and normal functions[J]. J Reine Angew Math, 1974,270:12-37.
[3] D. Blasi and J. Pau, A characterization of Besov-type spaces and applications to Hankel-type operators[J]. Michigan Math J,2008,56: 401-417.
[4] L Carleson, An interpolation problem for bounded analytic functions[J]. Amer J Math,1958,80:921-930.
[5] L Carleson, On the zeros of functions with bounded Dirichlet integrals[J]. Math Z,1958,56:289-295.
[6] L Carleson, Interpolation by bounded analytic functions and the Corona problem[J]. Ann of Math,1962,76:547-559.
[7] P Galanopoulos, N. Monreal Galan and J. Pau, Closure of Hardy spaces in the Bloch space[J]. J Math Anal Appl.,2015,429:1214-1221.
[8] D Girela, J. A. Pelaez and D. Vukotic, Integrability of the derivative of a Blaschke product[J]. Proc Edinb Math Soc,2007,50:673-687.
[9] N Monreal Galan and A. Nicolau, The closure of the Hardy space in the Bloch norm[J]. Algebra i Analiz. 2010,22:75-81, translation in St. Petersburg Math J,2011,22:55-59.
[10] ZHAO R. Distances from Bloch functions to some Mobius invariant spaces[J]. Ann. Acad Sci Fenn Math,2008,33:303-313.
[11] ZHU K. Bloch type spaces of analytic functions[J]. Rocky Mountain J Math,1993,23:1143--1177.
