氧缺陷TiO2-B作为可充电锂离子电池负极材料的第一性原理研究

孔令明　祝宝林　庞先勇,*　王贵昌

(1太原理工大学化学化工学院，太原030024；2南开大学化学系，先进能源材料教育部重点实验室，天津300071;3中国科学院煤炭化学研究所，煤转化国家重点实验室，太原030001)

氧缺陷TiO2-B作为可充电锂离子电池负极材料的第一性原理研究

孔令明1祝宝林1庞先勇1,*王贵昌2,3,*

(1太原理工大学化学化工学院，太原030024；2南开大学化学系，先进能源材料教育部重点实验室，天津300071;3中国科学院煤炭化学研究所，煤转化国家重点实验室，太原030001)

利用对氧缺陷的TiO2-B材料进行密度泛函理论的计算，阐述了氧空穴对于TiO2-B材料的电化学性质的影响。计算研究主要聚焦于缺陷材料的锂离子迁移和电子导电性等基本问题。计算结果表明在低锂离子浓度下(x(Li/Ti)≤0.25)，相比于无缺陷的TiO2-B，氧缺陷TiO2-B有着更高的插入电压和更低的b轴方向迁移活化能，意味着锂离子的嵌入也更容易，这对于可充电电池的充电过程是有利的。而在高浓度下(x(Li/Ti)=1)，锂饱和的氧缺陷TiO2-B相较于无缺陷的TiO2-B有着较低的插入电压，更有利于锂离子的脱嵌过程，这对于可充电电池的放电过程也是有利的。电子结构计算表明缺陷材料的禁带宽度在1.0－2.0eV之间，低于无缺陷的材料的3.0eV。主要态密度贡献者是Ti-Ov-3d，并且随着氧空穴的增加它的强度也变得更强。这就表明氧缺陷TiO2-B有更好的电子导电性。

TiO2-B；氧空穴；插入电压；迁移活化能；禁带宽

1 Introduction

Rechargeable Li-ion batteries(LIBs)are receiving large-scale applications in portable digital devices,energy storage instruments,and hybrid electric vehicles1－3.The search for high-performance electrodematerials has been of w ide interest to researchers4－6.Titanium dioxides,asa promising electrodematerial, have driven numerous studies because of their low price,nontoxicity,and reliable security.Especially,for several common polymorphs,such asanatase TiO2,rutile TiO2,brookite TiO2,and TiO2-B,lots of papers have employed them on advanced LIBs withhighenergy storageand power density7－11.Among these,TiO2-B w ith longer cycle life and better rate capability is the best candidate for thenegativematerialdue to the existenceof large open channels and a unique pseudocapacitive process12,13.However,practicalusagesof TiO2-B as other Ti-based oxide species are typically restricted by the low Li-ion diffusion coefficientand the bad electronic conductivity.

In recentyears,extensive researches regarding size controland materialmodification have been conducting to im prove the ion and electronic conductivity of TiO2-B.Some researchers demonstrated thatnanometer-sized TiO2-Bw ith larger surface areas and pore volumes w ould have an improved electrochem ical performance comparing to the bulk one14－16.They thought that reduced TiO2-B sizewould shorten the distance of electrons and Li-ion transportand providea largeelectrode/electrolyte contact area.However,the particle agglomerationwhich could cause to reduced surfaceareasand areal capacity is stilla stubborn problem for thepreparationof nanomaterials17,18.Also,therearesomeother studies in introducing the doped atoms or incorporating a second conductive phase into the TiO2-B to improve theelectronic conductivity19,20.It isobvious that these treatmentswould inevitably reduce the volumetric energy density of TiO2-B yet.To address this dilemma,some researchers put their efforts in introducing oxygen vacancieswithin the lattice21－24.Shin etal.25reported that theoxygen-deficientanatasewith awell-balanced Li+/e－transport displayed high electrochem ical properties.Zhang et al.26demonstrated that flow er-like hydrogenating TiO2-B w ith oxygen vacancies exhibited enhanced Li-ion and electronic conductivity. In termsof theoreticalstudies,many researchersemployed density functional theory(DFT)approach to elucidate themechanism of lithium insertion and diffusion in TiO2-B27－29.Arrouvel et al.27introduced a com putational study of the perfect TiO2-B to detect the insertion and transportprocesses.What′smore,thereare some calculation studies that reported the native O-vacancy and Tiinterstitial defects in the structure30－32.However,there is no indepth and clear theoretical study in investigating the effect of oxygen vacancieson Li-ion diffusion and electronic conductivity. In this paper,weuse the large scale system TiO2-Bw ith oxygen vacancies as the calculation model,employing the advanced computational techniquesbased on density functional theory,to explore the intrinsic features of the oxygen-deficient TiO2-B at atom ic level.

2 Computational techniques

To get the details of optim ized structures and corresponding energies,calculationsemploying the DFT+U methodwere implemented in theVienna Ab initio Simulation Package(VASP)33,34. Generalized gradientapproximation(GGA)of Perdew and Wang (PW 91)was introduced considering theheterogeneity of electron density35.Eigenstates of the electron wave functions were expanded on a plane-w ave basis setw ith a cutoff of 400eV for all calculations.The inner coresand electron-ion interactionswere described using pseudopotentialswithin the projectoraugmented wave(PAW)scheme36.Lattice systems weremodeled w ithin periodic boundary conditions.Full relaxation of all atomic positionswas carried outuntil the forceson allatomswere less than 0.5eV∙nm－1assuring geometrical and energetic convergence. Considering the efficiency of computations,the Brillouin zone wassampled through aMonkhorst-Pack 2×3×2 k-pointgrid for the structure optimizations37.The detailed discussion about the calculation parameterscan be found in theSupporting Information.

Itwas noteworthy that conventional DFT calculationswere imprecise for transitionmetaloxides.Thus,DFT+U methodwas introduced to evaluate the on-site Coulomb interactions in the localized d orbital and exchange interactions,by adding an effective Hubbard-U parameter to express the repulsion between electronson thesame orbital38.As to the oxygen-deficientTi-based oxides,theexistence of oxygen vacancies leads to the electronic states localized on theadjacent Tiatoms,and thus theaddition of U is necessary in this situation39－41.The Dudarev approach42is implemented in VASP with themagnitude of effective(U-J) chosen to be 3.0eV in the presentstudy(U=4.0eV,J=1.0eV). The detailed discussion about the chosen of(U-J)can be found in the Supporting Information.

In thiswork,our calculatedmodel,based on the TiO2-Bunitcell of Ti8O16,was constructed to Ti32O64containing 1×2×2 unit cells. The LiTi32O64－x(x=0,1,2)as the perfect/defectivemodelwas used to investigate energetic changes of the lithium insertion and transportw ithin the framework.Minimum energy pathway and the activation energy for lithium diffusion were calculated using the climbing-imagenudged elastic band(CI-NEB)method43.The total electronic density of states(DOS)and partial density of states (PDOS),whichwere related to the electronic conductivity,were also performed using an enlarged Monkhorst-Pack 4×6×4kpoint grid.Analysis of charge was conducted in thew ell-established Bader scheme,which can output the total charge of each atom within the enclosed Bader volume defining by the zero flux surfaces44.A lthough it isnot an absolutemeasure of charge,the Baderanalysisstill isausefulapproximation in providing a guide to changes in thenatureof theelectronic state.

In evaluating the relative importance of Li-ions insertion sites, we identified that the insertion voltage can bewell derived by theNernst equation used in previous studies of various lithiumintercalated transitionmetal com pounds45,46.In our scheme,one lithium atom inserted in the Ti32O64－x(x=0,1,2)model,and the insertion process can be simplified by the reaction

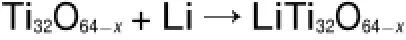
The insertion voltage can beexpressed as follows

where F means the Faraday constant,the freeenergy change(ΔG) can be approximated by the lithium internalenergy change(ΔE) since the contributions of the vibrational and configurational entropy are small to the insertion voltage47.The internal energy change(ΔE)isgiven by

where E(Ti32O64－x)and E(LiTi32O64－x)are the totalenergiesbefore and after the lithium intercalation,respectively.The energy of lithium metal,E(Li),was obtained by optim ization of bodycentered cubic Limetal(Im3m)with a0=0.3491 nm48,employing a k-pointgrid of 25×25×25.

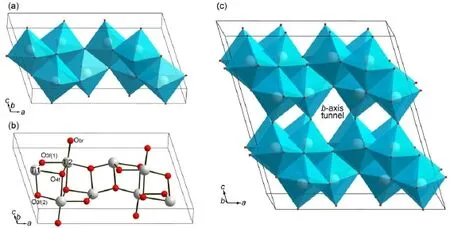
Fig.1 Bulk crystalstructureof TiO2-B (a)corner-sharing TiO6octahedraof the TiO2-B unitcell;(b)cage construction of the TiO2-B unitcell; (c)TiO6octahedra of ourmodel Ti32O64w ith the open b-axis tunnel in them idd le

Tab le 1 Experim entaland calculated structural parametersof TiO2-B

3 Results and discussion

3.1Crystals truc ture and oxygen vacancy

TiO2-B has amonoclinic crystalw ith the space group C2/m, consisting of edge and corner-sharing TiO6octahedra(shown in Fig.1(a)).The calculated lattice parameters of TiO2-B unit cell (Ti8O16)are listed in the Table1 and in good agreementw ith the experimental values49.Dalton etal.50labeled that TiO2-B contains two different typesof titanium atoms,that isTi1 and Ti2,and four nonequivalent oxygen atoms,namely Obrfor bridging oxygen atoms,O3f(1)for 3-fold coordinated oxygen atoms w ith bonds formed in[001]plane,O3f(2)for3-fold coordinated oxygen atoms w ith bonds formed in[010]p lane,and O4ffor 4-fold coordinated oxygen atoms(shown in Fig.1(b)).

In the structure,it isworth noticing that along the b-axis direction thereareopen pore tunnels(shown in Fig.1(c))which are the unique character of TiO2-B.These tunnels consisted of coterminous cavities are the favorite insertion siteand diffusion path for Li-ions.To investigateclearly the propertiesofdefectedmodel, it isnecessary to understand theexactnumberand typeof oxygen vacancies.Zhang et al.26found that oxygen vacancies not only existed in surfacebutalso inbulk phaseof thehydrogenating TiO2-B and they found that the oxygen content of deficient Ti2O3structure was 14.7%,whichmeant that the number of oxygen vacancies in ourmodel Ti32O64could beover2.Here,wemainly discussed the propertiesof the b-axis tunnels.So we assumed that there are two oxygen vacancies in ourmodel of Ti32O64and they are distributing along themiddle b-axis tunnel.

Fig.2(a)show ed the calculation model in our study.We can clearly find that four typesof oxygen are distributing around the b-axis tunnelw ith the identicalnumber(shown in Fig.2(b)).For the probableoxygen-deficient sites,we firstly discussed the one oxygen vacancy(Ov)situation to figureoutwhich typeof oxygen w aseasy for reducing.The reaction processwas sim plified as

Fig.2(c)shows the four typesof one-Ovmodels.Themarked Obr,O3f(1),O3f(2),and O4fare the reduced oxygen sitesshowing as the black ball.The reaction heat of reducing each type of oxygen atom swas calculated by the follow ing formula

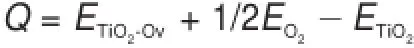
where Q was the reaction heat.ETiO2-Ov,EO2,and ETiO2,werethe energy of the oxygen-deficient TiO2-B(Ti32O63),the energy of oxygen molecule O2,and the energy of perfect TiO2-B(Ti32O64),respectively.

Table 2 lists the reaction heatof generating onevacancy for four types of oxygen atoms.We found that the orderof reaction heatwasas followsO3f(1)

Fig.2 Cage construction of TiO2-B (a)the fullview of our calculationmodelTi32O64;(b)the localview of the b-axis tunnelw ith the Bader chargemarked in the atoms; (c)the localview of four typesof one-Ovmodels;(d)the localview of four typesof two-Ovmodels. The full view of these oxygen-deficientmodels is same as the perfectTi32O64excep t for the reduced oxygen atomsw hich are rep laced by the black balls.

Table2 Propertiesof four typesof oxygen atom s

3.2Lithium inse rtion and d iffusion in the perfec t m odel

The lithium-ion diffusion in the TiO2-B is the key process for using TiO2-B as the lithium-cell anode,and to import firstly lithium-ion isnecessary,therefore it is important to analyze insertion of Li-ionsand detect the precise location in the calculatedmodel. Previous studieshave described three possible sites,labeled C,A1, and A2,in the perfect TiO2-B lattice27.Asnoted,the C sitewas in themiddleof the cavity along the b-axis channel,and at the center of the square-planararrangementof bridging oxygen atoms.The A1 sitewas located between two O3f(2)atoms in the TiO6octahedral layer.TheA2 sitewas lay between two Obratoms in theoxygen layer(shown in the Fig.3(a)).In the perfectmodelof LiTi32O64,our calculationsshowed that the insertion voltagesof the C,A1,and A2 siteswere 1.29,0.93,and 1.11V,respectively.The calculated voltages indicated that the C sitewas themost stable sitewhich was in keepingw ith previousstudies27.

As for lithium diffusion in the perfect TiO2-B,previous studies have elucidated three pathswhere the lithium atom hopped fromone C site to another ad jacent C site27,29.There are path(i)that migrates in the[001]planealong the[100]direction through the A2 sites;path(ii)thatmigrates between the two adjacentC sites along the b-axis channels;path(iii)thatm igrates along the[001] direction in the[100]plane through the A1 sites(show n in the Fig.3(b)).Theactivationenergiesof three diffusion pathsare listed in Table 3.We found that path(ii)possessed of the shortest diffusion distance of 0.377 nm and the lowestactivation energy of 0.51 eV as discussed by Arrouvel etal.27.Although theactivation energiesw ere slighthigher than the previous study,it can be attributed to the differenceof calculationmethod.Itwassuggested thatpath(ii)was the favored pathway for facile lithium diffusion. Also,we can conclude that path(i)and path(iii)with long diffusion ways and high activation energiesmake no significant contribution to the lithium diffusion in TiO2-B.Thus,we only considered the diffusion of path(ii)in the following defective models.The diffusion path was from one C site along b-axis to anotherC site.The transition statewas in them iddleof two C sites bondingwith two Obratoms.The transition statesalong three paths were shown in the Fig.S2 in Supporting Information.

Fig.3 Li-ions insertion and d iffusion in the cage construction of TiO2-B (a)fullview of three insertion sites;(b)threediffusion pathsof Li-ions in TiO2-B

Table 3 Lim igration paths between C sites and calculated m igration activation energies

Table4Liinsertion voltagesand m igration activation energiesalong b-axis in defected m odels

3.3Lithium insertion and d iffusion in the defected m odel

As for the defectedmodels,wealso detected the stable insertion sites of A1,A2,and C,and found that the C sitewas the best insertion site for Li-ions.The concrete insertion voltages still of three siteswere listed in Table S3 of Supporting Information.

For the situation of one-Ov,namely the system of LiTi32O63, Table 4shows the insertion voltage in the C site of O3f(1)and O4fmodels.Wecan see the insertion voltagesof stable Csitesare1.58 V,1.46V for O3f(1)and O4f,respectively.Itw as obvious that the insertion voltageswerehigher than thatof perfectmodel of 1.29 V,whichmeant that these sitesweremuch stable for insertion. However,the diffusion activation energies along the b-axiswere slightly higher than the perfectone.We noticed that the one-Ov structurewasasymmetry,which could give rise to an endotherm ic reaction for the diffusion along the b-axis and inducean increase of diffusion activation energies.

For the situation of two-Ov,namely the system of LiTi32O62,the models(1),(2),(3),and(4),all results of thesemodels arealso listed in Table 4.Due to the oxygen vacanciesoccurred in cavities along the b-axis one by one,thesemodelswere symmetric structure.Firstlywe studied the insertion voltageof Li-ions in the presenceof two-Ov.We found thatthe C site insertion voltagewas at the range from 1.33 to 1.38V,whichwasbelow thatof one-Ov modelsbutstillhigher than thatof perfectmodel.After getting the most stable binding configuration of Li-ions,then the diffusion processalong b-axis system was investigated by CI-NEBmethod. The diffusion energiesof thesemodelsat the rangeof 0.39－0.49 eV were lower than the perfectmodelof 0.51 eV.

It is important to analyze possible reasons thataffect the Li-ion diffusion barrier in the presenceof oxygen vacancies.Our studies showed that the diffusion energieswere decreased w ith the increase of oxygen vacancies,w hich meansmuch easier Li-ions diffusion.We considered that the introduction of oxygen vacancies must change the distribution of charges in the structure.So the analysis of charge was conducted both in the LiTi32O63and LiTi32O62.We took theone-OvmodelO3f(1)and the two-Ovmodel (2)as the analytic objects here.Fig.4(a)and 4(b)show ed the Bader charge of twomodels.It is obvious thatafter introducing oxygen vacancies the Bader chargeof Ti-Ov(the titanium atom close to oxygen vacancies)was increased by 0.2e－0.3e and the charge of oxygen around Ti-Ov was also increased slightly comparing to the perfectone(shown in the Fig.2(b)).Other atoms far away from the vacancy site had no significant changew iththeir Bader charge.Around themiddle b-axis tunnel,we can see that in Fig.4(a)there are only tw o Ti-Ov atoms w ith increased charge(markedw ith Ti+3.96e),while in Fig.4(b)there are four Ti-Ov atomswith increased charge(marked w ith Ti+3.91e).Since Li-ion insertion was also related to the Ti-Limutual repulsion, more charge-increased Ti-Ov atomsmay give rise to the increase of repulsion forcewhich could hinder the insertion.This canwell explain why the corresponding insertion voltagesare decreased in the two-Ovmodelscompared to theone-Ovmodel.

In the picture,we found that themodelO3f(1)(Fig.4(a))had a few charge change of oxygen atomswhile themodel(2)(Fig.4(b)) possessed of larger charge increase of oxygenatom s.As for the diffusion processoccurred along the b-axis,we detected that the distance of Li―Obrbonds in the transition state(0.161 nm)w as shorter than that of the C site(0.249 nm)(see Fig.S3 in the Supporting Information).Itmeans that the charge-increased Obratomswould give rise to a stronger electrostatic interaction betw een Li and Obratoms in transition state.In other w ords,the presence of oxygen vacancies stabilizes the transition statemore than thatof the stable state(i.e.,the C site),w hich results in the reduction of diffusion energy barrier.Furthermore,we considered the geometric effect.Because the Li-ionsmainly interacted w ith them iddle four bridging oxygen atoms,so we measured the distance of two bridging oxygen atom s(shown in the Table S4), and found that the distance hasw idened as the introduction of oxygen vacancies.As for the open tunnel,the broader it is,the easier the Li-ionsmigrate.Therefore,we think that the geometric effect isalso favorable for the diffusion.

Thismay explain the reason why two oxygen vacancies could further reduce the diffusion activation energies.As the increase of oxygen vacancies,we noticed thatchargesof oxygen around the tunnelswere increased in whole and the distance of two bridging oxygen atoms became wider.These could cause the energy difference between the stable insertion site and the transition state site further decreased,whichwouldmake theopen tunnelmore favorable for the diffusion.

3.4In fluence o f Li-ion insertion concen tration on the d iffusion p ro cesses

In above section,we detected theeffects of oxygen vacancies on the diffusion in the concentration of x(Li/Ti)=0.03125(the ratio of intercalated Liatomsand Tiatoms in the structure).Not only the oxygen vacancy but the existence of some lithium-ions in the electrode significantly affected the diffusion.Therefore,we also detected themutual effects of Li-ion on the diffusion in several different concentrations.

Fig.4Changeof Bader charge in defectedmodels (a)for theone-OvmodelO3f(1);(b)for the two-Ovmodel(2)

Table 5Inser tion voltages of different Li-ions concentration

For the lithium insertion in perfect TiO2-B,previous studies showed that the stability of insertion sitesmainly depended on two factors,the Li-Ti repulsion and themutual repulsion of Li-ions27. In the lower concentration(x(Li/Ti)≤0.25)mutual repulsionwas weak and the Li-Ti repulsion played a leading role.We found that C site had the longest Li-Tidistance comparing to theA1 and A2 sites,so C sitewas themoststable site.W hen x(Li/Ti)=0.25,all C sitesof TiO2-Bwereoccupied by Li-ions(shown in the Fig.S4(a)).While in thehigher concentration(0.25

Here,we should notice that the insertion voltage in defectedmodel Ti32O62is higher than that of perfect one in low Li-ion concentration(x(Li/Ti)≤0.25),while lower in high concentration (0.25

Fig.5DOSand PDOSof perfect TiO2-B in(a)and(b);DOSand PDOSofdefected modelO3f(1)in(c)and(d); DOSand PDOSof defectedmodel(1)in(e)and(f) Eg:energy gap

3.5Electron ic s tructu res

In this section,we studied the electronic structure of TiO2-B materials.The total electronic DOS and the PDOS were conducted w ithin the GGA+U scheme.Due to the existence of oxygen vacancies,the U correction is crucial for ourelectronic structure calculation51－53.Itwas known that TiO2was a sem i-conductivematerialwith theband gap of 3.0－3.2 eV52.Fig.5(a) and 5(b)showed the DOSand PDOS of perfectTiO2-B w ith the Fermienergy(EF)setting to zero.From Fig.5(a),itwasshown that theband gap of bulk TiO2-Bwasabout3.0eV correspondingwith the previous research52.However,the large band gap hindered the transportof electrons,whichmeantpoorelectronic conductivity. Fig.5(b)w as the PDOS of perfectmodel of LiTi32O64.We found that the common titanium 3d orbital(Ti-3d)and the oxygen 2p orbital(O-2p)were themain contributorof stateswhile the lithium 1s orbital(Li-1s)w asnegligible.

As for the defectedmodels,we took theone-OvmodelO3f(1)and the two-Ovmodel(1)asouranalytic objectshere,and the DOS of othermodelswasshowed in the Supporting Information Fig.S4. Fig.5(c)showed the DOSofmodelO3f(1).Itcan be seen thatnew peaksappeared w ithin the band gap(－3.0to 0.0eV).The reduced band gapwas about1.9 eV,whichmeantan enhanced electronic conductivity.As for themodel(1)of two oxygen vacanciesshown in Fig.5(e),ithad a further reduced band gap of 1.2 eV with a stronger intension of theemerging peaks.Wealso investigated the PDOS of thesemodels.Fig.5(d)and 5(f)showed the PDOS of modelO3f(1)andmodel(1),we can see that the PDOSof Ti-Ov-3d is the contributorof the reduced band gap.

Previousanalysis of charge showed that Ti―O bands existed in the form of covalentbond.Studiesshowed that,in the process of generating oxygen vacancies,the reduced oxygen could not take away all covalent electrons in the Ti―O bands30.The remaining electronsw ith a free state hopped between the oxygen vacancy titanium 3d unoccupied orbital,which largely improved theelectronic conductivity.Andw ith the increaseof oxygen vacancies,itw ill generatemore Ti-Ov atom s,and asa resultgive rise to a stronger peak of Ti-Ov-3d.Theband gap of alldefected modelswas reduced to about1.0－2.0eV(shown in the Fig.S5), which also suggested that in the oxygen-deficientmodelelectrons in the full filled bandwould easily transition up to empty band and exhibitbetter conductivity.This canwellexplain the reason that improved electronic conductivity occurred in the hydrogenated TiO2-B asdescribed by Zhang etal.26.

4Conlusions

In conclusion,we elaborated an emergingmaterialofoxygendeficient TiO2-B.Implementing advanced DFT+U scheme,we investigated the Li-ionsand electrons conductivity of thismaterial at theatomic level.We can conclude threemain points:

(1)In the low Li-ions concentration,itwas proved that the oxygen vacancies w ere favorable for the Li-ion insertion and diffusion.Thedefected TiO2-Bwithhigh insertion voltageand low diffusion barrier was easy for Li-ion intercalation,which was beneficial for the charge process.

(2)In thehigh Li-ionsconcentration,theoxygen-deficientTiO2-B w ith low Li-ion bonding energy is favorable to deintercalation, which isbeneficial for the discharge process.

(3)A narrow band gap was gotten in the defectedmodelw ith the small band gap of 1.2 eV.With the increase of oxygen vacancies,the statesof Ti-Ov-3d willbe strongerwith the intension, w hich gives rise to an enhanced electronic conductivity.

These results suggest that the defected TiO2-B exhibited better ionic and electronic conductivity can be used for a negativematerialof advanced rechargeable lithium-ion batteries.

Suppo rting In fo rm ation:The VASP calculation parameter tests,theauxiliary structuralpictures,and DOS figureshavebeen included.This information isavailable freeof charge via the internetathttp://www.whxb.pku.edu.cn.

References

(1)Tarascon,J.M.;A rmand,M.Nature 2001,414,359.doi: 10.1038/35104644

(2)Wagner,F.T.;Lakshmanan,B.;Mathias,M.F.Journalof PhysicalChemistry Letters2010,1,2204.doi:10.1021/ jz100553m

(3)A rmand,M.;Tarascon,J.M.Nature 2008,451,652.doi: 10.1038/451652a

(4)Liu,Y.;Wu,J.;Zhao,W.;Chu,J.;Qi,T.Chinese Journal of Chemistry 2013,31,1257.doi:10.1002/cjoc.201300380

(5)Tian,M.;Wang,W.;Wei,Y.;Yang,R.JournalofPower Sources2012,211,46.doi:10.1016/j.jpowsour.2012.03.084

(6)Xu,G.;Zhong,K.;Zhang,J.M.;Huang,Z.JournalofApplied Physics2014,116,063703.doi:10.1063/1.4892018

(7)Hong,Z.;Wei,M.;Lan,T.;Cao,G.Nano Energy 2012,1,466. doi:10.1016/j.nanoen.2012.02.009

(8)Anicete-Santos,M.;Gracia,L.;Beltrán,A.;Andrés,J.;Varela, J.;Longo,E.Phys.Rev.B 2008,77,085112.doi:10.1103/ PhysRevB.77.085112

(9)Chu,D.;Yuan,X.;Qin,G.;Xu,M.;Zheng,P.;Lu,J.;Zha,L. Journal ofNanoparticle Research 2007,10,357.

(10)Gao,Q.;Gu,M.;Nie,A.;Mashayek,F.;Wang,C.;Odegard, G.M.;Shahbazian-Yassar,R.Chem.Mat.2014,26,1660.doi: 10.1021/cm403951b

(11)Laskova,B.;Zukalova,M.;Zukal,A.;Bousa,M.;Kavan,L. JournalofPower Sources 2014,246,103.

(12)Zukalova,M.;Kalbac,M.;Kavan,L.;Exnar,I.;Grätzel,M. Chem.Mat.2005,17,1248.doi:10.1021/cm048249t

(13)Liu,S.;Wang,Z.;Yu,C.;Wu,H.B.;Wang,G.;Dong,Q.;Qiu, J.;Eychmüller,A.;Lou,X.W.Advanced Materials2013,25, 3462.doi:10.1002/adma.v25.25

(14)Bruce,P.G.;Scrosati,B.;Tarascon,J.M.Angewandte Chemie International Edition 2008,47,2930.

(15)Dylla,A.G.;Xiao,P.;Henkelman,G.;Stevenson,K.J.The JournalofPhysicalChemistry Letters2012,3,2015.doi: 10.1021/jz300766a

(16)Li,X.;Zhang,Y.;Li,T.;Zhong,Q.;Li,H.;Huang,J.Journal ofPower Sources 2014,268,372.doi:10.1016/j. jpowsour.2014.06.056

(17)Lv,C.J.;Hu,T.;Shu,K.;Chen,D.;Tian,G.MicroscopyResearch and Technique2014,77,170.doi:10.1002/jem t. v77.2

(18)Zhou,W.;Liu,H.;Boughton,R.I.;Du,G.;Lin,J.;Wang,J.; Liu,D.JournalofMaterialsChemistry 2010,20,5993.doi: 10.1039/b927224k

(19)Yin,W.J.;Wei,S.H.;Al-Jassim,M.M.;Yan,Y.Phys.Rev. Lett.2011,106,066801.doi:10.1103/PhysRevLett.106.066801 (20)Gai,Y.Q.;Li,J.B.;Li,S.S.;Xia,J.B.;Wei,S.H.Phys.Rev. Lett.2009,102,4.

(21)Yan,Y.;Hao,B.;Wang,D.;Chen,G.;Markweg,E.;Albrecht, A.;Schaaf,P.Journal ofMaterials Chemistry A 2013,1, 14507.doi:10.1039/c3ta13491a

(22)Li,G.;Zhang,Z.;Peng,H.;Chen,K.RSCAdvances2013,3, 11507.doi:10.1039/c3ra41858h

(23)Qiu,J.;Li,S.;Gray,E.;Liu,H.;Gu,Q.F.;Sun,C.;Lai,C.; Zhao,H.;Zhang,S.The JournalofPhysical Chemistry C 2014,118,8824.

(24)Myung,S.T.;Kikuchi,M.;Yoon,C.S.;Yashiro,H.;Kim,S. J.;Sun,Y.K.;Scrosati,B.Energy&Environmental Science 2013,6,2609.

(25)Shin,J.Y.;Joo,J.H.;Samuelis,D.;Maier,J.Chem.Mat. 2012,24,543.doi:10.1021/cm2031009

(26)Zhang,Z.;Zhou,Z.;Nie,S.;Wang,H.;Peng,H.;Li,G.;Chen, K.JournalofPower Sources2014,267,388.doi:10.1016/j. jpowsour.2014.05.121

(27)A rrouvel,C.;Parker,S.C.;Islam,M.S.Chem.Mat.2009,21, 4778.doi:10.1021/cm900373u

(28)A rmstrong,A.R.;A rrouvel,C.;Gentili,V.;Parker,S.C.; Islam,M.S.;Bruce,P.G.Chem.Mat.2010,22,6426.doi: 10.1021/cm102589x

(29)Panduw inata,D.;Gale,J.D.JournalofMaterials Chemistry 2009,19,3931.doi:10.1039/b902683e

(30)DiValentin,C.;Pacchioni,G.;Selloni,A.Journal ofPhysical Chemistry C 2009,113,20543.doi:10.1021/jp9061797

(31)Finazzi,E.;DiValentin,C.;Pacchioni,G.Journal ofPhysical Chemistry C 2009,113,3382.

(32)Shin,J.Y.;Samuelis,D.;Maier,J.Solid State Ionics2012, 225,590.doi:10.1016/j.ssi.2011.12.003

(33)Kresse,G.;Furthmüller,J.ComputationalMaterialsScience 1996,6,15.doi:10.1016/0927-0256(96)00008-0

(34)Kresse,G.;Hafner,J.Phys.Rev.B 1994,49,14251.doi: 10.1103/PhysRevB.49.14251

(35)Perdew,J.P.;Wang,Y.PhysicalReview B 1992,45,13244. doi:10.1103/PhysRevB.45.13244

(36)Kresse,G.;Joubert,D.Phys.Rev.B 1999,59,1758.

(37)Monkhorst,H.J.;Pack,J.D.Physical Review B 1976,13, 5188.doi:10.1103/PhysRevB.13.5188

(38)Anisimov,V.I.;Zaanen,J.;Andersen,O.K.PhysicalReview B 1991,44,943.doi:10.1103/PhysRevB.44.943

(39)Nolan,M.;Elliott,S.;Mulley,J.;Bennett,R.;Basham,M.; Mulheran,P.Physical Review B 2008,77,235424.doi: 10.1103/PhysRevB.77.235424

(40)Yang,J.;Lv,C.Q.;Guo,Y.;Wang,G.C.The Journalof ChemicalPhysics2012,136,104107.doi:10.1063/1.3692292

(41)Yang,J.;Cao,L.X.;Wang,G.C.JournalofMolecular Modeling 2012,18,3329.doi:10.1007/s00894-011-1337-4

(42)Dudarev,S.L.;Botton,G.A.;Savrasov,S.Y.;Humphreys,C. J.;Sutton,A.P.Physical Review B 1998,57,1505.doi: 10.1103/PhysRevB.57.1505

(43)Henkelman,G.;Uberuaga,B.P.;Jónsson,H.The Journalof ChemicalPhysics2000,113,9901.doi:10.1063/1.1329672

(44)Henkelman,G.;Arnaldsson,A.;Jónsson,H.Computational Materials Science 2006,36,354.doi:10.1016/j. commatsci.2005.04.010

(45)Morgan,D.;Van der Ven,A.;Ceder,G.Electrochemicaland Solid-State Letters2004,7,A30.

(46)Kang,K.;Ceder,G.Phys.Rev.B 2006,74,094105.doi: 10.1103/PhysRevB.74.094105

(47)Braithwaite,J.S.;Catlow,C.;Harding,J.H.;Gale,J.D. Physical Chemistry Chemical Physics 2001,3,4052.doi: 10.1039/b103928h

(48)Keller,D.V.;Kanda,F.A.;King,A.J.The JournalofPhysical Chemistry1958,62,732.doi:10.1021/j150564a024

(49)Feist,T.P.;Davies,P.K.JournalofSolid State Chemistry 1992,101,275.doi:10.1016/0022-4596(92)90184-W

(50)Dalton,A.S.;Belak,A.A.;Van derVen,A.Chem.Mat.2012, 24,1568.doi:10.1021/cm203283v

(51)Finazzi,E.;DiValentin,C.;Pacchioni,G.;Selloni,A.J.Chem. Phys.2008,129,154113.doi:10.1063/1.2996362

(52)Yang,K.S.;Dai,Y.;Huang,B.B.;Whangbo,M.H.Chem. Mat.2008,20,6528.doi:10.1021/cm801741m

(53)Graciani,J.;Ortega,Y.;Sanz,J.F.Chem.Mat.2009,21,1431. doi:10.1021/cm803436e

First-Principles Study on TiO2-B with Oxygen Vacancies as a Negative Material of Rechargeable Lithium-Ion Batteries

KONG Ling-Ming1ZHU Bao-Lin1PANG Xian-Yong1,*WANG Gui-Chang2,3,*
(1College ofChemistry and Chemical Engineering,Taiyuan University ofTechnology,Taiyuan 030024,P.R.China;
2DepartmentofChem istry,Key Laboratory ofAdvanced Energy Materials Chemistry(Ministry ofEducation), NankaiUniversity,Tianjin 300071,P.R.China;3State Key Laboratory ofCoalConversion, Institute ofCoalChemistry,Chinese Academy ofSciences,Taiyuan 030001,P.R.China)

Density functiona l theory calculationswere carried outon oxygen-de ficientTiO2-B to evaluate the effecto foxygen vacancies on its electrochem icalproperties.The computationalstudies focused on the lithium (Li)-ion transportand e lectronic conductivity of this defect-containingmateria l.Calcu lations on TiO2-Bwith low Li-ion concen tration(x(Li/Ti)≤0.25)suggest tha t com pa red w ith defec t-free TiO2-B,oxygen-deficient TiO2-B has a higher intercalation voltage and lowerm igration activation energy along the b-axis channel.This facilitates Li-ion interca lation,which is beneficia lfor the charge p rocess o f rechargeable batteries.Meanwhile,for TiO2-B w ith high Li-ion concen tra tion(x(Li/Ti)=1),satu rated oxygen-de ficient TiO2-B w ith low er insertion vo ltage favors Li-ion deinterca lation,which aids the discharge process.Electronic structure calculations suggest that the band gap of this defect-containingm ateria l is w ithin 1.0－2.0eV,which is na rrowe r than thatof de fect-free TiO2-B(3.0eV).Themain contributor to the band-gap narrow ing is the density of the Ti-Ov-3d state,which becomesmuch higheras the oxygen vacancy content increases,which increases electronic conductivity.

September 24,2015;Revised:December 29,2015;Published on Web:December 29,2015.

TiO2-B;Oxygen vacancy;Inte rcala ted voltage;Mig ra tion activation ene rgy;Band gap

O641；O 649

10.3866/PKU.WHXB201512292

*Corresponding authors.PANG Xian-Yong,Email:pangxy_tyut@126.com.WANG Gui-Chang,Email:wangguichang@nankai.edu.cn.

The projectwas supported by the State Key Program ofNaturalScience Foundation of Tianjin,China(13JCZDJC26800),Foundation of State Key Laboratory of CoalConversion,China(J15-16-908),and Natural Science Foundation of ShanxiProvince,China(2013011012-8).

天津市重点自然科学基金(13JCZDJC26800),煤转化国家重点实验室开放基金(J15-16-908)与山西省自然科学基金(2013011012-8)资助项目. ©Editorialofficeof Acta Physico-Chim ica Sinica