一类奇摄动半线性时滞抛物型偏微分方程的渐近解

包立平

(杭州电子科技大学理学院,浙江杭州310018)

一类奇摄动半线性时滞抛物型偏微分方程的渐近解

包立平

(杭州电子科技大学理学院,浙江杭州310018)

文中讨论了一类奇摄动时滞抛物型偏微分方程的初边值问题,得到了其形式渐近展开,证明了奇摄动半线性时滞偏微分方程的极大值原理,从而得到了最大值估计及相应的Schuader估计.在此基础上,得到了柱状区域上解的存在唯一性和渐近解的一致有效性.

奇摄动;半线性;时滞抛物型方程;渐近展开;Schuader估计;最大值原理;余项估计

§1 引 言

奇摄动时滞抛物型偏微分方程在各种学科的研究中得到了很大的应用.例如在种群动力学,传染病学等方面有重要应用;在随机微分方程的研究中也有重要价值.

近年来奇摄动时滞抛物型偏微分方程的研究得到了人们的重视,如A.R.Ansari,S.A.Bakr,G.I.Shishkin[1]研究了一类奇摄动时滞线性抛物型偏微分方程问题,得到了矩形域上的解的存在唯一性和一致有效渐近解.莫嘉琪[2]得到了小时滞的奇摄动抛物型偏微分方程的一致有效渐近解等.

本文研究如下一类奇摄动时滞抛物型偏微分方程的初边值问题

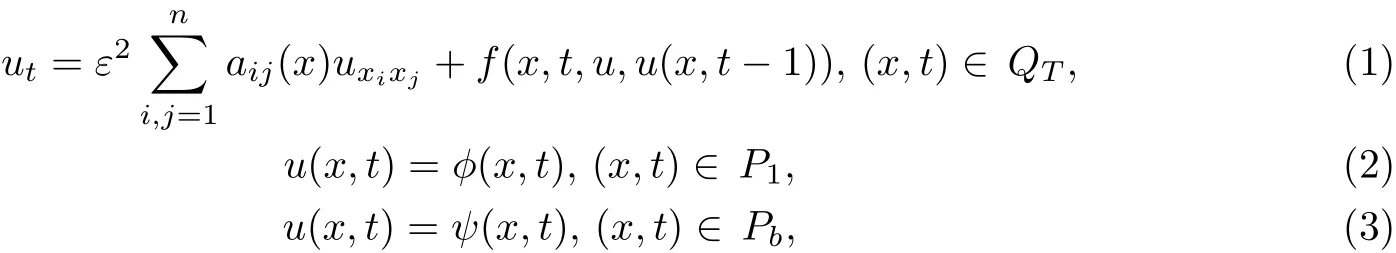
这里Ω∈Rn是有界凸区域,QT=Ω×[0,T],1＜T≤2,P1=∂Ω×[0,T],而Pb=Ω×[−1,0].

上述问题包含了如Hutchinson[3]方程等来源于不同研究邻域的多种问题,有重要的研究价值.

现在作如下假设:

[H1] 任取ξ∈

[H2]P1∈C2,

[H3] ψ(x,0)=ϕ(x,0),x∈ ∂Ω.

用分步法对(1)-(3)进行分析.在[0,1]上(1)-(3)可改写为:

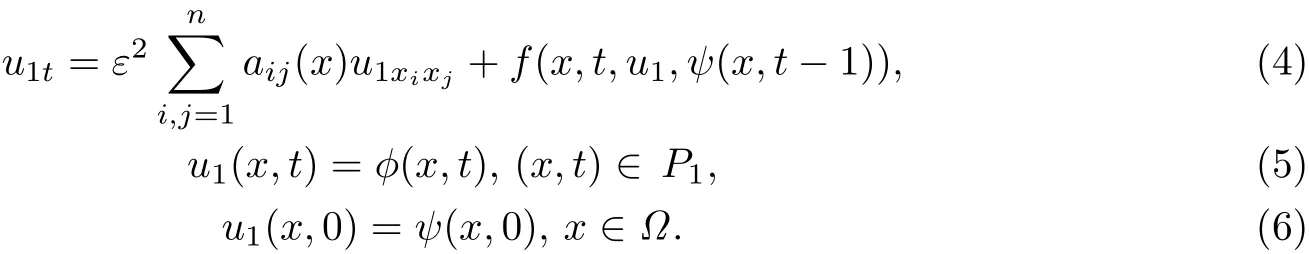
设(4)-(6)的解为u1(x,t),则在[1,T]上(1)-(3)为:

§2 形式渐近展开式的构造

首先从(4)-(9)开始,构造(1)-(3)的形式渐近展开式.

设(4)-(6)的解为u1(x,t),而(7)-(9)的解为u2(x,t),则(1)-(3)的解为

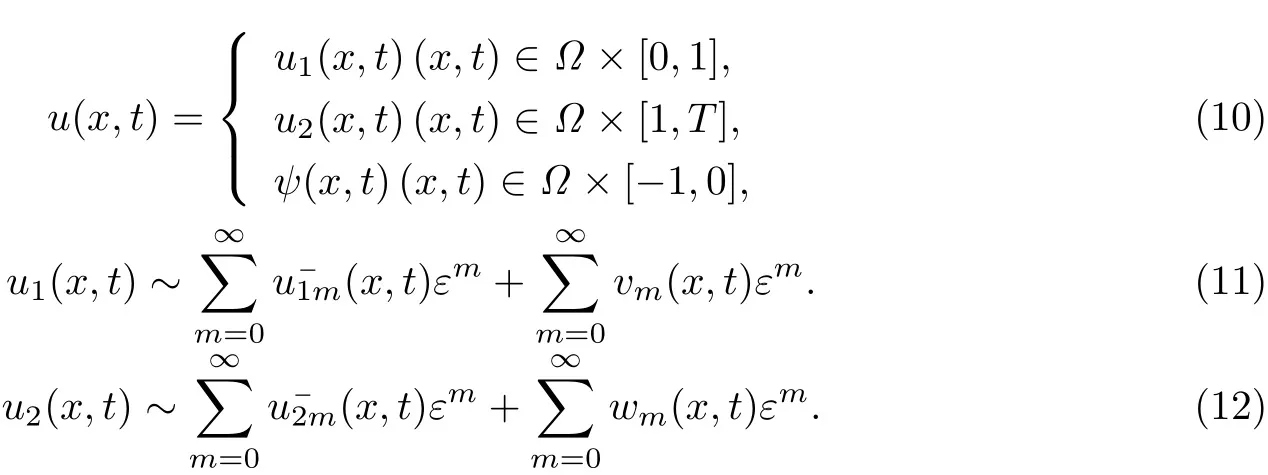
将(11)代入(4)-(6),并比较同次幂系数可得:

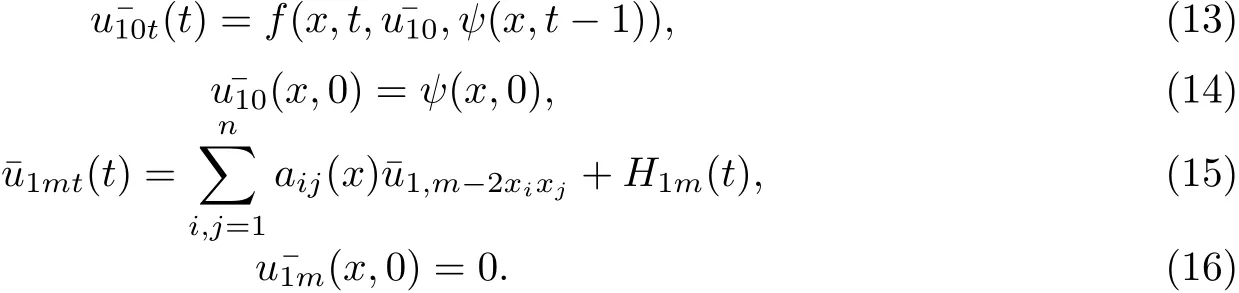
其中H1m是由和ψ(x,t−1)决定的函数.

由于f(x,t,y,z)∈C1,α,所以(13)-(14)的解存在唯一,(15)-(16)是线性方程,其解存在唯一.将(12)代入(7)-(9),并比较同次幂系数可得:

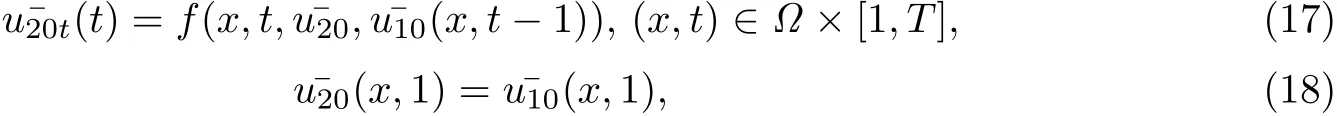
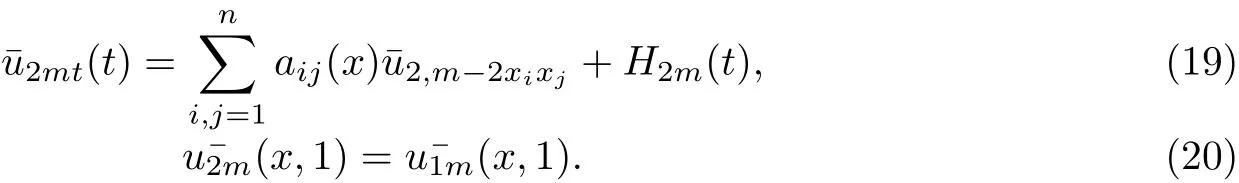
同理(17)-(20)的解存在唯一,所以构造了(11)-(12)的正则部分的解.

现在构造边界层解,首先在∂Ω附近作局部坐标变换,设新坐标为(ρ,θ),x∈Ω为Ω内在∂Ω附近的一点,ρ =dist(x,∂Ω). 则沿着法线方向从∂Ω上一点Q到x,θ=(θ1,θ2,···,θn−1)是Q在n−1维流形∂Ω上的非奇异坐标,则x的坐标为(ρ,θ).

在∂Ω的某邻域内(4)-(9)可以写作:

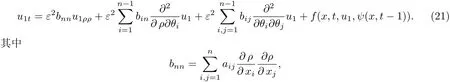
可得:

同理可得:

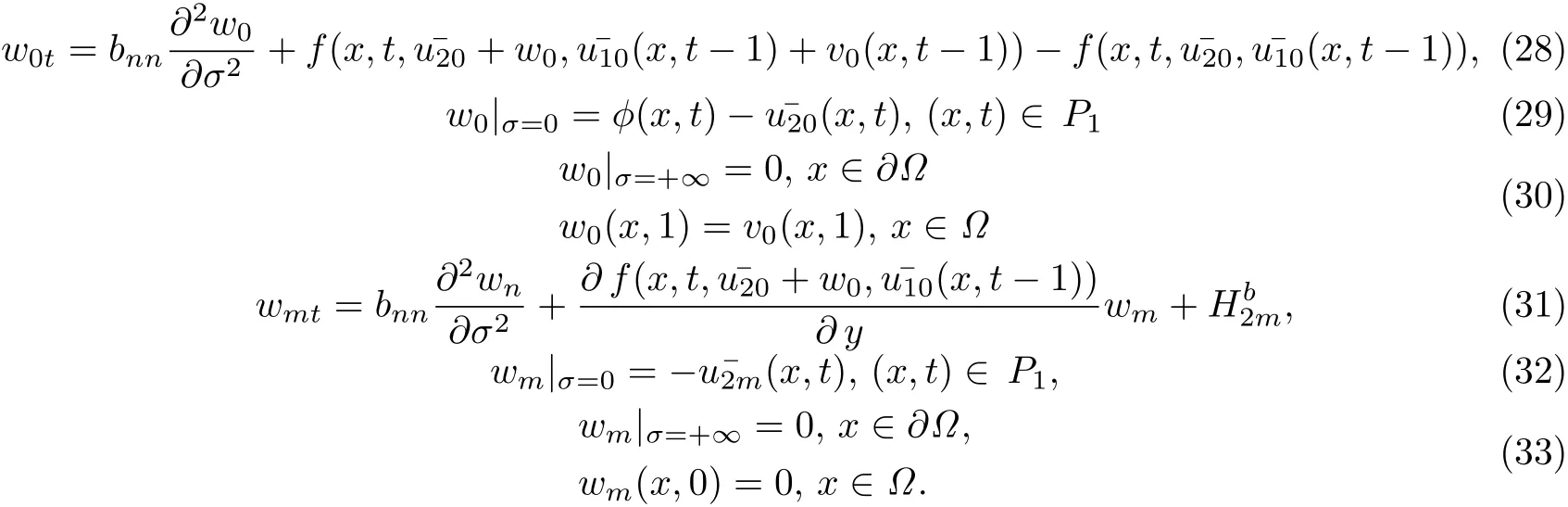
引理1(22)-(33)的解存在唯一.

证先证(22)-(24)的解唯一.设(22)-(24)有两个解v1和v2,令w=v1−v2,则

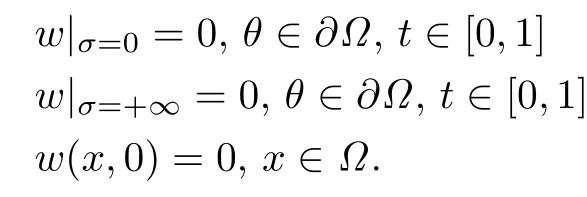
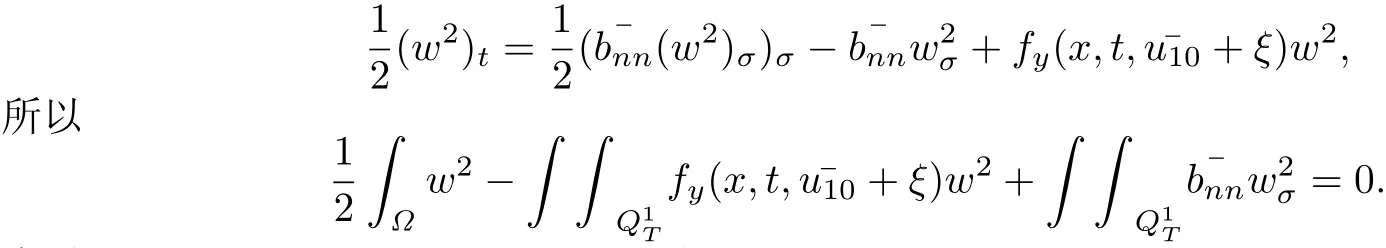
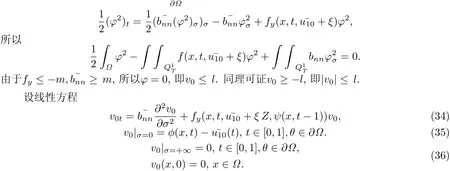
同理可证,(25)-(33)的解均存在唯一.

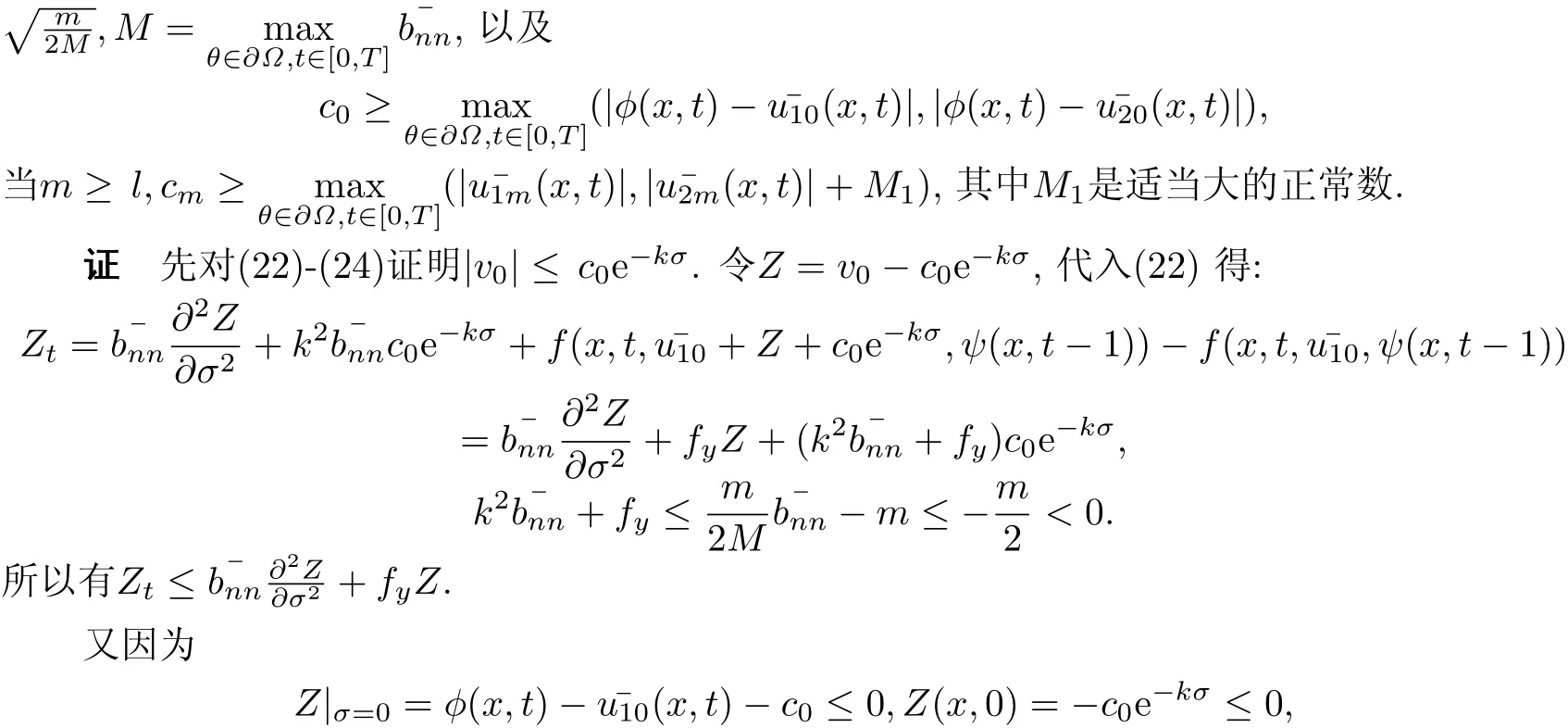
引理2(22)-(33)的解vn,wn,n=0,1,2,···,满足这里k=所以由极大值原理可知,Z ≤ 0,即,同理可知,所以同理可证:显然是正常数. 令Z=则有

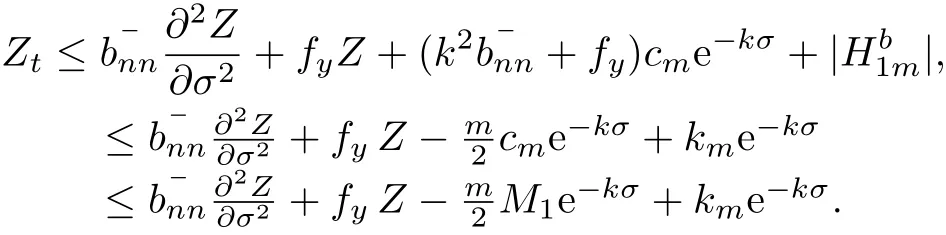
由于M1适当大,因此所以由极大值原理知,同理可证,

至此,构造了形式渐近解(11)和(12).

§3 解的存在唯一性

首先做(1)-(3)的解的最大值估计和Schuader估计.

定理1(极值原理) 若(H1)-(H3)的条件满足,令

同理对于w(x0,t0)=infQ1Tw(x,t)≤0,可以证得w(x0,t0)=0,即在同样方式可证QT上w(x,t)≡0,即(1)-(3)的解唯一.

§4 余项估计

这里H2(x,t,ε)由已知的和wm决定,因此是有界的,即|H2(x,t,ε)|≤M,用上述同样方式可证得即形式渐近展开式(11)和(12)一致有效.

§5 结束语

文中对一类半线性抛物型时滞偏微分方程的奇摄动问题进行了分析.运用边界层校正方法构造了形式渐近展开式,证明了奇摄动时滞半线性抛物型偏微分方程的极大值原理,从而得到了最大模估计和相应的Schuader估计,得到了在QT=Ω×[0,T]的柱状区域上解的存在唯一性,其解是属于的,得到了余项估计,因此证明了渐近解的一致有效性.

[1] Ausari A R,Bakr S A,Shishkin G I.A parameter-robust fi nite di ff erence method for Singularly perturbed delay parabolic partial di ff erential equations[J].Journal of Computational and Applied Mathematics,2007,205:552-566.

[2] 莫嘉琪,姚静荪.一类微分-差分反应扩散方程的渐近解[J].数学杂志,2011,311(1):133-137.

[3] Gourley S A.Travelling fronts in the di ff usive Nicholson’s blow fl ies equations with distributed delays[J].Mathematical and Computer Modelling,2000,32:843-853.

[4] Thandapani E,Savithri R.On Oscillation of a neutral partial functional di ff erential equations[J].Bulletin of the Institute of Mathematics Academia Sinica,2003,31(4):273-292.

[5] Pao Liu Chow.In fi nite-dimensional parabolic equations in Gauss-Sobolev spaces[J].Communications on Stochastic Analysis,2007,1(1):71-86.

[6] Xing Fuzou.Delay induced traveling wave fronts in reaction di ff usion equations of Kpp-Fisher type[J].Journal of Computational and Applied Mathematics,2002,146:309-321.

The asymptotic solution of a class of singular perturbed semi-linear delayed parabolic partial di ff erential equation

BAO Li-ping
(School of Science,Hangzhou Dianzi University,Hangzhou 310018,China)

In this paper,a class of initial boundary problem of the singular perturbed semi-linear delayed parabolic partial di ff erential equation is discussed.The formal asymptotic expansion of the problem is obtained.The maximum principle of the singular perturbed delayed semi-linear parabolic partial di ff erential equations is proved.Then,the maximum-norm estimation and Schauder estimation for this problem are obtained.By the maximum-norm estimation and Schauder estimation for this problem,the existence and uniqueness of the solution of the problem on the columnar zone is proved,and the uniformly valid estimation of the asymptotic expansion is gained.

singular perturbation;semi-linear;delay parabolic di ff erential equation;asymptotic expansion;Schauder estimation;maximum-norm estimation;estimation of the remainder

35B25;35K57

O175.12

A

:1000-4424(2016)03-0307-09

2015-11-25

2016-01-25

国家自然科学基金(51175134)