Effects of plasma radiation on the nonlinear evolution of neo-classical tearing modes in tokamak plasmas with reversed magnetic shear

Shuai Jiang(姜帅), Zheng-Xiong Wang(王正汹), Lai Wei(魏来), and Tong Liu(刘桐)

Key Laboratory of Materials Modification by Beams of the Ministry of Education,School of Physics,Dalian University of Technology,Dalian 116024,China

Keywords: neoclassical tearing mode,magnetohydrodynamics,nonlinear phenomena

1.Introduction

The dynamics of macroscale magnetohydrodynamic(MHD)instability plays a crucial role in the field of magnetic confinement fusion due to its potential to disrupt steady-state operations.[1-23]The neo-classical tearing mode (NTM), as one of the typical modes of MHD instabilities,has been widely explored theoretically and experimentally for decades.[1-15]NTM can be driven by the perturbed helical bootstrap current because of the pressure flattening inside the magnetic island,leading to the magnetic island destabilization.[1-11]Moreover,large NTM islands on different rational surfaces can interact with one another, affecting the transport and equilibrium of the plasma,and even causing the plasma disruptions in a highbeta plasma.[13,14]To avoid NTMs,numerous studies have explored various methods for triggering them to prevent the occurrence of seed magnetic islands.One notable development is the discovery that equilibrium toroidal shear flows can suppress the influence of NTMs, by reducing the NTM excitation threshold and the saturated island width.[17]In addition,the effects of increased shaping, elongation, and triangularity can be observed in the mechanisms triggering seed island formation,which alters the coupling between sawtooth stability and the flux surface.However, these effects are typically weak for NTMs.[9]Furthermore,on the early experimental device, Changet al.had observed the nonlinear neo-classical pressure-gradient-driven tearing modes in TFTR and made an important impact on the design of future tokamaks.[15]Additionally, various mechanisms can also affect NTM stability.For example, the interaction between energetic particles and NTM has been observed in ASDEX Upgrade and DIIID.[24,25]Therefore, it is critically necessary to further investigate the interplay between NTM and other effects for longpulse tokamak devices like ITER with the aim of maintaining highly confined plasma and achieving high-beta in the present and future.

In addition to MHD instabilities,some other mechanisms can critically impair the long-pulse discharge of tokamak,among which plasma radiation has been regarded as one of the key energy loss mechanisms.Such studies on plasma radiation have been carried out, including bremsstrahlung, recombination radiation, cyclotron radiation and line radiation.[16,26-34]It is worth mentioning that the line radiation comes mainly from the impurity radiation in the tokamak plasmas.Gateset al.found that islands could be driven by the plasma radiation and this performance was consistent with the density limit.[27]In Ref.[28],Tenget al.further investigated the tearing mode driven by the plasma radiation as well as illustrated the mechanism of density limit.However, the electron cyclotron radiation was not considered in their work.Apart from the theoretical and numerical analysis, Suttropet al.found that the impurity radiation could destabilize the (m,n)=(3,1) single tearing mode islands in ASDEX Upgrade, which grew during the current profile contraction phase.[29]Anm=2 island induced by the plasma radiation was explored in EAST approaching to the density limit when the loss of plasma radiation is more than the ohmic heating input.[30]Besides, Huet al.numerically studied plasma radiation response and MHD destabilization by a 3D-code JOREK and observed that the mode growth was propelled by the current,leading to the collapse in core temperature.[31]Furthermore,Salzedaset al.advanced a new theory that MHD instabilities could be induced by plasma radiation.Thus, the investigation of radiation induced instabilities is significantly important for future large tokamaks.For example, ITER is expected to work close to 100%edge radiation in order to decrease the thermal load on the divertor.[32]Additionally, these kinds of plasma radiation can also interact with all the magnet materials, causing modifications of plasma performance and ultimately affecting the lifetime of tokamak.[33]Recent advancements in studies have enhanced our understanding of the underlying mechanisms of the impact of plasma radiation on MHD instabilities.Nevertheless, there are still some unknown issues regarding the effects of plasma radiation on NTMs.[16,32,34]

On the other hand, reversed magnetic shear (RMS) configuration, as one of the main schemes in the experimental scenarios, is most likely to achieve the long-pulse steadystate operation for the toroidal fusion devices.[1,6,8,10,11,35-46]In the RMS configuration, the internal transport barrier can be triggered so that the core plasma pressure has a high gradient and then generates a high fraction of bootstrap current,which is recognized to be the steady-state configuration for the advanced tokamaks.[10]Nevertheless, the non-monotonic safety factorqprofile can produce two rational surfaces with the same helicity, resulting in a dangerous MHD instability named double tearing mode (DTM).Thus, the associated nonlinear dynamics of DTM is of particular significance in achieving a full understanding of fast dynamics interrelated to magnetic reconnection.More specifically, the magnetic surfaces can be destroyed by the DTM with a large growth rate, leading to confinement degradation, which is substantially associated with the major disruption in the JT-60U negative shear plasmas.[36,47]Furthermore, the modes of the cellular instabilities around the plasma surface indicate that they are DTMs.[48]It was first studied in Ref.[49]by using a skincurrent profile with two points of incoherence in the perturbation of the radial magnetic field in cylindrical tokamaks.In addition,DTM with high mode numbers can be further destabilized in the case that the two resonant surfaces are sufficiently close.The deformation of the magnetic island becomes quite strong, resulting in the fast reconnection of magnetic field lines.[50]Particularly,the instability of DTM is much stronger than that of single tearing mode, and the current penetration time is much faster than the diffusion time predicted by classical resistivity.It is interesting to note that explosive growth of the DTM has been observed after the Rutherford phase in the intermediateΔrscase (whereΔrs=|rs2-rs1| is the separation between the two rational surfaces).[8,32,50]The process is regarded as a new type of secondary instability by the width and the angle rating of triangularization.[51]It is noted that due to the flattening of the pressure profile in the magnetic island, the fraction of the local bootstrap current decreases,triggering the neo-classical double-tearing mode(NDTM)instability in RMS, which is different from the positive magnetic shear.In fact, a preliminary study on NDTM had been conducted by Yuet al.and they found that the effects of bootstrap current on the inner rational surface and outer rational surface were quite different.[1,11]In Ref.[52], due to the opposite shear direction of the rational surface in RMS,Yu calculated through analysis that the perturbed bootstrap current has a stabilizing effect on the inner island but a destabilizing effect on the outer island.Moreover, Wanget al.investigated linear and nonlinear NDTMs in RMS configuration with differentΔrsand analyzed the dynamics of complicated motions of magnetic islands by taking different bootstrap current fractions into consideration.[10]However, the previous works have hardly taken into account the effects of plasma radiation.Given the future development of magnetic confinement with RMS configuration, particularly in ITER, exploring the excitation of DTM is therefore crucial.

The aim to study the physical characteristics of future high-performance tokamaks such as ITER,however,demands a more explicit investigation of both the cooling effects induced by the evolution of plasma radiation and the NDTM response which is accountable for the disruption of steady-state operation in the magnetic confinement with the RMS configuration.Therefore,the complicated mechanism of plasma radiation effects on the NDTM with different safety factorqprofiles motivates this work.It is found that the cooling effect of plasma radiation can result in the destruction of NDTM in RMS configuration, especially under high intensities of plasma radiation with smallΔrscases, which should be taken seriously in the steady-state operation of magnetic confinement in the future.

The rest of this paper is organized as follows.The modelling equations are introduced in Section 2.In Section 3,the numerical results of NDTM performance as a result of plasma radiation with small, intermediate and large separations are shown.Finally, summary and discussions of the results are presented in Section 4.

2.Modelling equations

A set of reduced MHD equations in a cylindrical geometry(r,θ,z)is carried out to investigate the nonlinear features of the NDTM with plasma radiation in this work.The evolution equations of magnetic flux,vorticity field and pressure are written as follows:[6,10,34]

withR0being the major radius of the tokamak.

It should be mentioned that in the last term of Eq.(3),Pradis the plasma radiation power density,which results from the bremsstrahlung(Pb)as well as electron cyclotron radiation(Pc).These energy losses in the high-temperature plasma can be calculated through the Born approximation,[47]as

whereniis the ion density,including background ions and impurities.B,t,andare the electron density,the normalized magnetic field,the plasma temperature and the ionic effective charge,respectively.

Usually, the advanced tokamaks choose tungsten as the material for divertors.Thus, tungsten is considered as one of the important impurities in the tokamak discharge.[49,50]In this work,nim0=nim/ne, the ratio of tungsten densitynimto the electron densityne, measures the strength of impurity radiation.The effect of plasma radiation is added into the interior of the magnetic islands.A Gaussian distribution ofPradis assumed inside the magnetic island,which is expressed as

wherePrad0=Pb+Pcis the radiation at the O-point of magnetic islands.In this model,ris the radial position andχis the helical angle in this mole.Furthmore,(r0,χ0)is the center of the Gaussian distribution.Δris the half deposition width in the radial direction andΔχis the half deposition width in the helical angle direction.

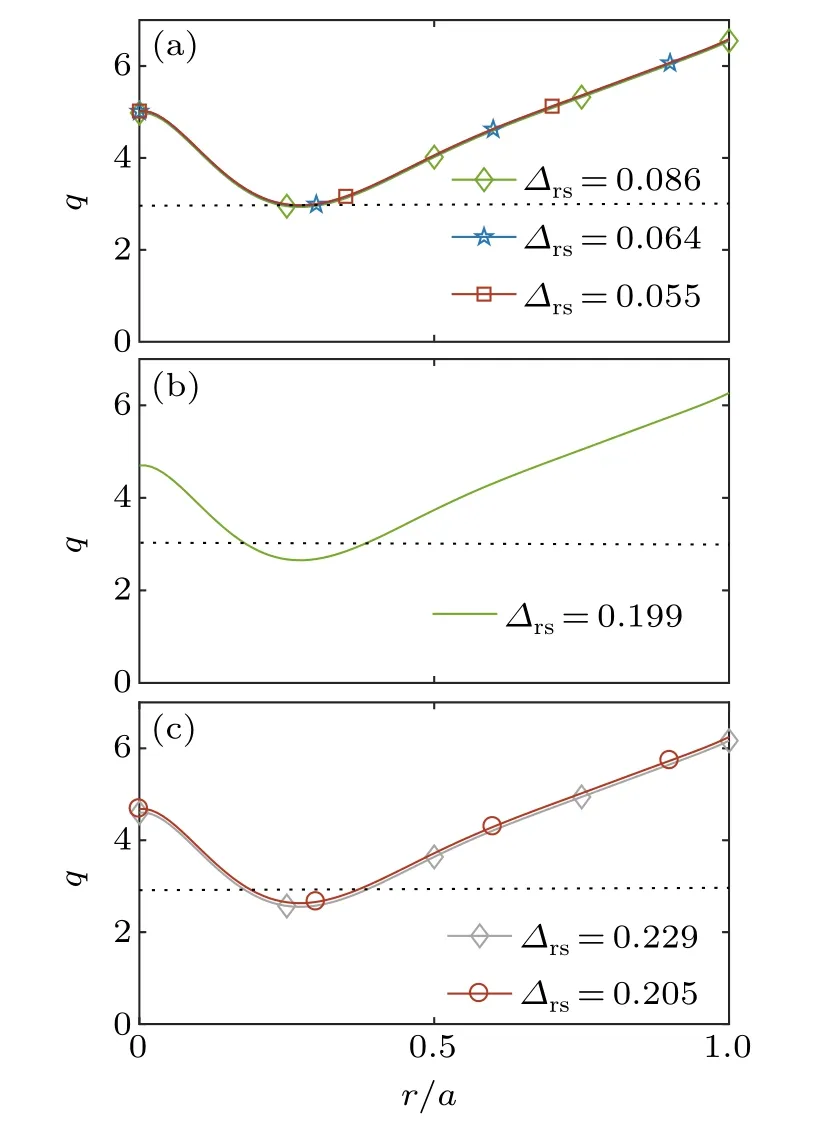
Fig.1.The nonmonotonic safety factor q-profiles with(a)small,(b)intermediate and(c)large separations between the two rational surfaces.

Given the initial equilibrium, the normalized three-field equations can be solved by an initial value code: MDC(MHD@ Dalian Code).[6,10,34,36,48]The finite difference method is employed in the radial direction, while the pseudo-spectral method is used for the angular direction.In addition, the two-step predictor-corrector method is applied in the time advancement.The initial pressure profile is chosen in the form ofp(r)=(1-rp1)p2and the plasma rotation is set asv0(r)=Ω0(1-r),whereΩ0is the plasma rotation frequency on the magnetic axis and normalized by the Alfv´en frequency 1/τA.

In this work,NDTMs with different separations between two rational surfacesΔrsare numerically studied by the radiation MHD model given above.The safety factor profiles with twoq=3 rational surfaces (rs1,2) are shown in Fig.1.The typical parameters are set asp1=2,p2=6,ε=a/R0=0.25,fb=0.2,Δr=0.8w,Δχ=π/3.Γ=5/3 is the ratio of specific heat of plasmas.wis the magnetic island width.The parameters of plasma radiation are approximatively calculated based on the basic parameters of the experiment from EAST,corresponding toB=2T,ni=ne=1019m-3.[53]We measure the electron temperatureTein Kelvin and the electron and ion densities,neandnirespectively in m-3, furthermore, the magnetic field intensityBin Tesla.

3.Numerical results

In this section, the basic characteristics of plasma radiation effects on the NDTM in different RMS configurations with single helicity(h=m/n=3, 0≤m ≤33)are performed for the static equilibrium.At first,the kinetic energy of NDTM with three kinds of different smallΔrsis shown.Then, the time evolutions of nonmonotonic safety factorqprofiles and the NDTM kinetic energy are presented and compared.Furthermore, the kinetic energy with different impurity radiation intensities in the intermediateΔrscase is analyzed.Finally,the interplay and physical mechanism between plasma radiation and NDTMs in the two largeΔrscases are presented and discussed.

3.1.The small Δrs case

The safety factor profiles in three smallΔrscases withΔrs= 0.086,Δrs= 0.064, andΔrs= 0.055 are shown in Fig.1(a).It is found that without plasma radiation, the kinetic energy exponentially increases in the linear phase and then it goes into a phase of quasi-periodic oscillation, as shown in Fig.2 (represents total perturbed kinetic energy of the system).Finally, the NDTM enters a saturation state in the nonlinear phase.However, taking plasma radiation into account, the kinetic energy gradually rises in the nonlinear phase.This phenomenon of gradual growth can be seen clearly aftert ＞8×103τA.As a result, a broad region of MHD turbulence-like behavior is generated, as illustrated in Fig.3(b).The effects of plasma radiation cause the potential distribution to widen radially, leading to the increase of the kinetic energy in the nonlinear phase.For a RMS configuration,in general,the bootstrap current perturbation has a destabilizing effect on the islands near the outer rational surface, but a stabilizing effect on them near the inner rational surface.However, the net effect of the bootstrap current perturbation on the NDTM is destabilization.Since the plasma radiation can play a cooling role in islands and then further modify the bootstrap current profile dramatically, the kinetic energy of NDTMs gradually grows in the nonlinear phase.

Figure 4 shows the time evolution of safety factor profiles in the cases with and without plasma radiation for differentΔrs.In all cases, the twoq=3 rational surfaces move first inwards radially in the early nonlinear phase.In the phase oft ∈■2×103τA,3×103τA■in Fig.4(a), for example, the two rational surfaces move inwards,because the bootstrap current profiles near both rational surfaces are modified differently.[10]Duringt ∈■3×103τA,4×103τA■, they start to move outwards due to the Ohm current, which is recovered through the source termEz0.Afterwards the competition between the bootstrap current modification and the source term may induce different dynamic changes of safety factor profiles.It is worth emphasizing that when the plasma radiation is included, the twoq=3 rational surfaces violently oscillate during the phase of the nonlinear growth of kinetic energy.Furthermore, from Fig.5 it can be seen that the perturbed current is increased near the rational surfaces,which in turn leads to oscillations in the safety factorqprofiles.Moreover,it is observed in Figs.4(b),4(d),and 4(f)that the radial profiles ofq=3 rational surfaces become significantly wider,corresponding to the broad region of MHD turbulence as shown in Fig.3(b).

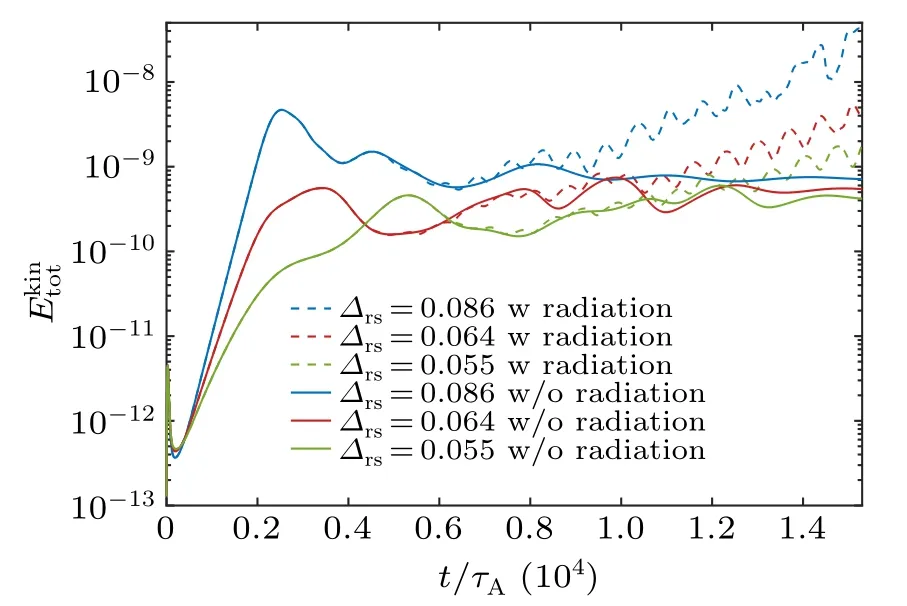
Fig.2.Time evolutions of the kinetic energy of NDTMs in Δrs=0.086,Δrs=0.064,and Δrs=0.055 with/without plasma radiation.

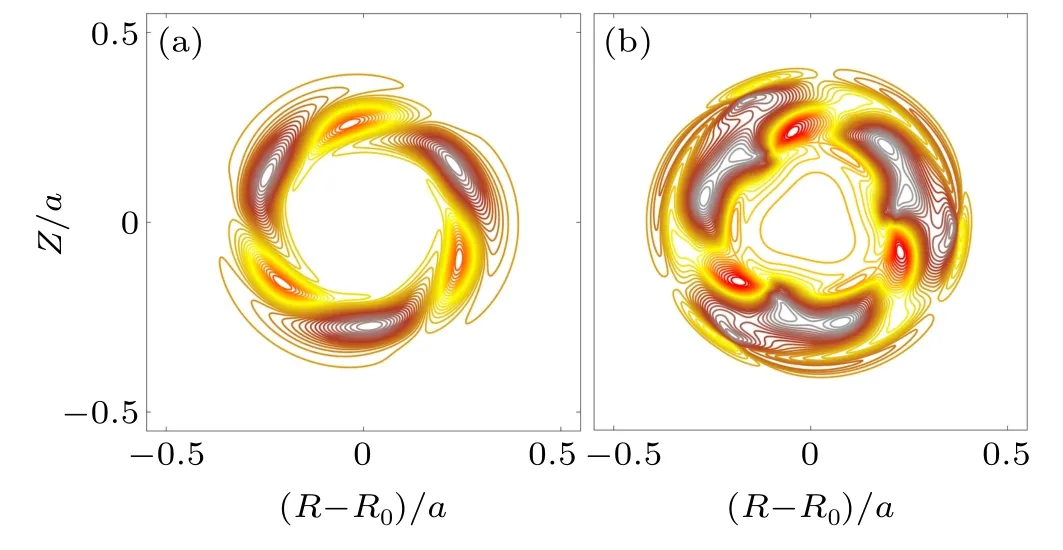
Fig.3.Typical contours of potential in Δrs =0.055 at t =1.2×104τA:(a)without plasma radiation,(b)with plasma radiation.

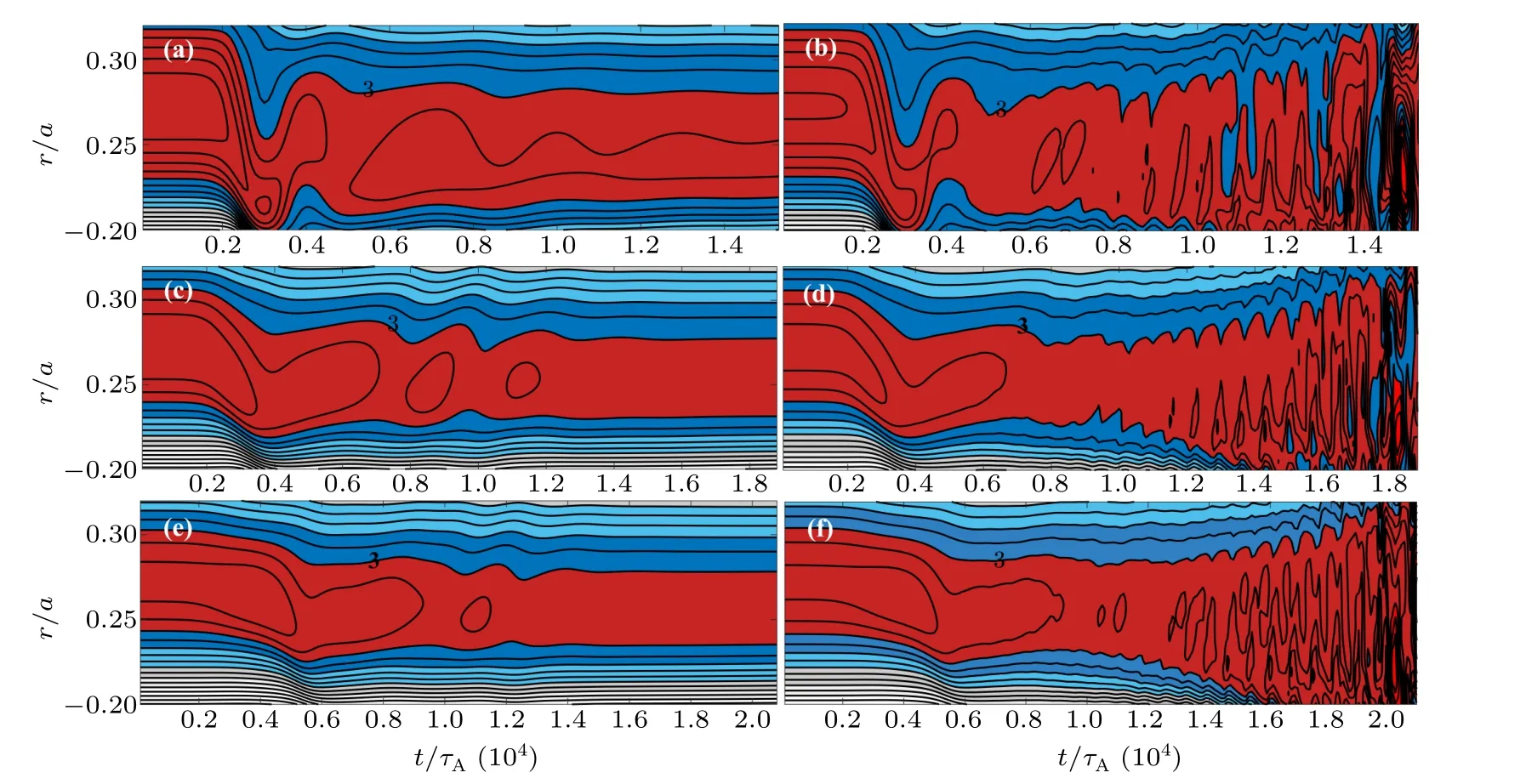
Fig.4.Dynamic changes of safety factor profiles for NDTM in(a)Δrs=0.086 without plasma radiation,(b)Δrs=0.086 with plasma radiation,(c)Δrs=0.064 without plasma radiation,(d)Δrs=0.064 with plasma radiation,(e)Δrs=0.055 without plasma radiation,(f)Δrs=0.055 with plasma radiation.The contour lines with q(r,t)=3 are indicated.

Fig.5.The equilibrium and perturbed current profiles for m/n=0/0 mode in Δrs=0.055.

3.2.The intermediate Δrs case

The nonlinear evolution of the kinetic energies of NDTM with different impurity radiation intensities in the intermediateΔrscase(Δrs=0.199)is given in Fig.6.The safety factor profile in the intermediateΔrscase is shown in Fig.1(b).It is found that an explosive growth phase can be induced by the strong coupling between magnetic islands on both rational surfaces.This explosive burst can lead to a fast driven nonlinear reconnection between both rational surfaces and thus can trigger off-axis sawteeth observed in RMS tokamak plasmas.[51,54]In the early nonlinear phase, the magnetic islands on both rational surfaces continue to grow and drive each other.When the magnetic separatrices of the magnetic islands on both rational surfaces merge together,the field lines of magnetic islands reconnect with the equilibrium field lines.Then the outer islands move inward and inner islands move outward in the radial direction,i.e.,exchanging their radial positions.Moreover,the burst time of such an explosive growth of NDTMs is clearly advanced by the plasma radiation,since it can effectively destabilize the islands on the outer rational surface.Figure 7 gives the typical contours of helical magnetic flux at the same simulation intervalt=8×103τAfor different radiation intensitynim0.Clearly, it is seen through comparing flux contours in Figs.7(a)-7(d)that the fast reconnection process is indeed accelerated by increasingnim0.

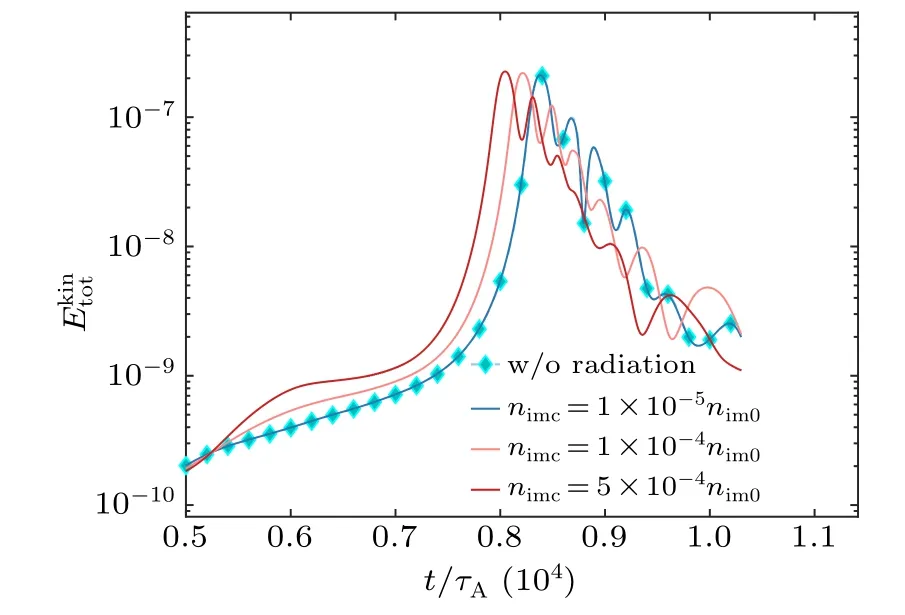
Fig.6.Time evolutions of the kinetic energy of NDTMs in Δrs =0.199 with/without plasma radiation and different impurity radiation intensities.

Fig.7.Typical contours of helical magnetic flux at t=8×103τA:(a) without radiation, (b) nim0 =1×10-5, (c) nim0 =5×10-5,(d)nim0=1×10-4.

3.3.The large Δrs case

In this section,two large separationΔrscases,Δrs=0.229 andΔrs=0.205 in Fig.1(c),are taken into account.Firstly,the physical characteristics of NDTM withΔrs=0.205 are analyzed.Without plasma radiation,no explosive burst of kinetic energy is found, as shown in Fig.8, since the separation between both rational surfaces is too large to trigger the fast reconnection of NDTM.Consequently,the magnetic islands on both rational surfaces are saturated with certain widths in the nonlinear phase,as shown in Figs.9(a)and 9(c).

Fig.8.Time evolutions of the kinetic energy of NDTMs in Δrs =0.205 with/without radiation.

Nevertheless,plasma radiation can induce a sudden burst of fast reconnection.This is because the cooling effect of plasma radiation within the magnetic island can further destabilize the NTM islands.As a result,the magnetic island coupling on both rational surfaces becomes strong enough to trigger fast reconnection in cases with strong plasma radiation effects, similar to the process illustrated in Fig.6.The fast reconnection,accompanied with the exchange of radial positions between inner and outer islands,is indicated in Fig.9(b).

It is noted that, the burst of explosive growth of NDTM is observed in the presence of the plasma radiation.While the NDTM without the plasma radiation effect cannot trigger such a burst for the same separation of two rational surfaces.This means that the NDTM becomes more dangerous,if plasma radiation appears in the RMS configuration.

When the separation is further expanded, such as in the case withΔrs=0.229, the burst still cannot be induced due to weak island coupling even in the presence of plasma radiation.Interestingly,it is noticed in Fig.10 that the inner islands are stabilized, while the outer islands are destabilized.It is known that the plasma radiation plays a role in cooling plasmas in the magnetic islands.Figures 11(a) and 11(b) show the pressure contours, indicating that the pressure profiles in islands with radiation are reduced much more than those without radiation.The decrease of pressure in islands increases the bootstrap current perturbations there.So, it is found that the plasma radiation can further stabilize the islands in the negative magnetic shear region,but destabilize them in the positive magnetic shear region.This is the reason that in spite of no burst,the inner island widths slowly decrease,while the outer island widths slowly increase.

In general,the ratio of parallel to perpendicular transport coefficientχ‖/χ⊥influences the NTMs significantly, since it can modify the pressure flattening and resultant bootstrap current in the magnetic islands.Figure 12 gives the evolution of inner and outer islands for differentχ‖/χ⊥.It is seen that in the nonlinear phase with plasma radiation, increasingχ‖/χ⊥can further destabilize the outer islands in the positive magnetic shear region,but stabilize the inner islands in the negative magnetic shear region.This is due to the fact that increasingχ‖/χ⊥can enhance the pressure flattening within the magnetic islands,and then increase the level of bootstrap current perturbations, which plays a role in stabilizing (destabilizing) the inner(outer)islands.

4.Summary

The nonlinear features of the radiation NDTMs in the RMS tokamak plasmas are numerically studied.The cooling effects of the impurity radiation on the dynamics of the magnetic islands in the cases with different separationsΔrsof twoq=3 rational surfaces are discussed in detail.In this paper,we find that the plasma radiation cannot induce a burst whenΔrsis too large or small, it should be nearly atΔrs=0.205.Furthermore,a burst cannot be induced whenΔrs＜0.199 whether the plasma radiation is considered or not.The results can be summarized as follows.

(i) In the case of a small separation between two rational surfacesΔrs, it is found that the cooling effects of plasma radiation in the magnetic island region can lead to the destabilization of the high harmonic NDTMs.Thus, a broad region of MHD turbulence is generated due to the continuous growth of the kinetic energies of the high harmonic NDTMs.Furthermore,the safety factor profile is found to oscillate violently after considering the plasma radiation effect.The Ohm source,which is proportional to the plasma radiation effect, is found to contribute to the fast oscillation phenomenon in the case of small separationΔrs.

(ii)In the case of intermediate separationΔrsin the RMS configuration,an explosive burst phenomenon induced by the fast reconnection of NDTMs can be clearly observed.Since the cooling effect of the plasma radiation can enhance the destabilizing effects of the bootstrap current perturbation on the NDTMs, it is found that the burst process of the kinetic energy is accelerated with the increase of the plasma radiation effect.

(iii)In the case of large separationΔrs,the NDTMs can be further destabilized by the cooling effects of plasma radiation.It is interesting to find that the explosive burst process of the fast magnetic reconnection can be induced by the plasma radiation in the case of large separationΔrs,even though the explosive burst cannot be induced by the NDTMs in the absence of plasma radiation for the same separationΔrs.Moreover,the stabilization/destabilization effects of the plasma radiation on the inner/outer magnetic islands are analyzed by varying the ratio of parallel transport coefficient to perpendicular transport coefficientχ‖/χ⊥.

Plasma radiation can be used to study plasma impurity composition, plasma densities, ions temperature, safety factors, effective charge, radiated power, etc.It is a general observation in tokamak that the electron densities are limited by an upper threshold, above which MHD instabilities develop that finally cause the plasma current to disrupt in ASDEX Upgrade.[29]Furthermore,it is worth mentioning that the disruption of the density limit is initially caused by impurity radiation.[26]Therefore,with the development of experimental diagnostics and the nonlinear code,it is expected that the numerical results reported here can play significant roles in the future for the prediction of the disruption caused by plasma radiation in tokamak experiments with different separations.

Acknowledgments

Project supported by the National Key R&D Program of China (Grant No.2022YFE03090000), the National Natural Science Foundation of China (Grant No.11925501), and the Fundament Research Funds for the Central Universities(Grant No.DUT22ZD215).