具有罗宾边值条件的一类奇摄动微分方程的内部层

德米 倪明康

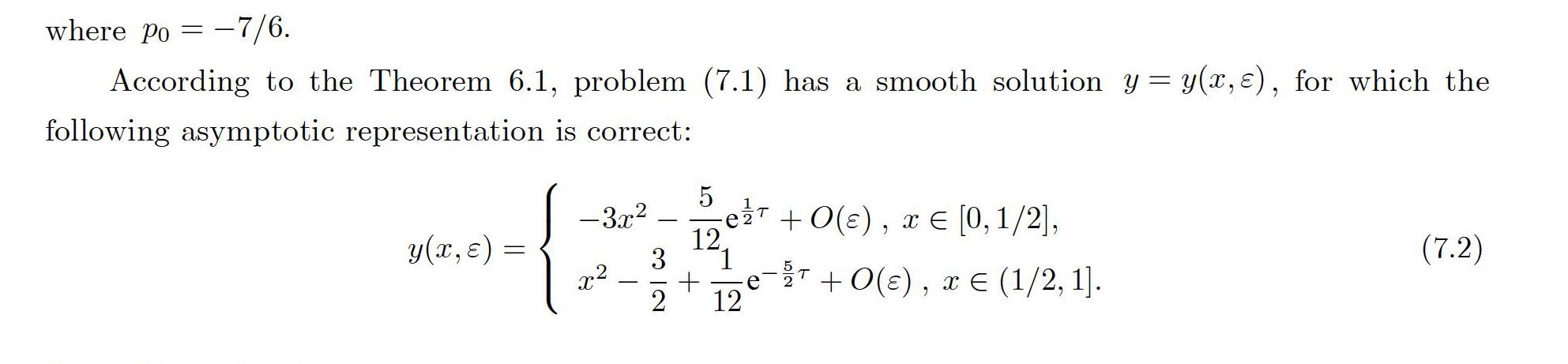
摘要：本文研究了一類具有罗宾边值条件的二阶奇摄动右端不连续微分方程，用边界层函数法构造了该类方程解的渐近表达式，最后用缝接法证明了该问题解的存在性，并给出了渐近解的余项估计.

关键词：奇摄动;渐近表达式;罗宾边值条件;内部层

[参考文献]

[1]NEFEDOV N N， NI M K. The inner layers in the one-dimensional reaction-diffusion equation with a discontinuous reactive term [J] .Journal of Computational Mathematics and Mathematical Physics， 2015， 55（12） ： 2042-2048.

[2] LEVASHOV N T， NEFEDOV N N. ORLOV A O. Stationary reaction-diffusion equation with a discontinuous reactive term [J].Journal of Computational Mathematics and Mathematical Physics， 2017， 57（5）： 854-866. DOI： 10.1134/S0965542517050062.

[3]VASILYEVA A B， BUTUZOV V F. NEFEDOV N N. Singularly perturbed problems with boundary inner layers EJl . Proceedings of theSteklov Mathematical Institute， Russian Academy of Sciences， 2010， 268： 268-283.

[4]VOLKOV V， NEFEDOV N N. Asymptotic-numerical investigation of generation and motion of fronts in phase transition models [M] //. Numerical Analysis and Its Applications. Berlin： Springer-Verlag， 2012： 524-531.

[5] VASILYEVA A B， BUTUZOV V F. Asymptotic Methods in the Theory of Singular Perturbations [M]. Moscow： Higher School， 1990： 208.