Iron isotopic analyses of geological reference materials on MCICP-MS with instrumental mass bias corrected by three independent methods

·····

Abstract Here we report iron(Fe)isotopic data of three pure Fe solution standards(IRMM-014,GSB Fe,and NIST 3126a)and five widely used geological reference materials(RMs)from the United States Geological Survey and Geological Survey of Japan obtained on a Neptune Plus multi-collector–inductively coupled plasma–mass spectrometer(MC-ICP-MS)in our laboratory over the past 3 years.The instrumental mass bias was corrected by three independent methods:sample-standard bracketing(SSB),Ni doping+SSB,and57Fe–58Fe double spike+SSB.Measurements reveal that both the Ni doping and double spike methods helped calibrate short-term fluctuations in mass bias.Collectively,almost all measurements of RMs yielded δ56Fe within ± 0.05 of recommended values,provided that each sample was measured four times on MC-ICP-MS.For the first time,new recommended values for NIST SRM3126a are reported(δ56Fe=0.363 ± 0.006,2SE,95%CI;and δ57Fe=0.534 ± 0.010,2SE).

Keywords Iron isotopic analyses⋅Sample-standard bracketing⋅Double spike⋅Ni doping⋅Reference materials⋅Precision and accuracy

1 Introduction

Iron(Fe),as a major element both in the superficial and inner part of Earth,naturally has 0,+2,and+3 valences and buffers the redox state of geologic systems(Beard and Johnson 2004;He et al.2015b;Dauphas et al.2017).Iron has four stable isotopes:54Fe,56Fe,57Fe,and58Fe.With its potential to trace geologic processes ranging from planetary formation to inter-mineral diffusion,and in particular redox state change,Fe isotope geochemistry has gained intensive interest from the community in the last 20 years(He et al.2015b;Dauphas et al.2017).

Precise and accurate isotopic analyses are required to reveal the limited Fe isotope fractionation in many geologic samples(e.g.,most igneous rocks have δ56Fe in a range of 0.10±0.20)(He et al.2015b;Dauphas et al.2017).Following Strelow(1980),Fe can be nearly quantitatively recovered from matrices using anion resins in most labs(He et al.2015a;Dauphas et al.2017;and references therein).The initial attempts on Fe isotopic analyses were conducted on a thermal ionization mass spectrometer(TIMS)using the double spike technique to correct instrumental mass bias(Johnson and Beard 1999;and references therein).Limited by the difficulty of ionization and non-ideal source evaporation,measurements on TIMS yield poor precision,typically ± 0.6(2SD)in δ56Fe(Johnson and Beard 1999;Fantle and Bullen 2009).Currently,most reported Fe isotopic data are measured on a multi-collector inductively coupled plasma–mass spectrometer(MC-ICP-MS)(Belshaw et al.2000;Weyer and Schwieters 2003;Dauphas et al.2009,2017;Millet et al.2012;He et al.2015a;Chen et al.2017),and the long-term reproducibility of δ56Fe can be better than 0.03(e.g.,Dauphas et al.2009;Millet et al.2012;He et al.2015a).ForFe isotopic analyseson MC-ICP-MS,isobaric interference and mass bias correction are the two major factors that limit precision and accuracy(e.g.,Dauphas et al.2009;He et al.2015a).The argon plasma source produces intensive argide inference (e.g.,40Ar14N,40Ar16O,40Ar16O1H)on the mass spectrum near Fe isotopes.Pseudo high-resolution(e.g.,on Neptune Plus and Nu II/III)is widely used and allows Fe ions to be partially discriminated from argide inference and measured on narrow low-mass-shoulders(Δmass＜0.010 atomic mass unit)(Weyer and Schwieters 2003).The new generation of MC-ICP-MS(e.g.,Nu Plasma 1700)can allow complete resolution of Fe ions from argide interference(Chen et al.2017).To correct instrumental mass bias,sample-standard bracketing(SSB)is routinely used(Dauphas et al.2017).The SSB method assumes that mass bias during sample measurements is the same as that during bracketing standard measurements,an assumption that is valid only when conditions between sample and standard solutions(e.g.,concentration,purity,and acidity)are perfectly matched(He et al.2015a;Dauphas et al.2017;and references therein).Such matching and stability of instrumental mass bias is occasionally difficult to achieve,especially when special samples(e.g.,seawater)are treated or MC-ICP-MS is aging.In this case,double spike or elemental doping in combination with SSB can be adopted(e.g.,Malinovsky et al.2003;Weyer and Schwieters 2003;Arnold et al.2004;Poitrasson and Freydier,2005;Lacan et al.2008;Millet et al.2012;Conway et al.2013;Finlayson et al.2015;Chen et al.2017).

This study reports Fe isotopic data of three solution standards(IRMM-014,GSB Fe,and NIST SRM3126a)and five widely distributed reference materials(BHVO-2,BCR-2,GSP-2,JP-1,and AGV-2)from the United States Geological Survey and Geological Survey of Japan during 2014–2018 at the Isotope Geochemistry Laboratory,China University of Geosciences,Beijing(CUGB).Mass bias was corrected through three independent methods:SSB,double spike+SSB,and elemental(Ni)doping+SSB.The long-term reproducibility of δ56Fe was better than 0.05,provided that each sample was measured four times on MC-ICP-MS,irrespective of the mass bias correction methods used.Except for a few outliers,standards measured in study yielded results consistent with updated recommended values(Craddock and Dauphas 2010;He et al.2015a;Sossi et al.2015).Compared to SSB alone,additional double spike and Ni doping corrections both helped overcome short-term fluctuations of the instrumental mass bias.For the first time,new reference values for NIST SRM3126a are given,facilitating future interlaboratory comparison.

2 Experiments

2.1 Chemical procedures

Sample dissolution and Fe purification procedures are after Dauphas et al.(2009)and have been reported elsewhere(He et al.2015a).Only a brief description is given below.Sample powders containing about 250 μg Fe were dissolved in a mixture of concentrated HF-HNO3acids,and then refluxed in aqua regia and HNO3-enriched aqua regia(HNO3:HCl=2:1).The dissolved samples were dried and re-dissolved in 0.5 mL 6-N HCl,and an aliquot of each sample solution containing about 100 μg Fe was processed through the column chemistry.Iron was separated from matrices by 1 ml AG1-X8 resin(200–400 mesh chloride form,Bio-Rad,Hercules,CA,USA)in a HCl medium.Matrix elements were removed using 8 ml 6-N HCl,and then Fe was collected with 9 mL 0.4-N HCl.The same column chemistry was typically performed twice to ensure the purity of the collected Fe.Recovery of Fe was＞99.5%,and the whole procedure blank was routinely below 10 ng and rarely reached 50 ng—negligible compared to the 100 μg sample Fe processed.The purified samples were dried with drops of HNO3to reduce possible organic materials from resins,and dissolved in 3%HNO3(v/v)prior to isotopic analyses.

2.2 Mass spectrometry

Iron isotopic ratios were measured on a Thermo Fisher Neptune Plus MC-ICP-MS at CUGB on high-resolutions modes(also referred to as medium-and high-resolution modes,e.g.,Weyer and Schwieters 2003).Samples were introduced through a Cetac auto sampler and Thermo Scientific Stable Introduction System(SIS)which combines quartz cyclonic and Scott type spray chambers and PFA Te flon self-aspirating micro-nebulizers with 50–100 μl/min uptake.53Cr,(54Cr+54Fe),56Fe,57Fe,(58Fe+58Ni),and60Ni were measured at L3,L1,Central,H1,H2,and H4 respectively,with a56Fe intensity typically around 15±5 V.Concentrations of unknown sample and bracketing standard solutions were well matched within 10%;the same batch of 3%HNO3was used to prepare all solutions to eliminate the effects of acid molarity mismatching.The same 3%HNO3was measured at the beginning of each sequence,and signals were subtracted for all the standards and samples as ‘‘On Peak Zero’’(OPZ)(Dauphas et al.2009;He et al.2015a).Each isotopic analysis consisted of a 30 s(s)baseline,3 s idle time prior to integration,and 25 cycles with 8.389 s integration each.Data were deduced of fline and reported in the traditional δ notation relative to IRMM-014, δiFewhere i is 56 or 57.

2.3 Instrumental mass bias correction

Instrumental mass bias correction for Fe isotopic analyses relies on the SSB method(Dauphas et al.2017).For the measurements in our lab before April 2016,we commonly adopted the SSB method alone for mass bias correction,details of which have been previously reported(He et al.2015a).After April 2016,short-term fluctuation in instrumental mass bias became frequent,due to aging of the MCICP-MS and/or failure of the temperature control system of the lab.To ensure accuracy and facilitate efficient measurements,we attempted to overcome these mass bias fluctuations by introducing the57Fe–58Fe double spike and Ni doping methods in addition to SSB.

2.3.1 Double spike method

The57Fe–58Fe double spike used in this study was prepared by mixing57Fe and58Fe single spikes from the Oak Ridge National Laboratory(USA)according to the optimized proportion calculated by Rudge et al.(2009).The prepared double spike was measured for its isotopic composition on MC-ICP-MS by SSB(Table S1).The double spike was typically mixed with unknown samples after purification,as in previous studies(Millet et al.2012;Finlayson et al.2015).Given the near complete recovery of Fe in the column chemistry(He et al.2015a),there was no artifact on measured isotopic ratios.The optimized proportion of double spike(q)in the mixed Fe solutions was near 50%(Rudge et al.2009).We adopted q～20%to reduce consumption of double spike.The few sessions with q ranging from 20%to 50%indicate this compromise had little effect on the precision and accuracy of Fe isotopic data(Table S3).Double spiked IRMM-014 solutions were measured bracketing every three to five unknown samples.The data were deduced off line using an Excel macro modified after He et al.(2017)that can be provided by the authors upon request.Typically,deduced56Fe/54Fe ratios for IRMM-014 in a single session were consistent within ± 0.10‰ (Fig.1).δ56Fe values were calculated for unknown samples relative to the mean56Fe/54Fe ratio of IRMM-014 in each session.Occasionally,time-related shifting in deduced56Fe/54Fe ratios for IRMM-014 was observed.If such shifting was＜0.10‰ between each two adjacent measurements on bracketing IRMM-014 in the whole session,δ values were calculated for unknown samples relative to the mean ratios of their bracketing IRMM-014.Otherwise,the data were rejected,and the samples were reanalyzed after optimizing MC-ICP-MS conditions.

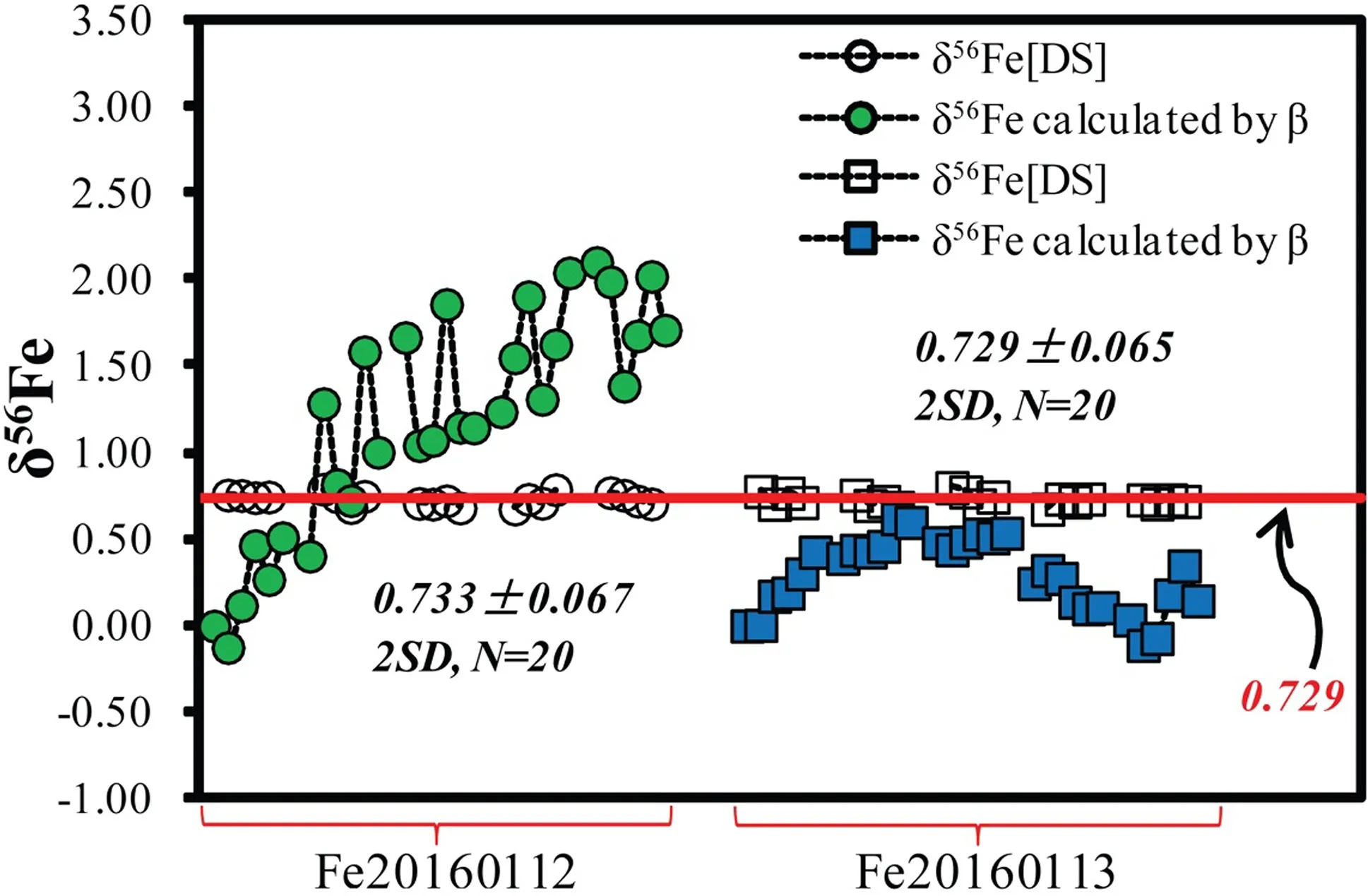
Fig.1 Mass bias correction by the57Fe–58Fe double spike method illustrated by two representative sessions (Fe20160112 and Fe20160113).Every four GSB measurements were bracketed by one IRMM-014 measurement.δ56Fe values for GSB Fe(open symbols)were calculated using56Fe/54Fe ratios with mass bias corrected by the double spike method relative to IRMM014.For a comparison,the mass bias(β)shifting during the sessions( filled symbols)is also shown in corresponding δ values relative to the β value of the first measurement of each session.The red line represents the recommended δ56Fe value of GSB Fe(0.729)(He et al.2015a)

2.3.2 Ni doping method

Elemental doping is widely used for isotopic measurements on MC-ICP-MS to overcome short-term fluctuations in instrumental mass bias(Marechal et al.1999;Arnold et al.2004;Poitrasson and Freydier 2005;Dauphas et al.2017).This method assumes that the mass bias factor for isotopes of the target element is the same as,or in linear covariation with,isotopes of doped element.Copper and Ni were used in previous studies to correct mass bias for Fe isotopic analyses(Dauphas et al.2009).Given that63Cu and65Cu cannot be measured simultaneously with Fe ions,peak jumping is required;the duration of the measurements is significantly extended.Therefore,we adopted Ni doping with mass bias corrected by60Ni/58Ni after Oeser et al.(2014)and Chen et al.(2017).The single element GSB Ni solution(1000 ppm)from China Iron and Steel Research Institute was dried and dissolved in 3%HNO3(v/v)as a 100 ppm solution.This diluted GSB Ni solution was mixed with purified unknown samples and bracketing standards targeting Ni:Fe of 1:1.In this condition,the doped Ni does not significantly affect the mass bias for Fe isotopic analyses(He et al.2015a).GSB Fe solutions doped with Ni were measured bracketing every two or three unknown samples.

For data deduction,mass basis on MC-ICP-MS is assumed to follow the exponential law:

The58Fe interference on58Ni was corrected based on54Fe signals,assuming58Fe/54Fe=0.0480734 given βFecalculated from measured56Fe/54Fe against 15.6986(Taylor et al.1992).It has been noted that such correction works well only for terrestrial samples,for which no mass-independent isotopic variation has been observed(Dauphas et al.2017).Natural mass-dependent Fe isotopic variations were compensated during the correction and will not cause deviation on measured60Ni/58Ni. A true60Ni/58Ni=0.385199 was adopted after(Gramlich et al.1989).In reality,βFeis usually not the same as βNi(e.g.,Malinovsky et al.2003;Poitrasson and Freydier 2005;Chen et al.2017). βFeand βNifor bracketing standards were calculated for each measured session.In the case that variation between βFeand βNiwas＞～0.016(e.g.,＞ 0.60 measured by δ56Fe),a linear regression between them was performed and used to calculate βFefor unknown samples.Otherwise, βFe= βNiwas adopted,due to the large uncertainty for the linear regression.Calculations demonstrate that such approximation had negligible effect on Fe isotopic compositions measured for unknown samples,e.g.,＜0.01 in δ56Fe.Data were treated off line;δ values were calculated relative to bracketing GSB Fe standards and reported relative to IRMM-014(He et al.2015a).

2.4 Internal errors

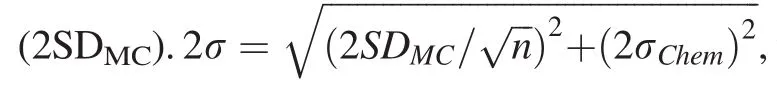
3 Results and discussion

3.1 Correction of short-term fluctuations in mass bias

The double spike technique has been considered to calibrate well for instrumental mass bias(Lacan et al.2008;Rudge et al.2009;Li et al.2011;Millet et al.2012;Conway et al.2013;Finlayson et al.2015;He et al.2017).However,this may not always be true for Fe isotopic analyses,due to the narrow mass spectrum shoulder allowing accurate analyses, fluctuations of argide interference intensity,and difficulty in58Ni correction on58Fe(Millet et al.2012;Finlayson et al.2015).Mismatching of acidity between unknown samples and their bracketing standards can cause mass-independent measured δ56Fe and δ57Fe based on SSB measurements(He et al.2015a),and thus may also lead to inaccurate results for Fe isotopic analyses using the double-spike technique.Furthermore,only one independent ratio can be obtained by doublespiked runs,given that Fe has only four isotopes;any massindependent effect on mass bias cannot be identified by raw data of unknown samples themselves.Special cautions on the reproducibility of measured isotopic ratios for geological reference materials with known isotopic composition and bracketing standards in each session are required to ensure accuracy.

As illustrated by two representative sessions measuring only GSB and IRMM-014 Fe(Fig.1),we demonstrated that short-term fluctuations in mass bias can be well calibrated by the double-spike technique.Typically,measured ratios of GSB and IRMM-014 Fe are stable.Abrupt shifting in measured ratios observed in previous studies(e.g.,Millet et al.2012)due to argide interference burst was not observed in the present study.We attribute this to good resolution of Fe ions from argide interference and magnetic field stability on the Neptune Plus.Occasionally,smooth shifting in measured ratios of bracketing standards can be observed.In the same conditions,tests on unspiked GSB Fe solution also reveal shifting on(57Fe/54Fe)Nwith mass bias internally corrected by56Fe/54Fe=15.6986(Taylor et al.1992),suggesting shifting of the magnetic field and gradual deviation from the optimized mass for Fe isotopic measurement.Except for a few cases,such shifting can be eliminated by calculation of δ values relative to bracketing standards as suggested by Millet et al.(2012).As shown previously(He et al.2015a),the GSB Fe solution contains detectable Ni with60Ni/54Fe=0.000121±5(2SE),corresponding to58Ni/58Fe～0.0653.With58Ni interference being monitored by60Ni and corrected using60Ni/58Ni measured on a 100 ppb GSB Ni at the beginning of each session,runs on double-spiked GSB Fe yielded accurate δ56Fe.This con firms that58Ni correction is not a problem for double-spiked Fe isotopic analyses in our lab at the quoted precision level(see Sect.3.2).

The double spike method has difficulty identifying mass-independent effects based on raw data of unknown samples themselves.To overcome this,we attempted to measure Fe isotopic ratios with the Ni doping method.As shown in Fig.2,introduction of the Ni doping method can well calibrate the mass bias shifting on MC-ICP-MS.In the case of short-term fluctuations in mass bias during session measurements,the Ni doping method can significantly improve reproducibility and accuracy.As exemplified by the session Fe20170221(Fig.2),the reproducibility of δ56Fe was poor～ 0.37(2SD)if calculated based on measured56Fe/54Fe directly,relative to bracketing standards without mass bias correction,but the reproducibility was improved to an acceptable level＜0.05(2SD)when mass bias was corrected by60Ni/58Ni.Furthermore,both representative sessions(Fe20170221 and Fe20170223)yielded56Fe/54Fe with mass bias corrected by60Ni/58Ni constant within±0.05 for bracketing Ni-doped GSB Fe solutions.This allows accurate measurement of several unknown samples between each two adjacent bracketing standards in routine sessions. δ57Fe and δ56Fe can be independently measured by the Ni-doping+SSB method(e.g.,Fig.3 and Table S3),which allows monitoring massindependent effects of all data.

3.2 Precision and accuracy

Results of three pure Fe solution standards(IRMM-014,GSB Fe,and NIST 3126a)and five widely used geological reference materials(RMs)from the United States Geological Survey and Geological Survey of Japan obtained in our laboratory over the past 3 years are reported in Table S2-3,summarized in Table 1,and shown in Figs.3 and 4.No significant interference can be identified in the three-isotope diagram (Fig.3).The long-term reproducibility of δ56Fe for almost all standards,measured by 2SD,was better than±0.05(Table 1),provided that each duplicate measurement consisted of multiple analyses(see Table S2–3)on MC-ICP-MS.The only exception was duplicate measurements on GSP-2 using the double spike+SSB method,which yielded a reproducibility of 0.060.Note that these duplicate double-spike measurements on GSP-2 yielded δ56Fe consistent with the recommended value(He et al.2015a)within±0.04(Table S2).The slightly large reproducibility for this standard(～0.06)may be due to the low number of duplicate measurements.The mean δ56Fe values of all solution and rock standards are consistent with updated recommended values(He et al.2015a)within±0.02,ensuring the accuracy of data obtained here.

Fig.2 Mass bias correction by Ni doping+SSB illustrated by two representative sessions(Fe20170221 and Fe20170223)and only the results of bracketing Ni-doped GSB Fe solutions are shown.In Fig.2a,the shifting of mass bias was expressed as δ56Fe values( filled symbols)relative to the first measured ratio,e.g.,δ56Fe=[(56Fe/54-Fe)measured/(56Fe/54Fe) fi rst-1]*1000.For comparison,δ56Fe values(open symbols)relative to the first measured ratio with Ni-doping correction are also plotted.In Fig.2b,we compare δ56Fe values relative to the average56Fe/54Fe ratios of the bracketing measurements with or without mass bias correction by Ni-doping

Fig.3 Three isotope plot of Fe isotopic data obtained in this study.The regression slope between the measured δ57Fe and δ56Fe is 1.472,consistent with what is expected by the equilibrium fractionation law(1.475)(Young et al.2002)within uncertainty

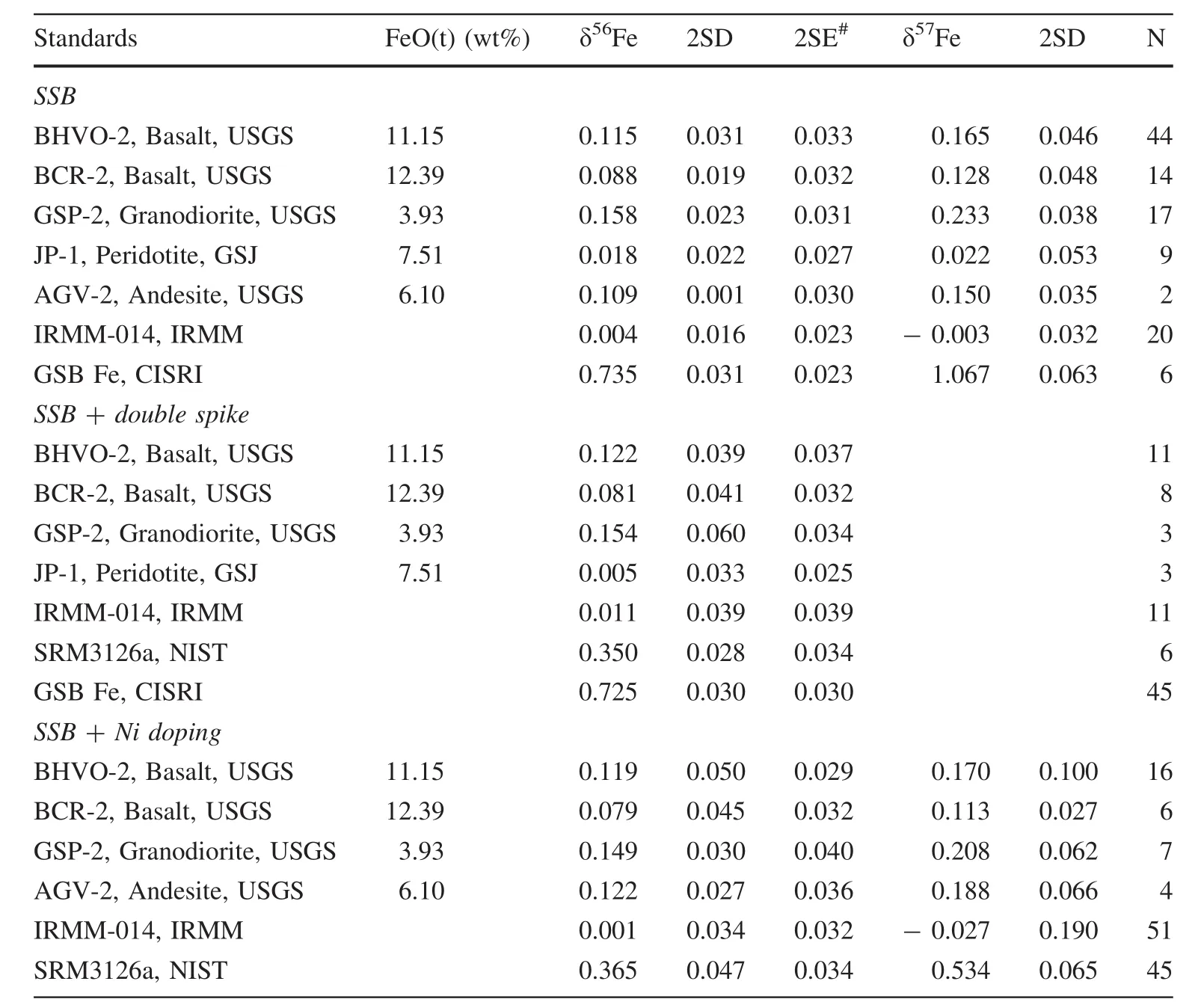
Table 1 Average iron isotopic compositions of standards measured during Sept.2014 to Jan.2018

Previous studies have shown that the long-term reproducibility and accuracy for Fe isotopic analyses can be improved by increasing measurement times on MC-ICPMS for each sample(Poitrasson and Freydier 2005;He et al.2015a).He et al.(2015a)reported that the long-term reproducibility and accuracy for δ56Fe were both better than 0.03 in our lab,provided that each sample was measured nine times on MC-ICP-MS with mass bias corrected by SSB.The data reported here demonstrate that accuracy at this level were routinely reached in our lab when the MC-ICP-MS was optimized and SSB was used(see the SSB data,summarized in Table 1 and shown in Fig.4).It is difficult,however,to directly demonstrate whether δ56Fe can be measured with a long-term reproducibility and accuracy of＜0.03 when mass bias of the MC-ICP-MS fluctuations and Ni-doping+SSB are used,given the limited dataset for standards with nine measurements each on MC-ICP-MS(Table S4).We assessed this issue using the Monte Carlo method.Nine ‘‘measured’’δ56Fe values were randomly collected from raw data for single measurement on each geological standard obtained by Nidoping+SSB(Table S5).The mean δ56Fe of these nine measurements was then calculated.Such calculation was repeated 200 times,generating a representative dataset;the long-term reproducibility is given in Table 2 and predicted to be＜0.03.These calculations suggest that application of the Ni-doping method can improve the long-term reproducibility and accuracy for δ56Fe to＜0.03 if each data consists of nine duplicate analyses on MC-ICP-MS,despite the fluctuating instrumental mass bias observed in our lab.This view is supported by the limited data with nine measurements each on MC-ICP-MS using Ni-doping+SSB,all of which give δ56Fe consistent with updated recommended values within±0.03(Table S4).

Fig.4 Iron isotopic compositions of 3 pure Fe solution standards(IRMM-014,GSB Fe and NIST SRM3126a)and 5 geological reference materials(BHVO-2,BCR-2,GSP-2,JP-1,and AGV-2)measured during a period from Sept.2014 to Jan.2018.Instrumental mass bias was corrected via three independent techniques,including SSB,double Spike+SSB,and Ni doping+SSB.Recommended values(RV)are from He et al.(2015a),while the reference value for NIST SRM3126a is provided in this study.Note that recommended values from He et al.(2015a)were calculated by a compilation of Fe isotopic data from ten independent labs

Fig.4 continued

Table 2 Mean and reproducibility(in 2SD)of Fe isotopic analyses with each sample being measured nine times on MC-ICP-MS predicted by Monte Carlo calculations based on raw data obtained for each geological standard in our Lab using Ni-doping+SSB

As an important indicator of data quality for Fe isotopic data of individual unknown samples,internal errors should be properly reported.Internal errors are usually reported in different ways for Fe isotopic data.One way is to use 2 standard deviations of results of multiple analyses on each sample(e.g.,Millet et al.2012;Xia et al.2017;Zhao et al.2017).Another way is to report internal errors as 2 standard errors of the mean for multiple analyses on each sample with or without the Student’s t-correction(Poitrasson and Freydier 2005;Weyer and Ionov 2007).The routine way to report internal errors adopted in our lab is after Dauphas et al.(2009)and detailed in Sect.2.4.Brie fly,internal errors were calculated based on the reproducibility of measurements on a large number of bracketing standards in each session and on the number of multiple analyses on each sample,with errors arising from the chemical procedures considered.Note that we report internal errors for double-spiked runs as 2 standard errors of the mean for multiple analyses on each sample.Most standards plotted along a 1:1 line in long-term reproducibility(2SD)versus average 2SE,especially for representative samples with large numbers of duplicate measurements(Fig.5).With accuracy given by the absolute difference between the measured and recommended δ56Fe,internal errors reported here are larger than the accuracy for most(＞90%)measurements(Fig.6).For the remaining measurements,internal errors largely agree well within the accuracy.Collectively,Figs.5 and 6 demonstrate that internal errors reported in this study are proper and,in most cases,conservative.

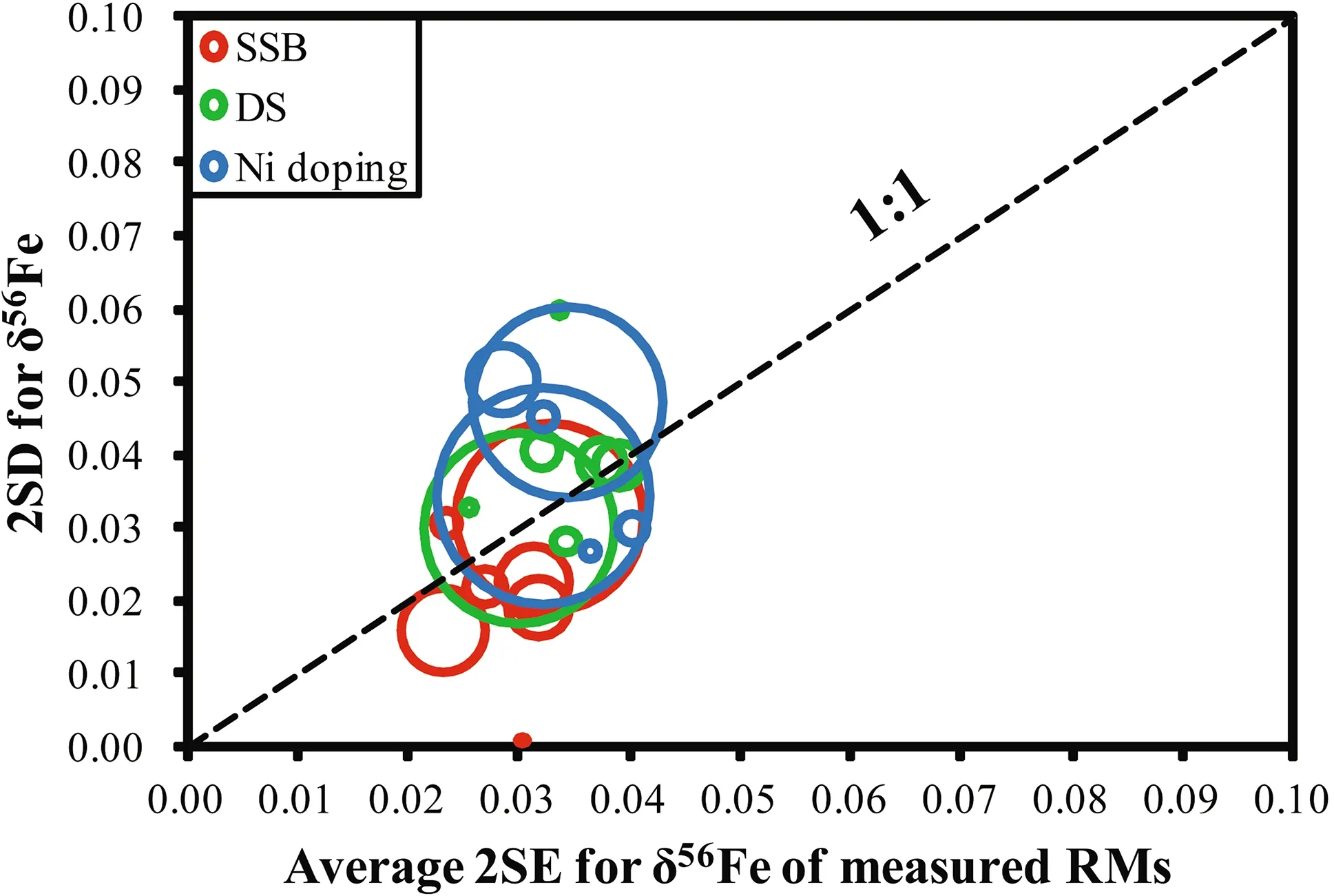
Fig.5 Summary of the relationship between the long-term reproducibility(2SD)and average 2SE of individual measurements for the same standard solutions and RMs.Most standards are plotted along a 1:1 line,especially for representative ones with large numbers of duplicate measurements,suggesting that internal errors reported in this study are proper.The larger bubble sizes reflect more duplicate measurements on individual samples

Fig.6 Summary of the relationship between the absolute difference between the measured and recommended δ56Fe values and internal errors(2SE)reported.MV,RV,and RMs refer to measured values,recommended value,and reference materials,respectively.RV is from He et al.(2015a).Except one out lier,all measured δ56Fe for solution standards and RMs are consistent with RV within±0.05,and thus ensure accuracy in the same level.Furthermore,internal errors reported are larger than the accuracy for most(＞90%)measurements.For the rest measurements,internal errors largely agree well with the accuracy.Again,this plot demonstrates that internal errors reported in this study are proper and,in most cases,conservative.Results of NIST 3126a are not shown in this plot,since its RV only based on data from this study

3.3 New recommended values

Except for NIST SRM3126a,all solution standards and geological reference materials measured in this study yield mean δ56Fe values consistent with the recommended values given by He et al.(2015a)within±0.01(Fig.4).Therefore,updating the recommended values for these standards is not necessary given the current analytical uncertainty.NIST SRM3126a has been previously measured at WHOI(δ56Fe=0.390 ± 0.130,2SD,N=10;Rouxel and Auro 2010)and IFREMER(Brest,France)(δ56Fe=0.420 ± 0.070,2SD,N=10;Chever et al.2015).Six-month measurements in our lab using two independent mass bias correction methods yielded a mean δ56Fe of 0.363±0.046(2SD,N=51),consistent with literature values within uncertainty.Here,we provide new recommended values for NIST SRM3126a(δ56Fe=0.363 ± 0.006, 2SE, 95% CI; δ57-Fe=0.534±0.010,2SE,95%CI)based on the large dataset from this study.Uncertainties are given by 2SD/√N.Literature values are not included because of the lack of information on measurement details.Calibration of NIST SRM3126a can facilitate future inter-laboratory data comparison,given that the supply of IRMM-014 is exhausted(He et al.2015a;Dauphas et al.2017).

4 Conclusions

This study reports a large high-precision Fe isotopic dataset for three solution standards and five geological reference materials,obtained on MC-ICP-MS with mass bias corrected by SSB,57Fe–58Fe double spike+SSB,and Ni doping+SSB over the past 3 years in Isotope Geochemistry Lab,China University of Geosciences,Beijing.The dataset demonstrates that δ56Fe can be routinely measured with an accuracy≤0.05 or≤0.03,provided that each sample is measured on MC-ICP-MS for four or nine times,respectively.Of particular importance,we have illustrated our attempts to overcome short-term fluctuation in the instrumental mass bias using the double-spike and Nidoping methods.New recommended values for NIST SRM3126a are given,with δ56Fe=0.363 ± 0.006(2SE)and δ57Fe=0.534 ± 0.010(2SE).

AcknowledgementsEfficient editorial handling by Dr.Wang Binbin and Miss.Yue Peng and comments from Dr.Huang Jian and two other anonymous reviewers are highly appreciated.Prof.Jianming Zhu,Dr.Yinghuai Lu,Dr.Lijuan Xu,Miss Ruiying Li,Miss Xunan Meng,Mr.Lixin Zhang,Mr.Ting Gao,and Mr.Yang Wang kindly provided helps during the chemical procedures and MC-ICP-MS measurements.This work was financially supported by the National Natural Science Foundation of China(41473016)and the State Key Laboratory of Geological Processes and Mineral Resources.