Unequal-thickness billet optimization in transitional region during isothermal local loading forming of Ti-alloy rib-web component using response surface method
2018-04-21KeWEIMeiZHANXiaoguangFANHeYANGPengfeiGAOMiaoMENG
Ke WEI,Mei ZHAN,Xiaoguang FAN,He YANG,Pengfei GAO,Miao MENG
State Key Laboratory of Solidi fication Processing,School of Materials Science and Engineering,Northwestern Polytechnical University,Xi’an 710072,China
1.Introduction
A large-scale component of titanium alloy with thin webs and high ribs that can meet the requirements of high performance and lightweight commonly serves as the key load-bearing structure in an aircraft.A typical large-scale Ti-alloy rib-web component(LTRC)is shown in Fig.1,of which the length is greater than 1300 mm,and the width is approximately 1000 mm,but the largest rib width is only 18 mm.The manufacture of this component by a conventional method not only involves a tremendous forming load but also produces forming defects easily in terms of larger geometric size,complex structure,and difficult-to-deform property of titanium alloy.At this point,development of an alternative manufacturing technology is necessary for decreasing the forming load and achieving a defect-free LTRC.Isothermal forming can reduce the deformation resistance of a material as well as the forming load of a press.The local loading technique can reduce the required load and control the forming defects by reducing the loading area and controlling the material flow.By combining the advantages of both isothermal forming and local loading,an isothermal local loading forming(ILLF)method was proposed by Yang et al.1–5to form an LTRC.According to the local loading feature,the LTRC is divided into three loading regions,which are the first-loading region,the second-loading region,and the transitional region,respectively,as shown in Fig.1.The forming process of local loading is implemented by alternation of loading dies,which will be discussed in Section 2.2.
The region near the die partition line is called the transitional region,which coordinates two alternated loading regions and takes a bridge effect between the first-and second-loading regions.Gao et al.6pointed out that a flowing material can transfer from a loading region into an unloading region during a forming process and twice transverse material flow with opposite directions occurs in the first-and second loading steps sequentially.Furthermore,Gao et al.7concluded that the transferred material is the conclusive reason for the folding defect in the transitional region.Thus,how to decrease the transferred material in the transitional region is an urgent problem to be solved.Besides,since the material flow in the transitional region is rather complicated comparing with the whole loading forming,how to improve the die filling capability to reach a high-precision shape of the transitional region is another problem to be solved.
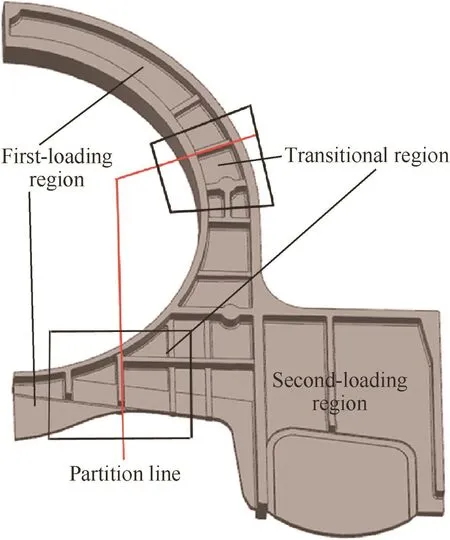
Fig.1 A schematic diagram of a typical LTRC.
By now,extensive investigations focused on the transferred material and folding defect in the transitional region of ILLF have been carried out.Zhang and Yang8disclosed that the transferred material is determined by geometrical parameters of the transitional region.They also qualitatively described the material transferred from a loading region into an unloading region.However,they didn’t provide an effective method for decreasing the transferred material.Gao et al.5revealed that using more loading passes to obtain a smaller reduction amount in each loading step is an effective method to avoid the folding defect.Nevertheless,Fan9presented that increasing loading passes would cause a workpiece to undergo a series of thermal cycles.In this case,the component would be in a high temperature state for a long time,which may result in grain coarsening and prolonging the manufacture procedure.This is inconsistent with the needs for a high-performance component and a low-cost production.Therefore,increasing loading passes is not the priority selection to avoid the folding defect in the transitional region.Moreover,Sun et al.10revealed that the positioning of the partition line along a rib is preferable for the transferred material filling in the cavity of that rib prematurely,which is beneficial to the forming quality of the transitional region.Gao et al.7analyzed the effects of process parameters on the transferred material.It was concluded that decreasing the spacer block thickness and increasing friction could decrease the quantity of the transferred material.They11also revealed that increasing the fillet radii of left and middle ribs in the transitional region is conducive to suppress the folding defect.The aforementioned research provided some good guidance for improving the forming ability of the transitional region in ILLF.However,either folding or die filling problem was considered in these researches.In the whole forming process,folding and die filling were both investigated by Kim et al.12,Wang et al.13,Park and Hwang14,respectively.It is indicated that folding and filling are two significant problems of common concern in the bulk forming process.On this basis,further research is required to consider both folding and filling in the transitional region of ILLF.
In decades,the finite element(FE)method integrated with an optimization design method for preforming billet has been extensively used for analyzing and controlling forming defects in the bulk forming process.By this means,the detailed locations of defects can be traced,and then subsequent modification or optimization of the billet can be made accordingly.
Torabi et al.15applied the Deform-3D FE software to simulate a blade forging process,in which the maximum filling ratio of the final die to the minimum flash volume,the forging force,and the strain variance of the final blade were considered as objectives of optimization,and then an optimized preforming billet with an extruded elliptical cross section was obtained by using the response surface method(RSM)and a multiobjective genetic algorithm.Yang et al.16also adopted the RSM to improve the deformation homogeneity of a typical aeroengine disk by optimizing the preforming billet based on FE simulation.Zhao et al.17presented backward simulations of a forging process which could directly provide a preforming billet from the final shape of forging.Lu et al.18combined FE simulation with a topological methodology to propose a criterion for element elimination and addition on the boundary of a preform shape.The objective of preform design is to achieve a desired preforming billet for sufficient filling of die cavity and flash minimization.Guan et al.19employed FE simulation and a quasi-equipotential field method to optimize a 3D preform shape in the hot forging of a pendulum mass,and the results showed that there were no under filling and folding defect.Knust et al.20introduced an evolutionary algorithm to optimize a performing billet by using a connecting rod as a demonstration part.The implemented fitness function was evaluated by considering the material distribution of the final part,the preform volume,and the shape complexity,and then the progressive optimization could be traced according to the FE analysis.
The aforementioned studies indicated that the preforming billet design is an essential stage in the whole loading process,especially for a large-scale complex component.However,those designed performing billets were only focused on the whole loading process.As mentioned above,during a local loading forming process,twice transverse material flow in the transitional region with opposite directions occurs in the first-and second-loading steps sequentially,which does not exist in the whole loading process.It has been demonstrated that the material flow in the local loading forming process is quite different from that in the whole loading forming process.However,those studies for the transitional region of ILLF only adopted equal-thickness billets(ETBs),which cannot adjust the initial material distribution due to the constantvolume principle.Gao et al.5pointed out that optimizing the billet may be an alternative way to avoid the folding defect in the transitional region.In addition,Wei et al.21presented that an unequal-thickness billet(UTB)can adjust the volume distribution with low cost and high efficiency in the whole loading process of an LTRC.The accurate volume distribution of a UTB has been proven to be an effective approach for solving the under filling problem in the forging of an LTRC,which provides a good reference for the geometry of a billet in the transitional region.Based on the above analysis,further research is required to adopt the geometry of a UTB to provide an optimum initial volume distribution,so as to avoid the folding defect and improve the die filling capability in the transitional region of ILLF.
According to the above-mentioned optimization design of preforming billets,the RSM has been widely used in metal forming processes22–24for constructing a surrogate model.However,a surrogate model can also be constructed by other methods such as the Kriging method and artificial neural networks(ANNs).A systematic comparison among various metamodel techniques has been conducted25–27,demonstrating that each technique has its own advantages and disadvantages.Thus,it is difficult to judge which model is the best one.
A Kriging model is very flexible due to the wide range of correlation functions.25The predicted results at the observed values are exactly the same as the actual values due to the ‘exact”interpolation of the Kriging method.It has better global predictions than those of regression analysis.Gao et al.7adopted the Kriging method to determine the forming limit considering defects in local loading forming.It can provide a guideline in the processing design of local loading forming of Ti-alloy rib-web components.Zhang28established a Kriging model to optimize the preform in the forging of a spherical hinge mandrel,and the results showed that the forming quality of the forging made a great improvement.However,the major disadvantage of the Kriging method is that the model construction process can be very time-consuming,especially for a large sample data set.
An ANN model is a nonlinear statistical model,and it must be used to estimate the coefficients of the model.This estimation process is called ‘network training”26.An ANN model can be constructed very easily based on the given input and output and trained to accurately predict process dynamics.This technique is especially meaningful for the complicated physical mechanism in a plastic forming process.A high accuracy ANN model was developed to predict the bending radius of a strip during incremental in-plane bending by Liu et al.29Based on the ANN model,the influence rules of the key parameters on the bending radius were revealed,and the process parameters were optimized.Kim et al.30adopted an ANN model to find the optimal billets for a plane-strain ribweb component in cold forging and for an axisymmetric ribweb component in hot forging.Although an ANN model has high prediction accuracy,it is hard to interpret and understand which variable factor is significant.Besides,it needs lot of input data to guarantee an accurate prediction of the model,so the time consumption of training for the networks can be overlong.
The RSM is a collection of statistical and mathematical methods.26Based on functional evaluation at selected points in a design space,an empirically based RSM model can be created by using second-order polynomial.The significance factor among design variables can be revealed based on significance analysis.Besides,the relationship between the controllable design variables and the objective value can be clearly visualized through a contour graph or 3D response surface plot.The major disadvantage of the RSM method is that interpolation errors are inevitable between the predicted and actual values.Nevertheless,if the errors are small enough(such as less than 10%),the RSM model can be proven to be reliable and acceptable.Two steps can be taken to evaluate the magnitude of the interpolation errors.The first one is to conduct a variance analysis.Then the average and maximum errors can be acquired.The second one is to verify the optimized value by a physical experiment.Satisfying results can also guarantee a good prediction accuracy by the RSM model.Thus,the disadvantage of the RSM method can be reduced or even eliminated based on the above two steps.Therefore,the RSM method was applied to develop surrogate models for the percentage of transferred material and the die under filling rate in this work.
In the present work,the folding evolution and die filling process in the transitional region were investigated by 3D FE simulation and physical experiment using an ETB.Then,the critical value of the percentage of transferred material during the second-loading step was determined by stepwise decreasing the reduction amount in the ETB.This critical value was taken as a constraint condition for avoiding the folding defect in the UTB optimization process.Subsequently,the percentage of transferred material and the die under filling rate were correlated with the UTB’s dimensional parameters by two RSM models.Based on these two RSM models,non-folding parameter combinations and optimum die filling were achieved.Finally,an optimized UTB was obtained and verified by simulation and experiment.
2.Characterization of the transitional region
2.1.Structure of the eigenstructure
In previous studies on ILLF,Sun and Yang31have revealed that the influence of the loading region on the unloading region is a short-range effect,which mainly takes place in the region from the die partition line to the nearest rib of the unloading region.Additionally,as mentioned above,the positioning of the partition line along the rib is beneficial to the forming quality of the transitional region.Therefore,in order to study the influence of the loading region on the unloading region in the transitional region in detail and reflect the detailed forming characteristics of a complex rib-web component,a typical eigenstructure was designed with three transverse ribs as well as two longitudinal ribs,as shown in Fig.2.The geometric parameters of this eigenstructure are shown in Table 1.It can be seen that the thicknesses of some webs and the distances between each rib are not equivalent,neither are the depth/width ratios of the ribs.
2.2.FE modeling
In order to fulfill the local loading forming process,a spacer block was implanted under the bottom die 1 during the first loading step,allowing the bottom die 1 to be protuberated,as shown in Fig.3(a).It can be seen that the first-loading region of a workpiece is above the bottom die 1,and then a forming load can be exerted on the workpiece only by the bottom die 1,not by the bottom die 2.During the second-loading step,as shown in Fig.3(b),the spacer block was removed,and then the two bottom dies remained in an identical horizontal level.By this means,the forming load can be exerted on the workpiece only by the bottom die 2.In this way,the local loading forming procedure can be conveniently applicable in a single-action hydraulic press.Attributing to the symmetrical feature of the eigenstructure in the center of ribs 4 and 5,a half of the FE model was established so as to enhance the simulation accuracy and reduce the simulation time.Besides,the two sides of the eigenstructure were restrained to simplify the FE simulation process in the transitional region of ILLF.The process parameters of the FEM simulation are listed in Table 2.It is noteworthy that the thickness of the spacer block(Hsb)should not be smaller than the reduction amount of the top die(L).Otherwise,the whole LTRC would be in contact with the two bottom dies during each loading step.Then,the subsequent forming load will be overlarge.This is inconsistent with the aim of local loading forming for saving the load.Based on the above analysis,the thickness of the spacer block and the reduction amount of the top die during each loading step were both defined as 14 mm in this research.

Fig.2 Eigenstructure of the transitional region.

Table 1 Geometric parameters of the eigenstructure.
The process parameters of the two loading steps are the same.The thermal events of the forming process can be neglected since isothermal forming is executed under constant high temperature and a low-speed top die.All the dies and the spacer block are considered as rigid bodies in consideration of the stiffness of the dies,which are much higher than that of the billet.The material of the workpiece is TA15 alloy,and its flow behavior was determined by an isothermal compression test which came from the work of Shen.32The elastic deformation of the material was neglected in the FE model.The von Mises yielding criteria and the isotropic hardening rule were applied.A shear friction model was used and the friction factor was determined to be 0.5 according to Zhang et al.33Additionally,the billet was discretized by tetrahedral elements,and the initial average mesh size was approximately 1/5 of the widths of ribs.Meanwhile,automatic remeshing was adopted to avoid meshing-induced singularity.
2.3.Reason analysis of forming defects
2.3.1.Possible forming defects
The local loading forming results of the eigenstructure were investigated by using an ETB firstly.Based on the constantvolume principle,the thickness of the ETB(He)is 23.5 mm which is calculated by the following equation:

According to the forming results after two loading steps,not only folding occurred in web 3 of the eigenstructure,but also undesired die filling was observed in the cavities of ribs 1 and 4.The folding in web 3 and underfilling of ribs 1 and 4 by FE simulation are illustrated in Fig.4(a).The red dots denote the position of the folding in the formed eigenstructure.Besides,the die filling capability can been directly captured through the minimum distance,which represents the distance between the workpiece and the die cavity.

Fig.3 FE model of the transitional region.

Table 2 Process parameters of the FE simulation.
Park and Hwang14and Torabi et al.15adopted lead as a model material at room temperature to study the material deformation of forging in the whole loading forming process.Gao et al.6and Zhang et al.34also employed lead as a model material to investigate the material flow and forming defect in local loading forming.These studies conf i rmed that the deformation behaviors of lead at room temperature have similar characteristics to those of the original material in a hot forming process.Thus,in order to simplify the experiment process and save the cost of the material,lead was also used to validate the FE model and investigate the problems of folding and die filling in this research.The material of dies is 5CrNiMo die steel.Consistent grease was used for lubricating between dies and the workpiece.After two local loading steps,the flash on the formed workpiece was removed.Then,a folding defect can be clearly observed in web 3 of the eigenstructure.Underfilling can also be observed on ribs 1 and 4 of the workpiece,as shown in Fig.4(b).The corresponding combined dies and ETB used for the experiment are shown in Fig.4(c).
The heights of ribs 1,2,and 3 on the symmetrical plane(Hleft,Hmiddle,Hright)and the distance between the folding and the center of rib 2(Dfold)were measured so as to quantitatively compare the simulated results with the experimental results.All measured values are listed in Table 3.It can be found that the average relative error is about 5.65%,while the maximum relative error is about 8.85%.The main reasons of induced errors between experimental and simulation results are as follows:
(1).The material could flow into the assembling clearance of the combined dies during the experiment,while the FE simulation was conducted in an ideal condition,which ignored the assembling clearance.Therefore,loss of the material in the experiment leads to higher simulated values ofHleft,Hmiddle,andHrightoverall when compared to experimental ones.
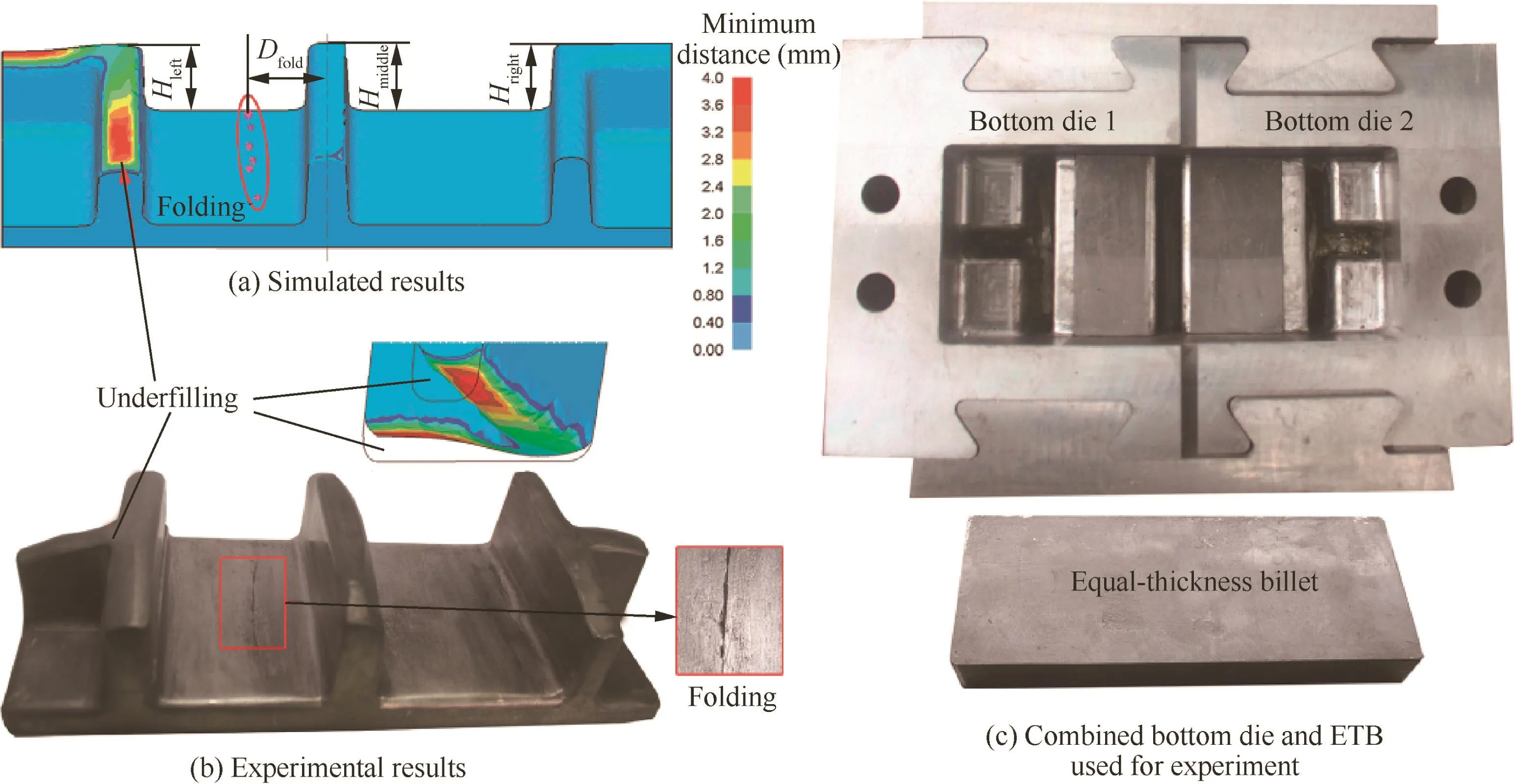
Fig.4 Forming results of an ETB after two loading steps.
(2).A smaller simulated value ofDfoldindicates that the transferred material in the experiment was more than that in the simulation.Thus,the flowing property of the actual test material at room temperature is relatively superior to that of Ti-alloy in hot forming.
(3).The frictional mechanism between dies and the workpiece is complicated,and the frictional factor is variable during the forming process of two loading steps.However,the frictional factor is considered as invariable in the FE simulation.
(4).In the experiment,the two sides of the workpiece were in contact with the die cavity wall,and thus friction could be produced there.While the two sides of the eigenstructure were restrained in the simulation,the friction between the two sides of the workpiece and dies was ignored.
The above comparisons and error source analysis demonstrate that the established 3D-FE model prediction agrees well with the experimental results,so the 3D-FE model in this study is reliable and usable.
2.3.2.Analysis of the folding
It can be found that there is non-folding observed after the first-loading step,so folding only occurs during the second one.The evolution of this folding can be divided into three stages,which are the initial stage,step formation stage,and folding occurrence stage,respectively,as shown in Fig.5(a)–(c).
The initial stage is illustrated in Fig.5(a),in which a clearance between the top die and the bottom die 1 can be seen,and the material distributed in the second-loading region fills the cavities of ribs 2,3,and 5 and flows into that clearance when the flowing material gets in contact with the bottom die 1,as depicted by the velocity vector diagram.Subsequently,more and more material,which is still distributed in the secondloading region,flows into the clearance as the top die descends,resulting in a step to be formed adjacent to the left side of rib 2.This is the step formation stage,as shown in Fig.5(b).With further increasing of the top die stroke,the material on the two surfaces of that step would be close to each other little by little,and thus folding occurs.This is the third stage,as shown in Fig.5(c).With ongoing of the top die,the clearance disappears and a neutral layer is generated,as the velocity vector diagram shows in Fig.5(c).Since then,the material on the left side of rib 2 will flow from both sides of the neutral layer,and no more material will be transferred into the first-loading region.It is indicated that once the neutral layer is generated around the folding zone,the material transferred into the first-loading region could be desisted.The above analysis demonstrates that the initial volume distribution in the second-loading region can greatly affect the amount of material transferred into the first-loading region during the second-loading step,and thus lead to a folding defect.
2.3.3.Analysis of the die filling
In the first-loading step,the vast majority of the material distributed in the first-loading region flows into the die cavities of ribs 1 and 4.Meanwhile,the rest of the material flows into the second-loading region.The workpiece after the first-loading step is illustrated in Fig.6(a).It can be found that ribs 1 and4 are underfilled.However,a small amount of material above the bottom die 2 could flow into the first-loading region,and then fill the die cavities of ribs 1 and 4.This part of material is conducive to compensate for the die filling of ribs 1 and 4.It is regarded that the material could flow into all the die cavities of the ribs in the transitional region during the secondloading step.Therefore,the analysis of die filling is only focused on the second-loading step.

Table 3 Comparison of forming results between FE simulation and experiment.
During the second-loading step,it is found that ribs 3 and 5 are fully filled before any other ribs,so ribs 3 and 5(including adjacent webs)are defined as an easy filling region here.It is noteworthy that if ribs 1 and 4(or 2)are fully filled earlier than the other ribs in the next research stage by the UTB,they are also called an easy filling region.Fig.6(b)illustrates the material flow state before the easy filling region is fully filled.There exists a neutral layer on the left side of rib 3.The free-flowing state of material would be remained when the material flow direction is toward two opposite directions along the neutral layer.Nevertheless,after the easy filling region is fully filled,if the total stroke of the top die is not finished at this moment,the material in the second-loading region would be compelled to reverse the flow direction,resulting in a material backflow to be produced.Subsequently,the material flow direction could only be toward the die cavity of rib 2,as shown in Fig.6(c).Even worse,if the die cavity of rib 2 is fully filled before the total stroke of the top die is finished,the material would cross rib 2,and then flow into the die cavities of ribs 1 and 4.In this process,it is inevitable that the die cavities of the fully-filled ribs bear the hydrostatic pressure of the filling material.The forming load could be increased sharply,which not only is detrimental to the forging equipment,but also leads to damage of the dies.Moreover,Zhang et al.34pointed out that if a rib cavity has no room to contain the filling material,it will make the filling material flow outward along the web and lead to redundant material reverse flowing in only one direction.Then,rib breaking and flow lines disturbance of the neighboring rib will be caused.From the analysis above,it may come to a conclusion that the die underfilling of ribs 1 and 4 after the second-loading step is directly caused by an improper initial volume distribution,which results in nonconcurrent die filling in the cavities of the ribs in the transitional region.
3.Optimization procedure
3.1.Optimization objective

Fig.5 Folding evolution during the second-loading step.

Fig.6 Shape and material flow state of the eigenstructure during the ILLF process.
With the above analysis of the die filling behavior,it can be concluded that the material flow state in the die cavity plays a significant role in the die filling process of the transitional region.Under the same stroke of the top die,the longer the time of the free-flowing material,the closer to the concurrent die filling in the cavities of ribs.Similarly,Park and Hwang14revealed that ribs should be filled simultaneously to decrease the forging load and wear in dies.It is suggested that concurrent or near-concurrent die filling in every cavity of a rib is significant for the forming quality of a component.Thus,in order to ensure a high-precision LTRC in the transitional region,the die underfilling rate φuis considered as the optimization objective for quantification of the die filling,which can be expressed by the following equation:

whereVactualis the actual volume of the eigenstructure when the easy filling region is fully filled before any other ribs during the second-loading step.It is noteworthy that if the stroke of the top die is unfinished at this moment,the corresponding material volume of the remaining reduction amount needs to be cut,so as to provide a better comparability for the die filling with different volume distributions of the billet.Here,the result of φuis 1.34%by an ETB.It can be concluded that with diminishing φu,the time of the free-flowing material is increased.Then,the concurrent die filling in every cavity of the ribs would be closer and closer.
3.2.Design variables
As mentioned above,the eigenstructure is very complex due to the different depth/width ratios of ribs,different distances between each rib,and different thicknesses of webs.Besides,different materials distributed in the loading region can result in different amounts of material transferred into the unloading region during the forming process.Therefore,with coupling effects of both reasons,the material undergoes complicated uneven plastic deformation and non-uniform flow,and thus makes the die filling process hard to control.To achieve a preferable die filling capability as well as avoid the folding defect in the transitional region of ILLF,a billet with an accurate volume distribution is necessary to ensure proper material flow.The geometry of a UTB can adjust the volume distribution with low cost and high efficiency,which can meet the needs of short cycle and low cost for manufacturing an LTRC.Therefore,a UTB is adopted in this research.To further simplify the geometry of the UTB,the shape of the UTB is divided into three sections.The thickness of each section is distinguished from those of others,and as a consequence,the following four types of UTB geometry can be acquired:(1)H2<H1&H2<H3(type 1);(2)H2>H1&H2>H3(type 2);(3)H3<H2<H1(type 3);and(4)H1<H2<H3(type 4),which can be seen in Fig.7,respectively.
Regarding the thickness variation between adjoining sections in the four UTB types,Zhang et al.35suggested that a beveling pattern may have universality in reducing the possibility of folding in a thickness variation region.Besides,it can meet the requirements of easy manufacture and flexible adjustment.Therefore,the beveling pattern for variable-thickness regions is adopted in this research.It can be defined as:

where Δl12and Δl23are the lengths of the beveling,and ΔH12and ΔH23are the thickness differences of adjoining sections.It can be seen from type 1 that the total area of the UTB(Aall)is constituted by f i ve subareas,which can be calculated as follows:

whereA1,A2,A3,A4,andA5are the areas of Area 1,Area 2,Area 3,Area 4,and Area 5,respectively.The volumes of two triangle zones(Area 2 and Area 4)which are surrounded by the beveling are relatively small when compared to the total volume of the eigenstructure,and thus a fixed value can be set forBleftandBrightto reduce the non-significant design variables so as to simplify the UTB optimization process.According to a suggestion by Zhang et al35,a value range of 2 to 5 for the beveling zone can reduce the possibility of a folding defect in the thickness variation region of a UTB,so the value ofBleftandBrightare limited to 2–5 accordingly.Due to the constantvolume principle and Eq.(3),increasing the value ofBleftandBrightcan also increase the volume ratio of the beveling zone in the UTB.To obtain the minimum volume ratio in the entire volume of the UTB,BleftandBrightare defined as the lowest value within the limited range of the beveling zone.Therefore,a value of 2 is advisable for bothBleftandBright.

Fig.7 Eigenstructure and four UTB types.
To study the different volume distributions of the UTB,f i ve key dimensional parameters are selected,which are the thicknesses of Area 1,Area 3,and Area 5(H1,H2,andH3,),and the distances between the center line of rib 2 and the adjoining lines of the thickness variation region(lleftandlright).Under the condition of the constant-volume principle,the volume of the UTB must always be kept constant,which means that the area ofAallshould be kept the same.Therefore,in the following analysis,H1,H3,lleft,andlrightare chosen as variable parameters to change the volume distribution of the UTB.H2is set as a dependent variable to ensure a constant total volume.The minimum and maximum values are determined by the characteristics of the eigenstructure as well as the size dimension of the closed boundary on the geometry of the UTB.Then,the relative variable values,i.e.,H1/He,H3/He,lleft/Lleft,andlright/Lright,are presented as factorsa,b,c,anddto acquire different initial volume distributions of the billet,as shown in Table 4.Among them,Lleftis the distance between the center lines of ribs 2 and 1,andLrightis the distance between those of ribs 2 and 3,as illustrated in Fig.7.
3.3.Constraint condition
To obtain a high-precision LTRC in the transitional region of ILLF,the first priority is to avoid the folding defect,because it is one of the most important factors influencing the service performance of a component.As mentioned above,the transferred material is the conclusive reason for the folding defect in the transitional region;therefore,by gradually decreasing the reduction amount of the top die while keeping the structural parameters of the die and spacer block constant,the quantity of the transferred material can be diminished,and then the maximum reduction amount of the top die for avoiding folding would be achieved.It can be seen that folding is located in web 3 of the eigenstructure,whose thickness is 14 mm,while the thickness of the ETB is 23.5 mm.As a consequence,the corresponding reduction amount in web 3(Ie)is 9.5 mm by the ETB.The different reduction amount in web 3 by the ETB can be defined as follows:

Table 4 Minimum and maximum values of the key dimension parameters of UTB.

Here,Heremains unchanged,whileTweb3gradually increases with the total reduction amount of the top die decreasing.In the following study,the initial value ofIeis 9.5 mm and the interval of the reduction amount is decreased by 0.1 mm.Then whether folding occurs or not can be observed through simulated results.
After stepwise decreasing the reduction amount in the ETB,the simulated results show that folding in web 3 occurs whenIeis between 8.9 mm and 9.5 mm,while folding can be avoided whenIeis down to 8.8 mm.The percentage of transferred materialMtis adopted so as to quantitatively describe the behavior of the transferred material,which can be expressed as:

whereVfis the volume of the workpiece in the first-loading region after the first-loading step,andVnis the volume of the workpiece in the first-loading region when a neutral layer is formed in web 3 of the eigenstructure during the second-loading step.Stepwise decreasing the reduction amount in the ETB suggests that the critical value ofIefor avoiding the folding defect must be between 8.8 mm and 8.9 mm.The correspondingMtfor avoiding the folding defect should be between 4.24%and 4.30%.Considering the safety margin in engineering applications,the lower value 4.24%ofMtis taken as a constraint condition for UTB optimization.
3.4.RSM models of Mtand φu
In general,the design of experiment(DOE)for the RSM is conducted by central composite design(CCD)and Box-Behnken design(BBD).A comparison between CCD and BBD has demonstrated that the experimental schemes of BBD are less than those of CCD,so BBD is more efficient than CCD.Moreover,BBD does not contain combinations for which all factors are simultaneously at their lowest or highest levels.These designs are beneficial for avoiding experimental schemes performed under extreme conditions,for which undesirable results might occur.36Therefore,in this study,BBD is chosen for finding out the relationship between the response functions(Mtand φu)and variables.According to the BBD method,29 schemes are designed by considering four factors with three levels,as shown in Table 5.After two loading steps,the calculated results ofMtand φuare also given in Table 5.
A second-order polynomial function is usually used as the surrogate model for the RSM,as expressed by the following equation:

whereyis the response,kis the number of input variables,xiandxjare the set of model input variables,and β0,βj,βjj,and βijrepresent the regression coefficients.Using the data in Table 5,the RSM models ofMtand φuare expressed by Eqs.(8)and(9),respectively,which have been f i tted using the stepwise regression method for the improvement of predictive accuracy by Design-Expert software.

The analysis of variance(ANOVA)is carried out so as to evaluate the RSM models ofMtand φu.The results of ANOVA analysis forMtand φumodels are shown in Tables 6 and 7,respectively.As it can be seen,the two RSM models are significant as theP-values are less than 0.01.TheF-values are 263.115 and 58.474,implying that the models are significant.In the tables,degree of freedom(df)is the number of values in calculation of statistic that are independent to vary.The values ofPon all models are smaller than 0.05,indicating that the model terms are significant.The adequacy measures ofR2and adjustedR2are in reasonable agreement and are both close to 1,indicating that these two models are adequate and meaningful.
Fig.8(a)and(b)shows the predicted(RSM)values versus the simulated(FEM)values.In these two figures,all points are close to a straight line.It can be seen that the predicted values have a better linear correlation with the simulated values.The above analysis suggests that the two developed RSM models are reliable to predictMtand φuin the transitional region of ILLF with significant accuracy.
3.5.Flowchart of optimization
Based on the above optimization procedure,the general trend of UTB optimization for obtaining a high-precision transitional region of ILLF can be broadly divided into two stages,as shown in Fig.9.
The first stage is named criterion of folding,which is indispensable for avoiding the folding defect and taken as a constraint condition for the subsequent die filling improvement.It is noteworthy that folding may not occur due to the distinguishing geometric shape of the transitional region.Under the circumstances,in order to achieve the critical value ofMtand take it as a constraint condition for die filling improvement,the interval of the reduction amount should be increased by 0.1 mm.Once folding occurs,Ie-0.1 can be determined as the maximum reduction amount in the ETB(Inon-folding)for avoiding folding of web 3,and the correspondingMtis the critical values of the percentage of transferred material forUTB optimization.The second stage is named die filling improvement.Parametric design of UTB geometry modeling has been applied to facilitate the changes of dimensional parameters.Then,BBD schemes for FEM can be established,and the results ofMtand φucan be calculated.Subsequently,the non-folding parameter combinations of the UTB can be determined by the RSM model ofMt,and an optimal UTB can be achieved by the RSM model of φu.Finally,FEM and experimental validations are both essential for evaluating whether folding occurs or not by the optimal UTB.

Table 5 BBD schemes and results of Mtand φu.

Table 6 ANOVA analysis of the RSM model of Mt.
4.Results and discussion
4.1.Feasibility of criterion of folding
The results ofMtby 29 BBD schemes and ETB are shown in Fig.10.Obviously,the results ofMtby f i ve schemes are below 4.24%,which are schemes 1,4,7,18,and 27,respectively.Their simulated results show that there is non-folding occurrence,as depicted by the forming result of scheme 27.Conversely,folding occurs by the rest of schemes,theMtvalues of which are all above 4.24%,as depicted by the forming result of scheme 29.
The above analysis further indicates that stepwise decreasing the reduction amount in the ETB provides a feasible way to determine the critical value ofMtfor predicting the folding occurrence in the transitional region.On this basis,the relation betweenMtand folding can be well acquired by adopting the geometry of the UTB.
4.2.Optimization results
According to the ANOVA analysis for the RSM model of φuin Table 7,factordalmost has no effect on φu,so it is fixed at the middle value of 0.8.In general,a safety margin is usually considered in engineering applications.Thus,the critical value ofMtshould be lower than 4.24%,which is defined asCtand expressed as:

where α(0.95 ≤ α < 1)is the safety factor.
Then the total optimization problem can be modeled as follows:

The nonlinear program method is applied to solve the above optimization problem.The purpose of optimization is to obtain a minimized die underfilling rate after the easy filling region is fully filled.Due to this,the optimal point and the shape of the optimal UTB with a consideration of the constraint condition are obtained,as shown in Fig.11,in which the contour plot of the response surface with factors a and b by the RSM model ofMtis shown,factor c is fixed at the optimal value of 1,and factor d is fixed at the middle value of 0.8.It can be seen that the non-folding region is located on the right side of the contour line ‘4.24”,and the value of the optimal UTB is inside this region.However,the value of the ETB is outside the non-folding region.To demonstrate the reliability of the optimum values,FE simulation and experiment with the optimal UTB are conducted,shown as the shape of the optimal UTB in Fig.11.

Fig.9 Flowchart of the UTB optimization procedure in the transitional region of ILLF.

Fig.10 29 schemes of BBD and ETB vs.Mt.
Fig.12(a)and(b)illustrate that the results ofMtand φuby the RSM and the FEM are close to each other.On one hand,the RSM and FEM results ofMtare both below 4.24%by the optimal UTB;meanwhile,non-folding is observed by the corresponding FEM and experimental results,as shown in Fig.12(c)and(d).On the other hand,it is revealed in Fig.12(b)that the die filling rate obtained in the optimal UTB case is increased by 32.8%,compared to the ETB outcomes.Furthermore,the experimental results also show that the heights of formed ribs 1 and 4 by the optimal UTB are higher than those of the ETB.It is demonstrated that the die filling capability is improved by the optimal UTB,allowing a high-precision LTRC in the transitional region of ILLF to be produced,as shown in Fig.12(e).The overall analyses suggest that the optimal design method of this work is efficient,and the optimal UTB is valid and reliable.

Fig.11 Contour graphs demonstrating the effects of factors a and b on Mt.
5.Conclusions
In this paper,the folding defect and die filling in the transitional region of ILLF for an LTRC were investigated by FE simulation and experiment.An optimal UTB was designed in conjunction with the RSM.The UTB optimization results showed that not only the folding defect was avoided,but also the optimum die filling was obtained.From this work,the following conclusions were drawn:
(1)Stepwise decreasing the reduction amount in the ETB in the transitional region provided a feasible way to determine the critical value of the percentage of transferred material for predicting the folding occurrence,which was taken as a constraint condition for avoiding the folding defect in the UTB optimization process.
(2)The percentage of transferred material was established with the parameter combinations of the UTB by an RSM model.Based on the critical value of the percentage of transferred material,non-folding parameter combinations of the UTB were achieved to guarantee non-folding defect occurrence in the transitional region of ILLF.
(3)The RSM model of the die underfilling rate was established for the purpose of UTB optimization based on the constant-volume principle.The optimization results showed that folding was avoided and the die filling rate wasincreased by32.8%,compared to the ETB outcomes.
Acknowledgements
The authors would like to gratefully acknowledge the supports of the National Natural Science Foundation of China(No.51575449),Research Fund of the State Key Laboratory of Solidification Processing(NWPU)of China(No.104-QP-2014),the 111 Project(No.B08040),the Fundamental Research Funds for the Central Universities(3102015AX004).
1.Yang H,Fan XG,Sun ZC,Guo LG,Zhan M.Recent developments in plastic forming technology of titanium alloys.Sci China Technol Sci2011;54:490–501.
2.Fan XG,Yang H,Gao PF.Through-process macro-micro finite element modeling of local loading forming of large-scale complex titanium alloy component for microstructure prediction.J Mater Process Technol2014;214(2):253–66.
3.Zhang DW,Yang H.Fast analysis on metal flow in isothermal local loading process for multi-rib component using slab method.Int J Adv Manuf Technol2015;79(9):1805–20.
4.Sun ZC,Zhang J,Yang H,Wu HL.Effect of workpiece size on microstructure evolution of different regions for TA15 Ti-alloy isothermal near-β forging by local loading.J Mater Process Technol2015;222:234–43.
5.Gao PF,Yang H,Fan XG,Lei PH.Quick prediction of the folding defect in transitional region during isothermal local loading forming of titanium alloy large-scale rib-web component based on folding index.JMaterProcessTechnol2015;219:101–11.
6.Gao PF,Yang H,Fan XG.Quantitative analysis of the material flow in transitional region during isothermal local loading forming of Ti-alloy rib-web component.Int J Adv Manuf Technol2014;75(9–12):1339–47.
7.Gao PF,Yang H,Fan XG,Lei PH.Forming defects control in transitional region during isothermal local loading of Ti-alloy rib-web component.Int J Adv Manuf Technol2014;76(5–8):857–68.
8.Zhang DW,Yang H.Distribution of metal flowing into unloaded area in the local loading process of titanium alloy rib-web component.Rare Metal Mat Eng2014;43(2):296–300.
9.Fan XG.Study on microstructure evolution during isothermal local loading forming of large-scale integral complex component of titanium alloys[dissertation].Xi’an:Northwestern Polytechnical University;2012.
10.Sun ZC,Yang H,Sun NG.Effects of parameters on inhomogeneous deformation and damage in isothermal local loading forming of Ti-Alloy component.J Mater Eng Perform2012;21(3):313–23.
11.Gao PF,Li XD,Yang H,Fan XG,Lei ZN.Improving the process forming limit considering forming defects in the transitional region in local loading forming of Ti-alloy rib-web components.Chin J Aeronaut2016;30(3):1270–80.
12.Kim SY,Tsuruoka K,Yamamoto T.Effect of forming speed in precision forging process evaluated using CAE technology and high performance servo-press machine.ProcediaEng2014;81:2415–20.
13.Wang JL,Fu MW,Ran JQ.Analysis and avoidance of flowinduced defects in meso-forming process:simulation and experiment.Int J Adv Manuf Technol2013;68(5):1551–64.
14.Park JJ,Hwang HS.Preform design for precision forging of an asymmetric rib-web type component.J Mater Process Technol2007;187–188(3):595–9.
15.Torabi SHR,Alibabaei S,Bonab BB,Sadeghi MH,Faraji G.Design and optimization of turbine blade preform forging using RSM and NSGA II.J Intell Manuf2017;28(6):1409–19.
16.Yang YH,Liu D,He ZY,Luo ZJ.Optimization of preform shapes by RSM and FEM to improve deformation homogeneity in aerospace forgings.Chin J Aeronaut2010;23(2):260–7.
17.Zhao GQ,Wang GC,Grandhi RV.Die cavity design of near flashless forging process using FEM-based backward simulation.J Mater Process Technol2002;121(2):173–81.
18.Lu B,Ou HA,Cui ZS.Shape optimisation of preform design for precision close-die forging.Struct Multidiscip Optim2011;44(6):785–96.
19.Guan YJ,Bai X,Liu MJ,Song LB,Zhao GQ.Preform design in forging process of complex parts by using quasi-equipotential field and response surface methods.Int J Adv Manuf Technol2015;79(1):21–9.
20.Knust J,Podszus F,Stonis M,Behrens BA,Overmeyer L,Ullmann G.Preform optimization for hot forging processes using genetic algorithms.Int J Adv Manuf Technol2017;89(5):1623–34.
21.Wei K,Yang H,Fan XG,Gao PF.Unequal thickness billet design for large-scale titanium alloy rib-web components under isothermal closed-die forging.Int J Adv Manuf Technol2015;81(5):729–44.
22.Guo LG,Dang L,Yang H,Zhang J,Zheng WD.identification of processing window for extrusion of large thick-walled inconel 625 alloy pipes using response surface methodology.Trans Nonferrous Met Soc China2016;26(7):1902–11.
23.Zhu XL,Liu D,Yang YH,Hu Y,Zheng Y.Optimization on cooperative feed strategy for radial–axial ring rolling process of Inco718 alloy by RSM and FEM.Chin J Aeronaut2016;29(3):831–42.
24.Zhang WW,Han C,Yuan SJ.Optimizationof pre-form shapes by response surface methodology for hydro-forming of 780 MPa torsion beam.Int J Adv Manuf Technol2016;85(5):1227–37.
25.Jin R,Chen W,Simpson TW.Comparative studies of metamodelling techniques under multiple modelling criteria.Struct Multidiscip Optim2015;23(1):1–13.
26.Chen V,Tsui KL,Barton RR,Meckesheimer M.A review on design,modeling and applications of computer experiments.IIE Trans2006;38(4):273–91.
27.Li YF,Ng SH,Xie M,Goh TN.A systematic comparison of metamodeling techniques for simulation optimization in decision support systems.Appl Soft Comput2010;10(4):1257–73.
28.Zhang ZY.Multi-target preform optimization for spherical hinge mandrel based on Kriging model methodology[dissertation].Zhenjiang:Jiangsu University;2016[Chinese].
29.Liu N,Yang H,Li H,Tang WT,Yan SL,Zhang HL.BP artificial neural network modeling for accurate radius prediction and application in incremental in-plane bending.Int J Adv Manuf Technol2015;80(5):971–84.
30.Kim DJ,Kim BM,Choi JC.Determination of the initial billet geometry for a forged product using neural networks.J Mater Process Technol1997;72(1):86–93.
31.Sun ZC,Yang H.Analysis on process and forming defects of large-scale complex integral component isothermal local loading.Mater Sci Forum2009;614:117–22.
32.Shen CW.Research on material constitution models of TA15 and TC11 titanium alloys in hot deformation process[dissertation].Xi’an:Northwestern Polytechnical University;2007[Chinese].
33.ZhangDW,YangH,LiHW,FanXG.Frictionfactor evaluation by FEM and experiment for TA15 titanium alloy in isothermal forming process.Int J Adv Manuf Technol2012;60(5–8):527–36.
34.Zhang DW,Yang H,Sun ZC.Analysis of local loading forming for titanium-alloy T-shaped components using slab method.J Mater Process Technol2010;210(2):258–66.
35.Zhang DW,Yang H,Sun ZC,Fan XG.Deformation behavior of variable-thickness region of billet in rib-web component isothermal local loading process.Int J Adv Manuf Technol2012;63(1):1–12.
36.Ferreira SLC,Bruns RE,Ferreira HS,Matos GD,David JM,Brandao GC,et al.Box-Behnken design:an alternative for the optimization of analytical methods.Anal Chim Acta2007;597(2):179–86.
杂志排行
CHINESE JOURNAL OF AERONAUTICS的其它文章
- Extension of analytical indicial aerodynamics to generic trapezoidal wings in subsonic flow
- Time-varying linear control for tiltrotor aircraft
- Global aerodynamic design optimization based on data dimensionality reduction
- Mesh deformation on 3D complex configurations using multistep radial basis functions interpolation
- Stagnation temperature effect on the conical shock with application for air
- Transient simulation of a differential piston warm gas self-pressurization system for liquid attitude and divert propulsion system
