Effects of tube system and data correction for fluctuating pressure test in wind tunnel
2018-04-21StteKeyLbortoryofAerodynmicsChinAerodynmicsReserchndDevelopmentCenterMinyng621000Chin
Stte Key Lbortory of Aerodynmics,Chin Aerodynmics Reserch nd Development Center,Minyng 621000,Chin
bLow Speed Aerodynamics Institute,China Aerodynamics Research and Development Center,Mianyang 621000,China
1.Introduction
The fluctuating pressure test in wind tunnel is the main approach to gain the unsteady aerodynamic loads.In low speed wind tunnel tests,the dynamic pressure sensor sometimes cannot be placed on the model surface directly because of the size,especially for the thin model such as the control plane of the aircraft.In this situation,the sensor is commonly placed inside the model,and a tube system is used to connect the pressure measurement point and the sensor.1In this way of measurement,fluctuating pressure has been distorted while transferred to the sensor by the tube system,2which leads to a great reduction of data accuracy.To solve this problem,the first step is to analyze the relationship between fluctuating pressure and parameters of the tube system,such as length,inside diameter,curvature,deflection angle,thickness,and material.
The effects of the tube system on fluctuating pressure can be described by the Frequency Response Function(FRF)of the tube system,3which is the ratio of the pressure at the inlet and outlet in frequency domain.Bergh and Tijdeman4derived the theoretical formulas of FRF.It can also be obtained by the experiment.The FRFs of different tube systems were analyzed5–16by the theoretical or experimental method,and the tube system design method was established based on the optimistic algorithm and restrictor.17–22According to the design method,the tube system is divided into several sections,and the length and inside diameter of every section are calculated by optimization algorithm.The short section with small inside diameter is always called restrictor.The designed tube system could remarkably improve the accuracy of magnitude of fluctuation pressure.
In the engineering application,some shortcomings are found for the tube system optimization design method.First,in the FRF theoretical formulation and optimization design method mentioned above,only two parameters—tube length and inside diameter—can be considered.But in the practice of wind tunnel test,other tube parameters may dramatically affect fluctuating pressure,such as tube curvature,deflection angle,thickness,and material.Second,the optimization design method for the tube system may enlarge the error of phase,though it could improve the accuracy of magnitude.The reason is that the magnitude of FRF is chosen as the optimization objective but the phase is not concerned by the optimization design method.It will have no problem if every single channel of fluctuating pressure is analyzed independently.If the relativity between several channels is concerned,the phase error leads to an absolutely wrong result.23,24For example,when the dynamic load on the control plane is calculated through surface integral of fluctuating pressure,the accuracy of the result is greatly dependent on the phase of fluctuating pressure.Third,the optimization design method for the tube system could only be effective in a limit frequency range,and the range is proportional to the complexity of the tube system.Fourth,the tube system designed by the optimization method is always so complex that it is difficult to manufacture and a non-ignorable error may be induced between the ideal results and actual measurements.19
In this paper,systematic study is conducted to investigate the effects of tube system parameters on fluctuating pressure by contradistinctive experimental method.The analyzed tube system parameters include tube length,inside diameter,curvature,deflection angle,thickness,material,restrictor length,restrictor inside diameter,and restrictor place.Then in order to eliminate the effects of the tube system and improve the data accuracy,test methods are presented for the fluctuating pressure measurement in low-speed wind tunnel.The results of the wind tunnel test are given to verify the effectiveness of the methods.
2.Methods for contradistinctive experiment
In order to analyze the difference between the pressure collected with and without the tube system,two Pressure Measurement Points(PMP)(denoted as PMP-1 and PMP-2)with the same pressure are set at first.PMP-1 is connected to a dynamic pressure sensor(denoted as Sensor-1)directly,while PMP-2 is connected to a dynamic pressure sensor(named Sensor-2)by a tube system.The difference between the signals collected by the two sensors may reflect the effect of the tube system on fluctuating pressure.
In the experiment,the two small holes on the organic glass plate are used to form PMP-1 and PMP-2,which is verified by having the same pressure.The results of the verifying experiment are presented later in this paper.A loud speaker is used to create fluctuating pressure.The schematic diagram of the contradistinctive experiment is shown in Fig.1.Fluctuating pressure signals are collected and analyzed by ENDEVCO 8510B-1 dynamic pressure sensor and LDS-Dactron FoucsⅡdynamic analyzer.The main parameters of the 8510B-1 sensor are as follows:measuring range of 0–1 psi(1 psi=6894.76 Pa),sensitivity of 200 mV/psi,and resonance frequency of 55 kHz.
The swept frequency excitation in range of 0–165 Hz is applied to generate fluctuating pressure.The signals of Sensor-1 and Sensor-2 are collected synchronously at sampling rate of 375 Hz for 4096 points.These two pressure signals collected by Sensor-1 and Sensor-2 are denoted asp1(t)andp2(t).Their Fourier transform are denoted asP1(f)andP2(f)correspondingly.In the symbols,trepresents time,andfrepresents frequency.
The FRF of the tube system is provided as follows:

Twenty time average is used to improve the smoothness of the FRF.The analyzing frequency is limited in range of 0–165 Hz.

Fig.1 Schematic diagram of measurement system.

Fig.2 Results of conformity checking experiment(frequency domain).
The magnitude and phase of FRF can reflect the effects of the tube system on fluctuating pressure.If|H2,1(f)|(magnitude of FRF)is greater than 1,it means that the magnitude of fluctuating pressure has been amplified.On the contrary,the magnitude of fluctuating pressure has been minified.In theory, φ2,1(f)(phase of FRF)is less than 0 rad,which means that the pressure signal is delayed by the tube system.Absolute phase is denoted as|φ2,1(f)|.The effects of the tube system are small if|H2,1(f)|and φ2,1(f)are close to 1 and 0 rad correspondingly.On the contrary,the effects are great.
PMP-1 and PMP-2 are desired to have the same pressure.The conformity is verified by the experiment,in which PMP-1 and PMP-2 are directly connected to Sensor-1 and Sensor-2 respectively without the tube system.The results of verifying experiment are given in Figs.2 and 3.Fig.2 shows|H2,1(f)|,φ2,1(f)and Coh2,1(f)(coherence function between the two pressure signals25).Fig.3 shows the comparison between signals collected by Sensor-1 and Sensor-2 in time domain.According to the results,|H2,1(f)|and Coh2,1(f)are very near to 1,and φ2,1(f)is very near to 0 rad.In conclusion,the conformity is perfect,and the two small holes on the organic glass plate can be used as PMP-1 and PMP-2 with the same pressure.
3.Effects of various parameters on FRF
3.1.Effects of tube length
By the contradistinctive experiment method,FRFs are obtained for tubes with different length(denoted asL).The PolyVinyl Chloride(PVC)tube with 1.2 mm inside diameter and 2.2 mm outside diameter(denoted as 1.2×2.2)is used in the experiment.This type of tube is often used in the lowspeed wind tunnel for fluctuating pressure test.This type of tube is used as the default type later in this paper if not specially specif i ed.

Fig.3 Results of conformity checking experiment(time domain).

Fig.4 Magnitudes of FRFs for tubes with different length.
For tubes with different length,the magnitude and phase of FRF are shown in Figs.4 and 5.According to the results,the length of tube has great effects on the magnitude and phase of FRF.As length increases,there are decreases in terms of the peak value and peak frequency of|H2,1(f)|,while increases can be seen in|φ2,1(f)|.For a certain length of tube,there is a frequencyf0.Asf>f0,the signal magnitude is amplified,while the signal magnitude is minified asf<f0.For example,under the condition ofL=500 mm andf=100 Hz,the magnitude is amplified to about 2.15,and the phase is about-60°.Under the condition ofL=1400 mm andf=100 Hz,the magnitude is minified to about 0.68,and the phase is about-190°.The modal frequencies(peak frequencies)of gas column can be qualitatively analyzed by using the one dimensional wave equation.26,27It reveals that the mode frequency is inversely proportional to the length,which is consistent with the results above.
3.2.Effects of tube inside diameter

Fig.5 Phase of FRFs for tubes with different length.
Under the condition ofL=500 mm,FRFs of tubes with different inside diameters are analyzed.FRFs are gained for the inside diameters(d)of 0.8,1.0,1.2,and 1.5 mm,and their|H2,1(f)|and φ2,1(f)are shown in Figs.6 and 7.From the results,it can be found that effects of inside diameter of tube on FRF are remarkable.Increases in insider diameter raise the peak value and peak frequency of|H2,1(f)|obviously,but lead to decreases in|φ2,1(f)|.These may be caused by the tube damping for the flowing gas.The tube with larger inside diameter has smaller damping,and thus has a greater peak value and peak frequency.
3.3.Effects of tube curvature
Under the condition ofL=500 mm,FRFs of tubes with different curvature are analyzed.The curvature radius(r)includes 3,5,8,10,15,20,30,40,50,60,80 and∞mm,and their|H2,1(f)|and φ2,1(f)are displayed in Figs.8 and 9.These figures reflect that tube curvature has few effects on the magnitude and phase of FRF.

Fig.7 Phase of FRFs for tubes with different inside diameters.
3.4.Effects of tube deflection angle
Under the condition ofL=500 mm and 8 mm curvature radius,FRFs of tubes with different deflection angles(δ)are analyzed.The definition of deflection angle is shown in Fig.10.The deflection angles of 0°,90°,180°,360°,720°and 1080°are analyzed,and their|H2,1(f)|and φ2,1(f)are given in Figs.11 and 12.The results show that the deflection angle of tube has a few effects on|H2,1(f)|and almost no effect on φ2,1(f).The peak value of|H2,1(f)|decreases when tube deflection angle increases.While deflection angle is less than 360°,the error of|H2,1(f)|is less than 1.5%,which can be ignored.So the results tell us that the tube system must be avoided to be twisted together in the wind tunnel test.
3.5.Effects of tube thickness and material
Under the condition ofL=500 mm and 1.5 mm inside diameter,FRFs of tubes with different tube thickness or material are analyzed.The types of analyzed tubes include 1.5×3.0 PVF tube,1.5×2.0 PVC tube,1.5×2.0 stainless steel tube,1.5×2.5 brass tube,1.5×2.0 heat shrink tube,1.5×3.0 silicone tube,1.5×2.5 silicone tube,and 1.5×2.0 silicone tube.Their|H2,1(f)|and φ2,1(f)are shown in Figs.13 and 14.It can be seen from the results that tube thickness and material have remarkable effects on FRF.In general,as the strength of tube increases,there are increases in terms of the peak value and peak frequency of|H2,1(f)|,while decreases can be seen in|φ2,1(f)|.These results indicate that the transmission of fluctuating pressure in the tube is a fluid-solid-interaction phenomenon,which is influenced by the material strength,surface smoothness,and so on.

Fig.8 Magnitude of FRFs for tubes with different curvature radiuses.

Fig.9 Phase of FRFs for tubes with different curvature radiuses.

Fig.10 Schematic diagram of deflection angle.

Fig.11 Magnitude of FRFs for tubes with different deflection angles.

Fig.12 Phase of FRFs for tubes with different deflection angles.

Fig.13 Magnitude of FRFs for tubes with different thickness or material.

Fig.14 Phase of FRFs for tubes with different thickness or material.

Fig.15 Magnitude of FRFs for tubes with different restrictors.
3.6.Effects of restrictor
Under the condition ofL=500 mm,FRFs of the tube systems with different types of restrictor are analyzed.The parameters of restrictors are given in Table 1.Restrictor is fixed 333 mm away from sensor.Their|H2,1(f)|and φ2,1(f)are illustrated in Figs.15 and 16.According to the results,FRF is remarkably affected by restrictor.Compared with the tube system without restrictor,|H2,1(f)|for the tube systems with restrictor has an obvious lower peak and is closer to 1 asf<f0.Butf0is reduced obviously,which results in|H2,1(f)|being apparentlyminified within high-frequency range.|φ2,1(f)|rises in a large frequency range.On the basis of the figures,as the length of restrictor increases,the peak value and peak frequency of|H2,1(f)|decrease notably,while there are increasesin|φ2,1(f)|.These results are consistent with the results given in Sections 3.1 and 3.2.

Table 1 Restrictor parameters.
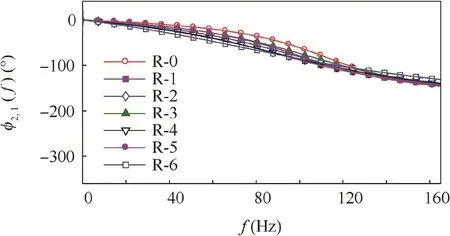
Fig.16 Phase of FRFs for tubes with different restrictors.

Fig.17 Magnitude of FRFs for tubes with different restrictor places.

Fig.18 Phase of FRFs for tubes with different restrictor places.
The effects of restrictor location on FRF are analyzed under the condition ofL=500 mm and restrictor R-4.The restrictor locations include 1/3L,2/5L,3/5Land 2/3L.Figs.17 and 18 demonstrate|H2,1(f)|and φ2,1(f).It can be seen that FRF depends strongly on the location of restrictor.The farther the restrictor is apart from the sensor,the smaller the peak value and peak frequency of|H2,1(f)|are.This can be qualitatively interpreted by one-dimensional wave equation and series tube system.28For a series tube system,if the total length is constant,the larger length for the free-free tube will result in a larger modal frequency for the series tube system.
In conclusion,restrictor could improve the accuracy of|H2,1(f)|in a limited low-frequency range,but the error of phase is enlarged,and so does the error of|H2,1(f)|in the high-frequency range.
4.Data correction scheme and examples
4.1.Data correction scheme
Because of the shortcomings mentioned in Section 1,the pressure distortion is corrected based on FRF by post-processing of data in the frequency domain in this paper.The data correction scheme is as follows:
(1)FRF of the tube system is calibrated by the contradistinctive experiments presented in Section 2;
(2)Pressure signal is collected by the calibrated tube system;
(3)The corrected pressure signals in frequency and time domain are calculated by

whereH2,1(f)is the calibrated FRF of the tube system,pc(t)the corrected fluctuating pressure,Pc(f)the Fourier transform ofpc(t)in frequency domain,FFT(·)the Fourier transform operation,and IFFT(·) the inverse Fourier trans form operation.
4.2.Examples
The data correction scheme is applied in the fluctuating pressure test for a radar dome of some airplane in 8 m×6 m wind tunnel.The test is run under the condition of dynamic pressureq=2800 Pa,β =0°,α =-9°,-6°,0°,6°,12°,16°,18°,20°,22°,24°,26°.α and β represent model angle of attack and side slip(see Fig.19).A tee joint is fixed at the pressure tap and one of the outlet is connected with 400 mm 1.2×2.2 PVC tube.The measurement scheme presented in Section 2 is used.p1(t)andp2(t)are collected synchronously at 2048 Hz for 10 s.

Fig.19 Schematic diagram of model angle of attack and side slip.

Fig.20 Comparison between signals in time domain with and without correction.

Fig.21 Comparison of magnitude with and without correction.

Fig.22 Comparison of phase with and without correction.
When α =24°and β =0°,the fluctuating pressure before and after correction are compared in Fig.20.The comparison of their magnitude and phase is shown in Figs.21 and 22.For the sequence of α,Fig.23 gives the Root Mean Square Error(RMSE)of fluctuating pressure.According to the results,it can be found that the fluctuating pressurep2(t)is distorted seriously.Due to the effects of the tube system,the magnitude is remarkably enlarged in the range of 100–180 Hz and the phase is delayed greatly as frequency increases.The corrected fluctuating pressurepc(t)is consistent withp1(t)which is collected without any tube system,both in time and frequency domain.After data correction,the RMSE is decreased by at least 90%.These results verified the effectiveness of the data correction scheme.

Fig.23 Comparison of RMSE with and without correction.
5.Improvements for wind tunnel test
In view of test accuracy and efficiency,some improvements should be adopted for fluctuating pressure test in wind tunnel when the tube system cannot be avoided.There are four aspects of improvements.
5.1 Standby tube systems
The precondition of the data correction methods mentioned in Section 4.1 is calibrating the FRF of the tube system.On the basis of the results given in Section 3,some tube parameters,such as length,diameter,thickness,and material,may obviously affect the FRF of tube.For every tube system with different parameters,the FRF needs to be calibrated by experiment.If the tube system is chosen casually,there will be a heavy calibration workload for engineering application in fluctuating pressure test in wind tunnel.It is an efficient method to prepare some standby tube systems.The FRFs of the standby tube systems are calibrated and saved in advance.Then these standby tube systems can be used in later wind tunnel test.Based on the saved FRFs,the distortions of fluctuating pressure,which are induced by tube systems,can be corrected by the methods presented in Section 4.1.
The mixed tube system of rigid metal tube and flexible PVC tube are always used in the wind tunnel test.The rigid metal tube is fixed at model pressure tap,and the flexible PVC tube connects the rigid metal tube and the dynamic pressure sensor.In order to eliminate the effect of tube diameter,thickness and material,the standby tube system is restricted to assemblage of 1.5×2.0 brass tube and 1.2×2.2 PVC tube.This type of tube system is convenient to assemble and has good air tightness.
Considering the requirements for different model sizes,25 types of standby tube systems are prepared.They are combinations of 100,200,300,400,500 mm 1.5×2.0 brass tube and 1.2×2.2 PVC tube.The 25 types of standby tube systems may fulfill the requirements of fluctuating pressure test in low-speed wind tunnel.
5.2 Database for FRFs of standby tube systems

Fig.24 Magnitude of FRFs for standby tube systems.

Fig.25 Phase of FRFs for standby tube systems.
The FRFs for the 25 types of standby tube systems are calibrated by the contradistinctive experimental method presented in Section 2.Their magnitude and phase of FRF are illustrated in Figs.24 and 25.They are f i t for polynomial,and the polynomial coefficients are saved in database for later applications.
5.3 Installation of tube systems
When tube systems are installed,there are some points that must be paid attention to.First,tube systems for every pressure tap should be chosen from the 25 types of standby tube systems.Second,the accuracy of tube length should be less than 5 mm.Third,according to the test results provided in Section 3.4,the effect of tube deflection angle may be nearly ignored if deflection angle is less than 360°,so tube systems must be prevented from twisting together.Fourth,the type numbers of the used tube systems should be recorded for every pressure tap.
5.4 Data post-processing
Following the type number of the tube system,the FRF is retrieved from the FRF database for the standby tube systems.The distortion of fluctuating pressure data is corrected using Eqs.(2)–(4).Then the fluctuating pressure data with high accuracy can be used to analyze the Root Mean Square(RMS),Power Spectral Density(PSD),and the model dynamic load.
6.Conclusions
(1)Systematic study is conducted to investigate the effects of tube system parameters on fluctuating pressure by contradistinctive experimental method.
(2)The analyzed tube system parameters include tube length,inside diameter,curvature,deflection angle,thickness,material,restrictor length,restrictor inside diameter,and restrictor place.It is found that all the parameters mentioned above except curvature have non-negligible effects on the FRF of the tube system for fluctuating pressure measurement.The larger length,smaller inside diameter,smaller strength,longer restrictor,and farther distance between restrictor and sensor will result in a smaller peak value and peak frequency of|H2,1(f)|,and a larger value of|φ2,1(f)|.
(3)Test methods were presented for the fluctuating pressure measurement in low-speed wind tunnel.They can obviously improve the data accuracy but not lose test efficiency.
(4)The wind tunnel test verified the effectiveness of the
proposed methods.
Acknowledgement
This study was supported by the Pre-research Fund of Vibration and Noise Control Technology(No.51334060101).
1.Li ZF.Special wind tunnel test techniques.Beijing:Aviation Industry Press;2010.p.418–9[Chinese].
2.Yoshida M,Kondo K,Suzuki M.Fluctuating wind pressure measured with tubing system.JWindEngIndAerod1992;42:987–98.
3.Irwin HPAH,Cooper KR,Girard R.Correction of distortion effects caused by tubing systems in measurements of fluctuating pressures.J Ind Aerodyn1979;5:93–107.
4.Bergh H,Tijdeman H.Theoretical and experimental results for the dynamic response of pressure measuring systems.Amsterdam:National Aero and Astronautic Research Institute;1965(Report No.:NLR-TR F.238).
5.Yang YJ,Chen QS,Cai F.Research on frequency response of tube pressure measurement system.J Exp Fluid Mech2006;20(2):85–9[Chinese].
6.Xu YH,Huang DQ,Mu S.A simple method for determining the dynamic characteristics of pressure transducer terminated at a chamber.Shanghai J Mech1981;4:47–55[Chinese].
7.Gumley SJ.Tubing systems for pneumatic averaging of fluctuating pressures.J Wind Eng Ind Aerod1983;12:189–228.
8.Gumley SJ.A detailed design method for pneumatic tubing systems.J Wind Eng Ind Aerod1983;13:441–52.
9.Holmes JD.Effect of frequency response on peak pressure measurements.J Wind Eng Ind Aerod1984;17:1–9.
10.Gerstoft P.A new tubing system for the measurement of fluctuating pressures.J Wind Eng Ind Aerod1987;25:335–54.
11.Su EH.Tubing system dynamic analysis and fluid numerical computation method.Harbin:Harbin Institute of Technology Press;1985.p.56–61[Chinese].
12.Cai YG.Dynamics of fluid transmission pipeline.Hangzhou:Zhejiang University Press;1989.p.15–43[Chinese].
13.Ueda H,Hibi K,Tamura Y,Fujii K.Multi-channel simultaneous fluctuating pressure measurement system and its applications.J Wind Eng Ind Aerod1994;51:93–104.
14.Roger H,George B,Richard F,Veilchko N.Digital filter adaptation for tubing response correction at reduced sampling frequencies.J Wind Eng Ind Aerod2010;98:833–42.
15.Yoshida A,Tamuraa Y,Kuritab T.Effects of bends in a tubing system for pressure measurement.J Wind Eng Ind Aerod2001;89:1701–16.
16.Yang J,Wang YF,Li C.Dynamic modeling of pressure pipelines and signal reconstruction.Proceedings of the 2011 International Conference on Instrumentation,Measurement,Computer,Communication and Control;2011 October 21–23;Beijing,China.Piscataway,NJ:IEEE Press;2011.p.78–81.
17.Holmes JD,Lewis RE.Optimization of dynamic-pressure-measurement systems.I.Single point measurements.J Wind Eng Ind Aerod1987;25:249–73.
18.Holmes JD,Lewis RE.Optimization of dynamic-pressure-measurement systems.II.Parallel tube-manifold systems.J Wind Eng Ind Aerod1987;25:275–90.
19.Zhou XY,Gu M.Optimization of dynamic pressure,easurement of s Single-channel tubing systems.JTongjiUniv2003;31(7):798–802[Chinese].
20.Xie ZN,Ni ZH,Shi BQ.Dynamic characteristics of tubing systems for fluctuating wind pressure measurements.Chin J Appl Mech2002;19(1):5–9[Chinese].
21.Xie ZN,Gu M.Optimal design for simultaneous wind pressure measurements.J Tongji Univ2002;30(2):157–63[Chinese].
22.Ni ZH,Yao WJ,Xie ZN.Integral of instantaneous pressure for studying wind-induced vibration of high-rise buildings.J Vib Eng2000;13(4):544–51[Chinese].
23.Guo MM,Xu YH,Huang DQ.Application of distorted signal correction in simultaneous pressure measurement.Chin Quart Mech2005;26(4):562–6[Chinese].
24.Guo MM,Huang DQ,Mu SU,Lin C,Xu YH.A method for simultaneous multi-fluctuating pressure measurement.J Exp Mech2005;20(1):123–7[Chinese].
25.Shaw JC.An introduction to the coherence function and its use in EEG signal analysis.J Med Eng Technol1981;5(6):279–88.
26.Zhou H,Liu YS,Yue ZF.Calculation analyze of pressure pulsation in fluid flowing pipeline.Mech Sci Technol Aerospace Eng2011;30(9):1435–8[Chinese].
27.Gudrun M.Modal analysis of hydraulic pipelines.J Sound Vib2013;332:3794–805.
28.Liu ZY,Gao ML,Ji YF.Calculation method of the gas pole immanence frequency in the design of the pipelines of a reciprocating compressor.Chem Eng Mach2008;35(4):212–5[Chinese].
杂志排行
CHINESE JOURNAL OF AERONAUTICS的其它文章
- Extension of analytical indicial aerodynamics to generic trapezoidal wings in subsonic flow
- Time-varying linear control for tiltrotor aircraft
- Global aerodynamic design optimization based on data dimensionality reduction
- Mesh deformation on 3D complex configurations using multistep radial basis functions interpolation
- Stagnation temperature effect on the conical shock with application for air
- Transient simulation of a differential piston warm gas self-pressurization system for liquid attitude and divert propulsion system
