Influences of preload on the friction and wear properties of high-speed instrument angular contact ball bearings

To ZHANG,Xioyng CHEN,*,Jiming GU,Zhengling WANG

aResearch Institute of Bearings,Shanghai University,Shanghai 200072,China

bShanghai Tian An Bearing Co.Ltd,Shanghai 201108,China

1.Introduction

In high-speed rotor systems for aviation,aerospace,and precision machine tools,pairs of preloaded angular contact ball bearings are commonly used.An appropriate axial preload,on one hand,can improve the rotation accuracy and support stiffness of the rotor,as well as reduce vibration and noise.1,2On the other hand,under high-speed and light-load conditions,by precisely controlling the preload,it is possible to effectively prevent orbit slipping and gyro sliding of balls,and reduce friction heating and wear.3,4Two main preload methods are often used in practice,constant-force preload and fixed-position preload,also called constant preload and rigid preload.The effects of preload on dynamic stiffness,5,6natural frequency,5,7vibration,2,8temperature rise,9,10and fatigue life4,11of spindle bearings with sufficient oil supply have been studied a lot.Some of the works have focused on preload optimization,3,10–12and revealed that the optimum preload can be determined by temperature rise,dynamic stiffness,and fatigue life for different speed ranges.Other researchers also investigated the impacts of different bearing preload methods on spindle dynamics.Li and Shin13presented the effects of bearing configuration on dynamic thermal and stiffness behaviors of high-speed spindles using a dynamic thermomechanical simulation model.Cao et al.14compared the effects of bearing preload methods on the dynamic performance of high-speed spindles by using a mathematical model as well as experiments,and pointed out that at high speeds and under cutting loads,the rigid preload method is more efficient in maintaining the dynamic stiffness of spindles than the constant preload one.

However,for starved-oil(lubricated by a plastic cage impregnated with a few milligrams of oil)or solid lubrication of high-speed instrument angular contact ball bearings,friction heating and wear are the main reasons of bearing failure.Liu et al.15established a wear life estimation model based on the quasi-static of Gyro-spin bearings for fixed-position preload.Gupta and Forster16built a numerical simulation model of wear for solid-lubricated ball bearings based on the overall dynamics of bearing elements.The time-averaged wear rates of balls,races,and the cage can be obtained by the computer model ADORE,which provides an analytical estimate of wear life for solid-lubricated ball bearings.However,researchers did not consider the impacts of different preload methods and the significant changes of preload caused by wear during the operation on the bearing wear life.This paper presents a coupled dynamic wear simulation model considering the differential sliding,spin sliding,and gyro sliding between balls and races for high-speed instrument rotor angular contact ball bearings.The changes of contact parameters and residual preload with the friction coefficient and wear volume under constant preload and fixed-position preload are analyzed.Thus,a foundation for more accurate prediction of bearing wear life can be laid.

2.Modeling and verification

Fig.1 Coordinate system.

According to the dynamic modeling method of rolling bearings in Refs.16,17,a dynamic wear simulation model of gyro rotor angular contact ball bearings was built up.It is assumed that the mass centers of bearing components coincide with their geometric centers,balls and the cage have six degrees of freedom,the mass center of the outer race is fixed,the mass center of the inner race have three or two degrees of freedom with constant or rigid preload respectively,and both the inner and outer races can rotate around the axis.The effect of lubrication is considered by setting a reasonable friction coefficient.For starved-oil or solid lubrication of angular contact ball bearings,there is no hydrodynamic pressure effect in the ball/cage and cage/guide lands contacts.For all contacts,normal and tangential forces are calculated by the Hertz contact theory and the Coulomb friction law.The friction coefficient in the contact area is constant.A variable step-size of the fourth-order Runge-Kutta method is used to solve the differential equations of bearing motion.

2.1.High-speed rotor bearing dynamic model

2.1.1.Coordinate system and kinematic equations

The centroid motion of bearing parts is described in the inertial coordinate system,and the rotation about the centroid is described in the body fixed or azimuth coordinate system.The coordinate system is defined as shown in Fig.1.The origin Oiof the inertial coordinate coincides with the center of locus of outer raceway groove curvature centers,Xiis along the bearing axial,and Zivertical up.The origin Oaof the azimuth coordinate frame is fixed to the ball center,Xais in the axial direction of the bearing,and Zais in the radial direction,where dmis the bearing pitch diameter and ψ is the ball azimuth angle.The cage coordinate frame origin Ocis fixed to the geometric center of the cage,Xcis along the cage axis,and Zcpoints to the first pocket hole center.

It can be achieved through three successive rotations from the inertial coordinate system to the body fixed coordinate system.17The transformation matrix is as follows:

Gupta deduced the relationship between the angular velocity of bearing parts and the rotation angle(η, ξ, λ),and obtained the kinematic equation17as

where ω1, ω2,and ω3are the angular velocity components of bearing parts.

2.1.2.Calculation of interaction forces and moments between bearing parts

For constant preload and fixed-position preload models,the positions of the ball center and raceway curvature centers at angular position ψjwith applied axial,radial,and moment loads are shown in Fig.2,where Ocois the groove curvature center of the outer raceway,andrepresent the positions of the ball center and the groove curvature center of the inner raceway before and after the loads being applied and during high-speed operation,respectively.

The geometric centers of the inner race and the ball in the inertial coordinate systemandcan be expressed as follows:

where BD=(fi+fo-1)Dw,fi/ois the inner/outer raceway groove curvature factor,and Dwis the ball diameter.α0is the initial contact angle. δaand δrare the axial and radial deformations of the inner race relative to the outer race.For fixed-position preload,δais a constant determined by static equilibrium.A1jand A2jare the axial and radial distances of the inner and outer race groove curvature centers,X1jand X2jare the axial and radial displacements between the ball center and the outer raceway groove curvature center,and Ri/ois the radius of locus of raceway groove curvature centers.ψjis the azimuth angle of the jth ball in the inertial coordinate system.The superscript indicates the coordinate system.

The position vector of the ball center relative to the race center in the race coordinate system can be written as

where Tir=T(0,Θ,0)is the transformation matrix from the inertial coordinate system to the inner race coordinate system,in which Θ is the tilt angle of the inner race around the Yiaxis.Then,the angular position θbrof the ball in the inner race coordinate system is obtained as

where rbr2and rbr3are components of

The vector of the curvature center at angular position θbrrelative to the inner race center is

The position vector of the ball center relative to the curvature center of the inner race in the azimuth coordinate system is

where Tia=T(ψj,0,0)is the transformation matrix from the inertial to the azimuth coordinate system of the ball.

The contact angle between the ball and the inner race is defined as

The contact deformation between the ball and the raceway is given as

where rbc1,rbc2and rbc3are the components of.

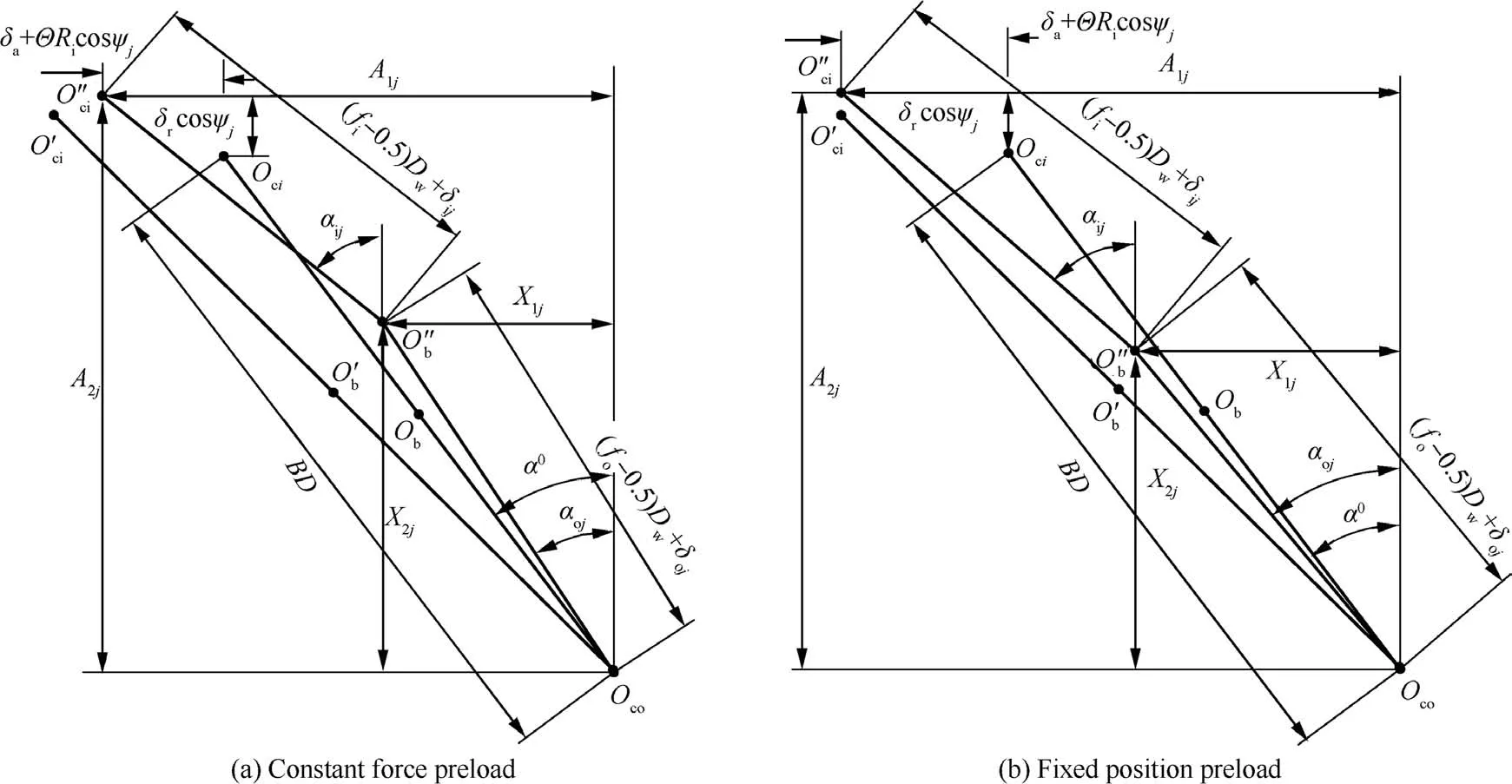
Fig.2 Positions of the ball center and raceway groove curvature centers at angular position ψjwith applied load.

The contact force is calculated according to the Hertz theory as follows:

where Kpis the load-deformation coefficient of point contact,and δ is the contact deformation.

For the ball/race interaction,the load and sliding velocity greatly vary across the elliptical contact zone.However,as the minor axis of the contact ellipse is generally narrow,the change of the sliding velocity along the minor axis can be ignored;thus,the contact area can be divided into equidistant strips along the major axis as shown in Fig.3.The origin Otof the contact coordinate frame is fixed at the center of the contact ellipse,Xtis along the major axis,and Ztis normal to the contact surface.a and b represent the semimajor and semiminor axes of the contact ellipse,respectively.

where Tat=T(0,π - αij,0)is the transformation matrix from the azimuth coordinate system to the contact coordinate system of the ball and the inner race.is the translation velocity of the inner race center,whileandare the angular velocities of the inner race and the ball.andare the position vectors of the strip center relative to the inner race center and the ball center,respectively.

The sliding velocity of the inner race relative to the ball at the strip center is

Then,the sliding speed Vkand the equivalent contact load Qkat the center point of each narrow strip can be calculated as follows:

where vk1and vk2are the components of,a is the semi major axis of the contact ellipse,xkis the distance from the strip center to the ellipse center,and m is the number of strips in the semi major axis.

The tangential force Ftkon the strip is obtained by

where μkis the friction coefficient on the strip.

Fig.3 The kth strip in the contact area.

The components of the tangential force along the major and minor axes are determined by the sliding velocity at the strip center as follows:

where φkis the angle between the sliding velocity at the strip center and the minor axis of the contact ellipse,and φk=arctan

In the contact coordinate system of the ball and the race,the moment vector of the contact strip acting on the ball is

The force vector of the race acting on the ball in the contact coordinate system is

where Qi/ois the contact force between the ball and the inner or outer race.

where Qcis the contact force between the ball and the cage,and Fctxand Fctzare the tangential force components produced by the ball/cage contact.is the position vector of the contact point between the ball and the cage pocket relative to the ball center in the contact coordinate system.

The total force and moment acting on the ball in the azimuth coordinate system are

where the superscripts it,ot,and ct represent the contact coordinate systems of ball/inner race,ball/outer race,and ball/cage,respectively,andis the force vector of the inner race acting on the ball in the ball/inner race contact coordinate system.Tita,Tota,and Tctaare the transformation matrices from the three contact coordinate systems to the azimuth coordinate system,respectively.

2.1.3.Calculation of time-averaged wear rate of bearing parts

Fig.4 Ball loading at angular position ψj.

Fig.5 Flow chart of the dynamic wear simulation program of angular contact ball bearings.

Fig.6 Time-averaged wear rates of ball and cage.

Table 1 Bearing geometrical parameters.

For high-speed instrument angular contact ball bearings,there are differential sliding,spin sliding,and gyro sliding between the ball and race contacts,as well as the sliding of the cage with the ball and guide lands.Friction heating and wear in the contact areas are unavoidable.The Archard adhesive wear theory16can be used to calculate the wear rate of bearing parts.where w is the wear rate of parts,Ksis the wear coefficient,H is the Brinell hardness of the material,and Q and V are the contact load and sliding speed,respectively.For the contact between the ball and the race,the contact ellipse is divided into equidistant strips along the major axis.The product QkVkof the equivalent load and the slip velocity on each strip can be calculated.Then,the QV value of the whole contact area is obtained,which can be substituted into the above equation to calculate the wear rate.For the ball/cage and cage/race interactions,the sliding velocity is normally very high and the size of the contact zone is quite small.It is reasonable to consider the interaction as a point or line contact and use Archard’s equation to compute the instantaneous wear rate.Once the wear rate is known at any time during the simulation time T,a time-averaged wear rate can be calculated asThe time-averaged wear rate of bearing parts can be used to estimate the wear life of the bearing.

2.1.4.Equilibrium constraint equations and differential equations of motion

In order to eliminate the high-frequency vibration caused by the elastic contact between the ball and the race,17and to effectively study the low-frequency performance of the cage motion,equilibrium constraints for balls are used in the model.At each time step,the radial and axial differential equations of the ball motion are replaced by the normal force equilibrium of the ball/race contact.The position of the rolling element center is determined by the equilibrium equation.The loads acting on the ball at angular position ψjare shown in Fig.4,and the equilibrium constraint equations of the ball can be written as

where the contact angles αijand αojare the functions of the ball center position,the subscripts i and o represent the inner and outer race,respectively,and Fcjis the centrifugal force of the ball.In Fig.4,Fitxjand Fityjare the tangential force components of the inner race acting on the ball,and Mgjis the gyro-scopic moment.Qij,Qoj,and Qcjare the contact forces,as discussed above.

Table 2 Bearing material properties.

Fig.7 Effects of the friction coefficient on wear parameters for different preload methods(ΔDw=0).

The position of the inner race center is also determined by the equilibrium constraint equation.For the constant preload model,the inner race center has three degrees of freedom,and the equilibrium equation is

where Fx,Fyand Fzare the axial and radial external loads acting on the inner race,and N is the number of balls.

For the fixed-position preload model,the inner race center is fixed in the axial direction,but it can move in the radial plane.The equilibrium constraint equation can be simplified as

The tangential force of the ball/race contact is relatively small compared to the normal force;therefore,it is not considered in the equilibrium equation in order to improve the computational efficiency.

The position of the inner race center and the axial and radial positions of the ball center can be determined by solving the equilibrium equations of the ball and the inner race.The circumferential position of the ball center is determined by the differential Eq.(28).

Equations of mass center motion and momentum moment of the ball are

where mbis the ball mass,r is the radius of the ball mass center locus,and r=X2j+Ro.Fψis the total force acting on the ball in the circumference direction,and Fψ=-Fb2,where Fb2is the component of Fab.Ibis the ball inertia moment,ωb1,ωb2,and ωb3are the angular velocity components of ωab,and Mb1,Mb2,and Mb3are the moment components of

Equations of mass center motion and momentum moment of the cage are

Fig.8 Effects of the friction coefficient on wear parameters for different preload methods(ΔDw=0.5 μm).

where mcis the cage mass,and Ic1,Ic2,and Ic3are the principal moments of inertia.xc,ycand zcare the displacements of the cage mass center in the inertial coordinate system,and ωc1,ωc2,and ωc3are the angular velocity components of.Fcx,Fcy,and Fczare the components of,while Mc1,Mc2,and Mc3are the components of

Combined solving the equations of mass center motion and momentum moment and Gupta kinematic equation,one can describe the general movement of bearing parts.

2.2.Dynamic block diagram and verification

For the different preload mechanisms of constant-force preload and fixed-position preload,the corresponding dynamic wear simulation program of angular contact ball bearings was developed.The program flow chart is shown in Fig.5.In the figure,i is the step number,t(i)is the simulation time,t_end is the end time,ht is the step size,ht_min is the minimum step size,and dh is the variable step factor.

Compare to the No.2 ball bearing example in Gupta’s Ref.17to verify the present program.The drag coefficient of the lubricant in the ball/race contact is 0.08–0.10(a constant coefficient of 0.09 is assumed in this paper),and the ball/cage and cage/race friction coefficient is 0.10.The bearing operates at a 30000 r/min shaft speed with a 5000 N thrust load and a 4000 N rotating load.The results of this paper program and ADORE are shown in Fig.6.

As can be seen from the time-averaged wear rates of the ball No.1(azimuth angle ψ=0 at the initial moment)and the cage in Fig.6,our results are consistent with ADORE’s in the trend and magnitude,and the value is also very close.The correctness of the program of the constant-force preload model is verified.As the program of fixed-position preload is modified on the basis of the constant-force preload model,the test results are also credible.

3.Results and discussion

The integral value QV of stress and slip velocity of the ball/race contact ellipse is an important parameter that affects the friction heating and wear of high-speed precision angular contact ball bearings.Taking a certain gyro rotor bearing as an example,the changes of the total QV value with the friction coefficient and wear volume under the two preload methods were analyzed.The wear volume of the ball and the race is equivalent to the decrease of the ball diameter ΔDw.Tables 1 and 2 describe the geometrical details and properties of the bearing materials.The wear coefficient in the table is derived from Gupta’s Ref.16.Since the time-averaged wear rate is directly proportional to the wear coefficient,the wear rate trend of bearing parts can be calculated.

3.1.Effects of friction coefficient

For different lubrication conditions,the ball/race drag coefficient μ was set as 0.02,0.05,0.08,and 0.11,18and the ball/cage and cage/race friction coefficient was estimated to be 0.05.19Under the operating conditions of a 30,000 r/min shaft speed and a 7 N thrust load,the effects of the friction coefficient on wear parameters for the two preload methods were analyzed.The wear volume is zero(ΔDw=0),and results are shown in Fig.7.

As can be seen from Fig.7(a)that,under the same initial preload,the QV value decreases with an increase of the friction coefficient for both preload methods.When the friction coefficient is larger than 0.05,the change tends to be stable.Fig.7(b)shows that the normal load of the ball/race contacts offixedposition preload is larger than that of constant-force preload,so the QV value is slightly larger for fixed-position preload.Fig.7(c)and(d)show the sliding velocity distributions in the contact ellipse.The sliding velocity along the major axis of the contact ellipse is caused by gyroscopic motion,and the sliding velocity along the minor axis is the combined velocity of differential sliding and spin sliding of the ball and the raceway.The axis of abscissa represents the dimensionless major axis of the contact ellipse by dividing the actual contact ellipse into equidistant strips.The sliding velocity at the center of each narrow strip is calculated and output.The sliding velocity distributions show that the gyro sliding velocity with a friction coefficient of 0.02 is slightly higher than that of 0.08,which leads to a larger QV value.In the rolling direction,there is one pure rolling point in the ball/outer race contact area,while two pure rolling points in the ball/inner race contact area.The sliding velocity of the ball/outer race contact is higher than that of the ball/inner race contact,which indicates that the ball spins mainly on the outer race.That is because the groove curvature factor of the outer race is larger(0.585),the resistance torque is small,and the ball is prone to spin on the outer race.

The wear of the ball and the raceway is equivalent to the ball diameter reduction.In Fig.8,when the ball diameter wear is 0.5 μm,the residual preload offixed-position preload is obviously smaller than that of constant-force preload,and the QV value decreases as the contact load decreases.The residual preload force here is calculated from the axial component of the contact force between the ball and the inner race.Fig.8(c)and(d)show that when the friction coefficient of the ball/race contact is 0.08,the gyro sliding velocity is very low that is almost zero,and the sliding velocity in the rolling direction is close for the two types of preload.Results indicate that fixed-position preload is more sensitive to a change of the wear volume.The ball diameter is reduced by 0.025%,and the preload is decreased by 60.33%.

3.2.Effects of wear volume

The friction coefficient between the ball and the raceway is given as 0.08,and the results of the ball diameter wear of 0,0.1,0.3,0.5,and 0.7 μm are shown in Fig.9.

Fig.10 Effects of the wear volume on wear parameters for different preload methods(μ=0.02).

As can be seen in Fig.9,when the friction coefficient is 0.08,the QV value of constant-force preload is basically kept constant with an increase of the ball diameter wear,while the QV value offixed-position preload is reduced along with the residual preload.The contact angle of the ball/inner race increases and that of the ball/outer race decreases for fixedposition preload,while both the contact angles increase slowly as the ball diameter wear increases for constant-force preload.The variation of the time-averaged wear rate of the ball is the same as that of the QV value.As shown in Fig.8(c)and(d),the preload force has little influence on the sliding velocity distributions of the contact ellipses when the friction coefficient is about 0.08.

Given the friction coefficient between the ball and the raceway as 0.02,the wear parameters change with the ball diameter wear as shown in Fig.10.With an increase of the ball diameter wear,the QV values fluctuate for both preload methods.This is caused by the variation of the sliding velocity components of the ball/race contacts under different wear volumes.For fixed-position preload,the QV value decreases with a decrease of the contact load on the whole before the ball diameter wear volume is 0.4 μm,and then increases obviously,which indicates that the sliding velocity of the ball/race contacts is increased significantly.Fig.10(c)and(d)show that,for fixed-position preload,when the friction coefficient is small as 0.02,the gyro sliding velocity increases greatly with an increase of the ball diameter wear for insufficient preload.Considering the effect of lubricant viscosity resistance20,when the ball diameter wear is larger than 0.6 μm,a slip occurs in the rolling direction due to an insufficient drag force between the ball and the raceway,which results in an obvious increase in the QV value.

4.Conclusions

(1)A wear numerical simulation model based on the angular contact ball bearing dynamics was established.Considering the in fluences of differential sliding,spin sliding,and gyro sliding of the ball/race contacts,the wear properties of the bearing changing with the friction coefficient,wear,and preload methods were quantitatively analyzed by the QV value of the ball/race contacts.This work can provide a theoretical basis for the preload and preload methods optimization of high-speed instrument ball bearings.

(2)Under the same initial preload,the QV value decreases with an increase of the friction coefficient for both preload methods,and that of the fixed-position preload is slightly greater.When the friction coefficient is larger than 0.05,the velocity of gyro sliding is very small,which can significantly reduce the QV value.The fixedposition preload is more sensitive to a change of the wear volume.The ball diameter is reduced by 0.025%,and the preload is decreased by 60.33%.

(3)Given a friction coefficient of 0.08 between the ball and the race,with the ball diameter wear increasing,the QV value of constant-force preload remains essentially unchanged,while that offixed-position preload reduces with the residual preload.For fixed-position preload,when the friction coefficient is small as 0.02,the gyro sliding velocity increases greatly with an increase of the ball diameter wear for insufficient preload.Meanwhile,a slip may occur in the rolling direction,which will lead to a significant increase in the QV value.

(4)The QV value of ball/race contacts varies with the lubrication condition and wear volume.An estimation of the bearing wear life under different preload methods requires a consideration of changes in the wear rate.

Acknowledgement

This study was supported by the National‘the twelfth fiveyear’Projects of Science and Technology of China.

1.Harris TA.Rolling bearing analysis-essential concepts of bearing technology.5th ed.New York:John Wiley&Sons,Inc.;2001.p.158–63.

2.Alfares MA,Elsharkawy AA.Effects of axial preloading of angular contact ball bearings on the dynamic of a grinding machine spindle system.J Mater Process Technol 2003;136(1–3):48–59.

3.Xu T,Xu GH,Zhang Q,Hua C,Tan HH,Zhang SC,et al.A preload analytical method for ball bearings utilizing bearing skidding criterion.Tribol Int 2013;67(11):44–50.

4.Kaczor J,Raczynski A.The effect of preload of angular contact ball bearings on durability of bearing system.J Eng Tribol 2015;229(6):723–32.

5.Cao YZ,Altintas Y.A general method for the modeling of spindle bearing systems.J Mech Des 2004;126(6):557–66.

6.Li JD,Zhu YS,Xiong QQ,Yan K.Research on axial dynamic stiffness offix-pressure spindle.J Xi’an Jiaotong Univ 2014;48(10):126–30[Chinese].

7.Deng SE,Wang YS,Li XN.Experimental study on the relationship between bearing preload and system natural frequency.J Aerospace Power 2010;25(8):1883–7[Chinese].

8.Spiewak SA,Nickel T.Vibration based preload estimation in machine tool spindles.Int J Mach Tools Manuf 2001;41(4):567–88.

9.Holkup T,Cao HR,Kolar P,Altintas Y,Zeleny J.Thermomechanical model of spindles.CIRP Annals Manuf Technol 2010;59(1):365–8.

10.Jeng YR,Gao CC.Investigation of the ball-bearing temperature rise under an oil-air lubrication system.J Eng Tribol 2001;215(2):139–48.

11.Hagiu GD,Ga fitanu MD.Preload-service life correlation for ball bearings on machine tool main spindles.Wear 1994;172(1):79–83.

12.Jiang SY,Mao HB.Investigation of variable optimum preload for a machine tool spindle.Int J Mach Tools Manuf 2010;50(1):19–28.

13.Li HQ,Shin YC.Analysis of bearing configuration effects on high speed spindles using an integrated dynamic thermo-mechanical spindle model.Int J Mach Tools Manuf 2004;44(4):347–64.

14.Cao HR,Holkup T,Altintas Y.A comparative study on the dynamic of high speed spindles with respect to different preload mechanisms.Int J Adv Manuf Technol 2011;57(9):871–83.

15.Liu CH,Chen XY,Gu JM,Jiang SN,Feng ZL.High-speed wear lifetime analysis of instrument ball bearings.J Eng Tribol 2009;223(3):497–510.

16.Gupta PK,Forster NH.Modeling of wear in a solid-lubricated ball bearing.Tribol Trans 1986;30(1):55–62.

17.Gupta PK.Advanced dynamic of rolling elements.New York:Springer-Verlag;1984.p.9–23.

18.Houpert L.Ball bearing and tapered roller bearing torque:analytical,numerical and experimental results.Tribol Trans 2002;45(3):345–53.

19.Kingsbury EP,Walker R.Motions of an unstable retainer in an instrument ball bearing.J Tribol 1994;116(2):202–8.

20.Schlichting H,Gersten K.Boundary-layer theory.Berlin:Springer-Verlag;2000.p.15-9,93–9,606–8.