GENERALIZED RADFORD BIPRODUCT HOM-HOPF ALGEBRAS AND RELATED BRAIDED TENSOR CATEGORIES

MA Tian-shuiWANG Yong-zhongLIU Lin-lin

(1.Department of Mathematics,School of Mathematics and Information Science,Henan Normal University,Xinxiang 453007,China)

(2.Henan Engineering Laboratory for Big Data Statistical Analysis and Optimal Control,School of Mathematics and Information Science,Henan Normal University,Xinxiang 453007,China)

(3.School of Mathematics and Information Science,Xinxiang University,Xinxiang 453003,China)

GENERALIZED RADFORD BIPRODUCT HOM-HOPF ALGEBRAS AND RELATED BRAIDED TENSOR CATEGORIES

MA Tian-shui1,2,WANG Yong-zhong3,LIU Lin-lin1

(1.Department of Mathematics,School of Mathematics and Information Science,Henan Normal University,Xinxiang 453007,China)

(2.Henan Engineering Laboratory for Big Data Statistical Analysis and Optimal Control,School of Mathematics and Information Science,Henan Normal University,Xinxiang 453007,China)

(3.School of Mathematics and Information Science,Xinxiang University,Xinxiang 453003,China)

In this paper,the Hom-type of Radford biproduct is introduced.By combining generalized smash product Hom-algebra and generalized smash coproduct Hom-coalgebra,we derive necessary and su ff cient conditions for them to be a Hom-bialgebra,which includes the well-known Radford biproduct.

Radford biproduct;quantum Yang-Baxter equation;Yetter-Drinfeld category

In this paper,we unify the Makhlouf-Panaite’s smash product in[10]and Ma-Li-Yang’s in[6],and then extend the Radford biproduct to a more general case.We also construct a class of braided tensor categories(extending the Yetter-Drinfeld category to the Hom-case),and provide a solution to the Hom-quantum Yang-Baxter equation.

2 Preliminaries

Throughout this paper,Kwill be a field,and all vector spaces,tensor products,and homomorphisms are overK.We use Sweedler’s notation for terminologies on coalgebras.For a coalgebraC,we write comultiplication Δ(c)=c1⊗c2for anyc∈C.And we denoteIdMfor the identity map fromMtoM.Any unexplained de finitions and notations can be found in[4–6,14].We now recall some useful de finitions.

De finition 2.1A Hom-algebra is a quadruple(A,µ,1A,α)(abbr.(A,α)),whereAis a linear space,µ:A⊗A−→Ais a linear map,1A∈Aandαis an automorphism ofA,such that

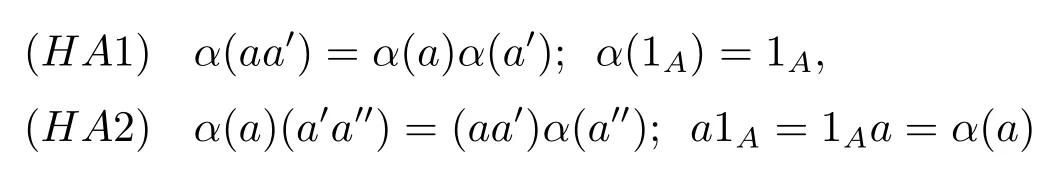
are satisfied fora,a′,a′′∈A.Here we use the notationµ(a⊗a′)=aa′.

Let(A,α)and(B,β)be two Hom-algebras.Then(A⊗B,α ⊗ β)is a Hom-algebra(called tensor product Hom-algebra)with the multiplication(a⊗b)(a′⊗b′)=aa′⊗bb′and unit 1A⊗1B.

De finition 2.2A Hom-coalgebra is a quadruple(C,Δ,εC,β)(abbr.(C,β)),whereCis a linear space,Δ:C−→C⊗C,εC:C−→Kare linear maps,andβis an automorphism ofC,such that

are satisfied forc∈A.Here we use the notation Δ(c)=c1⊗c2(summation implicitly understood).

Let(C,α)and(D,β)be two Hom-coalgebras.Then(C⊗D,α⊗β)is a Hom-coalgebra(called tensor product Hom-coalgebra)with the comultiplication Δ(c⊗d)=c1⊗d1⊗c2⊗d2and counitεC⊗ εD.

De finition 2.3A Hom-bialgebra is a sextuple(H,µ,1H,Δ,ε,γ)(abbr.(H,γ)),where(H,µ,1H,γ)is a Hom-algebra and(H,Δ,ε,γ)is a Hom-coalgebra,such that Δ andεare morphisms of Hom-algebras,i.e.,Δ(hh′)=Δ(h)Δ(h′);Δ(1H)=1H⊗1H,ε(hh′)=ε(h)ε(h′);ε(1H)=1.Furthermore,if there exists a linear mapS:H−→Hsuch that

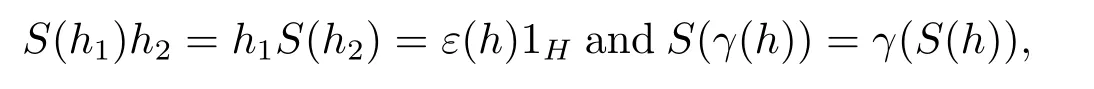
then we call(H,µ,1H,Δ,ε,γ,S)(abbr.(H,γ,S))a Hom-Hopf algebra.

Let(H,γ)and(H′,γ′)be two Hom-bialgebras.The linear mapf:H−→H′is called a Hom-bialgebra map iff◦γ=γ′◦fand at the same timefis a bialgebra map in the usual sense.

De finition 2.4Let(A,β)be a Hom-algebra.A left(A,β)-Hom-module is a triple(M,▷,α),whereMis a linear space,▷:A⊗M−→Mis a linear map,andαis an automorphism ofM,such that

are satisfied fora,a′∈Aandm∈M.

Let(M,▷M,αM)and(N,▷N,αN)be two left(A,β)-Hom-modules.Then a linear morphismf:M−→Nis called a morphism of left(A,β)-Hom-modules iff(h▷Mm)=h▷Nf(m)andαN◦f=f◦αM.

De finition 2.5Let(H,β)be a Hom-bialgebra and(A,α)a Hom-algebra.If(A,▷,α)is a left(H,β)-Hom-module and for allh∈Handa,a′∈A,

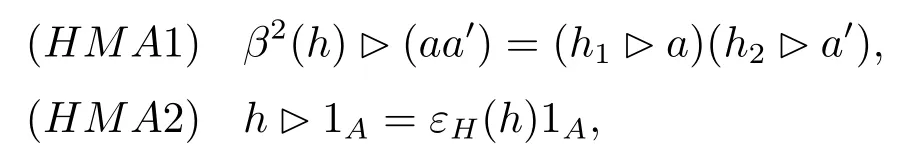
then(A,▷,α)is called an(H,β)-module Hom-algebra.

De finition 2.6Let(C,β)be a Hom-coalgebra.A left(C,β)-Hom-comodule is a triple(M,ρ,α),whereMis a linear space,ρ:M−→C⊗M(writeρ(m)=m−1⊗m0,∀m∈M)is a linear map,andαis an automorphism ofM,such that

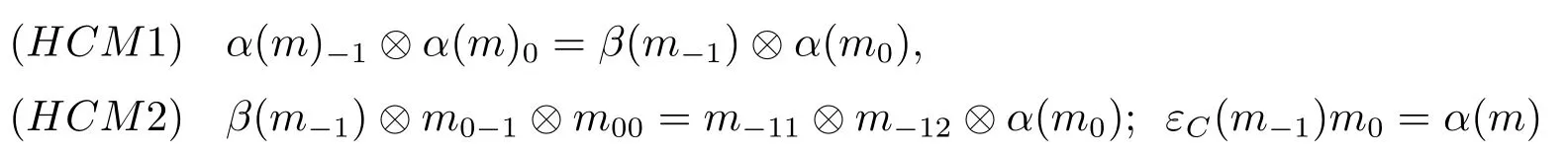
are satisfied for allm∈M.

Let(M,ρM,αM)and(N,ρN,αN)be two left(C,β)-Hom-comodules.Then a linear mapf:M−→Nis called a map of left(C,β)-Hom-comodules iff(m)−1⊗f(m)0=m−1⊗f(m0)andαN◦f=f◦αM.

De finition 2.7Let(H,β)be a Hom-bialgebra and(C,α)a Hom-coalgebra.If(C,ρ,α)is a left(H,β)-Hom-comodule and for allc∈C,

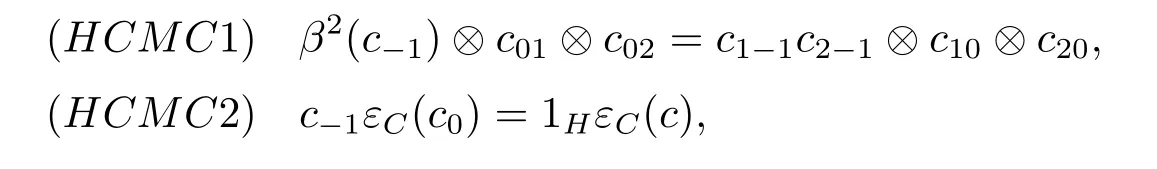
then(C,ρ,α)is called an(H,β)-comodule Hom-coalgebra.

De finition 2.8Let(H,β)be a Hom-bialgebra and(C,α)a Hom-coalgebra.If(C,▷,α)is a left(H,β)-Hom-module and for allh∈Handc∈A,

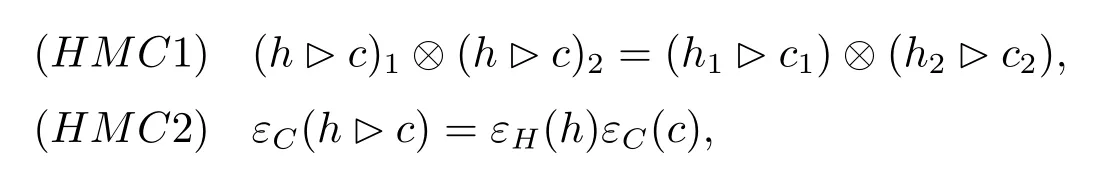
then(C,▷,α)is called an(H,β)-module Hom-coalgebra.

De finition 2.9Let(H,β)be a Hom-bialgebra and(A,α)a Hom-algebra.If(A,ρ,α)is a left(H,β)-Hom-comodule and for alla,a′∈A,

then(A,ρ,α)is called an(H,β)-comodule Hom-algebra.

3 Generalized Radford Biproduct Hom-Hopf Algebra

In this section,we first introduce the notions of generalized smash product Hom-algebraA#mHand generalized Hom-smash coproduct Hom-coalgebra.Then the necessary and sufficient conditions forA#mHandonA⊗Hto be a Hom-bialgebra structure are derived.

Proposition 3.1Let(H,β)be a Hom-bialgebra,(A,▷,α)an(H,β)-module Homalgebra andm∈Z.Then(A#mH,α⊗β)(A#mH=A⊗Has a linear space)with the multiplication(a⊗h)(a′⊗h′)=a(βm(h1)▷α−1(a′))⊗β−1(h2)h′,wherea,a′∈A,h,h′∈H,and unit 1A⊗1His a Hom-algebra.In this case,we call(A#mH,α⊗β)generalized smash product Hom-algebra.

ProofIt is straightforward by the de finition of Hom-algebra.

Remarks(1)Noting that(A#0H,α ⊗ β)is exactly the Ma-Li-Yang’s Hom-smash product in[5,6]and(A#−2H,α ⊗ β)is exactly the Makhlouf-Panaite’s Hom-smash product in[10].

(2)Ifα=IdAandβ=IdHin(A#mH,α ⊗ β),then one can obtain the usual smash productA#Hin[13].

(3)Let(H,µH,ΔH)be a bialgebra and(A,α)a leftH-module algebra in the usual sense with action denoted byH⊗A→A,h⊗ah·a.Letβ:H→Hbe a bialgebra endomorphism andα:A→Aan algebra endomorphism,such thatα(h·a)=β(h)·α(a)for allh∈Handa∈A.If we consider the Hom-bialgebraHβ=(H,β ◦µH,ΔH◦β,β)and the Hom-associative algebraAα=(A,α◦µH,α),then(Aα,α)is a left(Hβ,β)-module Hom-algebra with actionHβ⊗Aα→Aα,h⊗ah▷a:=α(h·a)=β(h)·α(a).

ProofStraightforward.

Proposition 3.2Let(H,β)be a Hom-bialgebra,(C,ρ,α)an(H,β)-comodule Homcoalgebra andn∈Z.Then()(=C⊗Has a linear space)with the comultiplication ΔCH(c⊗h)=c1⊗βn(c2(−1))β−1(h1)⊗α−1(c2(0))⊗h2,wherec∈C,h∈H,and counitεC⊗εHis a Hom-coalgebra.In this case,we call()generalized smash coproduct Hom-coalgebra.

ProofStraightforward.

Remarks(1)()is exactly the Li-Ma’s Hom-smash coproduct in[5].

(2)(2H,α ⊗ β)is exactly the dual version of the Makhlouf-Panaite’s Hom-smash product in[10].

(3)Ifα=IdAandβ=IdHin(A#mH,α ⊗ β),then one can obtain the usual smash coproductA×Hin[13].

Theorem 3.3Let(H,β)be a Hom-bialgebra,(A,α)a left(H,β)-module Hom-algebra with module structure▷:H⊗A−→Aand a left(H,β)-comodule Hom-coalgebra with comodule structureρ:A−→H⊗A.Then the following are equivalent:

(i)(A◇mnH,µA#H,1A⊗1H,ΔAH,εA⊗εH,α⊗β)is a Hom-bialgebra,where(A#mH,α⊗β)is a generalized smash product Hom-algebra and()is a generalized smash coproduct Hom-coalgebra.

(ii)The following conditions hold:

(R1)(A,ρ,α)is an(H,β)-comodule Hom-algebra;(R2)(A,▷,α)is an(H,β)-module Hom-coalgebra;

(R3)εAis a Hom-algebra map and ΔA(1A)=1A⊗1A;

(R4)ΔA(ab)=a1(βm+n+2(a2(−1))▷α−1(b1))⊗α−1(a2(0))b2;

(R5)βn+1((βm+1(h1)▷b)−1)h2⊗(βm+1(h1)▷b)0=h1βn+2(b(−1))⊗ βm+2(h2)▷b(0),wherea,b∈B,h∈Handm,n∈Z.In this case,we call(A◇mnH,α⊗β)generalized Radford biproduct Hom-bialgebra.

ProofBy a tedious computation we can prove it.

Remarks(1)Whenm=n=0 in Theorem 3.3,we can get[5,Theorem 3.3].

(2)Whenα=IdAandβ=IdHin Theorem 3.3,then one can obtain[13,Theorem 1].

Proposition 3.4Let(H,β,SH)be a Hom-Hopf algebra,and(A,α)ba a Hom-algebra and a Hom-coalgebra.Assume that(A◇mnH,α ⊗ β)is a generalized Radford biproduct Hom-bialgebra de fined as above,andSA:A→Ais a linear map such thatSA(a1)a2=a1SA(a2)=εA(a)1Aandα◦SA=SA◦αhold.Then(A◇mnH,α⊗β,SA◇mnH)is a Hom-Hopf algebra,where

ProofFor alla∈A,h∈H,we have

and the rest is direct.

4 Generalized Hom-Yetter-Drinfeld Category

In this section,we construct a class of braided tensor category,which extends the Yetter-Drinfeld category to the Hom-case.Next we give the concept of Hom-Yetter-Drinfeld module via generalized Radford biproduct Hom-Hopf algebra de fined in Theorem 3.3.

De finition 4.1Let(H,β)be a Hom-bialgebra,(U,▷U,αU)a left(H,β)-module with action▷U:H⊗U→U,h⊗uh▷Uuand(U,ρU,αU)a left(H,β)-comodule with coactionρU:U→H⊗U,uu(−1)⊗u(0).Then we call(U,▷U,ρU,αU)a(left-left)Hom-Yetter-Drinfeld module over(H,β)if the following condition holds:

for allh∈Handu∈U.

Proposition 4.2When(H,β)is a Hom-Hopf algebra,(HY D)is equivalent to

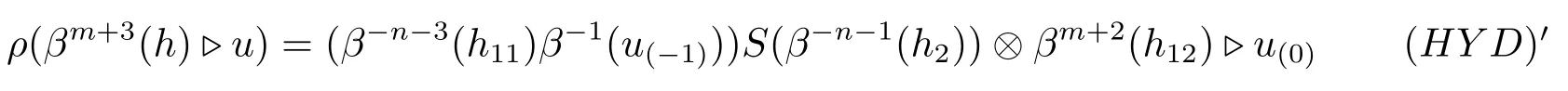
for allh∈H,u∈U.

Proof(HY D)(HY D)′.We have

(HY D)′=⇒(HY D)is proved as follows:

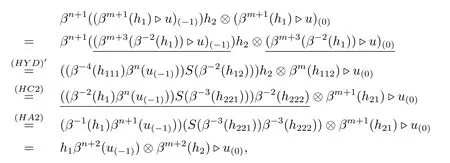
finishing the proof.

De finition 4.3Let(H,β)be a Hom-bialgebra.We denote byHHYD the category whose objects are Hom-Yetter-Drinfeld modules(U,▷U,ρU,αU)over(H,β);the morphisms in the category are morphisms of left(H,β)-modules and left(H,β)-comodules.

In the following,we give a solution to the Hom-quantum Yang-Baxter equation introduced and studied by Yau in[16].

Proposition 4.4Let(H,β)be a Hom-bialgebra and(U,▷U,ρU,αU),(V,▷V,ρV,αV)∈HHYD.De fine the linear map

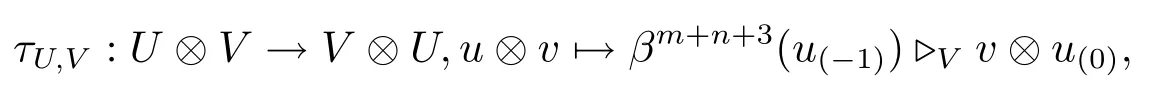
whereu∈Uandv∈V. Then we haveτU,V◦(αU⊗ αV)=(αV⊗ αU)◦ τU,V,if(W,▷W,ρW,αW)∈HHYD,the mapsatisfy the Hom-Yang-Baxter equation

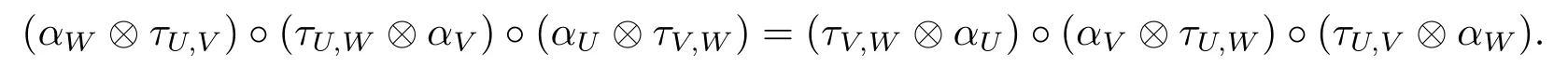
ProofIt is easy to prove the first equality,so we only check the second one.For allu∈U,v∈Vandw∈W,we have

The proof is completed.

Lemma 4.5Let(H,β)be a Hom-bialgebra,if(U,▷U,ρU,αU),(V,▷V,ρV,αV)are(leftleft)Hom-Yetter-Drinfeld modules,then(U⊗V,▷U⊗V,ρU⊗V,αU⊗ αV)is a Hom-Yetter-Drinfeld module with structures

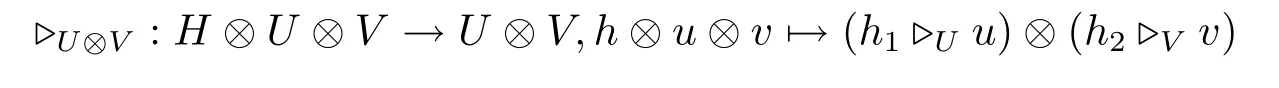
and

for allh∈H,u∈U,v∈V.

ProofIt is easy to check that(U⊗V,▷U⊗V,αU⊗ αV)is an(H,β)-Hom module and(U⊗V,ρU⊗V,αU⊗ αV)is an(H,β)-Hom comodule.Now we check the condition(HY D).For allh∈H,u∈U,v∈V,we have

finishing the proof.

Lemma 4.6Let(H,β)be a Hom-bialgebra,and

With notation as above,de fine the linear map

whereu∈U,v∈Vandw∈W.ThenaU,V,Wis an ismorphism of left(H,β)-Hom-modules and left(H,β)-Hom-comodules.

ProofSame to the proof of[9,Proposition 3.2].

Lemma 4.7Let(H,β)be a Hom-bialgebra and(U,▷U,ρU,αU),(V,▷V,ρV,αV)∈HHYD.De fine the linear map

whereu∈Uandv∈V.ThencU,Vis a morphism of left(H,β)-Hom-modules and left(H,β)-Hom-comodules.

ProofFor allh∈H,u∈Uandv∈V,we have

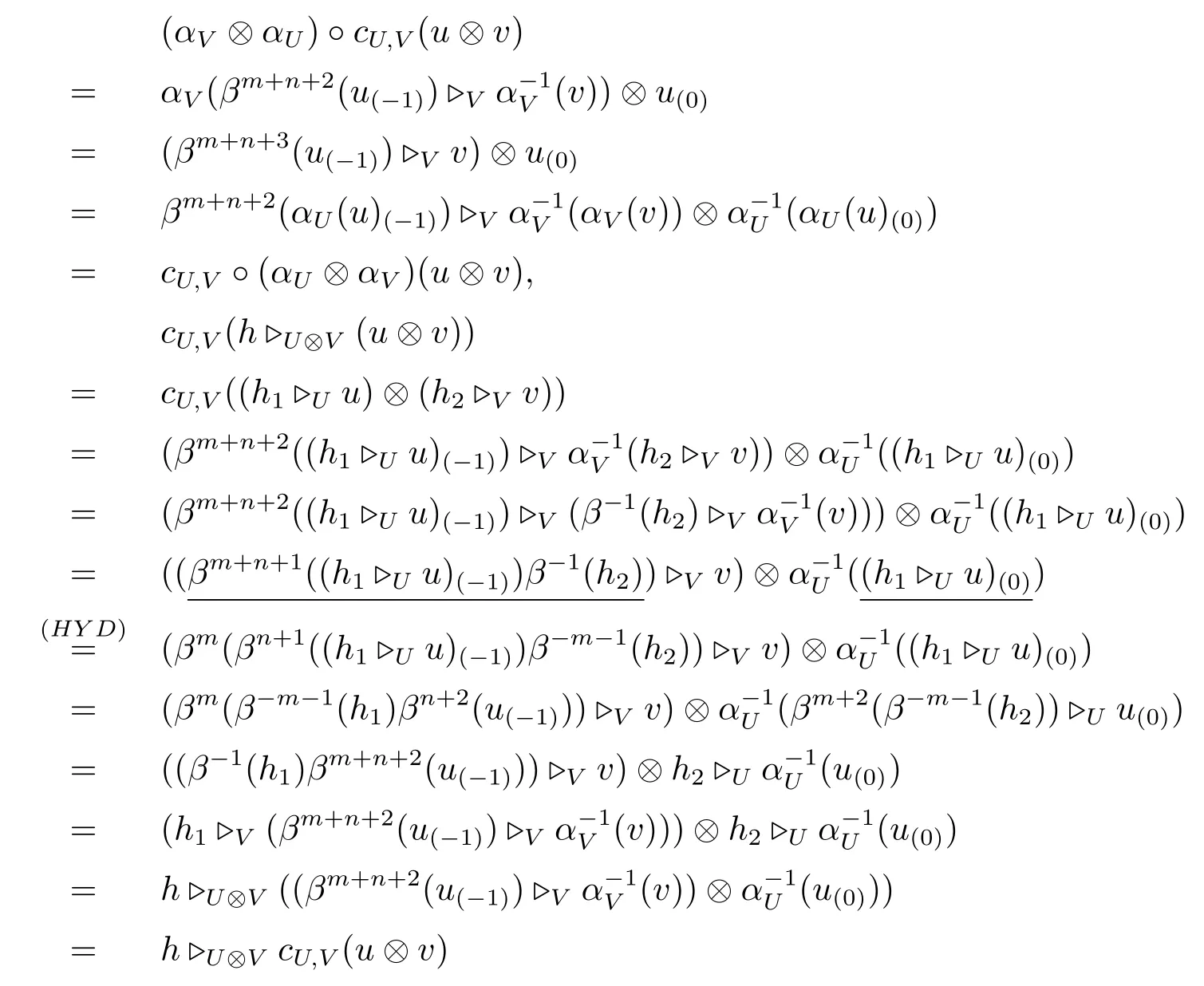
and

finishing the proof.

Theorem 4.8Let(H,β)be a Hom-bialgebra.Then the Hom-Yetter-Drinfeld categoryHHYD is a pre-braided tensor category,with tensor product,associativity constraints,and pre-braiding in Lemmas 4.5,4.6 and 4.7,respectively,and the unitI=(K,IdK).

ProofThe proof of the pentagon axiom foraU,V,Wis same to the proof of[9,Theorem 3.4].Next we prove that the hexagonal relation forcU,V.Let(U,▷U,ρU,αU),(V,▷V,ρV,αV),(W,▷W,ρW,αW)∈HHYD.Then for allu∈U,v∈Vandw∈W,we have

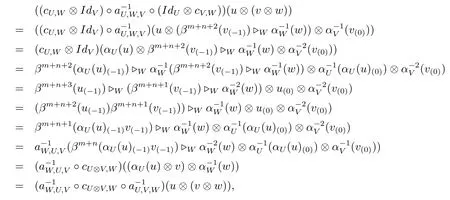
and and the rest is obvious.These complete the proof.

[1]Andruskiewitsch N,Schneider H-J.On the classi fication of finite-dimensional pointed Hopf algebras[J].Ann.Math.,2010,171(1):375–417.

[2]Hartwig J T,Larsson D,Silvestrov S D.Deformations of Lie algebras usingσ-derivations[J].J.Alg.,2006,295:314–361.

[3]Hu Naihong.q-Witt algebras,q-Lie algebras,q-holomorph structure and representations[J].Alg.Colloq.,1999,6(1):51–70.

[4]Kassel C.Quantum groups[M].Graduate Texts in Mathematics 155,Berlin:Springer Verlag,1995.

[5]Li Haiying,Ma Tianshui.A construction of Hom-Yetter-Drinfeld category[J].Colloq.Math.,2014,137(1):43–65.

[6]Ma Tianshui,Li Haiying,Yang Tao.Cobraided smash product Hom-Hopf algebras[J].Colloq.Math.,2014,134(1):75–92.

[7]Ma Tianshui,Li Haiying,Zhao Wenzheng.On the braided structures of Radford’s biproduct[J].Acta Math.Sci.Ser.B Engl.Ed.,2011,31(2):701–715.

[8]Majid S.Double-bosonization of braided groups and the construction ofUq(g)[J].Math.Proc.Cambridge Philos.Soc.,1999,125(1):151–192.

[9]Makhlouf A,Panaite F.Yetter-Drinfeld modules for Hom-bialgebras[J].J.Math.Phys.,2014,55:013501.

[10]Makhlouf A,Panaite F.Twisting operators,twisted tensor products and smash products for Homassociative algebras[J].Glasg.Math.J.,arXiv:1402.1893.

[11]Makhlouf A,Silvestrov S D.Hom-algebra stuctures[J].J.Gen.Lie The.Appl.,2008,2:51–64.

[12]Makhlouf A,Silvestrov S D.Hom-algebras and hom-coalgebras[J].J.Alg.Appl.,2010,9:553–589.

[13]Radford D E.The structure of Hopf algebra with a projection[J].J.Alg.,1985,92:322–347.

[14]Radford D E.Hopf algebras[M].KE Series on Knots and Everything,Vol.49,New Jersey:World Scientic,2012.

[15]Yau D.Module Hom-algebras[J].arXiv:0812.4695v1.

[16]Yau D.Hom-quantum groups II:cobraided Hom-bialgebras and Hom-quantum geometry[J].arXiv:0907.1880.

广义Radford双积Hom-Hopf代数和相关辫子张量范畴

马天水1,2,王永忠3,刘琳琳1
(1.河南师范大学数学与信息科学学院数学系,河南新乡 453007)
(2.河南师范大学数学与信息科学学院大数据统计分析与优化控制河南省工程实验室,河南新乡 453007)
(3.新乡学院数学与信息科学学院,河南新乡 453003)

本文研究了Radford双积的Hom-型.通过把广义smash积Hom-代数和广义smash余积Hom-余代数相结合,得到了他们成为Hom-双代数的充分必要条件,这一结果推广了著名的Radford双积.

Radford双积;量子Yang-Baxter方程;Yetter-Drinfeld范畴

O153.3

16T05;81R50

A

0255-7797(2017)06-1161-12

1 Introduction

LetHbe a bialgebra,A#Ha smash product algebra andA×Ha smash coproduct coalgebra.Radford(see[13])gave a bialgebra structure onA⊗H(named Radford biproduct by other researchers)viaA#HandA×H.Later,Majid made the following conclusion:to any Hopf algebraAin the braided category of Yetter-Drinfeld modulesHHYD,one can associate an ordinary Hopf algebraA★H,there called the bosonization ofA(i.e.,Radford biproduct)(see[8]).While Radford biproduct is one of the celebrated objects in the theory of Hopf algebras,which plays a fundamental role in the classi fication of finite-dimensional pointed Hopf algebras(see[1]).Other references related to Radford biproduct see[1,6–8,13,14].

The algebra of Hom-type can be found in[2]by Hartwig,Larsson and Silvestrov,where a notion of Hom-Lie algebra in the context ofq-deformation theory of Witt and Virasoro algebras(see[3])was introduced.There are various settings of Hom-structures such asalgebras,coalgebras,Hopf algebras,see[6,10–12]and so on.In[15],Yau introduced and characterized the concept of module Hom-algebras as a twisted version of usual module algebras.Based on Yau’s de finition of module Hom-algebras,Ma,Li and Yang[6]constructed smash product Hom-Hopf algebra()generalizing the Molnar’s smash product(see[13]),and gave the cobraided structure(in the sense of Yau’s de finition in[16])on().Makhlouf and Panaite de fined and studied a class of Yetter-Drinfeld modules over Hom-bialgebras in[9]and derived the constructions of twistors,pseudotwistors,twisted tensor product and smash product in the setting of Hom-case(see[10]).Li and Ma studied the Yetter-Drinfeld category of Hom-type via Radford biproduct(see[5]).Recently,Ma,Liu and Li extend the above results in the monoidal Hom-case.

date:2015-07-16Accepted date:2015-11-25

Supported by China Postdoctoral Science Foundation(2017M611291);Foundation for Young Key Teacher by Henan Province(2015GGJS-088);Natural Science Foundation of Henan Province(17A110007).

Biography:Ma Tianshui(1977–),male,born at Tanghe,Henan,associate professor,major in Hopf algebra and its application.