具有幂零奇点的七次Hamilton系统Abel积分的零点个数估计
2017-11-06马慧龙杨纪华
马慧龙,杨纪华
(宁夏师范学院数学与计算机科学学院,宁夏固原 756000)
具有幂零奇点的七次Hamilton系统Abel积分的零点个数估计
马慧龙,杨纪华
(宁夏师范学院数学与计算机科学学院,宁夏固原 756000)
本文研究了具有幂零奇点的七次Hamilton 系统的Abel积分的零点个数问题.利用Picard-Fuchs方程法,得到了Abel积分上零点个数,其中 Γh是,所定义的卵形线和是x和y的次数不超过n的多项式.
Hamilton系统;幂零奇点;Abel积分;Picard-Fuchs方程
1 引言
考虑如下Hamilton系统的扰动向量场

其中0<|ε|≪1,H(x,y)是关于x和y的m+1次实多项式,f(x,y)和g(x,y)是关于x和y的次数不超过n的实多项式.假设系统(1.1)的未扰动系统(1.1)ε=0有连续闭轨线族{Γh},Σ为Γh的最大存在开区间,即

考虑以下积分

(1.2)式称为Abel积分.寻找Abel积分零点个数的最小上界Z(m,n)称为弱Hilbert 16问题或Hilbert-Arnold问题[1].相关的研究很多.例如,Khovansky和Varchenko独立地证明了Z(m,n)的有限性,但是没有给出具体的表达式[2,3].李承治和张芷芬得到了Z(2,2)=2[4].对于H(x,y)=y2+x3−x,Horozov和Iliev通过研究相应的Picard-Fuchs方程得到Z(2,n)≤5n+15[5].另外,Petrov分别研究了Hamilton函数H(x,y)=y2−x+x3,H(x,y)=y2+x2−x4和H(x,y)=y2−x2+x4的相应Abel积分I(h)的零点的个数[6−9].对于4次Hamilton函数H(x,y)=−x2+x4+y4和H(x,y)=x2+y2+ax4+y4,Zhou和Li得到了相应Abel积分零点个数的上界[10,11].当m>4时,由于很难得到Abel积分I(h)的代数结构,目前研究结果很少[10].
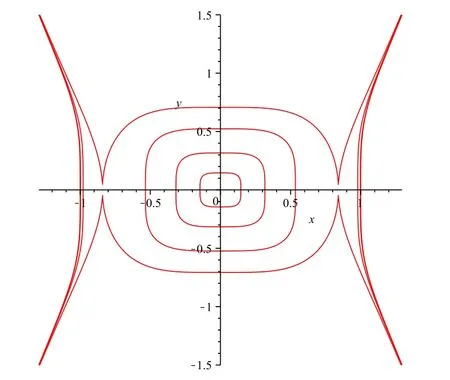
图1:系统(1.4)的相图
受文献[5,10,11]的启发,本文研究八次Hamilton函数
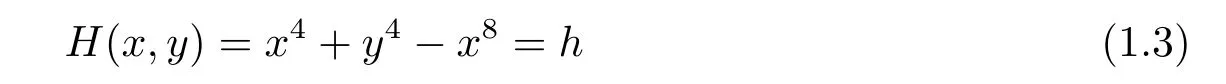
相应的向量场

记B(n)为Abel积分I(h)在上的零点个数(计重数),其中n=max{degf,degg}.本文的主要结果为
定理1.1对Hamilton函数
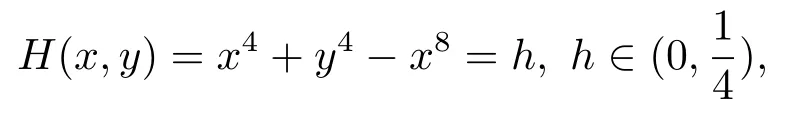
B(n)≤而且B(1)=0,其中[·]是取整函数.
2 Abel积分I(h)的代数结构
由分部积分公式可知
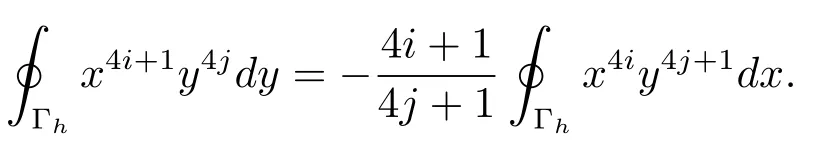
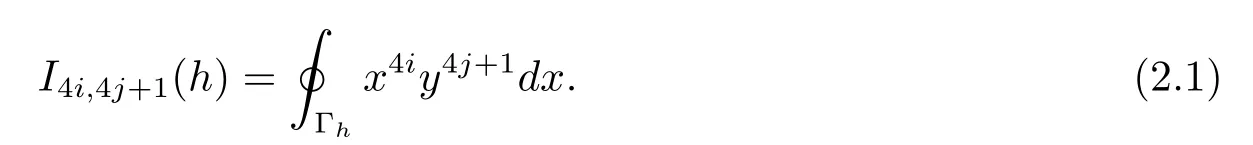
引理2.1假设n≥5,对于Hamilton函数(1.3),I(h)可表示为
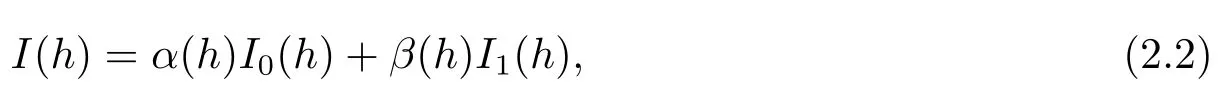
其中α(h)和β(h)是关于h的多项式,且k和k−1分别是degα(h)和degβ(h)的最小上界.
证分两步进行证明.
(1)证明对n=4i+4j+1=4k+1≥5,积分I4i,4j+1可以表示为Il,m(l+m=4k−3)和hIl,m(l+m=4k−7或4k−3)的线性组合.
事实上,对(1.3)式两端同时关于x求导可得

(2.3)式两端同乘以x4i−7y4j+1dx并沿着Γh积分可得
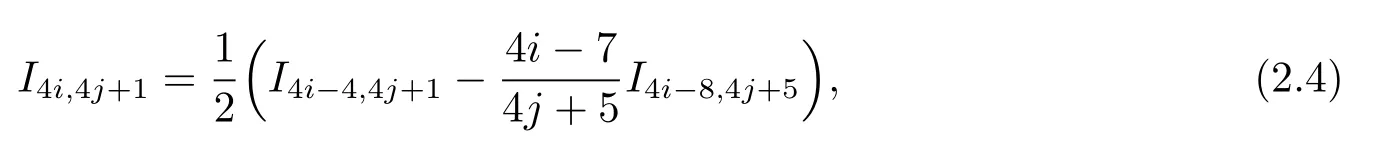
其中i≥2.(1.3)式两端同乘以x4iy4j−3并沿着Γh关于x积分可得
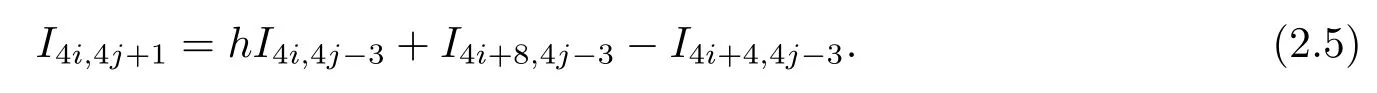
由(2.4)式可得
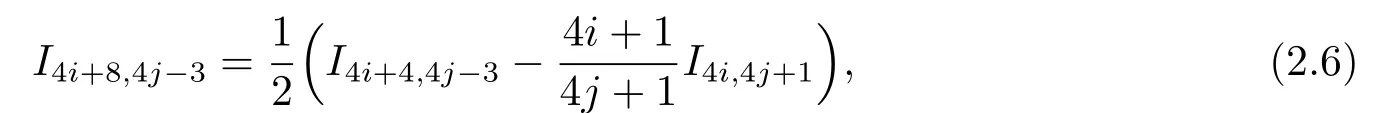
把(2.6)式代入(2.5)可得
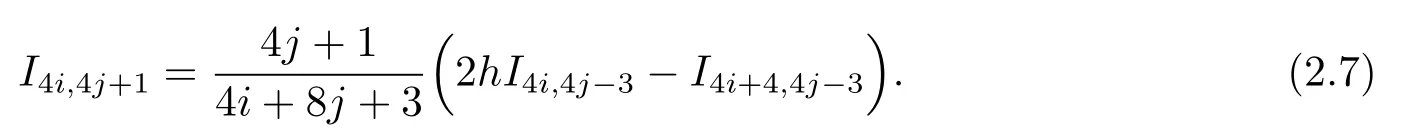
由(2.5)式可得
把(2.8)式代入(2.4)式可得
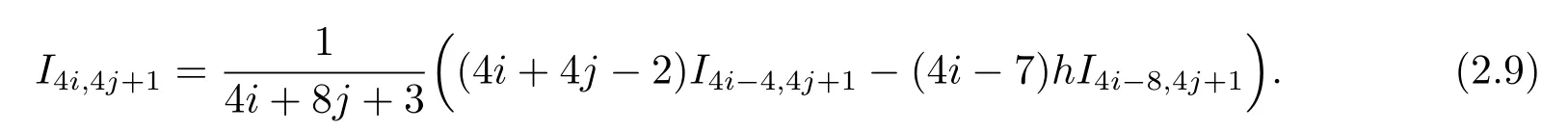
在(2.7)式中分别取(i,j)=(0,k),(1,k−1),在(2.9)式中分别取(i,j)=(2,k−2),(3,k−3),···,(k−1,1),(k,0),可得

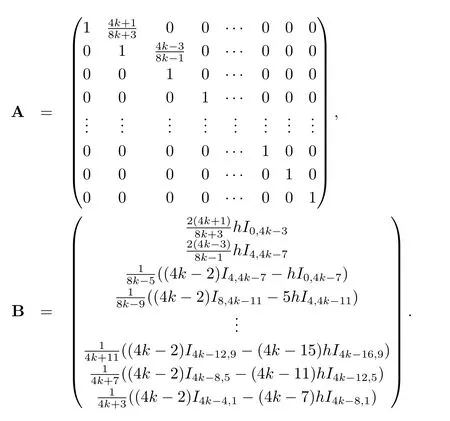
计算可得|A|=1,且B中元素仅含有积分Il,m(l+m=4k−3)和hIl,m(l+m=4k−7或4k−3).
(2)用数学归纳法证明I(h)=α(h)I0(h)+β(h)I1(h),并且
事实上,由(2.7)和(2.9)式可得

即当k=1,2时结论成立,其中n=4k+1.假设当k≤s−1时,结论成立.那么当k=s时,由等式(2.10)和第1步的结论可得

其中degα4k−3(h),degγ4k−3(h)≤k−1,degβ4k−3(h),degδ4k−3(h)≤k−2.因此
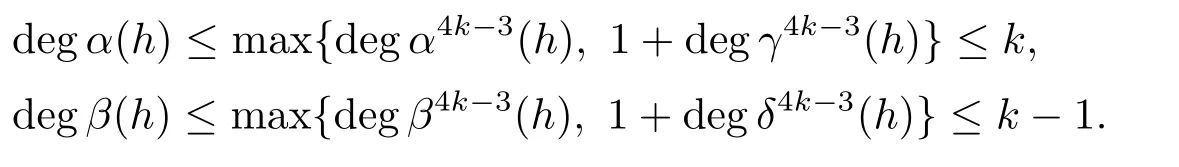
即对任意的n,并且k和k−1分别是degα(h)和degβ(h)的最小上界.证毕.
3 Picard-Fuchs方程和Riccati方程
本小节将得到I0和I1满足的Picard-Fuchs方程.
引理3.1对于Hamilton函数(1.3),I0和I1满足Picard-Fuchs方程

证(1.3)式两端同时关于h求导可得进而可得

所以

(3.2)式两端同乘以h可得

另一方面,
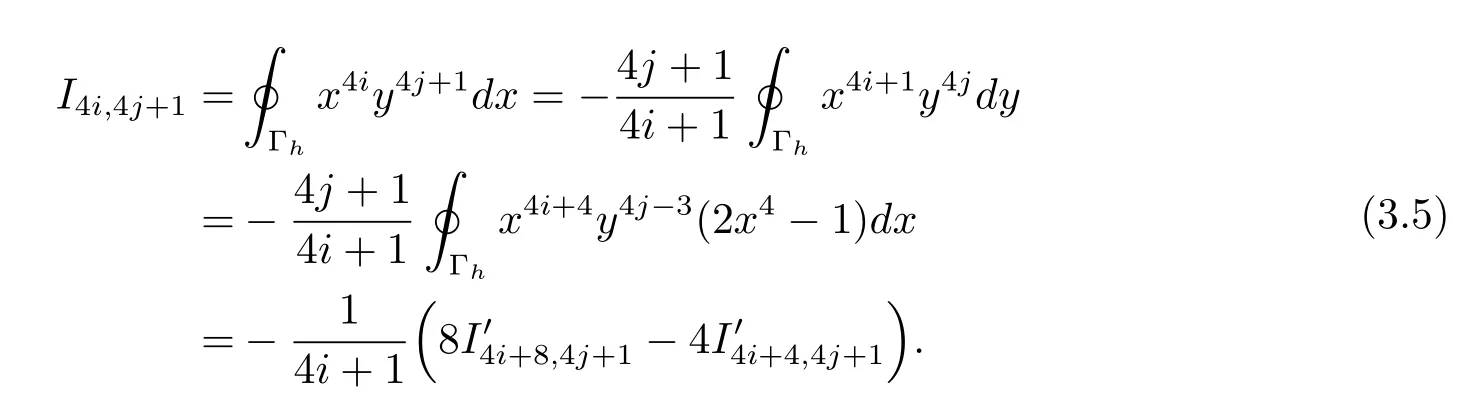
由(3.3)–(3.5)式可得

在(3.6)式中分别取(i,j)=(0,0)和(1,0)可得

注意到(2.11)式即可得结论成立.证毕.
引理3.2对于Hamilton函数(1.3),满足如下的Riccati方程
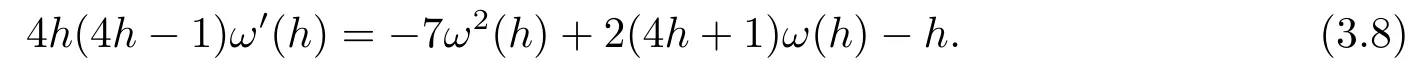
证由于结合(3.1)式即可得(3.8)式.证毕.
4 主要结果的证明
引理4.1设则W(h)满足
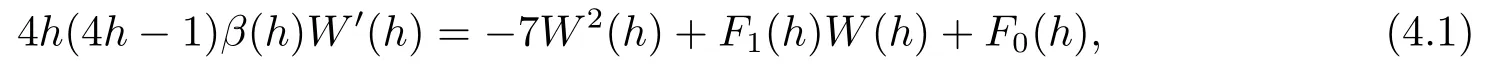
其中degF0(h)≤2p,F1(h)=4h(4h−1)β′(h)+2(4h+1)β(h)+14α(h),

证因为所以W′(h)=α′(h)+β′(h)ω(h)+β(h)ω′(h).注意到(3.8)式即可得证.证毕.
引理4.2假设Σ0=(a,b)⊂Σ,则在Σ0上有#W(h)≤#F0(h)+#β(h)+1,其中#W(h)表示W(h)在Σ0上零点的个数(计重数).
证分两步进行证明.
(1)如果α(h)和β(h)没有公因子,则W(h)和β(h)没有公共零点.设ξ1,ξ2,···,ξk是β(h)在 Σ0中的所有根,ξ0=a,ξk+1=b,ξj< ξj+1,j=0,1,2,···,k.设F0(h)在(ξj,ξj+1)中有ηj个零点.记h1,h2是W(h)在(ξj,ξj+1)中的两个相邻零点,则
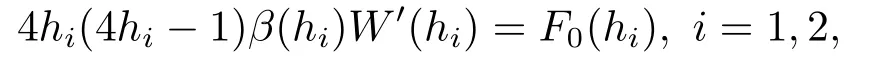
所以在(ξj,ξj+1)中F0(h1)F0(h2)≤0,也就是说W(h)在(ξj,ξj+1)中的任何两个相邻零点之间至少有F0(h)的一个零点.因此W(h)在(ξj,ξj+1)中至多有ηj+1个零点,进而可得
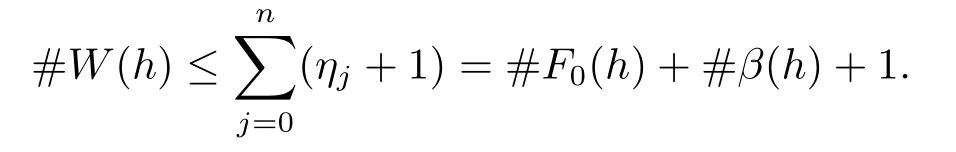
(2)如果α(h)和β(h)有公因子ν(h),记其中对于W1(h)按照(1)的证明过程可得结论成立.证毕.
定理1.1的证明当n≥5时,因为且I0(h)≠0,所以在上,W(h)与I(h)的零点个数相同.由引理4.2知
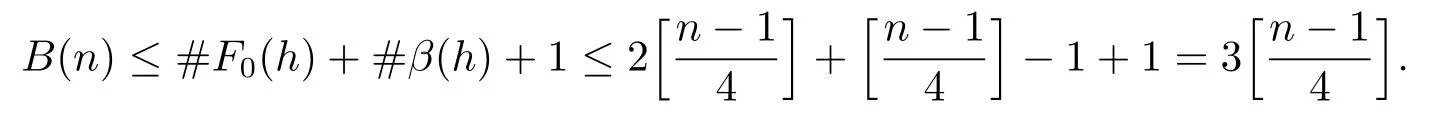
当n=1时,I(h)=c0I0,其中c0为非零常数.因为I0≠0,所以B(1)=0.证毕.
[1]Arnold V.Loss of stability of self-oscillation close to resonance and versal deformation of equivariant vector fields[J].Funct.Anal.Appl.,1977,11:1–10.
[2]Khovansky A.Real analytic manifolds with finiteness properties and complex Abelian integrals[J].Funct.Anal.Appl.,1984,18:119–128.
[3]Varchenko A.Estimate of the number of zeros of an Abelian integral depending on a parameter and limit cycles[J].Funct.Anal.Appl.,1984,18:98–108.
[4]Li C,Zhang Z.Remarks on 16th weak Hilbert problem forn=2[J].Nonlinearity,2002,15:1975–1992.
[5]Horozov E,Iliev I.Linear estimate for the number of zeros of Abelian integrals with cubic Hamiltonians[J].Nonlinearity,1998,11:1521–1537.
[6]Petrov G.Number of zeros of complete elliptic integrals[J].Funct.Anal.Appl.,1984,18:148–149.
[7]Petrov G.Elliptic integrals and their nonoscillation[J].Funct.Anal.Appl.,1986,20:37–40.
[8]Petrov G.Complex zeros of an elliptic integral[J].Funct.Anal.Appl.,1987,21:247–248.
[9]Petrov G.Complex zeros of an elliptic integral[J].Funct.Anal.Appl.,1989,23:160–161.
[10]Zhou X,Li C.Estimate of the number of zeros of Abelian integrals for a kind of quartic Hamiltonians with two centers[J].Appl.Math.Comput.,2008,204:202–209.
[11]Zhou X,Li C.On the algebraic structure of Abelian integrals for a kind of pertubed cubic Hamiltonian systems[J].J.Math.Anal.Appl.,2009,359:209–215.
ON THE NUMBER OF ZEROS FOR ABEL INTEGRALS OF HAMILTON SYSTEM OF SEVEN DEGREE WITH NILPOTENT SINGULARITIES
MA Hui-long,YANG Ji-hua
(School of Mathematics and Computer Science,Ningxia Normal University,Guyuan 756000,China)
In this paper,we study the number of zeros for Abel integrals of Hamilton system of seven degree with nilpotent singularities. By using the Picard-Fuchs equation method,we derive that the number of zeros of Abel integralson the open intervalis at mostwhere Γhis an oval lying on the algebraic curveandare polynomials ofxandyof degrees not exceedingn.
Hamilton system;nilpotent singularity;Abel integral;Picard-Fuchs equation
34C07;34C05
O175
A
0255-7797(2017)06-1227-07
2016-07-29接收日期:2017-06-20
国家自然科学基金(11701306);宁夏师范学院重点科研项目(NXSFZD1708;NXSFZD1606).
马慧龙(1975–),男,宁夏固原,讲师,主要研究方向:微分方程及其应用.
