The effect of Görtler instability on hypersonic boundary layer transition

Min Yu，Xiang-Jiang Yuan

China Academy of Aerospace Aerodynamics，Beijing 100074，China

The effect of Görtler instability on hypersonic boundary layer transition

Min Yu∗，Xiang-Jiang Yuan

China Academy of Aerospace Aerodynamics，Beijing 100074，China

H i G H L i G H T s

•Görtler instability may not dominate the transition process at hypersonic speeds.

•The interaction between Görtler and Mack modes promote the onset of the transition.

•Görtler vortices act as a catalyst to promote the nonlinear growth of the modes.

•Mack mode is more susceptible to nonlinear interaction than Görtler mode.

A R T i C L Ei N F O

Article history:

14 December 2015

Accepted 14 February 2016

Available online 4 March 2016

Concave surface

Görtler instability

Mack mode

Boundary layer transition

The evolution of Görtler vortices and its interaction with other instabilities are investigated in this paper. Both the Mack mode and the Görtler mode exist in hypersonic boundary-layer flows over concave surfaces，and their interactions are crucially important in boundary layer transition.We carry out a direct numerical simulation to explore the interaction between the Görtler and the oblique Mack mode. The results indicate that the interaction between the forced Görtler mode and the oblique Mack mode promotestheonsetofthetransition.TheforcedobliqueMackmodeissusceptibletononlinearinteraction. Because of the development of the Görtler mode，the forced Mack mode and other harmonic modes are excited.

©2016 The Authors.Published by Elsevier Ltd on behalf of The Chinese Society of Theoretical and Applied Mechanics.This is an open access article under the CC BY-NC-ND license（http:// creativecommons.org/licenses/by-nc-nd/4.0/）.

TheLaminar-turbulenttransitionofhypersonicboundary-layer flows is a fundamental and important subject.Reshotko［1］，Morkovin and Reshotko［2］，Saric et al.［3］，Schneider et al.［4］，and Fedorov［5］have reviewed and extensively discussed this subject.Boundarylayertransitioniscomplexathypersonicspeedsbecause different families of instability modes coexist，their nonlinear interaction is inevitable，and they have yet to be identified and discussed.In hypersonic boundary layers，primary unstable waves include first and second mode（Mack mode）instabilities，crossflow instability，Görtler instability，and attachment-line instability.

When boundary layer flows move along a concave wall，unstable waves induced by curvature effects are excited because of centrifugal forces and are subject to Görtler instability，which is manifested in the form of counter-rotating pairs of stationary streamwise vortex-like disturbances（Görtler vortices）.Görtler instability together with Mack modes and other instabilities play an important role in promoting earlier transition［6，7］over a concave surface in a hypersonic boundary layer.Many numerical investigations have focused on secondary instabilities of Görtler vortices［8，9］andtheinteractionsbetweenGörtlermodeandhighfrequencysecondmodes［7，10］.Inadditiontosecondaryinstability and Görtler-second mode interaction routes，there exist many other scenarios for transition over this surface.To this end，we examine the interaction between the Görtler and oblique Mack modes，and focus on the effect of Görtler instability on transition.

Directnumericalsimulationisperformedusingunsteadythreedimensional Navier-Stokes equations as

The flux vectors are:

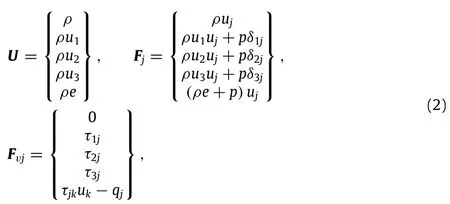
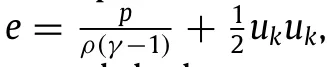
The stress tensorτijand the heat conduction term qjare given as:

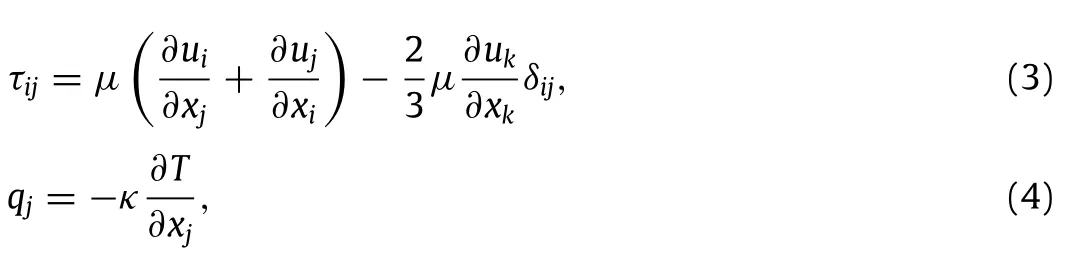
whereµ is the molecular viscosity coefficient，determined by Sutherland’s law.κrepresents the heat conductivity coefficient and can be calculated with a constant Prandtl number Pr.

The gas is assumed to be a thermally and calorically perfect gas satisfying

Using Steger-Warming’s splitting，we separate the inviscid fluxes into an upwind flux and a downwind flux.Then we apply a 5th-order weighted essentially non-oscillatory（WENO）scheme tothesefluxes.Fortheviscousterms，a6th-ordercentraldifference scheme is used.A 4th-order Runge-Kutta scheme is applied to the time integration.

The boundary conditions are specified as follows.The velocity distribution is prescribed at the inlet；at the upper boundary，the flow is approximated by the far field at infinity；the extrapolated boundary condition is enforced at the outlet；a non-slip condition is imposed on the surface.

The same flow configuration and conditions as those described in Ref.［7］are used in this study.The Mach number Ma in the free stream is assumed to be 6.The stagnation pressure and temperature are 896.3 kPa and 433 K，respectively.The wall condition is an adiabatic surface condition.The unit Reynolds number is 9.9×106per meter and a 20-m radius of curvature is applied.

We can obtain inlet forcing disturbances using the linear stability theory（LST）.Numerical simulation is performed under the condition where the Görtler mode has a spanwise wavelength of 7.5 mm to achieve the most amplified Görtler mode.We chose an unstable Mack mode with a frequency of approximately 18.8 kHz and a spanwise wavelength of 30 mm，which is close to the most unstable first mode.The initial amplitudes of the freestream velocity for different modes were set to be 0.01.In this part，we analyze three cases.For Case 1（Mack mode only），we introduce only the Mack mode at the inlet，and for Case 2（Görtler mode only），we introduce only the Görtler mode at the inlet.We then studied the linear and nonlinear developments of the forced modes from these two cases.In Case 3（Görtler-Mack mode），we simultaneously superimposed both the Görtler and Mack modes at the inlet to explore the interaction between the two.

After performing Fourier transformations of the time signal and spanwise direction of the wave disturbances，we can getthe amplitudes of these modes.In this paper，the streamwise velocity disturbance u′is used as the criterion to calculate the amplitudes of the modes.Figure 1 shows the downstream amplitude development of the forced modes（dash lines are obtained by LST）.At linear stages，the growth rate of the forced Mack mode is almost equal to that of the Görtler mode.The Mack mode in Case 1 develops linearly up to about x=1.8 m，and then the development turns into an early nonlinear regime.In Case 2，the forced Görtler mode experiences linear growth in almost the whole computation domain.Both modes in Case 3 depart from linear growth in a small downstream distance（about x=1.7 m）. Interaction between the Görtler and Mack modes can alter the growth characteristics of the forced modes.As shown in Fig.1，the Mack mode shows a larger change than the Görtler mode in the early nonlinear evolution region.We can conclude that，compared to the Görtler mode，the Mack mode is more apt to be influenced by nonlinear interaction.

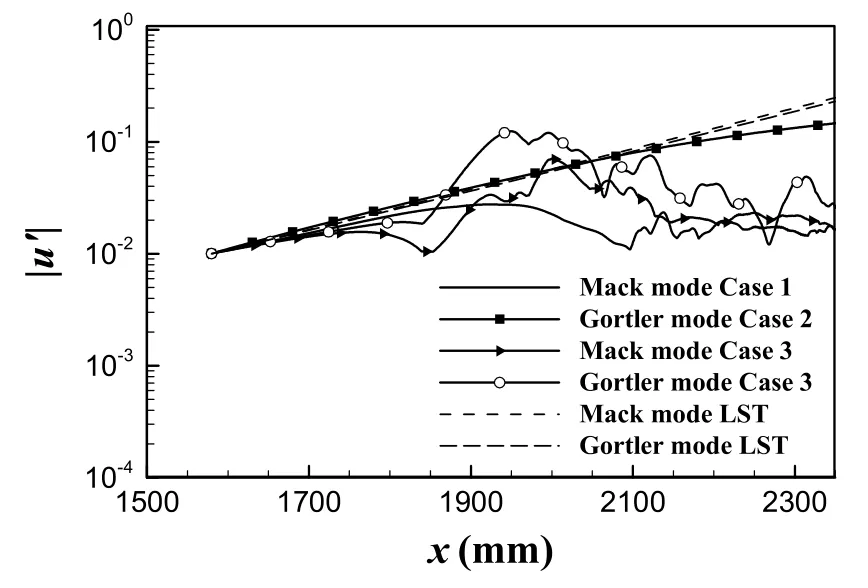
Fig.1.Streamwise velocity disturbance versus downstream distance for the cases.

Fig.2.Streamwise development of skin-friction coefficient for three different cases.

Figure 2 shows the evolutions of the skin-friction coefficient cffor the three cases.We observed no obvious increase of cfin Case 2 inside the computation domain，which means that a transition scenario is difficult to initiate using the Görtler mode only and its simulation is time-consuming.Case 1 in Fig.2 shows that using onlytheobliqueMackmodemayleadtoboundarylayertransition. The transition location in Case 3 is much closer to the upstream than that in Case 1，because of the presence of the Görtler mode. The interaction between the Görtler and Mack modes triggers the onset of a transition to the turbulent state.

Figure 3 shows the development of selected modes（h，k）.A particular wave is identified using its frequency h and spanwise wavenumber k with notation（h，k）.Here h denotes the multiple of the fundamental frequency（18.8 kHz）and k denotes the multiple ofthesmallestspanwisewavenumber（β=209.44m-1）.TheCase 2analysis，withnotransitionprocess，isneglected.Modes（1，1）and（0，4）denote the imposed Mack and Görtler modes，respectively. Mode（0，0）has the highest amplitude and increases in the whole computation domain，indicating the mean flow distortion.The fastgrowth of the amplitude triggers a spectrum broadening.Some damping modes are excited when the amplitude of the forced modes reachesa certainlevel.The nonlinear interaction term（1，5）in Case 3，generated directly from（1，1）and（0，4），grows strongly and early.Modes with a higher harmonic frequency and spanwise wavenumber，such as（0，8）and（2，1），are also amplified.As shown in Fig.3，the amplitudes of all modes reach the same order of magnitude as nonlinear saturation begins at about x=2.1 m（for Case 3）and further downstream（for Case 1）.

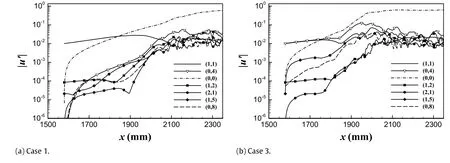
Fig.3.Development of streamwise velocity amplitude of selected modes from Case 1 and Case 3.

Fig.4.Streamwise velocity disturbance versus downstream distance for the cases with a spanwise wavelength of 15 mm.

Fig.5.　Streamwise development of skin-friction coefficient at a spanwise wavelength of 15 mm.

We analyzed the Mack mode with a spanwise wavelength of 15 mm and it does not show the strong streamwise amplification of the Mack mode mentioned above.Also，the same three cases（Mack mode only，Görtler mode only，and Görtler-Mack mode）are explored here.The streamwise amplitude development of the forced modes and the skin-friction coefficient are given in Figs.4 and 5.Because the amplification of the Mack mode is low，it is difficult to induce a transition by Mack mode only.When the Görtler mode is introduced，it interacts with the Mack mode and this interaction triggers the boundary layer transition.The Mack mode is shown being more sensitive than the Görtler mode and is influenced more by its interaction with the Görtler mode.Under thisspanwisewavenumbercondition，thetransitionbeginsfurther downstream.

In this study，we examined the development of Görtler vortices and the interaction between the Görtler and the oblique Mack mode using linear stability analysis and direct numerical simulation.The model we used was obtained from Ref.［7］，which leads to Görtler instability in a hypersonic boundary layer. However，differing from preceding investigations，in this paper we explore the role of Görtler instability on transition and the Görtler-oblique mode interaction route.Görtler instability may not dominate the transition process.A Mack mode with large streamwise amplification leads to transition occurring further downstream.But the interaction between the Görtler and Mack modes has a relatively greater influence on the boundary layer transition.Görtler vortices act as a catalyst to promote the nonlinear growth of the modes.The flow heads towards a transition because of the Görtler-Mack mode interaction.The evolution of the forced oblique Mack mode has a much greater effect than that of the Görtler mode in the nonlinear interaction stage.

［1］E.Reshotko，Transition issues at hypersonic speeds，AIAA paper，2006-707（2006）.

［2］M.V.Morkovin，E.Reshotko，T.Herbert，Transition in open flow systems:a reassessment，Bull.APS 39（1994）1-31.

［3］W.S.Saric，E.Reshotko，D.Arnal，Hypersonic laminar-turbulent transition，AGARD AR-391，2（1998）.

［4］S.P.Schneider，Laminar-turbulenttransitiononreentrycapsulesandplanetary probes，J.Spacecr.Rockets 43（2006）1153-1173.

［5］A.Fedorov，Transition and stability of high speed boundary layers，Annu.Rev. Fluid Mech.43（2011）79-95.

［6］C.W.Whang，X.L.Zhong，Direct numerical simulation of Görtler instability in hypersonic boundary layers，AIAA paper，1999-291（1999）.

［7］F.Li，M.Choudhari，C.L.Chang，Development and breakdown of Görtler vortices in high speed boundary layers，AIAA paper，2010-705（2010）.

［8］Ren Jie，Fu Song，Floquet analysis of fundamental subharmonic and detuned secondary instabilities of Görtler vortices，Science China Physics，Sci.China Phys.Mech.Astronom.57（2014）555-561.

［9］Jie Ren，Song Fu，Secondary instabilities of Görtler vortices in high-speed boundary layers，J.Fluid Mech.781（2015）388-421.

［10］C.W.Whang，X.L.Zhong，Nonlinear interaction of Görtler and second modes in hypersonic boundary layers，AIAA paper，2000-536（2000）.

20 October 2015

.

E-mail addresses:yumin8688@126.com（M.Yu），yuan_xj18@163.com（X.-J.Yuan）.

http://dx.doi.org/10.1016/j.taml.2016.02.003

2095-0349/©2016 The Authors.Published by Elsevier Ltd on behalf of The Chinese Society of Theoretical and Applied Mechanics.This is an open access article under the CC BY-NC-ND license（http://creativecommons.org/licenses/by-nc-nd/4.0/）.

in revised form

*This article belongs to the Fluid Mechanics