Isotope Effects on Two-Photon Population Transfer Processes of HF and DF

Yu-hui Png，Bin-in Wng，Yong-chng Hn∗，Shu-lin Cong，Ying-yu Niu. School of Physics nd Optoelectronic Technology，Dlin University of Technology，Dlin 116024，Chin . School of Science，Dlin Jiotong University，Dlin 116028，Chin



Isotope Effects on Two-Photon Population Transfer Processes of HF and DF

Yu-hui Panga，Bin-bin Wanga，Yong-chang Hana∗，Shu-lin Conga，Ying-yu Niub
a. School of Physics and Optoelectronic Technology，Dalian University of Technology，Dalian 116024，China b. School of Science，Dalian Jiaotong University，Dalian 116028，China

（Dated：Received on September 2，2015；Accepted on January 13，2016）

The isotope effects of XF（X=H，D）on the population transfer process via two-photon resonance excitation are investigated by solving the time-dependent Schr¨odinger equation. The vibrational levels υ=0 and 2 of the ground electronic state are taken to be the initial and target states，respectively，for the two molecular systems. The influences of the field peak amplitude and pulse duration on the population transfer process are discussed in detail. The pulse duration is required to be longer than 860 fs for the DF molecule to achieve a relatively high transfer probability（more than 80%），while the one for the HF molecule is just required to be longer than 460 fs. Moreover，the intermediate level υ=1 and the higher level υ=3 may play more important roles in the two-photon resonance process for the DF molecule，compared to the roles in the process for the HF molecule.

Key words：Population transfer，Two-photon resonance transition，Isotope effects，DF

∗Author to whom correspondence should be addressed. E-mail：ychan@dlut.edu.cn

I. INTRODUCTION

Population transfer between two quantum states has been extensively studied in recent years for the important applications in molecular spectroscopy［1］，chemical reaction dynamics［2］，collision dynamics［3］，and so on. Therefore，to obtain an efficient population transfer （close to 100%）from an initial state to a target state，various techniques are proposed，including the stimulated Raman adiabatic passage（STIRAP）［4］，the temporal coherent control［5］，the chirped adiabatic passage［6］etc. With the development and application of the ultra-intense and ultra-short laser pulse technique，the nonlinear optical phenomena may occur during the laser-molecule interactions. The multiphoton transition process is one of the most typical nonlinear phenomena. Accordingly，the multiphoton resonance is regarded to be an alternative way to achieve efficient population transfer. Gibson showed that the adiabatic transfer of population was possible on high order multiphoton（at least 12-photon）transitions［7］. Maeda et al. observed the population transfer in the Li atom between Rydberg states by high order multiphoton adiabatic rapid passage［8］. Demeter and Djotyan investigated the practical usefulness of multiphoton adiabatic transitions for the manipulation of the atomic mechanical state［9］. Recently，we have investigated the efficient population transfer process between the vibrational levels υ=0 and 2 of the HF system［10］. It is found that the two-photon transfer process was diabatic，in which the vibrational levels υ=1 and 3 may be involved. Although at some high field peak amplitudes，part of the population can reside on the intermediate state υ=1，a high transition probability to υ=2，can still be achieved via the twophoton transition process at appropriate laser parameters.

Since the isotopic substitution not only changes the mass of the system but also shifts the energy position of rovibrational levels，many researchers focus their attention on the isotope effects on various aspects of molecular dynamics in recent years. For the spectra of stimulated emission in the process of the H2+F2→HF and D2+F2→DF reactions，Basov et al. found that the emission energy in the transitions of 2-1 and 5-4 bands in the H2+F2mixture was the highest possible. However，in the D2+F2mixture，the emission energy maxima corresponded to the transitions of 3-2 and 8-7 bands ［11］. For the reactive collisions of hydrogen molecular cations，Stroe and Fifirig investigated the isotope effects on the cross sections of the dissociative excitation （DE），dissociative recombination（DR），vibrational excitation and vibrational de-excitation of H2+，D2+，and T2+［12］. They found that the DE，DR cross sections of D2+were smaller than those of H2+when the colliding election energies were below the direct DE threshold. For collision reactions in gas phase，the shape resonance phenomenon for exchange reactions of X+HOX（X=H，D）varies［13，14］with the substitution of the H atom for D atom. For the photoassociation reactions of X+F→XF（X=H，D），the isotope effect has been connected with the Frank-Condon overlap integral［15］. However，to the best of our knowledge，the isotope effects on the population transfer process via two-photon resonance excitation have not been well studied.

In this work，to study the isotope effects on the twophoton population transfer process，we consider the DF and HF molecular systems，and set the vibrational levels υ=0 to 2 of the ground electronic state to be the initial and target states for each system. The transition process between these two states is of importance because it is an overtone transition under the condition of one-photon resonance［16］. We investigate the influences of the field peak amplitude and pulse duration on the two-photon population transfer processes，and present detailed comparisons between the two systems. Because the vibrational energy difference for the DF molecule is smaller than that for the HF molecule，we expect that the optimized pulse duration for the DF molecule would be longer than that for the HF molecule. We also expect that the two-photon transition process of the DF system is diabatic，similarly to the HF system ［10］. Thus，the numerical calculations based on timedependent wavepacket method，which includes the influences of all vibrational levels，are performed to verify these expectations and to obtain detailed dynamics information. Moreover，in the calculations，we consider very large variation ranges for the laser intensity and the pulse duration，which might be instructive for relevant experimental research.

II. THEORETICAL CALCULATION

In the Born-Oppenheimer approximation，the timedependent Schr¨odinger equation（TDSE），describing the interaction of the diatomic molecule with a linearly polarized laser field，can be written as（where atomic units are used throughout unless indicated otherwise）：

The Hamiltonian can be expressed as

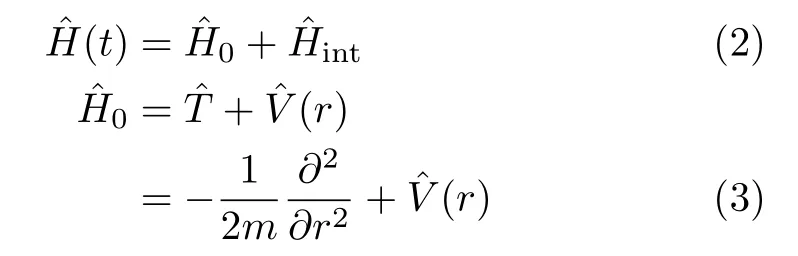
In this study，we neglected the influence of the rotational motion of the molecule. This treatment is raised from two considerations：（i）we focus on the vibrationalstate-selected population transfer process，（ii）the pulse duration we considered in this work is much shorter than the rotational periods of the two molecules. For the effect of the molecular rotation on the excitation process，one can refer to Ref.［17］.

The potential energy function，（r），is given by the Morse function：

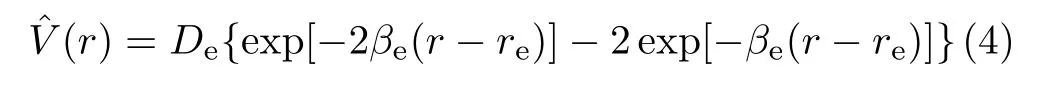
where the well depth of the HF molecule potential is De=0.225009 EH，the Morse argument βe=1.174014 a-10，the equilibrium internuclear distance re=1.732516 a0（EHis the Hartree energy and a0is the Bohr radius）. The interaction HamiltonianˆHintis given by

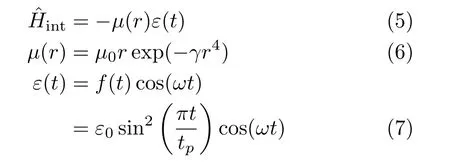
whereµ（r）and ε（t）are the dipole moment and the electric field，respectively，µ0=0.454141e（e is the electron charge）and γ=0.0064 Bohr-4. The relevant parameters used in Eq.（4）and Eq.（6）for the potential energy and dipole moment functions are taken from Ref.［18］. In Eq.（7），f（t）denotes the envelope of the electric-field，and ε0，tp，and ω are the field peak amplitude，pulse duration，and central frequency，respectively.

At the initial time，t=0，the diatomic molecule is assumed to be in the ground vibrational（υ=0）state. The initial wave function is obtained by using the Fourier grid Hamiltonian method［19］. Starting from this initial wave function，the time-dependent wave function is calculated by using the split-operator method［20-22］.

To perform the calculation for Eq.（8），the wave function is transformed between the momentum and coordinate grid representations to interact with the kinetic energy and potential energy operators，respectively. The fast Fourier transform（FFT）［23-26］method is used to accomplish the transformation between the two representations. In the calculation，120000 uniform time grids in the region of［0，2000］fs and 256 uniform space grids in the region of［0.05，20］Bohr are used. The numerical results have been checked to be converged，by using the smaller time grid size，longer time range，the smaller space grid size，and larger space range，respectively.

TABLE I　Comparisons between the one photon energy and the energy differences of the neighboring vibrational levels for the two molecular systems. The energies are shown in a.u.

The time-dependent population on a specific vibrational eigenstate |v〉is given by

III. RESULTS AND DISCUSSION

The reduced masses of the DF and HF molecules are 3319.561 and 1744.632 a.u.，respectively. In the calculation，the vibrational levels υ=0 and 2 of the ground electronic state are taken to be the initial and target states，respectively，for both the DF and HF molecules. The relevant vibrational levels and transition processes are schematically illustrated in Fig.1. The central frequency of the laser pulse used for the DF（HF）molecule is set to be 0.013062（0.017674）a.u.，which is half of the energy difference between the vibrational levels υ=0 and 2.

The variation of the final population versus the field peak amplitude and pulse duration is shown in Fig.2. The field peak amplitude varies from 0.001 a.u. to 0.06 a.u. with an interval of 0.0005 a.u. and pulse duration varies from 10 fs to 2000 fs with an interval of 10 fs. The variations of the final population on the vibrational levels υ=0，2，and 1 of the DF molecule are shown in Fig.2（a）-（c），respectively，and the corresponding ones for the HF molecule are shown in Fig.2（d）-（f），respectively. As seen in Fig.2（b）and（e），the final population on the target vibrational state υ=2 shows an oscillatory behavior dependent on the field peak amplitude and pulse duration for both the DF and HF systems. Comparing Fig.2（b）with（e），we found that the required pulse duration is longer than 860 fs for the DF molecule to achieve a relatively high transition probability（more than 80%），while that is only longer than 460 fs for the HF molecule. We found that there are many“bright stripe”regions in Fig.2（c）and Fig.2（f）. This indicates that part of the population may reside on the level υ=1 for the two systems during the transition process from level υ=0 to 2. Obviously，compared to the HF system，in the population transfer process from the initial state υ=0 to the target state υ=2，the population of the DF system may more easily reside on the intermediate state υ=1. We can understand this by comparing the energy differences，Eυ=1-Eυ=0-ħω，for the two molecular systems. As seen in Table I，the photon energy we used for either system is near-resonant to the transition energy between υ=0 and υ=1，and hence the population may reside on the level 1 during the two-photon absorption from υ=0 to υ=2. Moreover，the value，Eυ=1-Eυ=0-ħω for the DF system is quite smaller than the one for the HF system，and this is consistent with the findings that the population may more easily reside on υ=1 for the DF system.

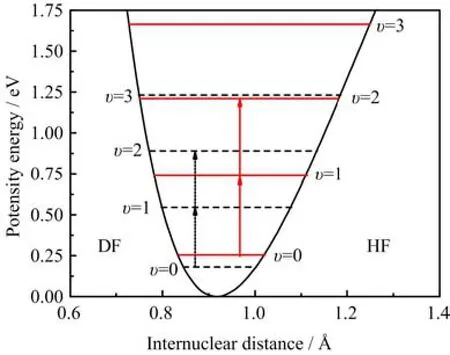
FIG. 1　The two-photon population transfer between the two vibrational levels υ=0 and 2 of the DF（dashed line）and HF （solid line）molecules.

In order to investigate the effects of the field peak amplitude on the final population in the two-photon population transfer for the DF and HF systems，we set the pulse duration at 1280 fs，corresponding to the vertical dashed line marked in Fig.2. The variations of the final population on all relevant levels as a function of the field peak amplitude are shown in Fig.3（a）for the DF molecule and Fig.3（b）for the HF molecule，respectively. In our considered range of field peak amplitude，it shows five peaks of the final population on the target state υ=2 for the DF molecule in Fig.3（a）. However，it shows six peaks for the HF molecule in Fig.3（b）. This phenomenon can be explained by the two-photon Rabi oscillations［27-30］. Rabi oscillation directly connects with the coupling of the relevant quantum states，and it can occur in both bound-bound transitions and the continuum-continuum interactions［28］. Many important phenomena are related with Rabi oscillation，such as the the Autler-Townes Splitting［29］and the interference structure in the photoelectron spectra［30］，etc. The stronger the coupling is，the more frequent the Rabi oscillation is. We calculate the constant coefficient of the two-photon Rabi frequency for the two systems. The constant coefficient of the DF system，0.00106 a.u.，is weaker than that of the HF system，0.00126 a.u. Consequently，the oscillation period of the final population with the field peak amplitude for the DF molecule isrelatively larger than the HF molecule. Thus，we can see that the coupling intensity between the υ=0 and υ=2 levels for the DF system is weaker than that for the HF system.

FIG. 2　The variation of the final populations on the initial level υ=0，the target level υ=2，and the intermediate level υ=1，for the DF（a-c）and HF（d-f）molecules versus the field peak amplitude and pulse duration. The central frequencies of laser pulse for the DF and HF systems are fixed at 0.013062 and 0.017674 a.u.，respectively.

FIG. 3　By fixing the pulse duration at 1280 fs，the variations of the final population on the all relevant levels as a function of the field peak amplitude are shown for（a）DF and（b）HF molecules，respectively.

As mentioned above，the intermediate state υ=1 can be involved for both systems during the two-photonresonant transition. As seen in Fig.3，the population residing on the level υ=1，P1，presents several peaks at certain field peak amplitudes. For both systems，the peak values for P1increase with the increase of the field peak amplitude. For the DF molecule，the peak values for P1increase from 5.4%to 32%，while for the HF molecule，the peak values for P1increase from 0.9% to 13.6%. Thus，in our considered range of field peak amplitude from 0.001 a.u. to 0.06 a.u.，the probability for the population residing on the level υ=1 can be better suppressed for the HF molecule than for the DF molecule.

Moreover，it can be seen from Fig.3 that the level υ=3 may also be involved for the DF system when the field peak amplitude is in the relatively strong range，roughly from 0.04 a.u. to 0.06 a.u. The peak probability for the population residing on level υ=3 is roughly 2.6%for the DF system. However，we do not find any population residing on υ=3 for the HF system in the same range of field amplitude.

FIG. 4　The variations of the final population on the vibrational levels υ=0，1，2，and 3 as a function of the pulse duration are shown for（a）DF and（b）HF molecules，respectively. The field peak amplitude is fixed at 0.0175 a.u.

FIG. 5　The population transfer processes of（a）DF and（b）HF molecules. The field peak amplitude and pulse duration are fixed at 0.0175 a.u. and 1280 fs，respectively.

We now investigate the variations of the final population on all relevant levels with the pulse duration for the two systems，by fixing the field peak amplitude at 0.0175 a.u.，corresponding to the horizontal dashed line in Fig.2. As shown in Fig.4，the population may reside on levels υ=1 and 3 in certain regions of pulse duration for both the two systems and the peak probability for the population residing on the level υ=1 decreases with the increase of the pulse duration. The peak probability for the population residing on the intermediate level υ=1 for the DF system decreases from 53%to 14% with the increase of the pulse duration from 400 fs to 2000 fs，while that for the HF system decreases from 32.8%to nearly 0%. The level υ=3 is involved for the DF molecule when the pulse duration is shorter than 600 fs，however，υ=3 is not involved for the HF molecule until the pulse duration is shorter than 400 fs. The maximal probability for the population residing on the level υ=3 of the DF molecule is roughly 28%and that of the HF molecule is roughly 4.7%. Thus，considering the population variation with the pulse duration，we can draw the same conclusion that it is more possible for the population to reside the intermediate level υ=1 and higher level υ=3 for the DF system，compared to the HF system.

By setting the field peak amplitude and pulse duration as 0.0175 a.u. and 1280 fs，respectively，we investigate the time-dependent population on all relevant levels during the two-photon transfer process. With this set of parameters，the almost complete population transfer can be achieved for both systems. The population transfer processes of the DF and HF molecules are shown in Fig.5（a）and（b），respectively. Although nearly complete population transfer are achieved with this set of laser parameters，the population transfer processes are nonadiabatic，because the levels υ=1 and 3 are involved in the process for both systems. The maximal temporary probabilities for the population excited to the levels υ=1 and 3 for the HF molecule are roughly 30%and 15.5%，respectively，while those for the DF molecule are roughly 56%and 26%，respectively. Thus，the intermediate level υ=1 and higher level υ=3 of the DF molecule may play more important roles in the two-photon resonance process than those of the HF molecule.

The difference，between the two systems，in the twophoton-resonant transition process can be understood by comparing the photon energy with the energy difference of the neighboring vibrational levels. As seen in Table I，for the DF system，the photon energy is more near-resonant with the neighboring vibrational levels，and hence，the one-photon transitions，such as，υ=0←→υ=1，υ=1←→υ=2，υ=2←→υ=3，may more possibly occur. This is because that compared to thecase of the HF molecule，the vibrational levels in the DF molecule are displaced more evenly and closely.

IV. CONCLUSION

The isotope effects on the two-photon-resonant population transition process are investigated for the DF and HF molecular systems. The vibrational levels υ=0 and 2 of the ground electronic state are taken to be the initial and target states. By solving the timedependent Schr¨odinger equation with quantum wave packet method，we discuss the influences of the field peak amplitude and pulse duration on the two-photonresonant population transfer process in detail as well as the roles of the intermediate level υ=1 and the higher level υ=3. The population residing on these two levels may increase with the increase of the field peak amplitude，and with the decrease of the pulse duration. By comparing the two molecular system，we find that it is more possible for the population to reside on υ=1 and υ=3 for the DF molecule，because the vibrational levels in the DF molecule are displaced more evenly and closely than the corresponding ones in the HF molecule.

V. ACKNOWLEDGMENTS

This work is supported by the Specialized Research Fund for the Doctoral Program of Higher Education （No.20130041120053），Scientific Research Foundation for Returned Overseas Chinese Scholars，State Education Ministry，the Science and Technology Research Funds of the Department of Education of Liaoning Province（No.L2013014），Liaoning Bai-qian Wan Talents Program，the National Magnetic Confinement Fusion Science Program（No.2013GB109005），the Fundamental Research Funds for the Central Universities （No.DUT15YQ105），and the National Natural Science Foundation of China（No.21473018，No.11274056，and No.11347012）.

［1］A. D. Bandrauk，Front. Chem. Dyn. 470，131（1995）.

［2］P. Dittmann，F. P. Pesl，J. Martin，G. W. Coulston，G. Z. He，and K. Bergmann，J. Chem. Phys. 97，9472 （1992）.

［3］J. L. Rinnenthal and K. H. Gericke，J. Chem. Phys. 111，9465（1999）.

［4］K. Bergmann，H. Theuer，and B. W. Shore，Rev. Mod. Phys. 70，1003（1998）.

［5］V. Blanchet，C. Nicole，M. A. Bouchene，and B. Girard，Phys. Rev. Lett. 78，2716（1997）.

［6］Y. B. Band and O. Magnes，Phys. Rev. A 50，584 （1994）.

［7］G. N. Gibson，Phys. Rev. A 72，041404（2005）.

［8］H. Maeda，J. H. Gurian，D. V. L. Norum，and T. F. Gallagher，Phys. Rev. Lett. 96，073002（2006）.

［9］G. Demeter and G. P. Djotyan，J. Opt. Soc. Am. B 26，867（2009）.

［10］B. B. Wang，Y. H. Pang，Y. C. Han，and S. L. Cong，J. Theor. Comput. Chem. 13，1450061（2014）.

［11］N. G. Basov，V. T. Galochkin，V. I. Igoshin，L. V. Kulakov，E. P. Martin，A. I. Nikitin，and A. N. Oraevsky，Appl. Opt. 10，1814（1971）.

［12］M. Stroe and M. Fifirig，J. Phys. B 42，205203（2009）.

［13］B. Fu，Y. Zhou，and D. H. Zhang，Chem. Sci. 3，270 （2012）.

［14］B. Fu and D. H. Zhang，J. Chem. Phys. 136，194301 （2012）.

［15］P. Shen，Y. C. Han，J. L. Li，K. Yi，C. Chen，and S. L. Cong，Laser Phys. Lett. 12，045302（2015）.

［16］I. V. Andrianov and G. K. Paramonov，Phys. Rev. A 59，2134（1999）.

［17］K. J. Yuan，Z. Sun，S. L. Cong，and N. Lou，Phys. Rev. A 74，043421（2006）.

［18］M. Kaluˇza，J. T. Muckerman，P. Gross，and H. Rabitz，J. Chem. Phys. 100，4211（1994）.

［19］C. C. Marston and G. G. BalintKurti，J. Chem. Phys. 91，3571（1989）.

［20］M. D. Feit，J. A. Fleck Jr.，and A. Steiger，J. Comput. Phys. 47，412（1982）.

［21］M. D. Feit and J. A. Fleck Jr.，J. Chem. Phys. 78，301 （1983）.

［22］M. D. Feit and J. A. Fleck Jr.，J. Chem. Phys. 80，2578 （1984）.

［23］W. H. Press，S. A. Teukolsky，W. T. Vetterling，and B. P. Flannery，Numerical Recipes，3rd Edn.，Cambridge：Cambridge University，600（1986）.

［24］J. W. Cooley and J. W. Tukey，Math. Comput. 19，297 （1965）.

［25］C. Temperton，J. Comput. Phys. 52，1（1983）.

［26］H. J. Nussbaumer，Fast Fourier Transform and Convolution Algorithms，2nd Edn.，Berlin：Springer-Verlag，80（1982）.

［27］A. F. Linskens，I. Holleman，N. Dam，and J. Reuss，Phys. Rev. A 54，4854（1996）.

［28］S. Magnier，M. Persico，and N. Rahman，Phys. Rev. Lett. 83，2159（1999）.

［29］Z. Sun and N. Lou，Phys. Rev. Lett. 91，023002（2003）.

［30］C. Meier and V. Engel，Phys. Rev. Lett. 73，3207 （1994）.

DOI：10.1063/1674-0068/29/cjcp1509186