Experimental study on the stability of plate anchors in clay under cyclic loading

Long Yu∗，Qi Zhou，Jun Liu

State Key Laboratory of Coastal and Offshore Engineering，Dalian University of Technology，Dalian 116024，China

Experimental study on the stability of plate anchors in clay under cyclic loading

Long Yu∗，Qi Zhou，Jun Liu

State Key Laboratory of Coastal and Offshore Engineering，Dalian University of Technology，Dalian 116024，China

A R T I C L E I N F O

Article history:

Received 23 October 2014

Accepted 29 December 2014

Available online 25 February 2015

Plate anchor

Clay

Cyclic loading

Strain softening

Although the bearing capacity ofplate anchors in clay has been studied extensively，the results considering the effects of offshore cyclic loading are relatively rare.In the present study，1 g model tests are carried out to investigate the effect of cyclic loading on the bearing capacity of plate anchors in clay.The ultimate pulloutcapacity ofplate anchors in clay decreases as the accumulated plastic shearstrain grows due to the strain-softening of clay under cyclic loading.The load-displacement curves of these tests are presented and the effects of overburden stress and cyclic loading amplitude on the strain-softening behavior are discussed.

©2015 The Authors.Published by Elsevier Ltd on behalf of The Chinese Society of Theoretical and Applied Mechanics.This is an open access article under the CC BY-NC-ND license（http:// creativecommons.org/licenses/by-nc-nd/4.0/）.

Plate anchors have been commonly used to provide pullout resistance for many engineering structures，such as transmission towers and earth retaining walls.Providing mooring system of offshore floating facilities with a simple and economical foundation，plate anchors have been increasingly used in offshore oil/gas exploration［1］.

During the past decades，considerable efforts have been made to estimate the pullout capacity of plate anchors under monotonic conditions，which includes model test studies of Das and co-workers［2-5］and Meyerhof and Adams［6］，numerical studies of Rowe and Davis［7］，Merifield and co-workers［1，8，9］，and Yu et al.［10］.However，these studies are limited to static analysis and the effects ofcyclic loading on the pulloutcapacity ofplate anchors are not very clear.

In order to take into account the combination ofstatic and cyclic loads in evaluation of the bearing capacity of foundations，Andersen et al.［11，12］carried out numerous direct simple shear（DSS）tests and triaxial tests to simulate the simplified stress conditions in the soil beneath the platform for a few typical elements along a potential failure surface.Thus the relationship between cyclic shear strength and number of cycles，average shear stress，overconsolidation ratio，cyclic shear stress was established，which can be used to determine the critical failure surface.Similarly，Wang et al.［13］took the octahedral shear stress as the failure criteria of soil elements，simplifying the effect of cyclic loading as the softening of equivalent cyclic strength to evaluate the bearing capacity of foundations.Zhou and Randolph［14，15］provided numerical investigations into soil characteristics，and presented an equation to reflect the effect of strain rate and strain softening on the value of undrained shear strength.

Datta et al.［16］carried out experimental model tests to study the effects ofmean load and the cyclic amplitude on the permanent anchor movement and post-cyclic static pullout capacity.Singh and Ramaswamy［17，18］studied the effects ofcyclic frequency and pre-loading on the behavior of circular plate anchors in clay.All these studies are in the scale of model test，i.e.，the stress level is obviously lower than that in the field.

Thispaperpresents the modeltestresults ofone low stress level test and two high stress level tests on plate anchors in overconsolidated kaolin clay.The strain-softening behavior of plate anchors in clay owing to disturbance of the cyclic loading was focused on.

The model tests were carried out in kaolin clay consolidated from slurry in a tank of 800 mm in height，700 mm in length，and 220 mm in width.The schematic layout of the consolidation setup is shown in Fig.1（a）.The end of lever can be adjusted manually to different height according to the settlement of the soil specimen to keep approximately horizontal.

The experimental set-up of the cyclic test is shown in Fig.1（b）. The model plate anchor（made of steel，210 mm long，50 mm wide，and 20 mm thick）is pre-embedded in the chamber.The length of the anchor is designed to exactly fit the width of the chamber，but with very slight gaps to avoid being stuck by the walls during loading.The overburden pressure was added to the soil surface by an air bag.

Fig.1.Schematic layout of the experimental set-up.（a）Clay consolidation.（b）Cyclic loading test.

Notations A Cross section area of a T-bar or an anchor B Anchor width d Displacement of a T-bar or an anchor F Soil resistance force H Embedment depth of an anchor N Cycle number NcBearing capacity factor of a T-bar or an anchor Q Surcharge pressure on soil surface suThe undrained strength of clay su0The undrained shear strength of clay before remoulding γUnit weight of clay δremThe residual shear strength of fully remolded soil ξ，ξ95Present accumulated strain and that of 95%remolded soil σvThe overburden pressure at the depth of plate anchor υPoisson’s ratio

Hebei kaolin clay was used in this study.The liquid and plastic limits are 58%and 27%respectively.Homogeneous and deaired slurry at a moisture of 116%（two times the liquid limit），was prepared in a ribbon-blade mixer equipped with a vacuum pump. After mixed for at least 5 h，the slurry was pumped into the consolidation tank.The consolidation pressure was increased by 3 kPa in each step.The consolidation after each load increment is assumed to finish when the settlement of the sample is lower than 1.0 mm during 24 h.The total time required for consolidation，which depends on the final consolidation pressure，was about 25 days.It should also be noted that the model plate anchor was manually placed into the slurry after a certain period of consolidation when the clay was soft enough to let the anchor in but strong enough to avoid further settlementdue to the self-weightof the plate anchor.

A T-bar penetrometer of diameter 5 mm and length 20 mm and a plate-bar penetrometer of diameter 12 mm were used to determine the shear strength profile of the kaolin clay before the loading of anchor.These tests were all conducted at a rate of 0.2 mm/s which equals to the loading rate of the plate anchor in Tests 2 and 3.It is assumed that the loading rate is low enough to get rid of the dynamic influence.Note that there is a limitation of the travel range of the loading hydro-cylinder.A total displacement larger than 20 cm was achieved by:（1）apply a displacement of 20 cm or less then stop；（2）disconnect the rod and withdraw the hydrocylinder；（3）extend the length of the rod and re-connect it to the hydro-cylinder；（4）apply a required displacement again.

Fig.2.Result of the T-bar test in Test 1.

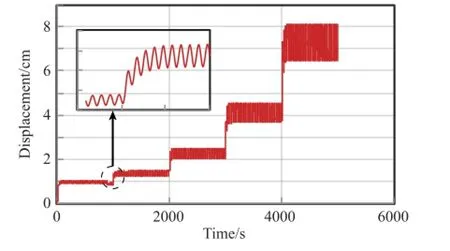
Fig.3.Displacement history of plate anchor in Test 1.

A T-bar test was carried out before the model test to investigate the clay strength in Test1.The T-bar was penetrated monotonically to the depth of 428 mm，and then was moved up and down with amplitude of 84 mm for 5 cycles.The T-bar test result shows that the average shear strength of the clay around the anchor position in Test1 is about25 kPa，as shown in Fig.2.The value ofthe bearing capacity factor of the T-bar was selected as Nc=10.5［19］.

Due to the low overburden pressure，a cavity is formed behind the T-bar during penetrating because of the high strength ratio su/σv，where suis the undrain strength of clay andσvis the overburden pressure at the depth of plate anchor.It is the reason that the resistance of the T-bar under cyclic loading is very small.

For the low stress level case，the anchor was subjected to a displacement load comprised offive sinusoidalcurves with various average values and various amplitudes.Specifically，the average displacement and the amplitude were both increased by about 2 times after each loading period of 1000 s，as shown in Fig.3.The loading frequency is 0.1 Hz in the whole loading process of Test 1.The resistance-displacement curve of the plate anchor is shown in Fig.4.The softening of the resistance is shown in Fig.5.It can be seen that the residual bearing capacity after several cycles became quite small.This is because that a cavity was formed behind the anchor during penetrating or lifting and cracks were observed along the shear bonds.

According to the numerical results［10］，the fully flow back mechanism can be mobilized if the overburden pressure is large enough（σv＞8su）.The value of the surcharge pressure in Test 2 is q=270 kPa（thusσv=q+γH≈19su）.A series of T-bar penetrometer tests were conducted before the cycling of the plate anchor in Test 2.The average shear strength of the clay around the anchor embedment depth is approximately 15 kPa.

In Test 2，the anchor was uplifted for 48 mm and then pushed back to the initial position，and repeated 9 times.The resistance response of the plate anchor in Test 2 is shown in Fig.6.It can be seen that the result of Test 2 shows much more obvious hysteresis behavior by comparison with that of Test 1.

The softening behavior of the resistance response in Test 2 is shown in Fig.7.The maximum resistance in the second cycle is 88% of the resistance at the end of the lifting in the first cycle.In the last cycle，the maximum resistance is 72%of the maximum resistance in the first cycle.Note that in Test 1（low stress level）the maximum resistance in the last cycle is only 15%-30%of the maximum resistance in the first cycle.

A numerical simulation of the result of Test 2 is carried out using the strain-softening model of FLAC.The softening behavior of the clay is governed by Eq.（1）［14，15］，whereδremis the residual shear strength offully remolded soil，ξ/ξ95is the ratio between the present accumulated strain and that of95%remolded soil，su0is the strength before any remolding.Only the first two loading cycles are simulated.The minimum element size of the mapped mesh is 0.125 min width.The width ofplate anchor is 4 m.The embedment depth of the anchor is 40 m.The clay density is 1000 kg/m3and the cohesion is 25 kPa.ThusγH/su0=16，making sure that the full localized failure mechanism would occur.

Fig.4.Load-displacement curve of the anchor in Test 1.

Fig.5.Maximum bearing capacity factors versus cyclic number in each loading stage in Test 1.

Fig.6.Load-displacement curves of Test 2.

Fig.7.Maximum bearing capacity factors versus cyclic number in each loading stage in Test 2.

Fig.8.Comparison between the model test result and the numerical result for Test 2.

The comparison of the model test result and the numerical results is demonstrated in Fig.8 and Table 1.Variousvalues ofξ95and δremhave been tried.The strain-softening of plate anchor of model Test 2 agrees well with the numerical curves as the ultimate capacity of the plate anchor is continually softening during the first two uplifting stages.It can be concluded that the strain-softening model in FLAC can approximately reflect the strain-softening behavior of the clay，by properly selecting the parameters ofξ95and δrem.It confirms that fully localized soil flow mechanism was mobilized in Test 2.

In Test3，the cyclic loading amplitude increases from0.25 cmto 2 cm，as shown in Fig.9.The T-bar test shows that the undrained shear strength of the soil at the plate anchor position in Test 3 is about 10 kPa.

The load-displacement response in Test 3 is shown in Fig.10. When the load amplitude is small，the load-displacementresponseshows an elastic character.With the increase ofload amplitude，the result shows more and more hysteresis character.It can be seen that the response goes into fully plastic failure at a displacement of around d/B=0.2，after where the resistance keeps stable.

1 g model tests were conducted to investigate the strain softening behavior of the bearing capacity of plate anchors in clay under cyclic loading.Numericalanalyses were also conducted using FLAC to quantify the strain softening parameters.Conclusions based on these results can be made below.

（1）For the low stress level case，a cavity is formed beneath the anchor after a certain pullout displacement in the first cycle.As a result，the residual bearing capacity after several cycles is quite small.

（2）For the high stress levelcases，the bearing capacity decreases as the accumulated plastic shear strain grows due to the strainsoftening behaviorofthe clay.Because ofthe fully flow back mechanism，the resistance of the anchor under cyclic loading shows obvious hysteresis，and the absolute value of maximum uplift resistance is similar to that of the maximum push-down resistance. Residual bearing capacity of the plate anchor was over 70%of the maximum pullout resistance during the first uplift loading.

（3）Loading amplitude has great effect on the shape of load-displacement curve.More hysteresis character can be mobilized when increasing the loading amplitude.

This work was supported by the National Natural Science Foundation of China（51121005 and 51209033），the Natural Science Foundation of Liaoning Province，China（2013020154），and the Fundamental Research Funds for the Central Universities of China（DUT14ZD206）.

Table 1Comparison between numerical and test results for Test 2.

Fig.9.Displacement history of Test 3.

Fig.10.Load-displacement response in Test 3.

［1］R.S.Merifield，S.W.Sloan，H.S.Yu，Stability of plate anchors in undrained clay，Geotechnique 51（2001）141-153.

［2］B.M.Das，A procedure for estimation of ultimate capacity of foundations in clay，Soils Found.20（1980）77-82.

［3］B.M.Das，R.Moreno，K.F.Dallo，Ultimate pullout capacity of shallow vertical anchors in clay，Soils Found.25（1985）148-152.

［4］B.M.Das，V.K.Puri，Holding capacity of inclined square plate anchors in clay，Soils Found.29（1989）138-144.

［5］B.M.Das，A.J.Tarquin，R.Moreno，Model tests for pullout resistance of vertical anchors in clay，Civ.Eng.Pract.Des.Eng.4（1985）191-209.

［6］G.G.Meyerhof，J.I.Adams，The ultimate uplift capacity of foundations，Can. Geotech.J.5（1968）225-244.

［7］R.K.Rowe，E.H.Davis，The behaviour of anchor plates in clay，Geotechnique 32（1982）9-23.

［8］R.S.Merifield，A.V.Lyamin，S.W.Sloan，Stability of inclined strip anchors in purely cohesive soil，J.Geotech.Geoenviron.Eng.131（2005）792-799.

［9］R.S.Merifield，S.W.Sloan，A.V.Lyamin，The stability of inclined plate anchors in purely cohesive soil，Fac.Eng.Surv.，Report TR-2003-03（2003）.

［10］L.Yu，J.Liu，X.Kong，Y.Hu，Numerical study on plate anchor stability in clay，Geotechnique 61（2011）235-246.

［11］K.H.Andersen，R.Lauritzsen，Bearing capacity for foundations with cyclic loads，J.Geotechnic.Eng.114（1988）540-555.

［12］K.H.Andersen，J.H.Pool，S.F.Brown，Cyclic and static laboratory tests on drammen clay，Am.Soc.Civ.Eng.J.Geotech.Eng.Div.106（1980）499-529.

［13］J.Wang，Y.Liu，Y.Xing，H.Di，Estimation of undrained bearing capacity for offshore soft foundations with cyclic load，China Ocean Eng.12（1998）213-222.

［14］H.Zhou，M.F.Randolph，Computational techniques and shear band development for cylindrical and spherical penetrometers in strain-softening clay，Int. J.Geomech.7（2007）287-295.

［15］H.Zhou，M.F.Randolph，Resistance of full-flow penetrometers in ratedependent and strain-softening clay，Geotechnique 59（2009）79-86.

［16］M.Datta，S.K.Gulhati，G.Achari，Behaviour of plate anchors in soft cohesive soils under cyclic loading，Indian Geotech.J.20（1990）206-224.

［17］S.P.Singh，S.V.Ramaswamy，Influence of frequency on the behaviour of plate anchors subjected to cyclic loading，Mar.Geores.Geotech.26（2008）36-50.

［18］S.P.Singh，S.V.Ramaswamy，Effects of cyclic frequency and pre-loading on behaviour of plate anchors，in:Geotechnical Special Publication，vol.252-260，American Society of Civil Engineers，Shanghai，China，2010.

［19］M.S.Hossain，Y.Hu，M.F.Randolph，D.J.White，Limiting cavity depth for spudcan foundations penetrating clay，Geotechnique 55（2005）679-690.

∗Corresponding author.

E-mail addresses:longyu@dlut.edu.cn（L.Yu），15998641859@163.com（Q.Zhou），junliu@dlut.edu.cn（J.Liu）.

*This article belongs to the Solid Mechanics