利用Adomain分解法求时间分数阶薛定谔方程的近似解

默会霞,余东艳,隋鑫

(北京邮电大学理学院,北京100876)

利用Adomain分解法求时间分数阶薛定谔方程的近似解

默会霞,余东艳,隋鑫

(北京邮电大学理学院,北京100876)

非线性薛定谔方程是现代科学中非常普遍的非线性模型之一.通过Adomain分解,得到了(2+1)维和(3+1)维非零势阱时间分数阶薛定谔方程的近似解.利用Adomain分解不用像相关文献中那样将解函数的实部和虚部分别去求解,从而简化了求解过程.

薛定谔方程;Adomain分解法;分数阶导数;分数阶积分

1 引言

分数阶微积分产生于流体力学、生物学、物理学等领域.其广泛的应用引起了数学界、工程界及其它很多领域专家学者的关注.特别地,分数阶微分方程可用来描述流体力学、生物学、物理学等领域的一些自然现象.因此对分数阶微分方程求解的研究非常重要[1-5].

非线性薛定谔方程是现代科学中非常普遍的非线性模型之一.在玻色-爱因斯坦凝聚体、等离子物理、非线性光学、流体动力学等领域中有着重要应用.关于非线性方程求解的方法有很多,例如:Adomain分解法[6-7]、变分迭代法[8]、同伦分析法[9]、同伦摄动法[10]等.

文献[1]中,Khan用同伦分析法求得了分数阶势阱和非势阱薛定谔方程的近似解.但此法要将薛定谔方程中的解函数的实部和虚部分开,分别去求解,比较复杂.受文献[11]的启发,将利用Adomain分解法,研究文献[1]中的(2+1)维和(3+1)维非零势阱时间分数阶薛定谔方程的近似解.本文不必将解函数的实部和虚部分开,直接利用Adomain分解法迭代计算就可得到其近似解,简化了运算,且其近似解与文献[1]中的完全一致.

其中

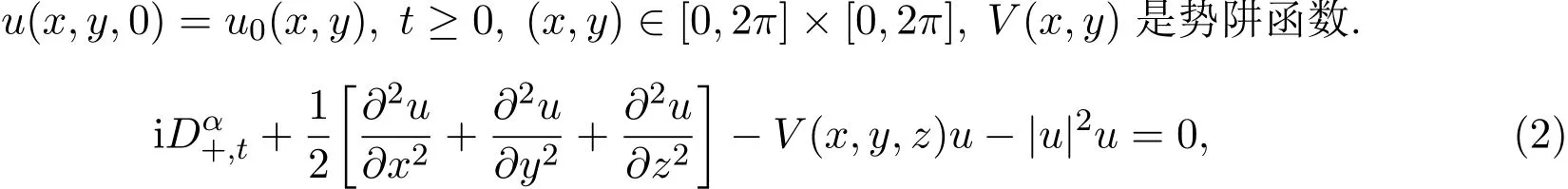
其中u(x,y,z,0)=u0(x,y,z),t≥0,(x,y,z)∈[0,2π]×[0,2π]×[0,2π],V(x,y,z)是势阱函数.

2 基本定义及引理

定义2.1[12]设f∈L1[0,+∞],α>0,则α阶的黎曼-刘维尔分数阶积分定义为:

定义2.2[12]设f∈L1[0,+∞],α>0,则R+上α阶的Caputo分数阶导数定义为:

其中n∈N,且n−1<α≤n,t>0.如果α=n是正整数,则此导数就是经典的n阶导数.

引理2.1[1,11]黎曼-刘维尔分数阶积分算子和Caputo分数阶导数具有以下性质:

其中α,β>0,t≥0,x∈R,m∈N满足m−1<α≤m.

3 Adomian分解法的基本理论

设n维方程可以写成下列形式

其中L是一个可逆线性算子,R是其余的线性部分,N代表一个非线性算子,u是一个变量为x1,x2,···,xn的n元函数.

现将方程(4)改写成:

因为L是可逆的,则

一般的,可以令L−1Lu=u−ϕ,其中ϕ满足条件Lϕ=0.

故方程(6)还可以写为:

对方程(7)进行参数化,则

设

其中Nu为

An即Adomain多项式.Adomain多项式可由下式给出:

将(9)和(10)式代入方程(8),并比较λ同次幂的系数,可以得出

利用给定的初值u0,u1,u2,···,都可以通过方程(12)得出,从而得到特解u.

4 时间分数阶薛定谔方程的近似解

本节用Adomian分解法,具体地求(2+1)维和(3+1)维时间分数阶薛定谔方程的近似解.

例4.1(2+1)维时间分数阶薛定谔方程:

其中t≥0,0<α≤1,u(x,y,0)=sinxsiny,(x,y)∈[0,2π]×[0,2π]且i2=−1.

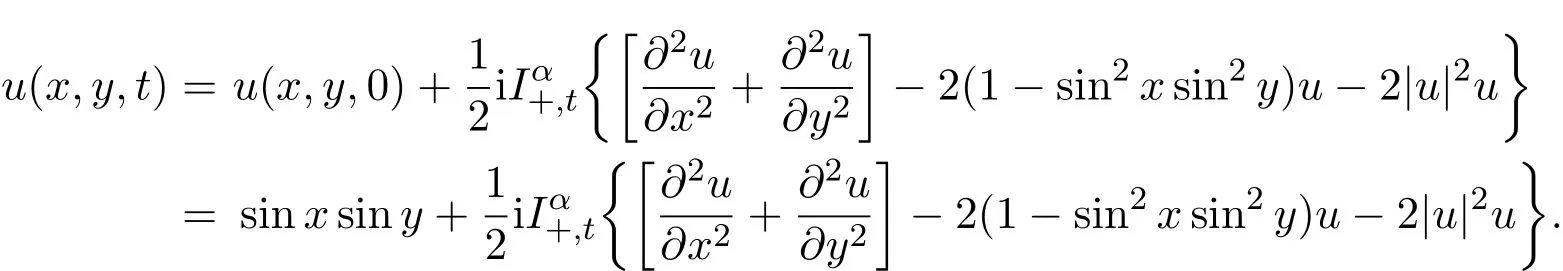
假设

则由(12)式,得到

取N=|u|2u,则由(11)式计算可得

通过对(14)和(15)式的计算,得到

其中系数cn取值如下:

因此,u(x,t)的4阶近似值是:

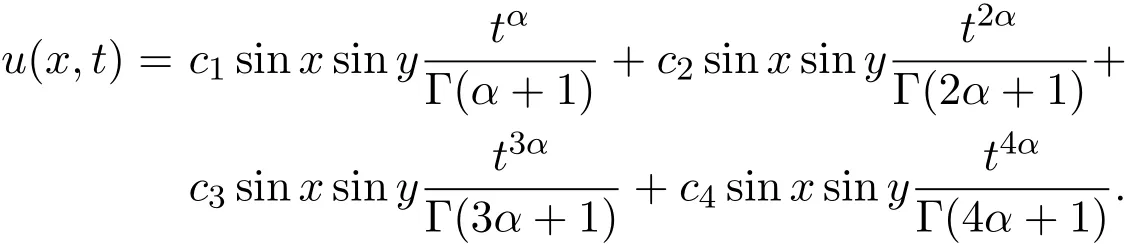
注意到,当α=1时,

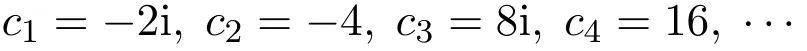
因此,当α=1时,可得方程(13)的精确解为:

此精确解与[5-8]的结果是一致的.

例4.2(3+1)维时间分数阶薛定谔方程:

其中t≥0,0<α≤1,u(x,y,z,0)=sinxsiny sinz,(x,y,z)∈[0,2π]×[0,2π]×[0,2π].

仿照例4.1的求解过程,可知

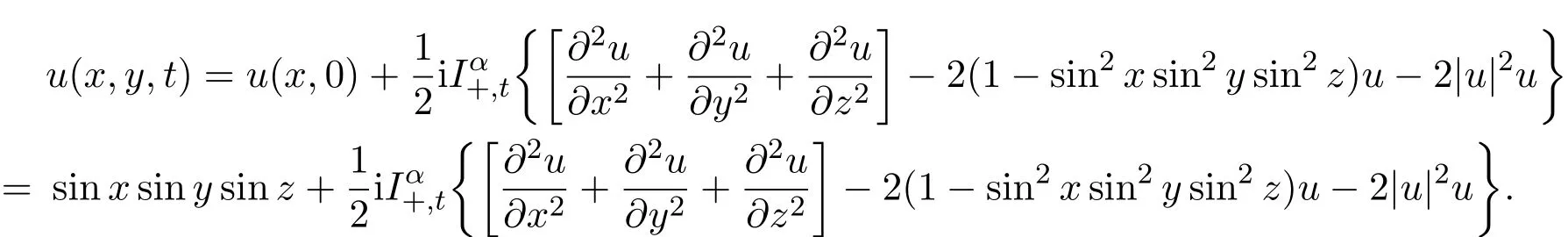
假设

利用(12)式,

取N=|u|2u,则由(11)式,可得到A0,A1,A2,A3,A4,···的值如(15)式.经过对(15)和(17)式的迭代计算,得到

其中cn是系数,其值为:

由此得到此方程的4阶近似解为:

注意到,当α=1时,

因此,当α=1时方程(16)的精确解为:

此解与文献[5-8]给出的解完全一致.

[1]Khan N A,Jamil M,Ara A.Approximate solutions to time-fractional Schr¨odinger equation via homotopy analysis method[J].International Scholarly Research Network ISRN Mathematical Physics,2012,Article ID 197068,11pages.

[2]Samko S G,Kilbas A A,Marichev O I.Fractional Integrals and Derivatives:Theory and Applications[M]. Yverdon:Gordon and Breach,1993.

[3]Hilfer R.Applications of Fractional Calculus in Physics[M].Singapore:World Scienti fi c,2000.

[4]Kilbas A A A,Srivastava H M,Trujillo J J.Theory and Applications of Fractional Di ff erential Equations[M]. Amsterdam:Elsevier Science Limited,2006.

[5]Sahadevan R,Bakkyaraj T.Invariant analysis of time fractional generalized Burgers and Korteweg-de Vries equations[J].Journal of Mathematical Analysis and Applications,2012,393:341-347.

[6]Khuri S.A new approach to the cubic Schr¨odinger equation:an application of the decomposition technique[J].Applied Mathematics and Computation,1998,97:251-254.

[7]Sadighi A,Ganji D D.Analytic treatment of linear and nonlinear Schro¨odinger equations:a study with homotopy-perturbation and Adomian decomposition methods[J].Physics Letters A,2008,372(4):465-469.

[8]Wazwaz A M.A Study on linear and nonlinear Schrodinger equations by the variational iteration method[J]. Chaos,Solitons&Fractals,2008,37(4):1136-1142.

[9]Liao S J.On the homotopy analysis method for nonlinear problems[J].Mathematics and Computation, 2004,147(2):499-513.

[10]He J H.The homotopy perturbation method for nonlinear oscillators with discontinuities[J].Applied Mathematics and Computation,2004,151(1):287-292.

[11]Herzallah M A E,Gepreel K A.Approximate solution to the time-space fractional cubic nonlinear Schr¨odinger equation[J].Applied Mathematical Modelling,2012,36:5678-5685.

[12]Podlubny I.Fractional Di ff erential Equation[M].London:Academic Press,1999.

Approximate solutions to the Schr¨odinger equation with time fractional derivatives via the Adomain decomposition method

Mo Huixia,Yu Dongyan,Sui Xin
(School of Science,Beijing University of Posts and Telecommunications,Beijing100876,China)

Nonlinear Schr¨odinger equation is a general nonlinear model in modern science.Using the Adomain decomposition method,we construct the approximate solutions to the(2+1)and(3+1)dimensional time fractional Sch¨ordinger equations with nonzero trapping potential.It is not necessary for us to decompose the solution function into real part and imaginary part as in relative references.So,the Adomain decomposition simpli fi es the procedure of solving the equation.

Schr¨odinger equation,Adomain decomposition method,fractional derivative,fractional integral

O29

A

1008-5513(2014)05-0460-07

10.3969/j.issn.1008-5513.2014.05.004

2014-03-12.

国家自然科学基金(11161042).

默会霞(1976-),副教授,研究方向:调和分析及其在偏微分方程中的应用.

2010 MSC:26A99