几何、调和平均组合的最佳广义对数平均界＊

2012-10-27钱伟茂

浙江师范大学学报（自然科学版） 2012年3期

关键词：展开式调和湖州

张帆，钱伟茂

(1.湖州职业技术学院，浙江湖州 313000;2.湖州广播电视大学，浙江湖州 313000)

几何、调和平均组合的最佳广义对数平均界＊

张帆1，钱伟茂2

(1.湖州职业技术学院，浙江湖州 313000;2.湖州广播电视大学，浙江湖州 313000)

应用初等微分学知识，对几何平均、调和平均的几何组合与广义对数平均进行了比较，解决了如下问题：对于 α∈(0，1)，使双向不等式 Lp(a，b)≤Gα(a，b)H1－α(a，b)≤Lq(a，b)对所有的 a，b ＞0 成立的最大 p 和最小q分别是多少?

不等式;广义对数平均;几何平均;调和平均

对固定的a，b＞0和a≠b，Lp(a，b)关于p∈R是连续和严格递增的.特别地，文献［1-9］从广义对数平均Lp(a，b)中发现了许多著名的不等式.广义对数平均甚至在经济学、物理学、气象学中也有应用［10-12］.若记2个正数a，b的算术平均、几何平均、指数平均、对数平均和调和平均分别为A(a，b)=则有著名的不等式

文献［13-15］得到了如下不等式：

对所有 a，b＞0，a≠b成立.

1986 年，Alzer证明了如下不等式［16］：

对所有 a，b＞0，a≠b成立.

下面2个结论由Alzer等［17］给出：

定理1［17］不等式

定理 2［17］设 a，b 是实数且 a≠b.若0 ＜a，b ＜e，则

若 a，b≥e，则

本文的目的是解决如下问题：对于α∈(0，1)，使双向不等式

对所有a，b＞0成立的最大p和最小q分别是多少?

1 引理

引理1当t＞1时，设f(t)则

证明由f(t)的定义得：

式(3)中：

由式(2)～式(6)和式(8)可知，当t＞1时，f(t)＞0.

由式(2)～式(6)和式(9)可知，当t＞1时，f(t)＜0.引理1证毕.

引理2当t＞1时，设h(t)=则

证明由h(t)的定义得：

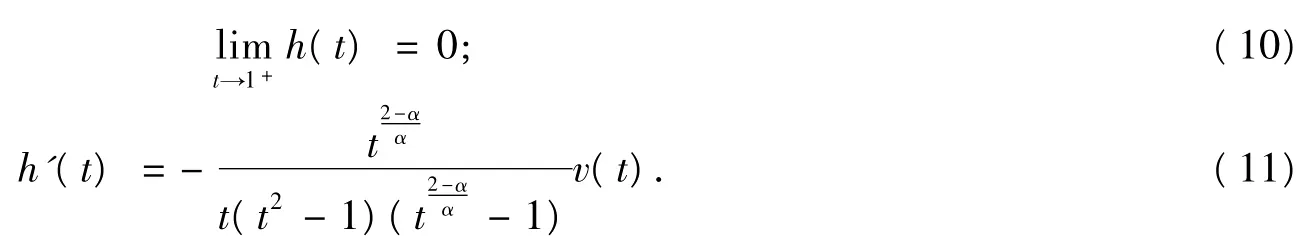
式(11)中：

由式(10)～式(13)及式(15)知，当t＞1时，h(t)＜0.

由式(10)～式(13)及式(16)知，当t＞1时，h(t)＞0.

2 主要结果

定理3若 α∈(0，1)，则对所有 a，b＞0，有：

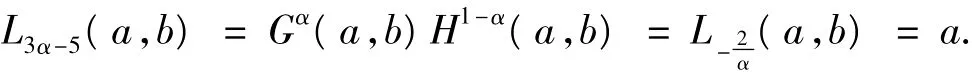
2)若 a=b，则由式(1)得，对∀α∈(0，1)，

由引理1得：

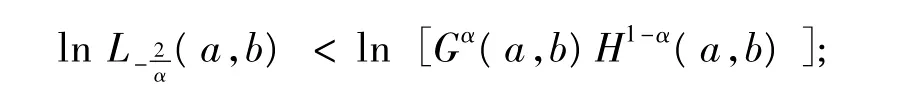
情形1α∈对∀ε∈(0，1)，∀x∈(0，1)，由式(1)得

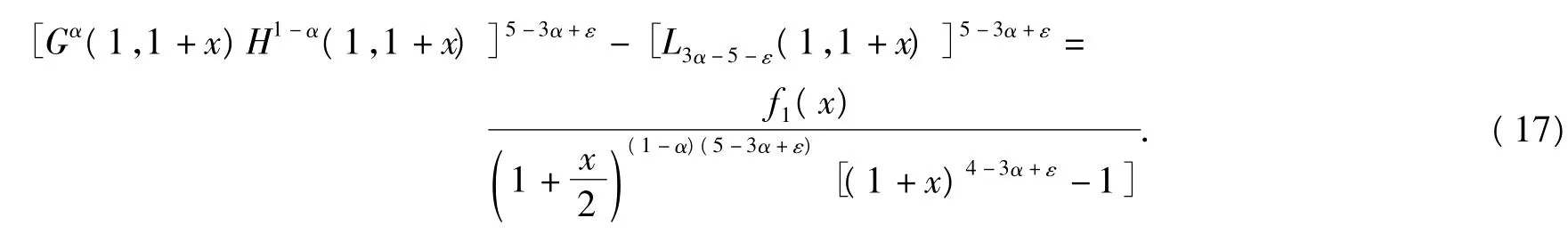
式(17)中，

设x→0，利用泰勒展开式得

情形2α∈对于∀ε∈(0，4 －3α)，∀x∈(0，1)，由式(1)有

式(21)中，

设x→0，利用泰勒展开式，有

另一方面，对于∀ε＞0，有：

［1］Stolarsky K B.The power and generalized logarithmic means［J］.Amer Math Monthly，1980，87(7)：545-548.

［2］Pearce C E M，Pecaric J.Some theorems of Jensen type for generalized logarithmic means［J］.Rev Roumaine Math Pures Appl，1995，40(9/10)：789-795.

［3］Mond B，Pearce C E M，Pecaric J.The logarithmic mean is a mean［J］.Math Commun，1997，2(1)：35-39.

［4］Chen Chaoping，Qi Feng.Monotonicity properties for generalized logarithmic means［J］.Aust J Math Anal Appl，2004，1(2)：2-4.

［5］Abuhany A A K，Salem S R，Salman I M.On steffensen's integral inequality with applications［J］.J Rajasthan Acad Phys Sci，2006，5(1)：1-12.

［6］Qi Feng，Chen Shouxin，Chen Chaoping.Monotonicity of ratio between the generalized logarithmic means［J］.Math Inequal Appl，2007，10(3)：559-564.

［7］Shi Huanshi.Schur-convex functions related to Hadamard-type inequalities［J］.J Math Inequal，2007，1(1)：127-136.

［8］Chen Chaoping.The monotonicity of the ratio between generalized logarithmic means［J］.J Math Anal Appl，2008，345(1)：86-89 .

［9］Qi Feng，Li Xiaoai，Chen Shouxin.Refinements，extensions and generalizations of the second Kershaw's double inequality［J］.Math Inequal Appl，2008，11(3)：457-465.

［10］Pittenger A O.The logarithmic mean in n variables［J］.Amer Math Monthly，1985，92(2)：99-104.

［11］Kahlig P，Matkowski J.Functional equations involving the logarithmic mean［J］Z Angew Math Mech，1996，76(7)：385-390.

［12］Polya G，Szego G.Isoperimetric inequalities in mathematical physics［M］.Princeton：Princeton University Press，1951.

［13］Leach E B，Sholander M C.Extended mean values II［J］.J Math Anal Appl，1983，92(1)：207-223.

［14］Sandor J.A note on some inequalities for means［J］.Arch Math：Basel，1991，56(5)：471-473.

［15］Carlson B C.The logarithmic mean［J］.Amer Math Monthly，1972，79：615-618.

［16］Alzer H.Ungleichungen für mittelwerte［J］.Arch Math：Basel，1986，47(5)：422-426.

［17］Alzer H，Qiu Songliang.Inequalities for means in two variables［J］.Arch Math：Basel，2003，80(2)：201-215.

Optimal generalized logarithmic mean bounds for the combination of geometric and harmonic means

ZHANG Fan1，QIAN Weimao2

(1.Huzhou Vocational＆ Technical College，Huzhou Zhejiang 313000，China;2.Huzhou Broadcast and TV University，Huzhou Zhejiang 313000，China)

It was compared the generalized logarithmic mean with the geometric combination of geometric and harmonic means by the elementary differential calculus.It was discussed for α∈(0，1)，the greatest value p and the least value q，such that the inequality Lp(a，b) ≤Gα(a，b)H1－α(a，b) ≤Lq(a，b)held for all a，b＞0.

inequality;generalized logarithmic mean;geometric mean;harmonic mean

O178

0 引言