第二类q－Beta算子的逼近性质

蔡清波

（泉州师范学院 数学与计算机科学学院，福建 泉州362000）

第二类q－Beta算子的逼近性质

蔡清波

（泉州师范学院 数学与计算机科学学院，福建 泉州362000）

基于q－积分的概念，研究了第二类q－Beta算子的逼近性质.通过计算得到了算子的各阶矩量及中心距量，并由光滑模和k－泛函分析得到算子的局部逼近性质.由于当q→1－时，算子退化到经典的第二类Beta算子，故其是对第二类Beta算子收敛性质的一种推广.

q－积分；q－Beta算子；矩；收敛阶

0 引言

近年来，q－整数在逼近论中的应用成为该领域的一个研究热点.自1997年Phillips在文［1］中提出q－Bernstein算子以来，很多学者对相关问题进行了研究，并得到了许多重要的结论［1－4］.

首先，引入与q－整数及q－微积分的相关一些概念［5－6］.对任意固定的实数q＞0及非负整数k，定义q－整数为更一般地，对于非整数t，定义分别定义q－阶乘及q－二项系数为和定义无穷限q－积分为假设级数绝对收敛.此外，定义如下函数q－Gamma积分定义为且满足Γq（t＋1）＝ ［t］qΓq（t），Γq（1）＝1.

定义第二类q－Beta函数为

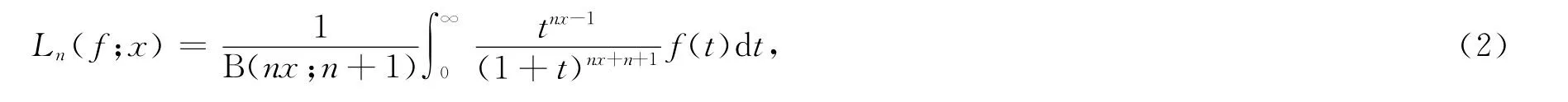
其中算子Ln（f；x）是由Stancu定义的正线性算子，在区间（0，∞）上是局部可积函数，当t→∞，n→∞时是以多项式阶增长的［7］.在此基础上，定义第二类q－Beta算子.设f∈C［0，∞），q∈ （0，1），则

值得注意的是，若q→1－，则Ln，1－（f；x）即为式（2）所定义的算子.

2 引理及证明

引理1 设f∈C［0，∞），q∈ （0，1），则如下等式成立：

证明 由q－Beta函数的定义（1），易得故（4）式成立.此外由于K（x；t＋1）＝qtK（x；t）［4］，从 而 有又因从而故（5）式成立.最后，由于故（6）式成立，引理1得证.

注1 由引理1，当q→1－时，Ln，1－（1；x）＝1，Ln，1－（t；x）＝x和为第二类Beta算子的各阶矩量［8］.

引理2 设f∈C［0，∞），q∈ （0，1），则有

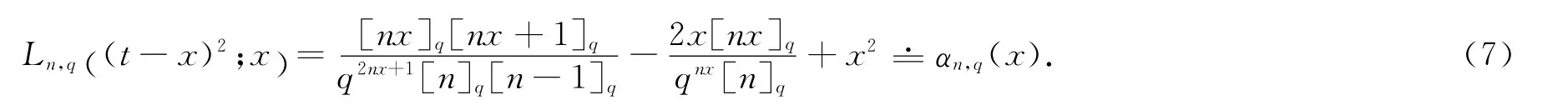
证明 由Ln，q（t－x）2；（x＝Ln，q（t2；x）－2xLn，q（t；x）＋x2及引理1易得证.）

注2 由引理2，当q→1－时为第二类Beta算子的二阶中心距量［8］.

3 主要结论

令CB［0，∞）为定义在［0，∞）上的实值连续有界函数空间，定义范数为k－泛函定义为其中δ＞0，由文献［9］中的定理2．4知，存在常数C＞0，使得

定理1 设f∈CB［0，∞），q∈ （0，1），则存在常数C＞0，使得对x∈ ［0，∞）有

其中αn，q（x）如（7）式所定义．

证明 构造辅助算子

令g∈C2B，由Taylor展开及（11）式，有从而，由（10）式及引理2，有

其中δn，q（x）如（7）式所定义．又由（10）和（3）式及引理1，有

从而由（10）和（12）式可得

在上述不等式右端中，对g∈W2取下确界有

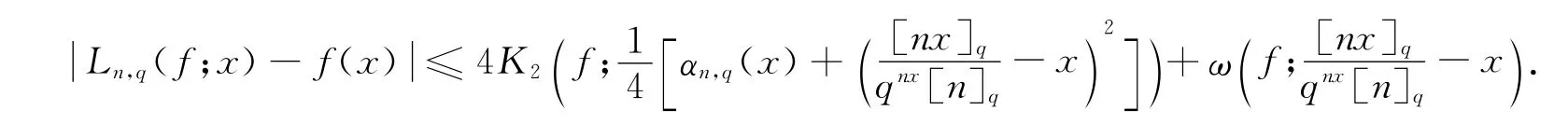
注3 令q＝｛qn｝为满足0＜qn＜1的序列，则有及从而得到算子Ln，qn（f；x）收敛到f（x）的点态收敛阶.

考虑如下函数类：设Hx2［0，∞）为定义在［0，∞）上且满足条件的函数f的集合，其中Mf为只依赖于f的常数.记Cx2［0，∞）为包含于Hx2［0，∞）的所有连续函数子空间.定义f在闭 区间［0，a］，a＞0上的光滑模为

定理2 设f∈Cx2［0，∞），q∈ （0，1），ωa＋1（f；δ）为有限区间［0，a＋1］⊂ ［0，∞），a＞0上的光滑模，则有其中αn，q（a）如（7）式所定义.

证明 当x∈ ［0，a］，t＞a＋1，由t－x＞1有

当x∈ ［0，a］，t≤a＋1时，有

由（13）和 （14）式 可 得，当x∈ ［0，a］，t≥ 0 时 有从而所以，由Schwartz不等式及引理2知，当q∈（0，1），x∈ ［0，a］时，有取定理2得证.

［1］Phillips G M.Bernstein Polynomials Based on theq－integers［J］.Ann Numer Math，1997，4：511－518.

［2］Mahmudov N I.Statistical Approximation of Baskakov and Baskakov－Kantorovich Operators Based on theq－integers［J］.Cent Eur J Math，2010，8（4）：816－826.

［3］Do~gru O，Duman O.Statistical Approximation of Meyer－K¨onig and Zeller Operators Based onq－integers［J］.Publicationes Mathematicae Debrecen，2006，68（1／2）：199－214.

［4］De Sole A，Kac V G.On Integral Representation ofq－gamma andq－beta Functions［J］.Atti Accad Naz Lincei Cl Sci Fis Mat Natur Rend Lincei（9）Mat Appl，2005，16：11－29.

［5］Gasper G，Rahman M.Basic Hypergeometric Series，Encyclopedia of Mathematics and its applications［M］.UK Cambridge：Cambridge University Press，1990：35.

［6］Kac V G，Cheung P.Quantum Calculus［M］.Ne wYork：Universitext，Springer－Verlag，2002.

［7］Stancu D D.On the Beta Approximation Operators of Second Kind［J］.Rev Anal Numér Théor Approx，1995，24：231－239.

［8］Abel U.Asymptotic Approximation with Stancu Beta Operators［J］.Rev Anal Numér Théor Approx，1998，27：5－13.

［9］DeVore R A，Lorentz G G.Constructive Approximation［M］.Berlin：Springer，1993.

Approximation Properties of the Second Kindq－Beta Operators

CAI Qing－bo
（SchoolofMathematicsandComputerScience，QuanzhouNormalUniversity，Quanzhou362000，China）

We introduced the second kind of Beta operators based on the concept ofq－integral.We computed the moments and central moment of the operators，and investigated local approximation properties by the modulus of continuity andk－functional with analysis techniques.

q－integral；q－Beta operators；rate of convergence；moments

O174.41

A

1004－4353（2011）03－0208－04

2011 -05 -07

福建省教育厅科技项目（JK2011041）

蔡清波（1981—），男，讲师，研究方向为算子逼近论及计算几何.