Generalized Maximum Principles and Stochastic Completeness for Pseudo-Hermitian Manifolds∗

Yuxin DONG Weike YU

Abstract In this paper,the authors establish a generalized maximum principle for pseudo-Hermitian manifolds.As corollaries,Omori-Yau type maximum principles for pseudo-Hermitian manifolds are deduced.Moreover,they prove that the stochastic completeness for the heat semigroup generated by the sub-Laplacian is equivalent to the validity of a weak form of the generalized maximum principles.Finally,they give some applications of these generalized maximum principles.

Keywords Pseudo-Hermitian manifold,Omori-Yau type maximum principles,Stochastic completeness

1 Introduction

The present paper is mainly devoted to the study of generalized maximum principles and stochastic completeness for pseudo-Hermitian manifolds.For the convenience of readers,an introduction to pseudo-Hermitian geometry will be presented in Section 2,and the definition of stochastic completeness for pseudo-Hermitian manifolds will be given in the front part of Section 4.Roughly speaking,pseudo-Hermitian manifolds are CR manifolds of hypersurface type with positive definite pseudo-Hermitian structures(see Section 2 for the precise definition).Let(M2m+1,H,J,θ)denote a pseudo-Hermitian manifold,where(H,J)is a CR structure of type(m,1)and θ is a pseudo-Hermitian structure.It carries a positive definite Levi form Lθon H which is induced by the complex structure J and the pseudo-Hermitian structure θ.Furthermore,on a pseudo-Hermitian manifold,there is a natural 2-step sub-Riemannian structure(H,Lθ)which induces the Carnot-Carath´eodory distance rccon M.So we can see that the pseudo-Hermitian manifolds carry rich geometric structures.

In this paper,firstly,by using a similar method as in[24],we establish a generalized maximum principle for pseudo-Hermitian manifolds(see Theorem 3.1).Consequently,in terms of the sub-Laplacian and Hessian comparison theorems,respectively,we obtain the following generalized maximum principles of Yau and Omori types,respectively.

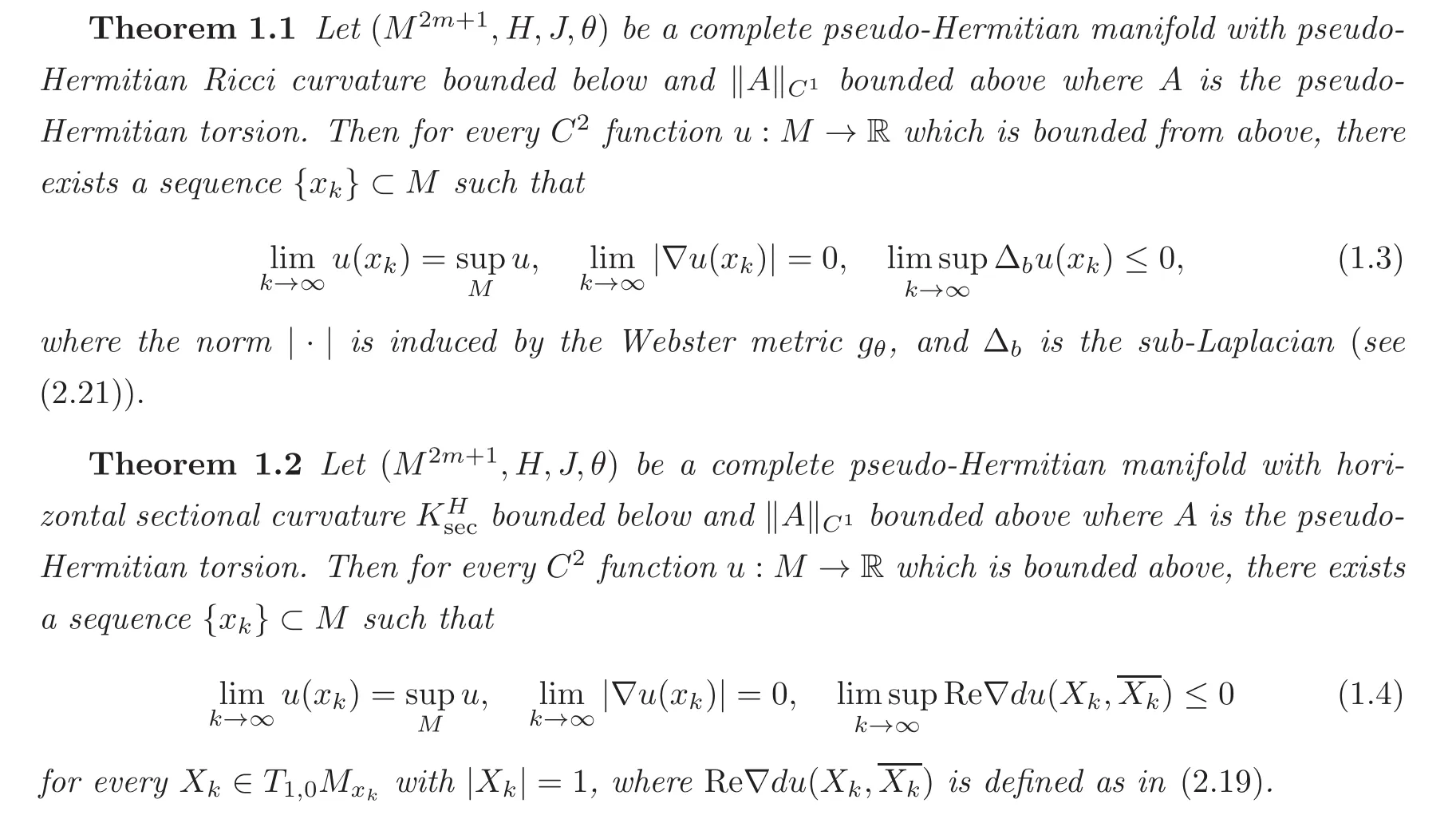
Moreover,we study the relationship between the generalized maximum principles and stochastic completeness for the heat semigroup generated by the sub-Laplacian.Indeed,we prove that the stochastic completeness of a pseudo-Hermitian manifold is equivalent to the validity of a weak form of the generalized maximum principles,which is a pseudo-Hermitian form of[23,Theorem 1.1].

Theorem 1.3 Let(M2m+1,H,J,θ)be a non-compact pseudo-Hermitian manifold.The following statements are equivalent:

At last,we will give two applications of the generalized maximum principles for pseudo-Hermitian manifolds,which are similar to some results in Riemannian case(cf.[26,32],ect.).One is to study differential inequalities on a pseudo-Hermitian manifold,and the other is to investigate the obstruction of pseudo-Hermitian scalar curvature to CR conformal deformations.

2 Preliminaries

In this section,we present some facts and notations in pseudo-Hermitian geometry(cf.[4,13]).

A CR manifold is a real smooth orientable 2m+1 dimensional differentiable manifold M equipped with a complex subbundle T1,0M of complex rank m of the complexified tangent bundle TM⊗C such that

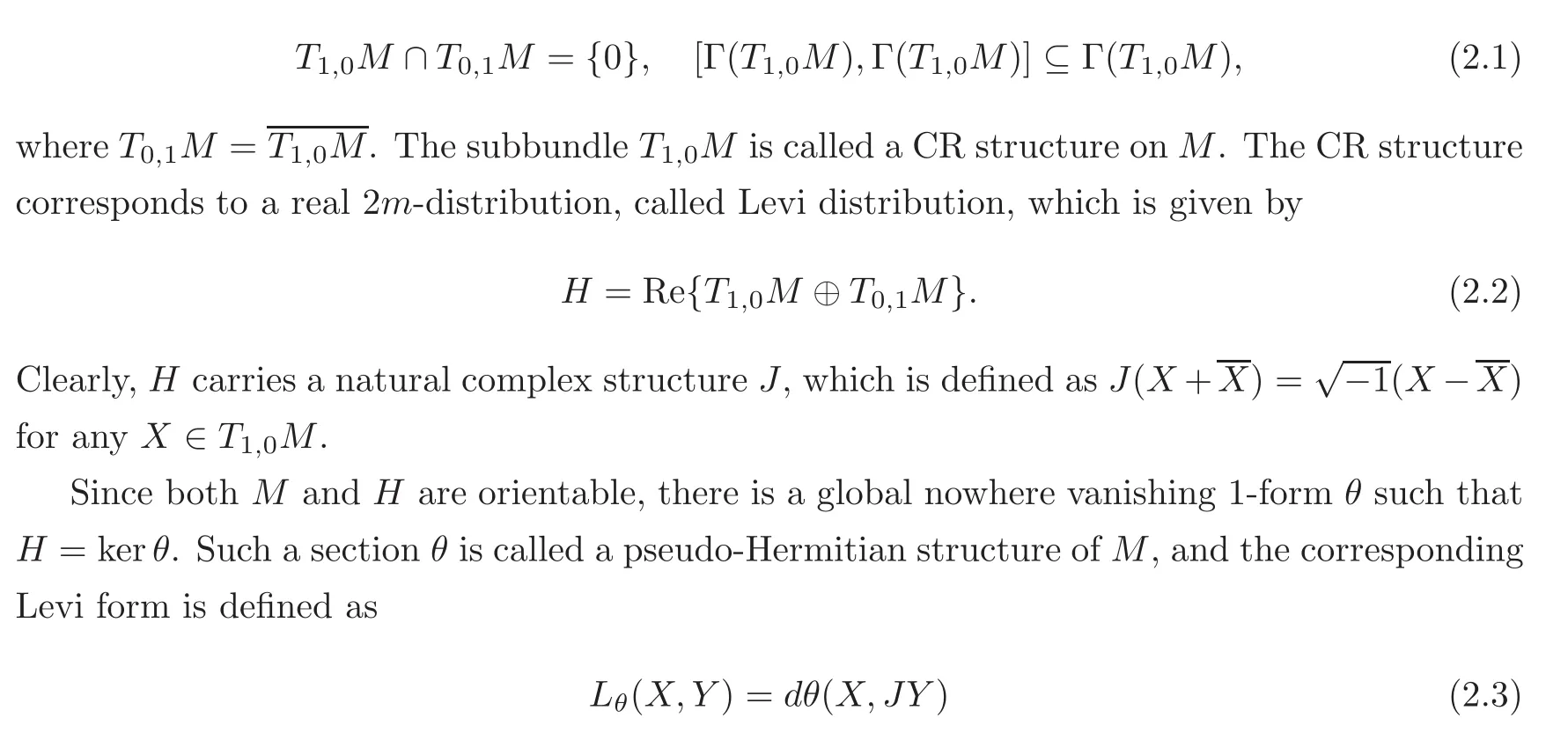
for any X,Y ∈H.The second condition in(2.1)implies that Lθis J-invariant,and thus symmetric.If the Levi form Lθis positive definite on H,(M2m+1,H,J)is said to be strictly pseudoconvex.Such a quadruple(M2m+1,H,J,θ)is called a pseudo-Hermitian manifold.

The non-degeneracy of Lθon H implies that all sections of H together with their Lie brackets span TxM at each point x∈ M.In fact,(M2m+1,H,Lθ)is a 2-step sub-Riemannian manifold.A Lipschitz curve γ :[0,l]→ M is said to be horizontal if γ′(t) ∈ Hγ(t)a.e.in[0,l].From the well-known theorem of Chow-Rashevsky(cf.[9,25]),it follows that for any two points p,q∈M,there is a horizontal Lipschitz curve joining p and q.Consequently,the Carnot-Carath´eodory distance is defined by

which is usually called the Webster metric.

For a pseudo-Hermitian manifold(M2m+1,H,J,θ),one can define two notions of completeness by using the Carnot-Carath´eodory distance rccof Lθand the Riemannian distance r of gθ,respectively.Actually,they are equivalent,because rccand r are locally controlled by each other(cf.e.g.,[20]).

On a pseudo-Hermitian manifold,there is a canonical connection preserving the CR structure and the Webster metric,which is called the Tanaka-Webster connection.

Theorem 2.1(cf.[34—35])Let(M2m+1,H,J,θ)be a pseudo-Hermitian manifold with the Reeb vector field ξ and Webster metric gθ.Then there is a unique linear connection ∇ on M satisfying the following axioms:

(1)∇XΓ(H)⊂ Γ(H)for any X ∈ Γ(TM).

(2)∇J=0,∇gθ=0.

3 Generalized Maximum Principles for Pseudo-Hermitian Manifolds

If{xk}lie in some compact subset of M,then u attains its maximum at some point,hence the results of Theorem 3.1 hold by the classical maximum principle.So we just need to consider

Remark 3.1(1)The proof shows that we just need γ to be C2in a neighborhood of xk.Therefore,using the trick of Calabi(cf.[5]),this theorem remains valid for the important case γ(x)=r2(x),where r(x)is the Riemannian distance with respect to gθfrom a fixed point o to x.

(2)Similar generalized maximum principle for Riemannian manifolds was established by[24].

In order to obtain the generalized maximum principle of Yau’s type in pseudo-Hermitian geometry,we need a sub-Laplace comparison theorem as follows.

Taking γ(x)=r2(x)and G(r)=r2+1 in Theorem 3.1 again and combining with Lemma 3.2,we obtain the generalized maximum principle of Omori’s type in pseudo-Hermitian geometry as follows.

4 Stochastic Completeness for Pseudo-Hermitian Manifolds

Analogue to the stochastic completeness in Riemannian Geometry,one can also introduce a similar definition for pseudo-Hermitian manifolds.

Definition 4.1 A pseudo-Hermitian manifold(M,H,J,θ)is said to be stochastically complete if Pt1=1 for all t>0.

Remark 4.3 By the semigroup property of Pt,one can show that Pt1=1 for all t>0 if and only if Pt1(x)=1 for some(x,t)∈M×R+(cf.[15,Theorem 6.2]).

Now we discuss the relationship between stochastic completeness and generalized maximal principles.For this purpose,we need the following lemmas.

Lemma 4.1 Let Ω be a relatively compact connected open subset of a pseudo-Hermitian manifold(M2m+1,H,J,θ).Let Lu= ∆bu − λu,where λ is a positive constant.If u is abW1,2(Ω)function satisfying

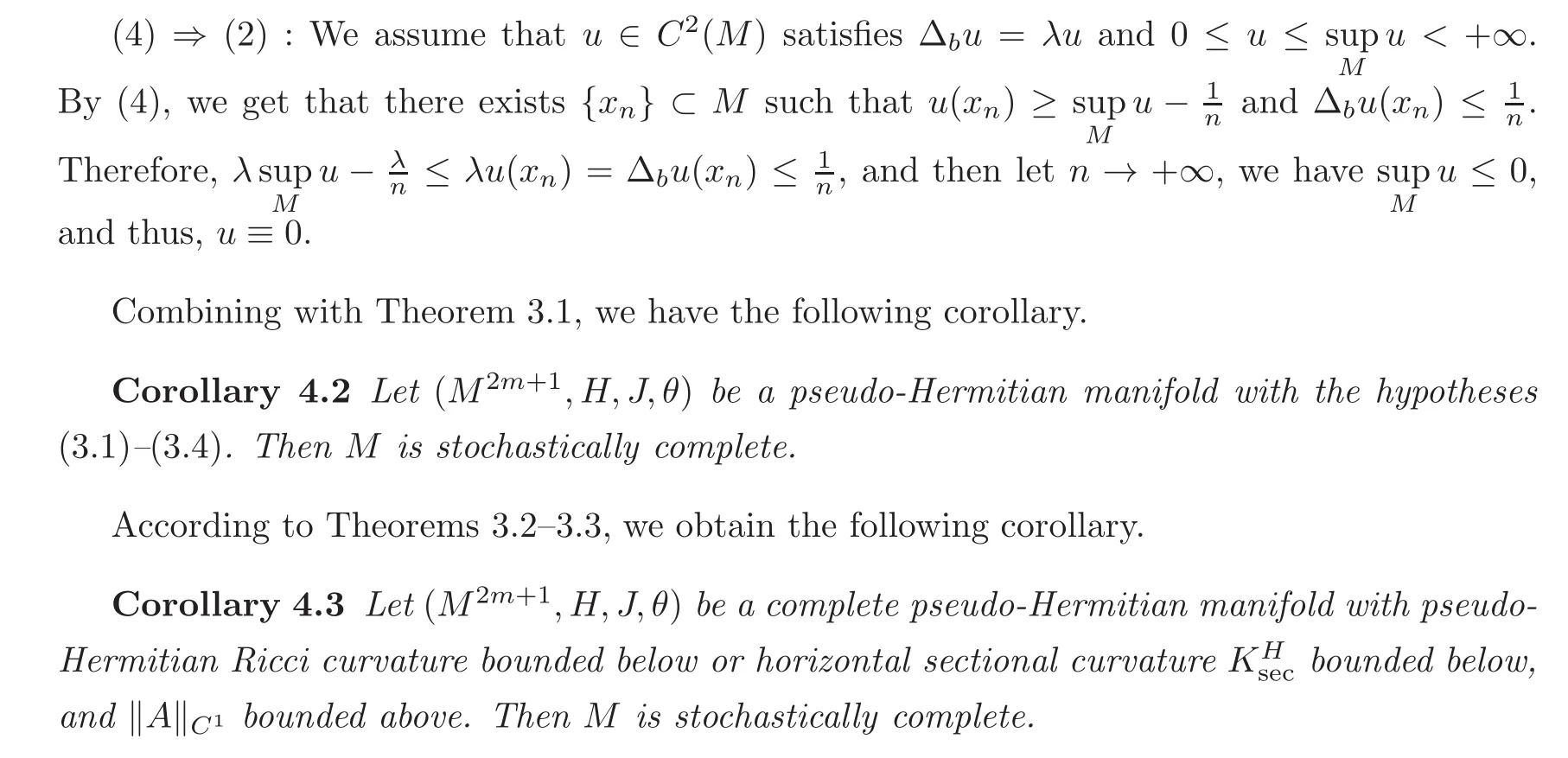
Before ending this section,we would like to mention that the stochastically completeness of the heat semigroup Ptgenerated by the sub-Laplacian on a contact manifold was investigated in[2]too.They gave a sufficient condition,in terms of a generalized curvature inequality,to deduce the stochastically completeness.Finally it is also obvious that our Theorem 4.4 may be generalized to more general sub-Riemannian manifolds.

5 Application