Fractional soliton dynamics of electrical microtubule transmission line model with local M-derivative

Nauman Raza,Saima Arshed,Kashif Ali Khan and Mustafa Inc

1 Department of Mathematics,University of the Punjab,Quaid-e-Azam Campus,Lahore,Pakistan

2 Department of Mathematics,University of Engineering and Technology,Lahore,Pakistan

3 Department of Computer Engineering,Biruni University,Istanbul,Turkey

4 Department of Mathematics,Science Faculty,Firat University,Elazig,Turkey

5 Department of Medical Research,Medical University Hospital,China Medical University,Taichung,Taiwan,China

Abstract In this paper,two integrating strategies namely exp[- φ (χ )]and -expansion methods together with the attributes of local-M derivatives have been acknowledged on the electrical microtubule(MT) model to retrieve soliton solutions.The said model performs a significant role in illustrating the waves propagation in nonlinear systems.MTs are also highly productive in signaling,cell motility,and intracellular transport.The proposed algorithms yielded solutions of bright,dark,singular,and combo fractional soliton type.The significance of the fractional parameters of the fetched results is explained and presented vividly.

Keywords: solitons solution,microtubule,nonlinear transmission line,-expansion method

1.Introduction

The investigation of a nonlinear routine is mandatory to understand the principles of the universe.Because of the wide significance of physical systems,the dissemination of modulated matter-waves like the solitary waves is the significance parameter as it is persisting for several years.To expedite the solitons experiment,Russel [1] in 1834 started work on it,and Zabusky et al [2] performed computer simulation regarding these soliton waves.The said domain of research in the systems of nonlinear theory has flourished as a rich field in the last three decades.No doubt,representation of chaotic electrical transmission (ET) lines are managed by laboratory experiments but these are very narrow in numbers.Further,the influence of network properties along the nonlinear ET lines was done by Pelap et al[3]where the soliton’s energy has been talked over with several frequency modes and inductances.Apart from that,some of the modern development of mathematical physics animated the probe of solutions[4–6]for nonlinear equations which boost-up in the myriad fields of physics.These unfolding are imperative in observing the principles of physical systems.In the ongoing past,there are multiple but distinctive ongoing schemes for integrability that choose several traveling wave solutions of diverse nonlinear evolutionary equations.These schemes incorporate the technique of Jacobi elliptic,extended trial equation,F-expansion,the generalized differential transform,modified simple equation,Adomian decomposition,thexpansion,the first integral,sine-cosine algorithm,the single wave ansatz strategy,and a series of other schemes mentioned in [7–9].

Microtubules (MTs) are one of the three principal components of cytoskeletal polymers involved in a certain figure of particular cellular actions incorporating the organelle’s traffic using motor proteins,cellular engineering,cell division,motility,and a doable functioning in information processing within neuronal tasking.MT are hollow cylinders with a lumen,a polymer of alpha-tubulin and beta-tubulin.As they join/fuse,they make a dimer.The dimer has two identical or similar tubulin,named as a heterodimer.These dimers arrange themselves in head the tail manner and forming protofilaments(PFs).Thirteen of these PFs make a long chain of polymers and these polymers will put together in the form of a sheet and this sheet is rolled or converted into a tube.That is called a MT whose diameter is 25 nm thick.Its applications are in cardiac growth like the cardiomyocyte MT network (which is implemented in hypertrophy).According to what MTs work and exercise the electrical signals/electrical information was still untold in a larger sense.Sataric et al[10]launched the new model of MT as a chaotic ET line converted it into some rings.And developed a stochastic mathematical relation for the local electrostatic potential integrated with the ionic wave disseminating along an MT.Further,he [11] presents a nonlinear dimer's dynamics structure within MTs.And with the help of numerical and analytic techniques,the kink soliton solution is formed,which is superintended for the energy shifting along the MTs.The dynamical behavior of MTs can be modified into the nonlinear equation(s).These equations portrayed the natural experiences into the mathematical prototypes that crop up in the several fields of engineering,optical fibers,human biology,fluid mechanics,geochemistry,etc.In the current article,the electrical MT model and impact of capacitance nonlinearity on the engendering of ionic heartbeats are diagnostically considered.Quickened movement of the MT offers to ascend to electrodynamic wonders which assumes a crucial job in intracellular flagging and data handling [12–24].Nur et al[25]present a methodology to elucidate the solutions of nonlinear equations depicting the MT dynamics by the aid of exp[-φ(χ)]-expansion method.The majority of these electrodynamic frameworks happen as nonlinear halfway differential conditions.These conditions have moved toward becoming a consideration in pretty much every field of life,for example,hypothetical material science,material science,liquid elements,acoustics,cosmology,oceanography,astronomy,atomic physics and plasma material science,shallow water wave stream,nonlinear optics [26,27],and some with biophysical implications can be seen in [28,29].

Fractional calculus (FC) is known as the extension of integer-order calculus [30].And most of the investigation of derivatives and anti-derivatives of non-integer(arbitrary,real,or complex)order is one of the emerging branches of(FC),as FC has a lot of significance in the numerous fields of science and engineering:at present its applications can be seen in the economy,fluid mechanics,image processing,biological models,and the most impressive in weather-controlling.Usage of special functions also provides insights into the importance of FC in the other branches of mathematics.Some of the certain attributes of FC involve rules of linearity,product,quotient rule and,chain rule,also include the definition of function composition in the calculus of integerorder.Especially,the attributes of FC are connected with the ordinary calculus of order one,as we set the order of the FC equal to one.In 2014,Khalil is the person who prompted the‘local derivative’,that is persistent with the classical description of derivatives and holds all the patent obligations of the said rate of change,but note that,it does not meet the criteria of some famous results like theorem of mean value,extended mean value,and Roll’s theorem [31].To remove that glitch,in 2018,the local M-derivative have been introduced by Sousa et al,where this new definition endorsed the idea prompted by Khalil et al and fulfill all of the above attributes [32].

In fractional derivative,several useful techniques have been introduced in multiple investigations to cite the nonlinear fractional evolution equations for exploring the traveling wave solutions [33–35].Currently,Gao et al [36]suggested an easy method,the modified expansion function method plus the sinh-Gordon technique to expedite some new solitons solutions with the M-fractional paraxial wave equation.And under the influence of several nonlinear forms,Ghafri et al [37] explored the symmetry version in the fractional Ginzburg–Landau equation.Further,Fujioka et al [38]discussed the fractional dimensions by creating the fractional optical solutions in a generalized nonlinear Schrödinger equation.

No doubt,the mathematical processes managed with derivatives and integrals of arbitrary and complex orders are named FC.So,it opens a smart window to describe and understand the behavior of complex systems in an upgraded way.To apprehend the dynamics of biological systems,mathematical prototypes employing ordinary differential equations with integer-order,have been significantly utilized.But the attributes of most biological models have memory or aftereffects.These effects have a significant role in the disease spread.The disease spread in the future will be influenced by the involvement of memory effects on past events.The distance of memory effect reveals the history of disease dissemination.Thus,memory effects on the escalation of an infectious malady can be probed with the aid of fractional derivatives.And the development of such models by fractional differential equation has more privileges than classical integer-order mathematical modeling,in which such actions are abandoned.In some cases,the models of FODEs look more well established with the real happening than the integer-order models.The reason behind that FD activates the description of the memory and hereditary attributes inherent in numerous materials and mechanisms.So,there is an exponential need to study and use the differential of fractional-order as proposed by Rihan and Utoyo [39,40].Some of the current examinations in biological science proposed that MT dynamics in the adult brain function is the crucial process of learning and memory and it may be agreed in degenerative maladies,such as Alzheimer's disease.This enhances the likelihood of targeting MT dynamics in the making of current therapeutic agents as can be noticed in[40,41].FC is also appropriate for designing the electrical attributes of biological models.As Zoran et al [42] found a modern family of generalized prototypes for electrical impedance and invoked them to human skin by laboratory data fitting.Baleanu with other authors [43] present research by adopting some productive integration schemes to achieve the outcomes of optical soliton space–time-fractional nonlinear equation for the MTs dynamics,which examined as one of the most significant parts in cellular processes of biology.They use the attributes of the fractional complex transformation and conformable derivatives which involve the results of hyperbolic,rational,complex,and exponential functions.The outcomes of this research in fractional medium provide significant upshots and grabbing the attributes of the nonlinear waves.

The current work is carried out to explore the effects of time-fractional evolution on the MT transmission lines model by employing the properties of local M-derivative.For its implementation,we are providing the general results or solutions of the proposed model by adopting exp[-φ(χ)]and-expansion methods together with graphical illustrations.The next part of the article is designed as follows: the elementary portion of the local M-derivative along with certain attributes is explained in the 2nd section.The concise overview of the adopted technique is insight in section 3.The detail of the suggested model is presented in section 4 and the quest of soliton results via suggested techniques can be seen in section 5.Results of the obtained solutions have been discussed in the same section.Lastly,section 6 provides the conclusion corner.

2.Interpretation of local M-derivative

This component proposed the fundamental attributes and definition of the local M-derivative [32].Ifh:R+∪{0 }→R,0<λ<1,andt> 0 then the function h,which is defined as

is called the local M-derivative of order λ,whereEδ(·),∀ω>0,is referred as the Mittag–Leffler function containing one parameter [44].In the specified cut(0,b) andb>0,if h(t)is differentiable,and as one get the existence of limt→0+{h(t)},

and

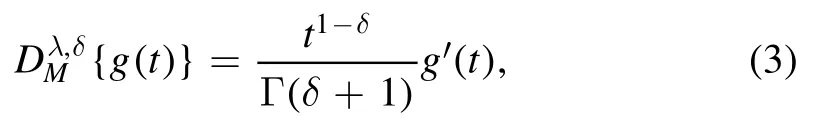
Apply the chain rule,one get:

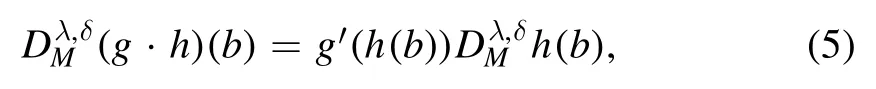
therefore,the connection from equations (4) and (5) can be determined mentioned below:

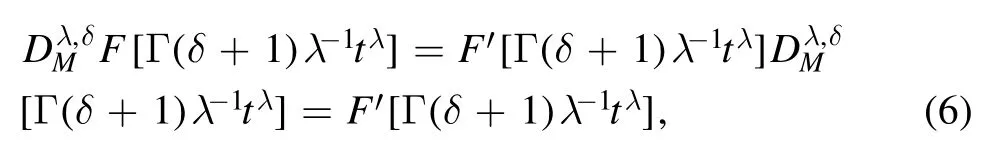
together with

and eventually we get

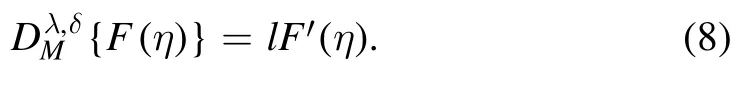
where l represent some constant.

3.Suggested analytic techniques

This component provides the delineation of two suggested techniques like the exp[-φ(χ)] and-expansion method.Consider the following general nonlinear PDE

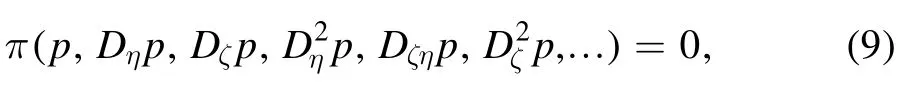
wherep=p(ζ,η)is function to be determined.Invoking the wave transformation

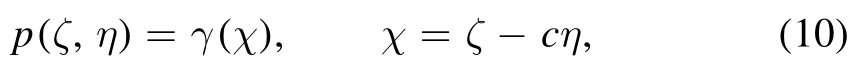
the above PDE is reduced to nonlinear ODE as

3.1.Method of exp［-φ（χ）］-expansion

In the current method,the truncated series i.e.the wave solution of equation (9) is of the type

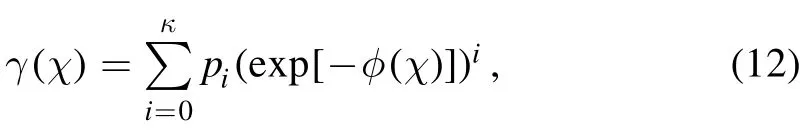
where piare the values to be evaluated andφ(χ) is the result or outcome of the below mentioned equation

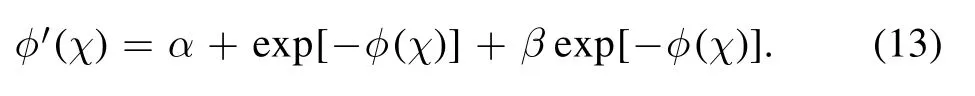
Some of the cases related to the general solutions of equation (13) are mentioned below.

Case 1Whenβ≠0,α2-4β>0,then

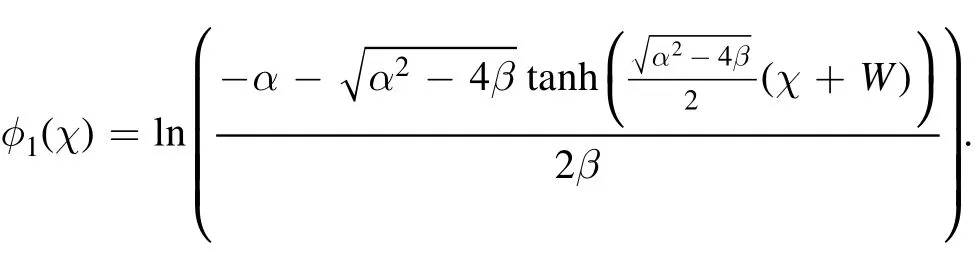
Case 2Whenβ≠0,α2-4β<0,then

Case 3Whenβ= 0,α≠0,andα2-4β>0,then

Case 4Whenβ≠0,α≠0,andα2-4β=0,then

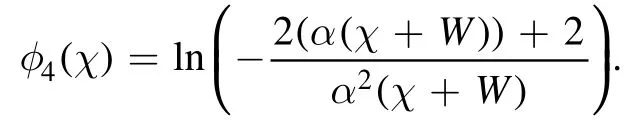
Case 5Whenβ= 0,α= 0,andα2-4β=0,then

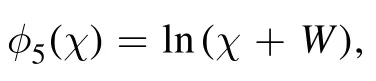
where W being the integration constant.The value of κ is originated from equation (11),by acknowledging a balance between greatest order derivative with greatest order nonlinearity.

Inserting the value ofγ,γ′in equation (11),we get

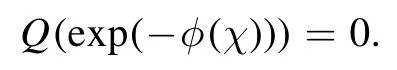
As each powers of (e xp[ -φ(χ)])jidentified to zero,one can obtained a nonlinear network of algebraic equations in pi.After putting the values of piin equation (12),the exact solutions are obtained for equation (9).

In this technique,the truncated series i.e.the traveling wave solution of equation (9) is of the type

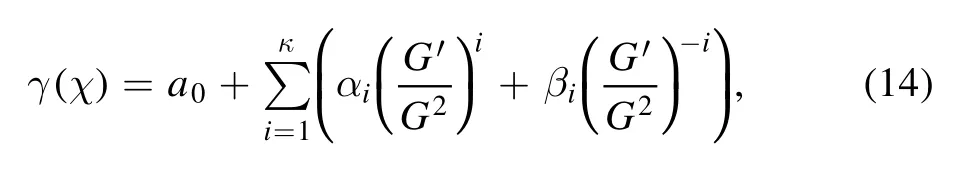
Note it that,G=G(χ) is the result of an ODE.

with the constraint thatα≠0,β≠1 are integers.a0,αi,βiare constants to be found.

Below mentioned are some cases related to the general solutions of equation (15).

Case 1

Ifαβ> 0,

Case 2

Ifαβ< 0,

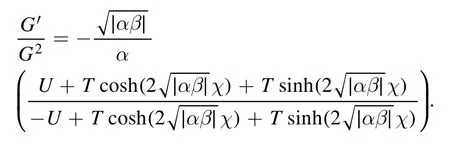
Case 3

Ifα≠0,β=0,

where T and U are nonzero constants.On the same way,the value of κ is originated from equation (11),by acknowledging a balance between greatest order derivative with greatest order nonlinearity.

Inserting the value ofγ,γ′in equation (11),we get

4.Governing model

Contemplate

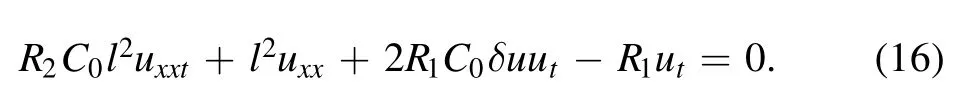
as the structure of MTs like (nonlinear) RLC transmission line,whereR1= 109ΩX,R2= 7 × 10-9represent the longitudinal and transversal parcel of the resistance of an rudimentary ring,whilel= 8 × 10-9andC0= 1.8 ×10-15F represent the length and entire maximal capacitance of the elementary rings (ER).δ(δ< 1) is the tool to describe the nonlinear features of ER in MT.Note that,with an uprising concentration of counter-ions,the capacitance C0should vary because of theβ-TTsvariability.The connection between the elementary capacitor with voltage is chaotic,as like the charge-voltage connection for a reverse-biased diode junction.Now we consider the

the value of b is anticipated to be infinitesimal,and then from the figure 1 and after applying the local M-derivative,we can write down

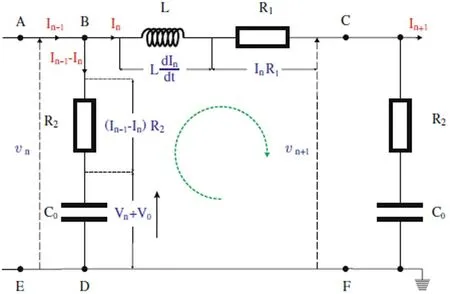
Figure 1.Geometry of the nth ER betwixt the dotted lines.

Now from the nth MT ring,its section BC after applying the local M-derivative wave transformation and usage of section 2,we get:

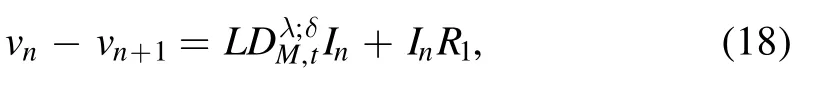
where the voltages across the transmission lines AE and CF are vnandvn+1.Likewise,ifVn+V0is the voltage over the variable type capacitorthen we get

Note it that,the bias-voltage of the capacitor is represented by V0.Further

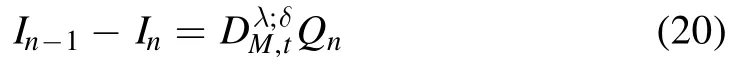
indicates the current through the line BD.From equation(18),we get

and

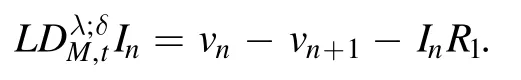
Use equation (20),we will get

Further,it implies

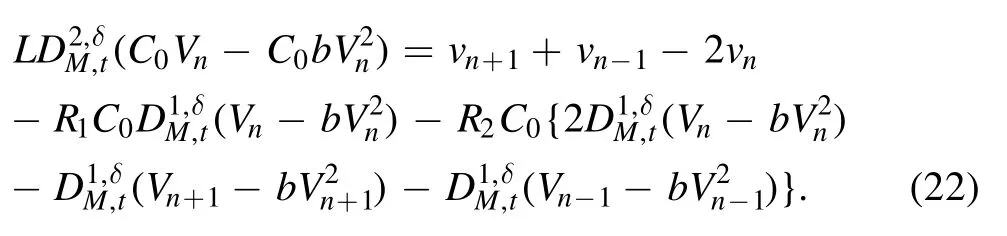
Remember that the voltage Vnswitches moderately from the ring n to its vicinity,and with the help of Taylor’s expansion,we can carefully unfold Vnin a continuum estimation in the form of a small spatial parameter l,

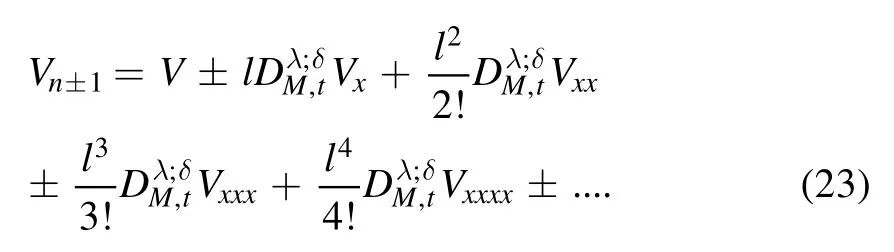
and ignoring some higher order terms of l,we can express the Taylor expansion as

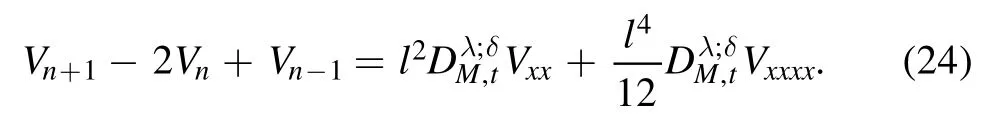
Then equation (22) with the use of equations (23),(24)becomes

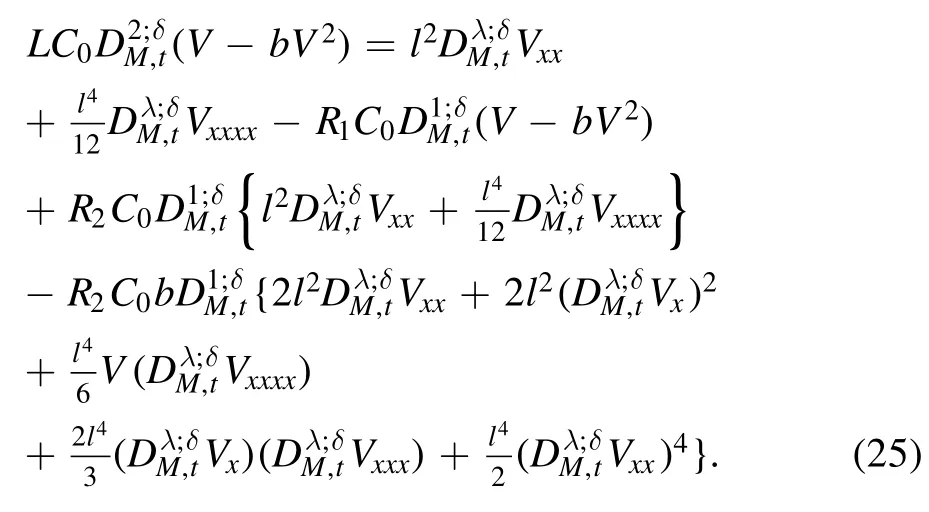
Since the time differences of the local voltage V are inappreciable as compared to the fixed background voltage V0,one can guess that the time-dependent derivative is of the order of the infinitesimal parameter ∊,as well as the chaotic behavior,terms bV2of voltage are of the order∊2.So equation (25) can be obtained by choosing only the principal terms:

Since LC0is very small,so the term occurring on the lefthand side will be ignored and,we get; so the final fractional form of equation(16)with the help of above-mentioned work can be written as:

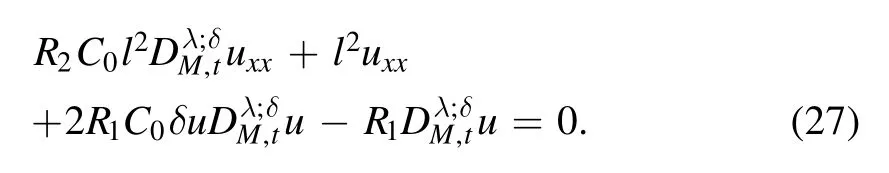
where the local M-derivative of order λ of a ruleu(x,t),w.r.t t is identified asImportant to note that ifδ= 1=λ,equation (27) can be modified into the original equation.

5.Solitary wave solutions via proposed techniques

Consider the resemblance variables of fractional traveling wave mentioned below:

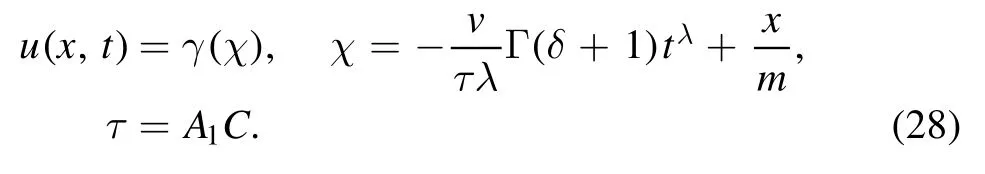
The theme of this work is to seek solutions like soliton,shock wave and singular type for the above said MT equation.Applying the above transformation,equation (27) takes the following form after integrating once

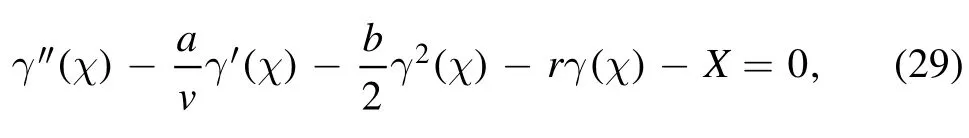
where the dimensionless parameters are defined as

Employing the homogenous balance principle in equation(19)impartκ= 2.Then equation (12) becomes

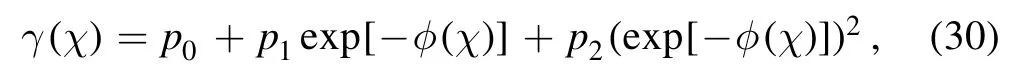
where the factorsp0,p1,p2needed to be calculated.As section 3 enforced to adopt the proposed method of solution technique,the values of unknown constantsp0,p1,p2have been found.The emerging solutions are elaborated as as follows

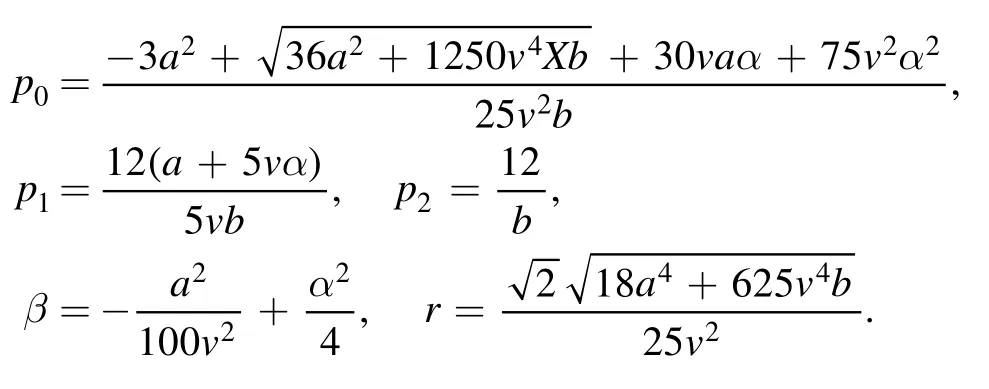
Following are the solutions obtained by exp[-φ(χ)]-expansion method.

Whenα2-4β>0andβ≠0,then hyperbolic solution has been gained as

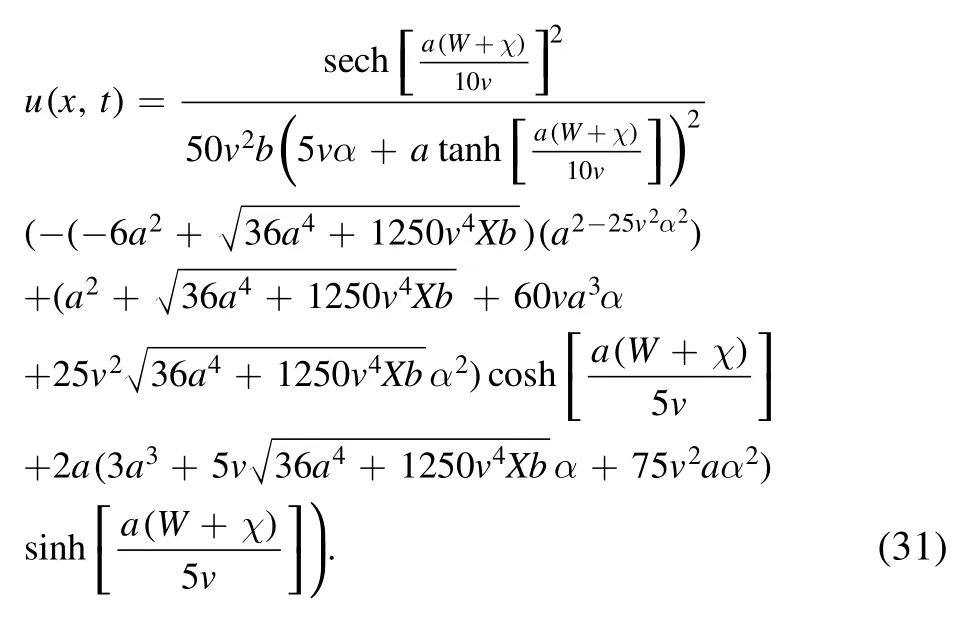
Figure 2 shows the graphical representation of equation (21)of the wave solutionsu(x,t) for different chooses of fractional values in(a)λ= 0.65,δ= 0.8 and in(b)λ= 1,δ= 1.Further,figure 2(c) explains the 2D plot of equation (21)versus time t at x = 2 for several fractional values.

Whenα2-4β>0andβ= 0 andα≠0 then singular soliton solution has been obtained as

Figure 2.Graphical view of equation(31)for fractional parameters λ and δ.

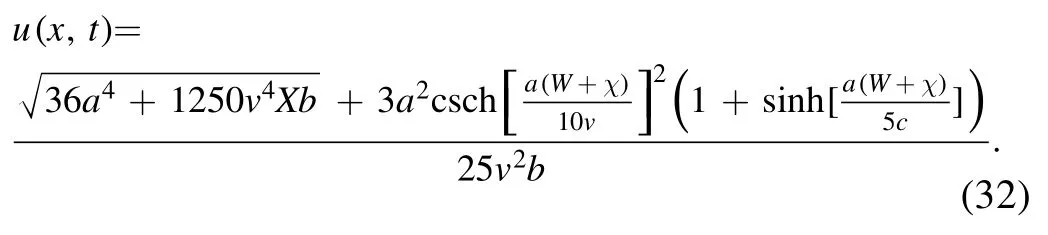
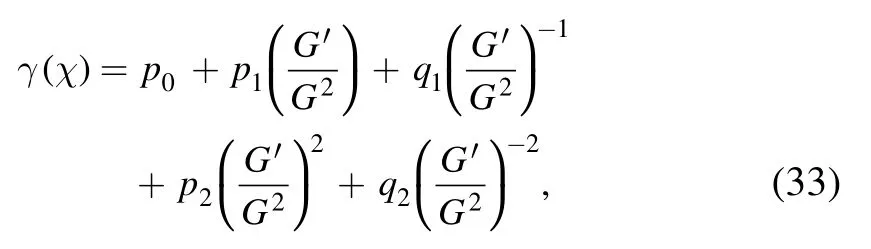
wherep0,p1,p2,q1,q2are constants to be calculated.Adopt the same proposed technique as mentioned in section 3,the values of unknown constants have been obtained.The emerging results are compiled as follows.

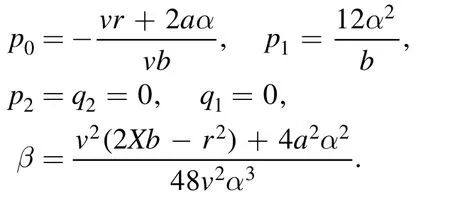
The following soliton solutions are obtained like

Ifαβ> 0,the said periodic but singular type is fetched as

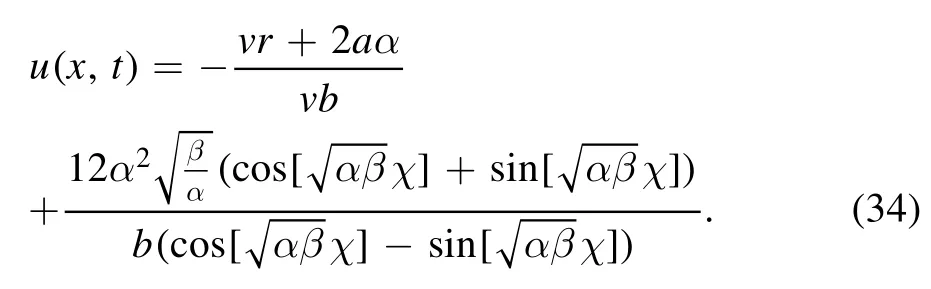
The said singular type solution forαβ< 0,is fetched as

The wave profilesu(x,t) of equation (25) are shown vividly in figure 3 against different chooses of fractional valuesλ= 0.65,δ= 0.8,in(a)λ= 0.85,δ= 0.9 and in(b)λ= 1,δ= 1 in(c).Figure 3(d)explains the 2D plot of equation(21)versus time t at x = 2 for several fractional values.

Figure 3.Graphical view of equation (35) for fractional values λ and δ.

Ifβ=0,α≠0,the wave solution of plane type is elucidated as

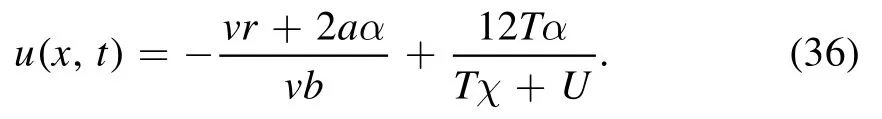
6.Conclusion

In this paper,exp[-φ(χ)]and-expansion techniques have been invoked successfully on the electric model ofMT to yield the fractional dark,singular,bright,and combo soliton results by using the attributes of local M-derivative.The 3Dgraphs are depicted to fetch the fractional solutions,also the influence of fractional parameter λ is shown in 2D-graphs against the numerous values of λ.Such sorts of the solution are useful for the inquisition of some real-time physical processes.Although the above-mentioned techniques have some deficiencies i.e.it contains lengthy calculations but due to the availability of symbolic calculators such as Maple etc,one can get the controlled results efficiently.Also,theselection of factors produces several facts of these results and their graphical faces to be type illustrative without rendering the article so massive,should more realizations be expected.Further,these techniques can also be enforced adroitly for a wide range of NLEEs.

ORCID iDs