Joule–Thomson expansion of RN-AdS black hole immersed in perfect fluid dark matter

Yihe Cao,Hanwen Feng,Wei Hongand Jun Tao

Abstract In this paper,we study the Joule–Thomson expansion for RN-AdS black holes immersed in perfect fluid dark matter.As perfect fluid dark matter is one of the dark matter candidates,we are interested in how it influences the thermodynamic properties of black holes.Firstly,the negative cosmological constant could be interpreted as thermodynamic pressure and its conjugate quantity as the thermodynamic volume,which give us more physical insights into the black hole.Moreover,we derive the thermodynamic definitions and study the critical behaviour of the black hole.Secondly,the explicit expression of Joule–Thomson coefficient is obtained from the basic formulas of the pressure,the volume,the entropy and the temperature.Then,we obtain the inversion curves in terms of charge Q and parameter λ.Furthermore,we analyse the isenthalpic curve in T–P graph with the cooling–heating region determined by the inversion curve.At last,we derive the ratio of minimum inversion temperature to critical temperature and compare the result with that in the RN-AdS case.

Keywords: Joule–Thomson expansion,black hole,perfect fluid dark matter

1.Introduction

Since Bekenstein and Hawking’s first study,black holes as thermodynamic systems have been an interesting research subject in the theory of gravity[1–5].The thermodynamic properties of AdS black holes have been studied since the early work of Hawking–Page phase transition[5].Another notable thing is that the dark energy which corresponds to the cosmological constant is introduced to the first law of black hole thermodynamics.Particularly,the negative cosmological constant can be interpreted as thermodynamic pressure and treated as a thermodynamic variable [6,7].And its conjugate quantity can be regarded as volume of black holes once the thermodynamic laws of black hole mechanics were generalised[8].Based on this idea,the charged AdS black hole thermodynamic properties and plenty of other characteristics were successfully investigated [9–24].

In the extended phase space(including P and V terms in the black hole thermodynamics),the phase transition of charged AdS black holes is remarkably coincident with van der Waals liquid–gas phase transition [18,25,26].An interesting aspect of van der Waals system is Joule–Thomson expansion,which indicates that the heating and cooling zones emerge through the throttling process.Since the phase structure and the critical behaviour of AdS black holes are similar to van der Waals system,the Joule–Thomson expansion of AdS black holes was firstly investigated by Ökcü and Aydıner [27].In the extended thermodynamics,one identifies the enthalpy H with the mass of the black hole[8].Joule–Thomson expansion is characterised by the invariance of enthalpy,so the throttling process is also an isenthalpic process.The slope of the isenthalpic curves equals the Joule–Thomson coefficient μ which determines the final change of temperature in this system.One can use the sign of the Joule–Thomson coefficient to determine the heating and cooling zone.Subsequently,there are many studies on Joule–Thomson expansion in various black holes [28–49].

The standard model of cosmology suggests that our universe is compiled of dark matter,dark energy and baryonic matter.And the existence of dark matter and dark energy has been proved by several experiments and observations [50].There exists a greater velocity scattered of stars in the galactic plane than expected which means that more mass is required and it is thought to be provided by dark matter [51,52].Therefore,it is meaningful to consider the black hole solutions surrounded by dark matter or dark energy.As one of the dark matter candidates,the perfect fluid dark matter (PFDM)has been considered in [53,54].A new class of solutions of the Einstein equations was presented by applying the perfect fluid relations,and a new logarithmic term was introduced to explain the observed asymptotic behaviour at large distance.While astrophysical observations show that there exists a supermassive black hole surrounded by dark matter halo[55,56] and many black hole solutions within dark matter have been proposed.Particularly,spherically symmetric black hole solutions surrounded by PFDM have been obtained in[54,57–59].Plenty of properties about this kind of black holes were discussed in[60–71].We consider the existence of dark matter in the observable universe,and the interesting perfect fluid model helps to study the influence of dark matter on various properties of black holes.Especially,we are interested in the Joule–Thomson expansion of the black hole and curious about the effect of PFDM on it.

This paper is organised as follows.In section 2,we investigate the thermodynamic properties of RN-AdS black holes immersed in PFDM.In section 3,we discuss the Joule–Thomson expansion of this kind of black holes,which includes the Joule–Thomson coefficient,the inversion curves and the isenthalpic curves.Furthermore,we compare the critical temperature with the minimum of inversion temperature,while the influence of the PFDM parameter λ and charge Q on inversion curves is also discussed.Finally,we discuss our results in section 4.

2.RN-AdS black hole immersed in PFDM

The minimum coupling of dark matter field with gravity,electromagnetic field and cosmological constant is described by the action [54,59,72]

where G is the gravitational constant,Λ is the cosmological constant,Fμνis the electromagnetic tensor andLDMis the Lagrangian which is related to the density of PFDM.

The spacetime metric of static and spherically symmetric RN-AdS black holes immersed in PFDM is defined as[60,72]

where dΩ2= dθ2+sin2θdφ2and f(r) is given by

with Q being the charge of the black hole and λ is related to the dark matter density and pressure.This metric reduces to the RN-AdS black hole whenλ= 0.For the given spacetime metric in the case whereλ≠0,the stress energy–momentum tensor of the dark matter whose distribution is like anisotropic perfect fluid which reads as

where the density,the radial and the tangential pressures are given by [67]

Note that for a PFDM distribution we shall restrict ourselves to the caseλ> 0 which gives positive energy density.

The cosmological constant Λ is often parameterised by the AdS radius l according to

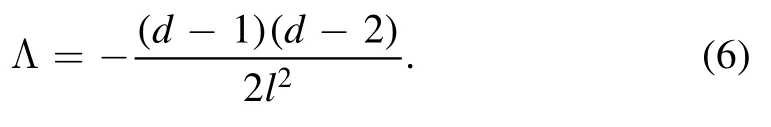
And the negative cosmological constant is related to thermodynamic pressure P

The entropy of the black hole at the horizon is given by

The event horizonr+is the solution off(r+) =0,which indicates the black hole mass M can be expressed in event horizon+ras

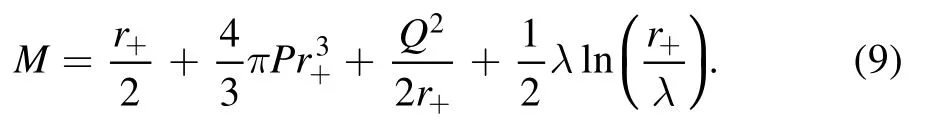
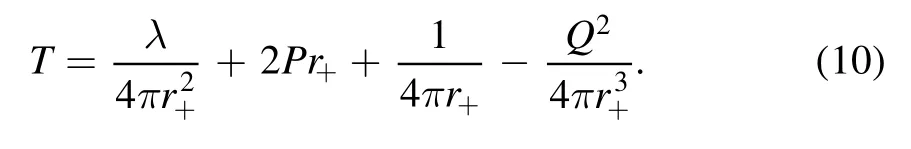
The first law of thermodynamics can be expressed as [72]

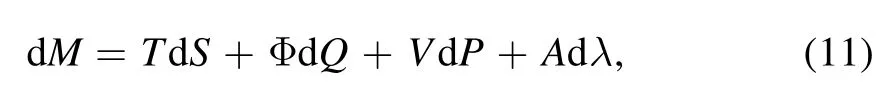
where the thermodynamic volume V of the black hole,the electrostatic potential Φ and the conjugate quantity A are given by

The Hawking temperature T versus event horizon+rand entropy S are shown in figure 1 for different values of Q withλ= 1 and P = 0.075.The Hawking temperature is zero corresponding to the extreme black hole.When the temperature is positive and Q,P and λ are fixed,the slope the isobar numerically equals to the partial derivativeand it determines the sign of specific heat capacity (CP=And figure 1 implies the similarity between this kind of black holes with van der Waals system [14,21,42].

Next,we will study the critical behaviour of the black hole.From equation (10),we get the expression of pressure

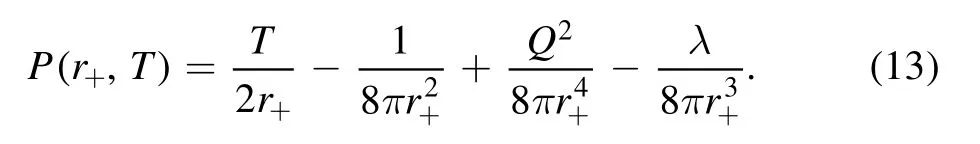
Figure 1.The temperature T of RN-AdS black hole immersed in PFDM versus +r and S for different values of Q,whereλ = 1 and P = 0.075.

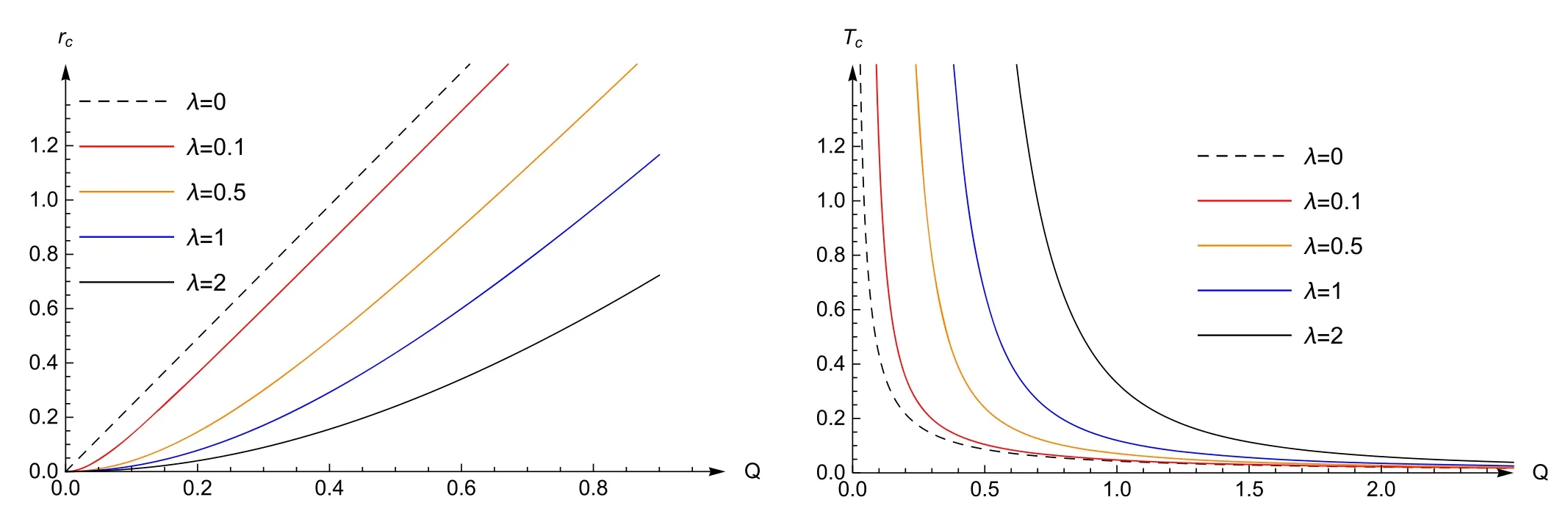
Figure 2.Critical radius rc and critical temperature Tc versus Q of RN-AdS black hole immersed in PFDM.

At the critical point,we have

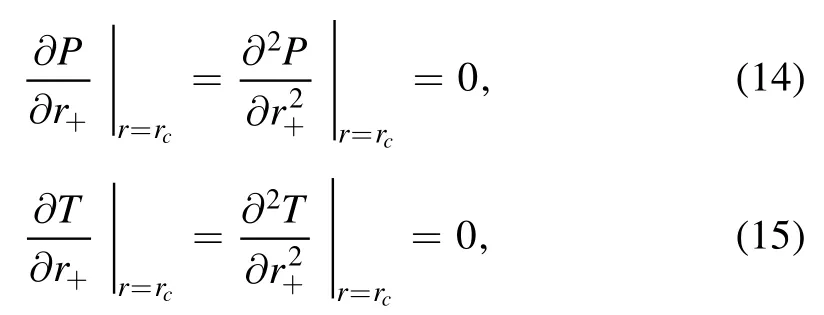
so the critical point rcis obtained as

When the PFDM parameterλ= 0,the critical radius reduces towhich is the same with that of RN-AdS black holes[18].By using the equations(10),(13)and(16),we can determine the critical temperature Tcand critical pressure Pc,

The critical temperature and critical pressure of RN-AdS black hole are[18],which is consistent with equations (17) and (18) whenλ= 0.The critical variables depend on the charge Q and the parameter λ.Here we focus on the critical temperature because it will be useful to analyse the Joule–Thomson expansion of the black hole in the next section.One can plot the critical point rcand the critical temperature Tcwith varying Q and λ in figure 2.The critical temperature decreases while the charge Q increases.If the temperature is less than the critical temperature Tc,the black hole can undergo a first-order phase transition between the small black hole and the large black hole,which is similar to the van der Waals fluids.Furthermore,one can go back to the RN-AdS case as in [27] by taking the limitλ→0.When the charge Q is fixed,the critical radius decreases with the increase of PFDM factor λ,and the critical temperature rises with the increase of parameter λ.Thus,the influence of PFDM on the critical behaviour of RNAdS black holes is obvious.

We derive the thermodynamic definitions of RN-AdS black holes immersed in PFDM,and we study the critical behaviour of the black hole.In the next section,we investigate the Joule–Thomson expansion of RN-AdS black holes immersed in PFDM.

3.Joule–Thomson expansion

The instability of the black holes appears in the process of the Joule–Thomson expansion.And we introduce Joule–Thomson coefficient μ to determines the cooling and heating phases of the isenthalpic expansion.

The specific heat at constant pressure for the black holes is obtained by

Regarding parametersQ,P,λas constant and substituting the equations (12) and (10) into (19),we have the isobaric heat capacity of this black hole

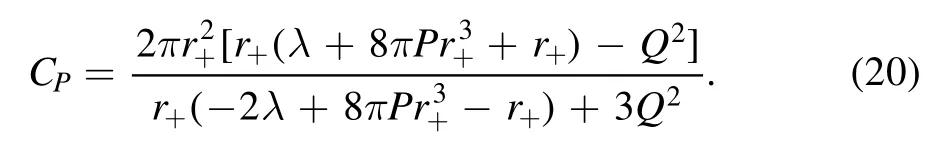
For the Joule–Thomson expansion of the van der Waals fluids,the fluids pass through a porous plug from one side to the other with pressure declining during the throttling process.Therefore,we apply this corresponding concept to black hole thermodynamics and the enthalpy M of the black hole keeps constant throughout this process.Besides,the partial differential of temperature versus pressure of the black hole is defined as Joule–Thomson coefficient μ,

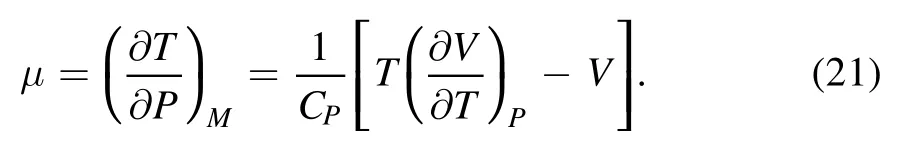
By substituting equations (10),(20) and (12) into (21),we obtain

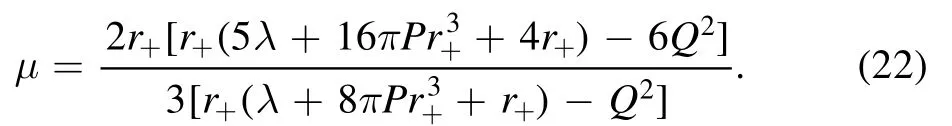
The Joule–Thomson coefficient μ versus the horizon+ris shown in figure 3 where the parameterλ= 0,0.5,1 and 2,pressure P = 0.075 and charges Q = 0.7.There exists a divergence point and a zero point at different values of λ and the influence of PFDM on the Joule–Thomson coefficient is apparent.The divergence point here reveals the information of Hawking temperature and corresponds to the extremal black hole,and it is clear that the divergence point of the blue curve in figure 3 is consistent with the zero points of Hawking temperature in figure 1 in the case of Q = 0.7.

Therefore,we are only interested in the right part of figure 3 where the temperature is above zero.Besides,the Joule–Thomson coefficient of RN-AdS black hole immersed in PFDM can be both positive and negative,which means that there remain both cooling and heating stages.Forμ> 0,the temperature of the black hole decreases,in other words the black hole is cooling down when the pressure goes down in the process of throttling.On the contrary,μ< 0 means that the black hole heats up after the throttling process.So there are inversion points at which the cooling–heating transition occurs whenμ= 0 and the temperature of the black hole atμ= 0 is called the inversion temperature.

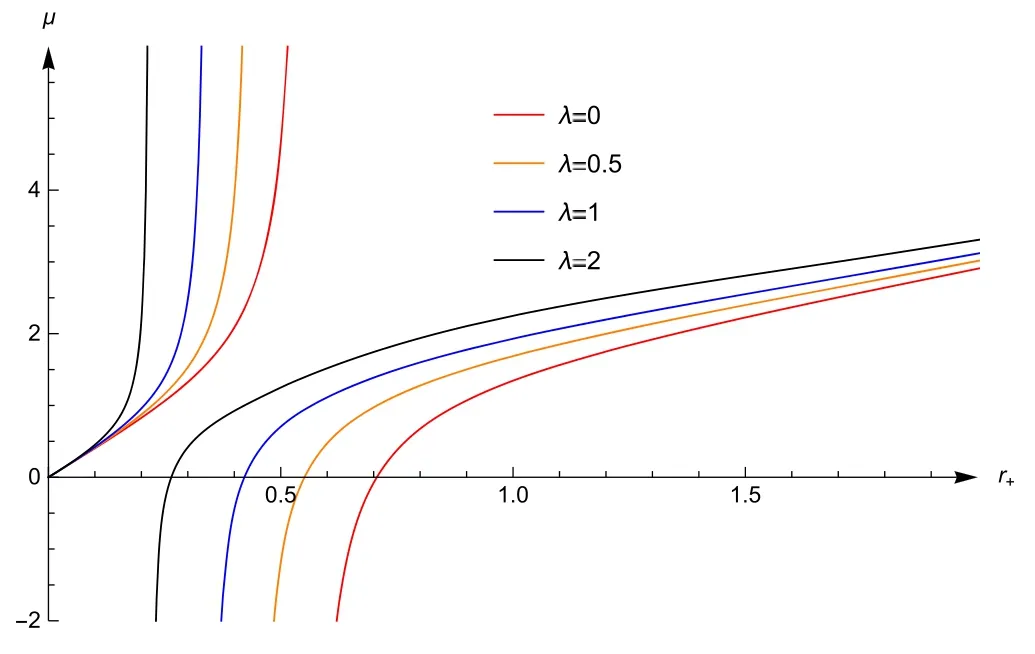
Figure 3.Joule–Thomson coefficient μ of RN-AdS black hole immersed in PDFM for Q = 0.7,P = 0.075 andλ = 0,0.5,1 and 2.

One can obtain the inversion temperaturebyμ= 0.While using the equations (12) and (10),inversion temperature Tiin terms of inversion pressure Piis expressed as

Bringing this equation into equation(13)atP=Pi,we derive

From the above results,the inversion curves Ti–Piin figure 4 with different Q and λ are plotted,which are similar to the case of charged RN-AdS black holes [27].At low pressure,the inversion temperature Tidecreases with the increase of charge Q and increases with the increase of λ.This phenomenon is just the opposite of high-pressure situation.In addition,there are some numerical effects of PFDM on the inversion temperature when other thermal quantities remain unchanged.We find the density of PFDM and the charge have the opposite effect on the inversion curves.It can be observed that the inversion temperature still increases monotonically with the growth of inversion pressure like RN-AdS black holes.Meanwhile,the inversion curves are not closed which differs from the van der Waals fluids.When the inversion pressure equals zero,the inversion temperature reaches a minimum value.And the minimun inversion temperature increases with the charge Q and decreases with the PFDM factor λ.

The Joule–Thomson expansion occurs in the isenthalpic process.And for a black hole,enthalpy is mass M.The isenthalpic curves can be obtained from equations (9) and(13)in figure 5 where inversion curves and isenthalpic curves are both presented.

The intersections of the black inversion curves and the blue isenthalpic curves coincide with the extreme points of isenthalpic curves.And the isenthalpic curves have positive slopes above the inversion curves indicating that cooling occurs.And the isenthalpic curves have negative slopes under the inversion curves indicating that the heating occurs.We can conclude that the region above this inversion curve is the cooling zone while the one below is the heating zone.The temperature rises when the pressure goes down in the heating zone.In contrast,the temperature is lower with reducing pressure in the cooling zone.Moreover,comparing the four graphs,the temperature and pressure decrease with larger charge Q or smaller λ,and PFDM statistically have an influences the isenthalpic curves.

Figure 4.Inversion curves of RN-AdS black hole immersed in PFDM.

Figure 5.The isenthalpic curves of RN-AdS black hole immersed in PFDM.From bottom to top,the isenthalpic curves correspond to increasing values of M.

Furthermore,one can obtain the minimum inversion temperaturecorresponding to Pi= 0 as

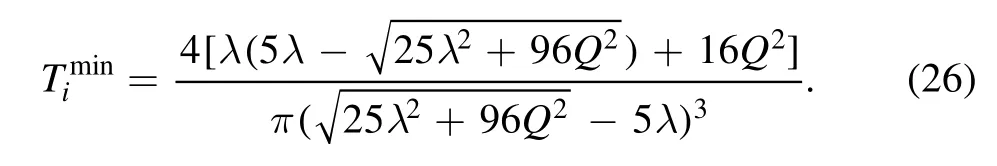
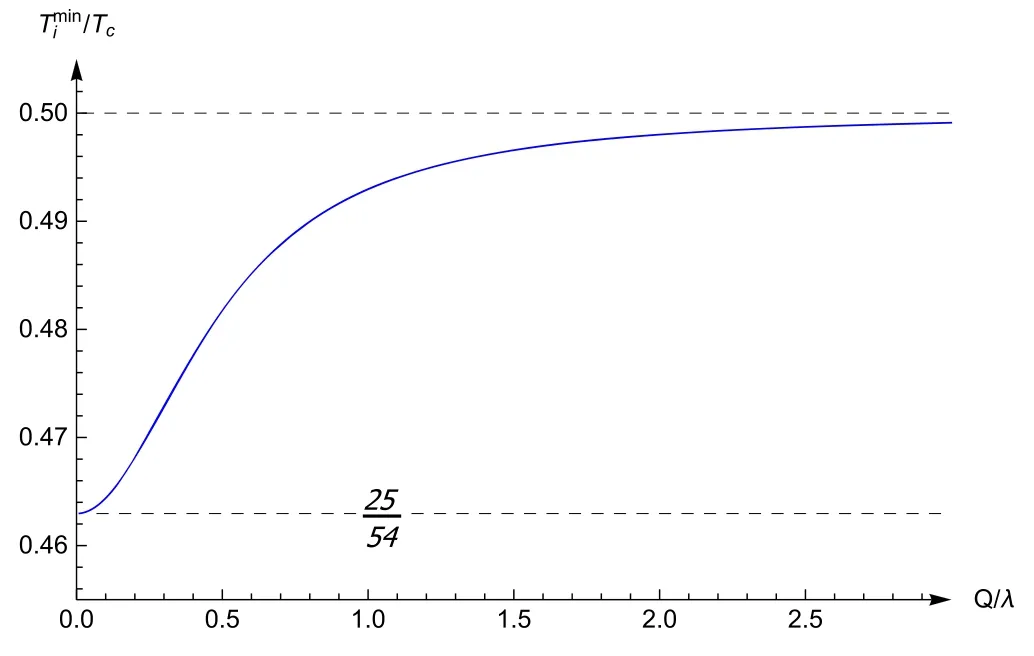
Figure 6.The ratio Tc of RN-AdS black hole immersed in PFDM.

The ratio of inversion temperature to critical temperatureis obtained according to equations (26) and (17)

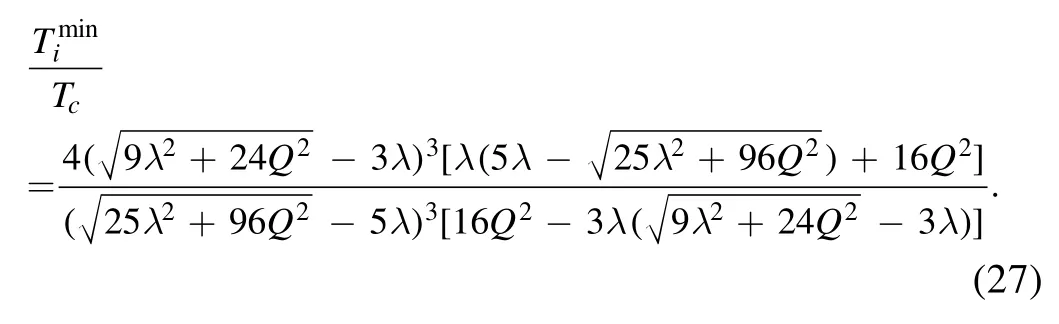
It has been shown in[27]that the ratio for the RN-AdS black holes equals 1/2,but in the case of PFDM,this value should be corrected.The ratio grows with increasingand the ratio versusis shown in figure 6.When→0,in other words,Q→0 orλ→∞,the ratio has a leading term which is 25/54

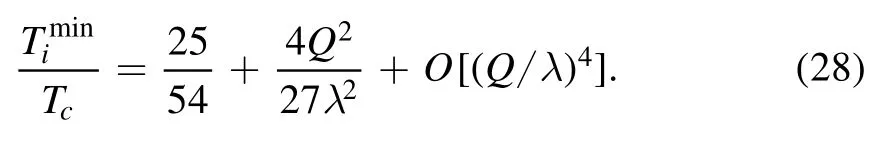
Furthermore,by taking the limitλ→0,the ratio is approaching 1/2 corresponding to the RN-AdS case [27].

4.Conclusion

In this paper,we discuss the Joule–Thomson expansion for RN-AdS black holes immersed in PFDM.In the extended phase space,the cosmological constant is identified with the pressure and its conjugate quantity is the thermodynamic volume.Since the black hole mass is interpreted as enthalpy,an isenthalpic process can be applied to investigate the heating and cooling region of this system accoding to the Joule–Thomson expansion.By analysing the Joule–Thomson coefficient μ,we find the divergent point of μ coincides with the point where Hawking temperature is zero which corresponds to the extremal black hole case.Moreover,we plot the inversion curves and the corresponding isenthalpic curves in the T–P plane with different λ and Q.From the results mentioned above,we can conclude how the PFDM parameter λ affects the thermodynamic quantities,Joule–Thomson coefficient and the inversion temperature.In addition,one can easily go back to the RN-AdS case by taking the limitλ→0.We also discuss the isenthalpic curves with different values of M during the throttling process.

Furthermore,we derive the ratio of minimum inversion temperature to critical temperature and the inversion curves in terms of charge Q and parameter λ.It has been shown in[27]that the ratio for the RN-AdS black holes is equal to 1/2,while in the case of PFDM,the ratio depends on both charge Q and parameter λ.In our study,the ratio ofis rewritten in terms ofQ/λ.In figure 6,the ratio is about 25/54 for smallQ/λ,and the ratio approaches 1/2 for enormousQ/λ,which is the same with that of RN-AdS black holes.

Acknowledgments

We are grateful to thank Shihao Bi and Feiyu Yao for useful discussions.This work is supported by NSFC (Grant No.11947408 and 11875196).

ORCID iDs