Structural,mechanical,electronic properties,and Debye temperature of quaternary carbide Ti3NiAl2C ceramics under high pressure:A first-principles study∗
2021-03-19DiyouJiang姜迪友WenboXiao肖文波andSanqiuLiu刘三秋
Diyou Jiang(姜迪友), Wenbo Xiao(肖文波), and Sanqiu Liu(刘三秋)
1Key Laboratory of Nondestructive Testing,Ministry of Education,Nanchang Hangkong University,Nanchang 330063,China
2Fujian Science&Technology Innovation Laboratory for Energy Devices of China(21C-LAB),Ningde 352100,China
3Department of Physics,Nanchang University,Nanchang 330047,China
Keywords: quaternary carbide Ti3NiAl2C ceramics, structural properties, mechanical properties, electronic properties,Debye temperature,first-principles
1. Introduction
Mn+1AXnphases(M represents the early transition metal of B group, n represents 1/2/3, A stands for an element of A group, and X is C or N) have both metallic and ceramic properties with its hexagonal close-packed structure, which have attracted great interest from researchers due to its excellent performances such as high melting point, high temperature stability,high temperature resistant to oxidation,high corrosion resistance,high elastic modulus,significant machinability, excellent resistant to thermal shock, good thermal and electrical conductivity, low density and micro-ductility at room temperature.[1-10]In addition, Mn+1AXnphases also exhibit good radiation resistance,[11]especially at high temperatures,[12-15]and are considered as a potential structural material for nuclear fission and fusion reactors.[16-18]In the Mn+1AXnphases,many materials have been studied,such as Ti2AlC, [19,20] Ti3AlC2[21,22]and Ti3SiC2[23,24]compounds.
On the other hand,the composite carbides of Ti2A1C and Ti3A1C formulations have high efficiency when used as abrasives for glass polishing. With nickel proposed as a possible binder, the research on the Ti-Ni-Al-C quaternary system began to arouse the interest of researchers. The quaternary system: titanium-nickel-aluminum-carbon (Ti3NiAl2C)was established for 1100◦C (quench), and a quaternary ηcarbide with a lattice parameter of a=11.40-11.463 ˚A was found to exist.[25]The H-phase Ti2AlC and the perovskite phase Ti3AlC are no longer stable in the presence of minor amounts of nickel.[25]Cubic Ti3NiAl2C compounds as quaternary carbide ceramics[25]may also have the characteristics of the Mn+1AXnphases. So far,however,this material has not been further investigated,especially in theory.
In addition,high pressure can cause a material to undergo phase changes that change its chemical and physical properties, such as structural, mechanical, thermodynamic, optical, electronic, and magnetic properties.[26-35]At the same time, computational materials science can be used to investigate the structure performance relationship and design materials since the traditional experimental design is expensive and time-consuming. Computer simulation can improve the chemical and physical properties of specific compounds and greatly reduce the number of components to be prepared and characterized.[36-43]
In this paper,our purpose is to improve the chemical and physical properties of cubic Ti3NiAl2C compounds through pressurization based on density functional theory. Therefore,we focus on investigating the effect of pressure on the mechanical properties of quaternary carbide Ti3NiAl2C ceramics.
2. Model and computational details
Figure 1 shows the crystal structure of cubic Ti3NiAl2C compounds, which belongs to the Fd-3m space group. The model of cubic Ti3NiAl2C compounds consists of 112 atoms,which including 16 C, 32 Al, 16 Ni, and 48 Ti atoms. The valence electron of C, Al, Ni, and Ti atoms is C-2s22p2, Al-3s23p1, Ni-4s23d8, and Ti-4s23d2, respectively. Using VASP software,the first principles method according to density functional theory (DFT) along with the plane-wave pseudopotential is used to complete the current calculations.[44,45]According to the projector augmented wave method(PAW),[46]it can characterize the relationship between core ions and valence electrons. The generalized gradient approximation(GGA)of Perdew and Wang (PW91) is used to calculate the exchangecorrelation potentials.[47]3×3×3 Monkhorst-Pack meshes as sampling k-point in Brillouin-zone.[48]All calculated plane wave cutoff energy is set to 430 eV,and further increasing the cutoff energy has little effect on the energy. Gaussian smearing method is used for all calculations,and its smearing width is 0.05 eV.
According to the relationship between pressure and volume, the Birch-Murnaghan equation of state (EOS) can be expressed as[28,35,49]
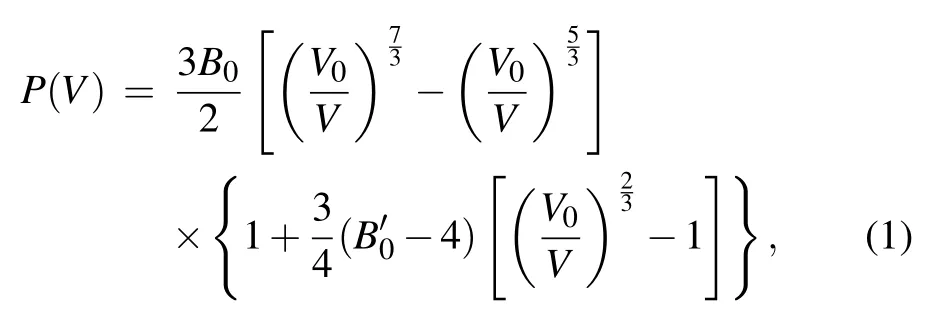


According to Voigt-Reuss-Hill averaging scheme,[50-53]a series of required mechanical parameters can be obtained by fitting the elastic constants, such as bulk modulus (B),Young’s modulus(E),shear modulus(G),Poisson’s ratio(ν),anisotropy coefficients (A), and Cauchy pressure (C′). The equation constructed can be expressed as
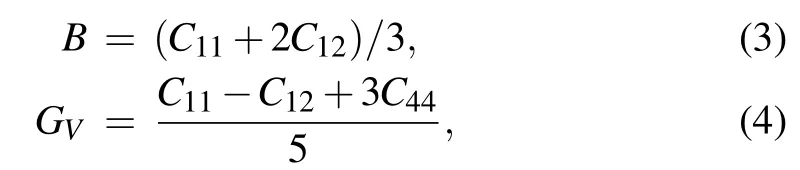

Debye temperature (ΘD) is an important parameter for studying the thermodynamic properties. Therefore, it is necessary to explore the Debye temperature of quaternary carbide Ti3NiAl2C ceramics at different pressures.Debye temperature can be expressed as[54]

where kBis the Boltzmann constant,h is the Planck constant,NAis the Avogadro number, ρ is the mass density, m is the molecular weight,n is total number of atoms per formula,and vmis the average sound velocity.
The average sound velocity (vm) can be acquired by the following equation:[54]
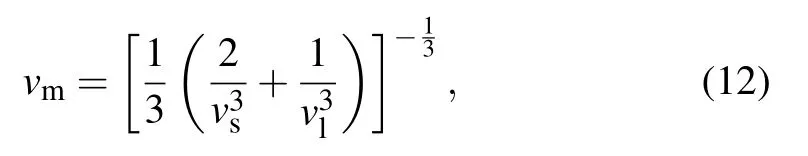
where vlis the longitudinal sound velocity and vsis the shear sound velocity,which can be calculated based on the B and G.The equation constructed is as follows:[55]
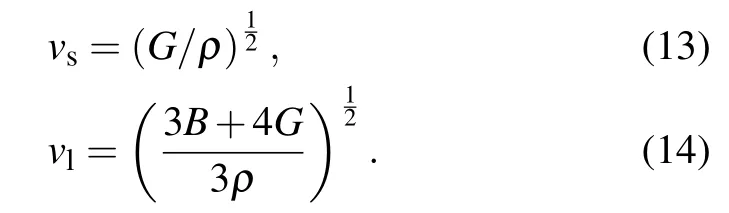
3. Results and discussion
3.1. Structural properties
As shown in Fig.1, we pressurized and optimized the crystal structure of quaternary carbide Ti3NiAl2C ceramics with a pressure range of 0-110 GPa and an interval of 10 GPa.At the same time, the optimized atomic positions and crystal configurations are used as the model for the study of mechanical properties.
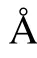
cubic lattice at different pressures. The lattice constants of quaternary carbide Ti3NiAl2C ceramics decrease with the increase of pressure due to the shortened bond lengths,as shown in Fig.2.
Table 1. The lattice constants a, b and , volume of cubic Ti3NiAl2C compounds at different pressures, and the bulk modulus B0(GPa)and its pressure derivative at 0 GPa.

Table 1. The lattice constants a, b and , volume of cubic Ti3NiAl2C compounds at different pressures, and the bulk modulus B0(GPa)and its pressure derivative at 0 GPa.
Pressure(GPa) a b c V B0 B′0 (GPa)0 11.454 11.454 11.454 1502.69 164.58 3.47552 10 11.244 11.244 11.244 1421.54 20 11.080 11.080 11.080 1360.35 30 10.939 10.939 10.939 1309.13 40 10.819 10.819 10.819 1266.39 50 10.713 10.713 10.713 1229.41 60 10.617 10.617 10.617 1196.81 70 10.531 10.531 10.531 1167.80 80 10.451 10.451 10.451 1141.42 90 10.380 10.380 10.380 1118.27 100 10.313 10.313 10.313 1096.77 110 10.247 10.247 10.247 1075.79

Fig.1. Crystal structure model of cubic Ti3NiAl2C compounds. Black,pink,grey,and blue balls are C,Al,Ti,and Ni atoms,respectively.

Fig.2. The bond lengths of quaternary carbide Ti3NiAl2C ceramics at different pressures.
The bond lengths of quaternary carbide Ti3NiAl2C ceramics decrease with the increasing pressure. At zero pressure,there are three kinds of bonds,namely Ti-Al,Ni-Al,and Ti-C bonds.At pressures of 20 GPa,30 GPa,40 GPa,60 GPa,and 70 GPa,the new bond lengths of Ti-Ni,Ti-Ti,Al-Al,Ti-Al, and Ti-Ti are produced, respectively. At the same time,we can see that the Ti-Ti and Ti-Al bonds appear twice in the process of pressurization. It indicates that pressurization can lead to the diversity of the bonding of quaternary carbide Ti3NiAl2C ceramics.
Based on Eqs. (1) and (2), the relationship between volume and pressure can be expressed by the pressure-volume curve. At the same time, we can get these results by fitting the Birch-Murnaghan equation of state. Figure 3 plotted the pressure-volume curve of quaternary carbide Ti3NiAl2C ceramics,and the volume decreases with the increasing pressure.

Fig.3.Birch-Murnaghan fitting curve of quaternary carbide Ti3NiAl2C ceramics based on volume(˚A3)and pressure(GPa).
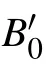
As shown in Fig.4,we can normalize the lattice constant and volume of quaternary carbide Ti3NiAl2C ceramics to investigate the effect of pressure on the cell structure. We can see that the normalization constants of the lattice constant and volume decrease with the increase of pressure. Obviously,the normalization constant of the volume decreases faster. However, we can also see that quaternary carbide Ti3NiAl2C ceramics cannot be compressed endlessly. The reason is that as the pressure increases, the volume is continuously compressed, and the bond length between atoms is shortened,which leads to the increase of repulsive force between atoms and makes the crystal difficult to be compressed. We can see from Fig.4 that the volume of quaternary carbide Ti3NiAl2C ceramics is compressed to about 72%.
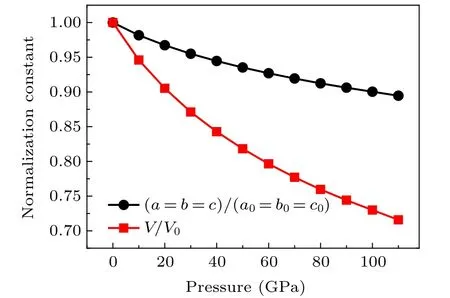
Fig.4. The normalization parameter of the volumes and lattice constants of cubic Ti3NiAl2C compounds at different pressures.

Table 2. Elastic constants(Cij,in GPa),Young’s modulus(E,in GPa),shear modulus(G,in GPa),bulk modulus(B,in GPa),B/G ratio,Poisson’s ratio(ν),Cauchy pressure(C′,in GPa)and anisotropic coefficients(A)of cubic Ti3NiAl2C compound at different pressures(GPa).
3.2. Charge transfer
The charge transfer between atoms can reduce the energy of the compounds to achieve a stable structure. Based on electronegativity analysis, the Ni and C atoms of quaternary carbide Ti3NiAl2C ceramics can gain charge, while the Ti and Al atoms can lose charge. Therefore, Ti and Al can produce positive charges, and C and Ni can produce negative charges.
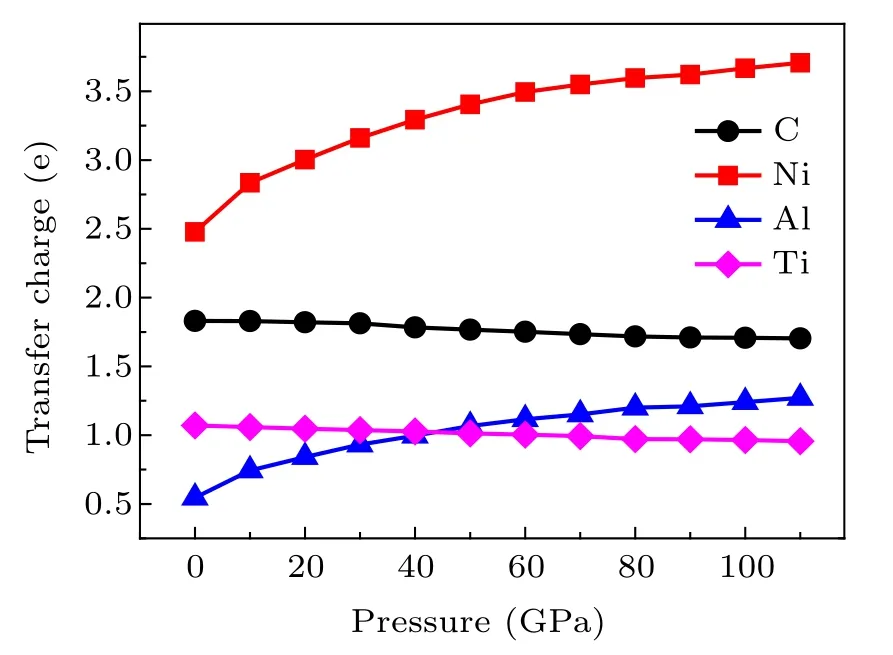
Fig.5. The transfer charges of quaternary carbide Ti3NiAl2C ceramics at different pressures.
As shown in Fig.5,we investigated the effect of pressure on the charge transfer of quaternary carbide Ti3NiAl2C ceramics.According to the Bader charge calculation,we can see that the charge gained by the C atom decreases with the increasing pressure,but the change is not obvious,and the charge transfer is relatively stable. The charge gained by Ni atoms obviously increases with the increasing pressure. Similarly,we can also clearly know that the charge loss of Ti atom decreases with the increase of pressure, but the charge loss is not obvious,and the charge transfer is also relatively stable. However,the charge loss of Al atom obviously increases with the pressure.These results show that pressure has a greater effect on the charge transfer of Ni and Al atoms in cubic Ti3NiAl2C compounds,but it has a smaller effect on the charge transfer of C and Ti atoms. How much is the charge transfer? According to Marcus’s charge transfer theory,it may be caused by orbital interaction.
3.3. Mechanical properties
Firstly, we fitted the elastic constants of quaternary carbide Ti3NiAl2C ceramics at different pressures, and then exported the mechanical parameters such as Poisson’s ratio,Cauchy pressure, elastic modulus, and anisotropic factors based on the Eqs.(3)-(10),as shown in Table 2.
The elastic constant C11is often used to characterize the stiffness of compounds. It can be seen from Table 2 that the C11increases with the pressure,indicating that the stiffness of quaternary carbide Ti3NiAl2C ceramics can be enhanced by pressurization.C12is generally used to characterize resistance to lateral deformation. Similarly, it can also be seen that the C12increases with the pressure,indicating that pressurization can also increase resistance to lateral deformation. The reason is that pressure makes the bond length shorter,the interaction between atoms is strengthened, and the bond energy also increases,leading to an improvement of the mechanical properties of quaternary carbide Ti3NiAl2C ceramics. At the same time,as shown in Table 2,we can also see that the elastic constants of quaternary carbide Ti3NiAl2C ceramics at 50-60 GPa are equivalent to that of pure tungsten(Tungsten is considered the most promising first wall material).[56-66]
In addition,the relations(C11−C12)>0,C11>0,C44>0, (C11+2C12)>0[67]serves as the criterion for determining the mechanical stability of cubic systems. We can deduce that the elastic constants of quaternary carbide Ti3NiAl2C ceramics at different pressures obviously meet the stability criteria. It indicates that these structures are mechanically stable.Meanwhile,the phonon dispersion of cubic Ti3NiAl2C ceramics(the primitive cell with 28 atoms)at zero pressure is calculated based on density functional perturbation theory(DFPT),as shown in Fig.6. It is obvious that cubic Ti3NiAl2C ceramics is dynamically stable.
Bulk modulus is generally used to evaluate the material’s resistance to deformation under external forces.[68,69]We can see from Table 2 that the bulk modulus of quaternary carbide Ti3NiAl2C ceramics increases with the increasing pressure,indicating that pressurization can improve the ability to resist deformation. At the same time, it is found that the bulk modulus (163.3 GPa) at zero pressure is higher than Ti2AlC(135.8 GPa)[62]and Ti3AlC2(156.2 GPa)[62]ceramics but lower than Ti3SiC2(185.3 GPa)[63]ceramics, which means that cubic Ti3NiAl2C ceramics has certain advanced properties. Shear modulus is generally used to characterize the material’s resistance to shear deformation.[68,69]We can also see from Table 2 that the shear modulus increases with the pressure, indicating that pressurization can improve the ability of quaternary carbide Ti3NiAl2C ceramics to resist shear deformation. Young’s modulus corresponds to that of C11, which also characterizes the stiffness of materials. Similarly,we can also see from Table 2 that Young’s modulus increases with the pressure,which is equivalent to the conclusion of C11.

Fig.6. The phonon dispersion curves of cubic Ti3NiAl2C ceramics at zero pressure is calculated based on DFPT.
According to the Pugh theory,[69]the (B/G) ratio can characterize the ductile and brittle behavior of solid materials. 1.75 is usually used to determine whether a material is ductile or brittle. We can see from Table 2 that the B/G value increases with the increase of pressure, indicating that pressurization can improve the ductility of quaternary carbide Ti3NiAl2C ceramics. At the same time, we can also see that the B/G values are all lower than 1.75 when the pressure is below 40 GPa, indicating that quaternary carbide Ti3NiAl2C ceramics has a brittle phase. However, the brittle phase of the cubic Ti3NiAl2C compound changes from brittle to ductile when the pressure reaches 40 GPa. This excellent performance is not available in Mn+1AXnphases, such as Ti2AlC,[70]Ti3AlC2,[70,71]and Ti3SiC2[71]compounds at different pressures, which fully reflects the advanced nature of quaternary carbide Ti3NiAl2C ceramics. Poisson’s ratio is generally used to evaluate the plasticity of solid materials.The quality of plasticity is proportional to Poisson’s ratio.[72]Obviously, pressurization can improve the plasticity of quaternary carbide Ti3NiAl2C ceramics.
According to the characteristic parameter of Cauchy pressure, it can be determined whether the material has metallic or covalent bond behavior. The positive/negative values of Cauchy pressure indicate that the material has metallic/covalent bond behavior, respectively, as well as ductility/brittleness.[28,73]As shown in Table 2,we can see that Cauchy pressure increases with the pressure, indicating that pressurization can improve the metallic bond behavior of quaternary carbide Ti3NiAl2C ceramics.At the same time,we can also see that quaternary carbide Ti3NiAl2C ceramics changes from covalent bond to metallic bond at pressure to 20 GPa.Eventually,metallic bonds dominate in the quaternary carbide Ti3NiAl2C ceramics.
The material’s anisotropy is quantitatively characterized by the anisotropy factor (A). The values of A generally have three types: A >1,A <1,and A=1. A >1 or A <1 indicates that the material has anisotropy, and the greater the deviation from 1, the more severe the anisotropy, and A=1 indicates that the material is isotropic. We can see from Table 2 that the A values increase with the pressure, which indicates that pressurization may aggravate the degree of anisotropy of quaternary carbide Ti3NiAl2C ceramics.
3.4. Electronic properties
To further understand the bonding characteristics of quaternary carbide Ti3NiAl2C ceramics at different pressures and investigate the mechanical properties and structural stability mechanisms,the total density of states(TDOS)of quaternary carbide Ti3NiAl2C ceramics at different pressures (0 GPa,50 GPa, 80 GPa, 110 GPa) is plotted in Fig.7. We can see from Fig.7(a) that the TDOS at the Fermi level has no gap,indicating that quaternary carbide Ti3NiAl2C ceramics at different pressures exhibits metallic characteristics. We can also see from Fig.7(a)that the shape of the TDOS curve changed slightly,which indicates that the structures of quaternary carbide Ti3NiAl2C ceramics at different pressures did not change drastically,nor did the structural phase change.
However,as the pressure increases,the TDOS of quaternary carbide Ti3NiAl2C ceramics shows a downward trend,which indicates a decreased inter-atomic hybridization energy and weaker inter-atomic hybridization. At the same time, we found that the distance between the valence band and conduction band of the TDOS widens with the pressure, indicating that the delocalization of quaternary carbide Ti3NiAl2C ceramics is enhanced.
In general, the TDOS value at the Fermi level (Df) can indirectly reflect the hardness of the intermetallic,because the hardness is inversely proportional to Df.[74,75]As shown in Fig.7(b), The Dfvalue of quaternary carbide Ti3NiAl2C ceramics at the Fermi level decreases with the increasing pressure,which indicates that the hardness increases with the pressure. It is consistent with the trend of elastic constants in Table 2.
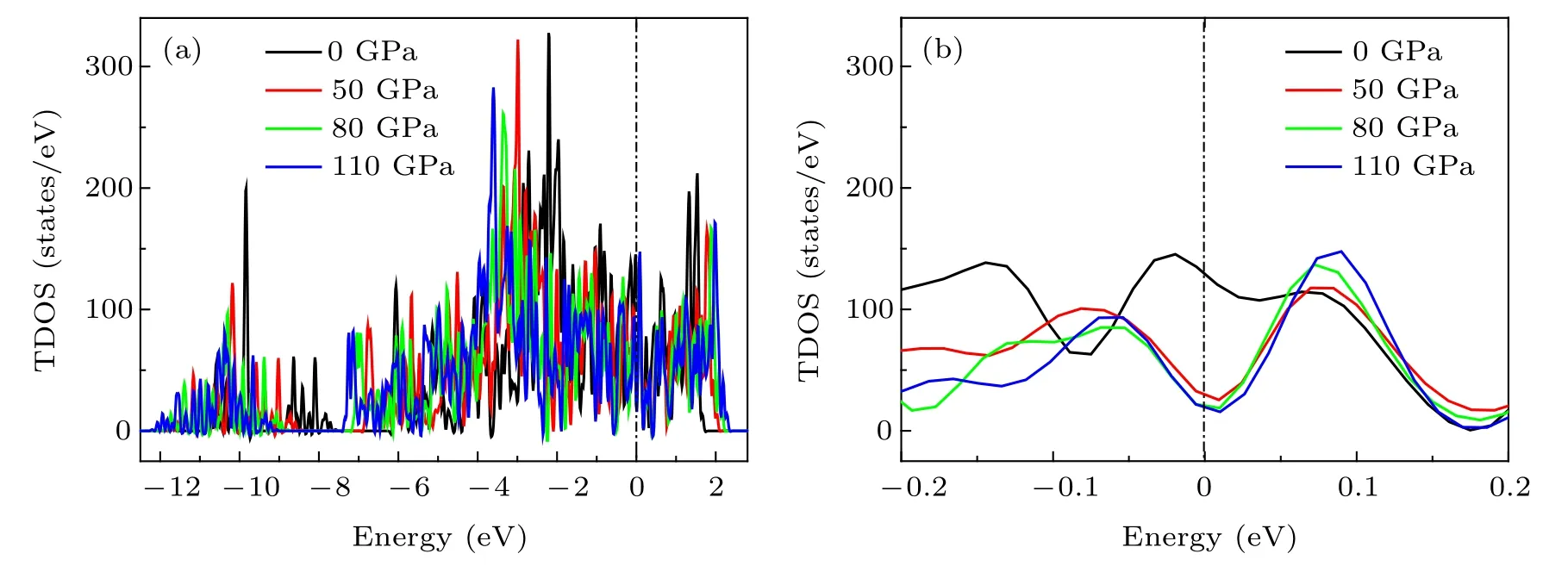
Fig.7. The total density of states of quaternary carbide Ti3NiAl2C ceramics at different pressures(0 GPa,50 GPa,80 GPa,110 GPa).The energy is with respect to the Fermi level.

Table 3. Mass density (ρ, in g·cm−3), Debye temperatures (ΘD, in K), average wave velocity (vm), longitudinal sound velocity (vl), shear sound velocity(vs),melting point(Tm,in K),and hardness(H,in GPa)of quaternary carbide Ti3NiAl2C ceramics at different pressures.
3.5. Debye temperature,melting point,and hardness
Debye temperature(ΘD)comes from the atomic thermal vibration theory of solids, which corresponds to the highest frequency of lattice vibration.ΘDis often used to describe the interatomic bond strength of solid materials.[35,76,77]It is related to many physical quantities of solids, such as hardness,melting point, elasticity, and specific heat. In general, high Debye temperature indicates that solids have high modulus,high melting points and high hardness,and vice versa.
As shown in Table 3, we can see that the ΘDvalues increase with the increase of pressure,indicating that pressurization can enhance the Debye temperature of quaternary carbide Ti3NiAl2C ceramics. It also indicates that pressurization can increase the strength of the interatomic bonding force.
Melting point (Tm) and hardness (H) are also two critical characteristic parameters of solids. At the same time,H is usually used as an important index to evaluate the wear properties of solids.[78]Herein, it is necessary to investigate the two parameters. Based on the elastic constant C11,shear modulus G,and bulk modulus B,the melting point[79]and Vickers hardness[80]formulas are as follows:
As shown in Table 3, the melting point and hardness increase with the increase of pressure,indicating that pressurization can enhance the melting point and hardness of quaternary carbide Ti3NiAl2C ceramics. In addition, the elastic strain failure(H/E)[19]and the plastic strain failure(H3/E2)[19]are usually used to evaluate the wear and resistance to plastic deformation of solids, respectively. It can be seen from Table 3 that the H/E and H3/E2values decrease with the increasing pressure,which indicates that the wear and resistance to plastic deformation of quaternary carbide Ti3NiAl2C ceramics may be weakened under pressure.
4. Summary and conclusion
In this paper,we investigate the structural,electronic,mechanical properties,and Debye temperature of quaternary carbide Ti3NiAl2C ceramics under high pressure according to the first-principles method.The quaternary carbide Ti3NiAl2C ceramics still has a cubic structure under pressure(0-110 GPa).At zero pressure,there are only three kinds of covalent bonds in Ti3NiAl2C:Ti-Al,Ni-Al,and Ti-C.However,at the pressures of 20 GPa, 30 GPa, 40 GPa, 60 GPa, and 70 GPa, new Ti-Ni, Ti-Ti, Al-Al, Ti-Al, and Ti-Ti bonds are formed, respectively. The results show that pressurization may result in the bond diversity of quaternary carbide Ti3NiAl2C ceramics. When the pressure reaches 20 GPa, the covalent bonds turn into metallic bonds. The volume of quaternary carbide Ti3NiAl2C ceramics can be reduced to 72% of the original volume. The effect of pressure on the charge transfer of Ni and Al atoms in quaternary carbide Ti3NiAl2C ceramics is greater than that of C and Ti atoms. The mechanical strength and ductility of quaternary carbide Ti3NiAl2C ceramics can be improved by pressure treatment. At 50-60 GPa, its mechanical strength is comparable to that of pure tungsten, and the material changes from brittleness to ductility. However,the anisotropy of quaternary carbide Ti3NiAl2C ceramics becomes more serious with the increasing pressure. In addition,pressurization can also improve the Debye temperature,melting point, and hardness of quaternary carbide Ti3NiAl2C ceramics,but the wear resistance is decreased.
猜你喜欢
杂志排行
Chinese Physics B的其它文章
- Nonlocal advantage of quantum coherence in a dephasing channel with memory∗
- New DDSCR structure with high holding voltage for robust ESD applications∗
- Nonlinear photoncurrent in transition metal dichalcogenide with warping term under illuminating of light∗
- Modeling and analysis of car-following behavior considering backward-looking effect∗
- DFT study of solvation of Li+/Na+in fluoroethylene carbonate/vinylene carbonate/ethylene sulfite solvents for lithium/sodium-based battery∗
- Multi-layer structures including zigzag sculptured thin films for corrosion protection of AISI 304 stainless steel∗
