Lawson-Mislove问题的一个必要条件

孙涛, 邹志伟

Lawson-Mislove问题的一个必要条件

孙涛1, 邹志伟2

(1. 湖南文理学院 数理学院, 湖南 常德, 415000; 2. 南华大学 数理学院, 湖南 衡阳, 421001)

借助于Dcpo上的Scott拓扑, 引进Scott吸收Dcpo的概念, 并证明了函数空间上Scott拓扑与Isbell拓扑一致的必要条件是该函数空间的值域Dcpo是Scott吸收的。结果表明, Scott吸收性是Lawson-Mislove问题的一个必要性刻画。

函数空间; Isbell 拓扑; Scott 拓扑; Scott吸收Dcpo

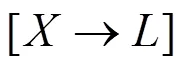
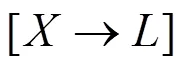
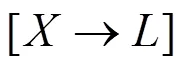
1 基本概念

2 主要结果

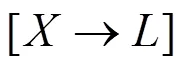
注2 Scott吸收Dcpo的例子很多, 如完备格以及具有最大元的Dcpo等。非Scott吸收Dcpo的例子也容易得到, 如文献[3]中Example3.1与Example3.2所例举的Dcpo。

证明 采用反证法。

3 结论

本文在分析了函数空间上Isbell拓扑与Scott拓扑之结构的基础上证明了二者一致的必要条件是函数空间的值域Dcpo具有Scott吸收性质。

该结果缩小了使得Isbell拓扑与Scott拓扑一致的值域Dcpo的寻找范围。对进一步解决Lawson-Mislove问题具有一定意义。

[1] Lawson J D, Mislove M W. Problems in domain theory and topology, in: Open Problems in Topology [M]. Amsterdam: Elsevier Science Publishers, 1990.

[2] Mill J V, Reed G M. Open Problem in Topology [M]. Amsterdam: North-Holland, 1990.

[3] Liu Y M, Liang J H. Solution to two problem of J.D. Lawson and M. Mislove [J]. Topology and its Application, 1996, 69: 153–164.

[4] Xi X Y, Yang J B. Coincidence of Isbell and Scott topologies on domain function space [J]. Topology and its Application, 2014, 164: 197–206.

[5] Gierz G, Hofmann K H, Keimel K, et al. Continuous lattices and Domains [M]. Cambridge: Cambridge University, 2003.

[6] Schwarg F, Week S. Scott topology, Isbell topology and continuous convergence [J]. Lecture Notes in Pure and Applied Mathematics, 1985, 101: 251–271.

[7] Engelking R. General Topology [M]. Warszawa: Polish Scientifific Publishers, 1977.

[8] Birkhoff G. Lattice Theory [M]. Providence: American Mathematical Society, 1940.

A necessary condition for Lawson-Mislove Problem

Sun Tao1, Zou Zhiwei2

(1. College of Mathematics and Physics, Hunan University of Arts and Science, Changde 415000, China; 2. College of Mathematics and Physics, University of South China, Hengyan 421001, China)

Based on the Scott Topology on Dcpo, the concept of Scott absorbed Dcpo is introduced. And it is proved that if the Scott Topology and the Isbell Topology on a functional space are coincident, then the valued Dcpo in the functional space is Scott absorbed. This result gives a necessary condition for Lawson-Mislove Problem.

functional space; Isbell Topology; Scott Topology; Scott absorbed Dcpo

10.3969/j.issn.1672–6146.2021.01.001

O 189.11

A

1672–6146(2021)01–0001–04

孙涛, suntao5771@163.com。

2020–09–25

国家自然科学基金项目(11901194&11801264); 湖南省自然科学基金(2019JJ50406); 湖南省高校青年骨干教师资助项目。

(责任编校: 张红)