Applying the New Extended Direct Algebraic Method to Solve the Equation of Obliquely Interacting Waves in Shallow Waters
2020-09-28KURTAliTOZARAliandTASBOZANOrkun
KURT Ali, TOZAR Ali, and TASBOZAN Orkun
Applying the New Extended Direct Algebraic Method to Solve the Equation of Obliquely Interacting Waves in Shallow Waters
KURT Ali1), *, TOZAR Ali2), and TASBOZAN Orkun3)
1) Department of Mathematics, Faculty of Science and Art, Pamukkale University, Denizli 20160, Türkiye 2) Department of Physic, Faculty of Science and Art, Mustafa Kemal University, Hatay 31001, Türkiye 3) Department of Mathematics, Faculty of Science and Art, Mustafa Kemal University, Hatay 31001, Türkiye
In this study, the potential Kadomtsev-Petviashvili (pKP) equation, which describes the oblique interaction of surface waves in shallow waters, is solved by the new extended direct algebraic method. The results of the study show that by applying the new direct algebraic method to the pKP equation, the behavior of the obliquely interacting surface waves in two dimensions can be analyzed. This article fairly clarifies the behaviors of surface waves in shallow waters. In the literature, several mathematical models have been developed in attempt to study these behaviors, with nonlinear mathematics being one of the most important steps; however, the investigations are still at a level that can be called ‘baby steps’. Therefore, every study to be carried out in this context is of great importance. Thus, this study will serve as a reference to guide scientists working in this field.
conformable fractional derivative; new extended direct algebraic method; interacting wave equation; shallow water waves
1 Introduction
The behaviors of physical systems, although also defined by nonlinear dynamics, are basically defined by factors such as volume, temperature, pressure, and density. These quantities are some macroscopic projections or reductions of the sum of the whole microscopic state of the system. Although the interactions of the equilibrium systems at the microscopic level are nonlinear, they are greatly beneficial to our understanding of the system. However, time-dependent systems, called dynamical systems, are different from equilibrium systems and are difficult to study. In recent decades, nonlinear science, whichis studying the spatiotemporal behavior of dynamical systems, has attracted much attention (Epstein and Showal- ter, 1996; Xu, 2018; Aliyu, 2019).
Ocean water wave is among the top subjects of nonlinear science that have aroused much interest in recent years(Osborne,2018;Stuhlmeier and Stiassnie,2019).Themath- ematical modeling of the dynamic structure of oceans involves many microscopic variables, and this has led engineers, physicists, and mathematicians to concentrate on oceanic water waves. Several partial differential equations (PDEs) modeling the behavior of the oceanic waves have been proposed. Among these, the Korteweg-de Vries (KdV) equation (Johnson, 2002)
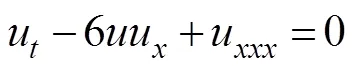
is the most popular PDE, due to its prediction about solitons, which are not only important for oceans but also for fiber optics (Mitschke, 2017), solid state physics (Ovidko and Romanov, 1987), and nuclear physics (Birse, 1990). The mathematical elegance of this equation is indisputable, but its limitation is that it only describes waves propagating in one direction.
Multidirectional propagation and interaction of a wave can lead to very temporary and extreme wave amplification (Pelinovsky, 2000). Oblique wave-wave interactions frequently occur at shores due to the shallow diffraction, refraction, and reflection. Sometimes such interactions of solitary waves can generate a more amplified new wave. A new equation called the Kadomtsev-Petviashvili (KP) equation, which is a generalized form of the KdV equation to 2+1 dimensions, has been proposed to fill the gap of accounting interaction of multidirectional propagated waves (Lan, 2016). Although the Boussinesq equation is more successful in explaining this phenomenon (because the KP equation needs a dominant pro- pagation direction and a too-long wavelength) (Chen and Liu, 1995), the KP equation is preferable for analyzing the obliquely interacting waves due to its complete integrability and availability of analytical solutions (Mulase, 1984).

where,andare nonzero arbitrary constants. For the values=3/2,=1/4 and=3/4, the KP equation turns into a potential KP (pKP) equation:
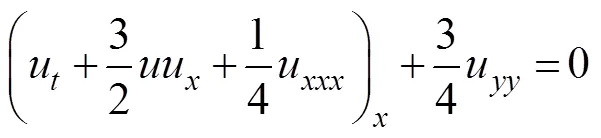
which has a very wide spectrum of applications, ranging from ocean modeling to physical applications (Li and Zhang, 2003).
In nonlinear modeling, the integer-order derivative is generally used to explain the physical and engineering aspects of the considered event. By the time scientists recognized that the integer-order derivative is inadequate for describing the nonlinear and complex events in nature, they sought the best way to model such events. This question was answered in Leibniz’s letter to L’Hospital, marking the beginning of the study on fractional calculus. Since then, several scientists have proposed many types of fractional derivatives and integrals, such as the Riemann-Liouville, Caputo, and Grünwald-Letnikov definitions. However, while using these definitions, scientists discovered some deficiencies in the applications and solution procedure to problems. For instance, both the Riemann-Liouville, Caputo, and Grünwald-Letnikov definitions do not satisfy the chain rule, Leibniz rule, and the derivative of a quotient of two functions. Recently, Khalil(2014) proposed a well-behaved, understandable, applicable, and efficient definition of fractional derivative and integral, called the conformable fractional derivative and integral.
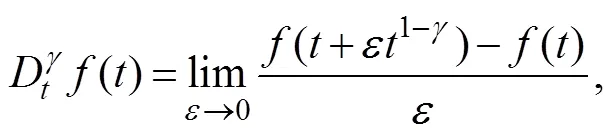
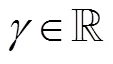
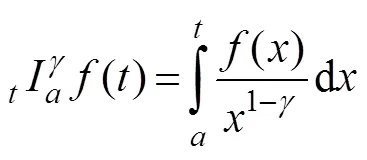
where the Riemann improper integral exists (Khalil,2014).
Some basic properties of the conformable fractional derivative are given below (Khalil, 2014; Abdeljawad, 2015):
whereÎ(0, 1) andindicates composition of two functions.
This new derivative definition is similar to the limit definition of the Newtonian integer-order derivative. In addition, this derivative definition satisfies the basic properties of the integer-order derivative. These two advantages of the conformable fractional derivative make this definition attractive and useful. Numerous articles on the application of conformable fractional derivative have been published due to its useful and understandable nature (Tasbozan, 2017, 2018; Abdeljawad, 2019). For example, Korkmaz(2018) applied the sine-Gordon expansion method to obtain the exact solutions to conformable time-fractional equations in regularized long waves. Rezazadeh(2018) employed the extended direct algebraic method to obtain the exact solution of time-fractional Phi-4 equations. Ilie(2018) used the first integral method to obtain the exact solution of some conformable fractional differential equations. Çenesiz(2017) expressed the solutions of the Hirota-Satsuma coupled KdV system using the tanh and homotopy analysis methods.
In this study, we obtain the solitary and traveling wave solutions of time-fractional pKP equation that describe the interaction of multidirectional propagated waves in shallow waters using the new extended direct algebraic method for the first time in the literature. To the best of our knowledge, all the obtained solutions have not been previously reported in the literature and will be beneficial for the computer simulation of the water waves in shallow waters.
2 A Brief Description of the New Extended Direct Algebraic Method
In this section, we describe the new extended direct algebraic method (Rezazadeh, 2017). The nonlinear time-fractional PDE is of the form:
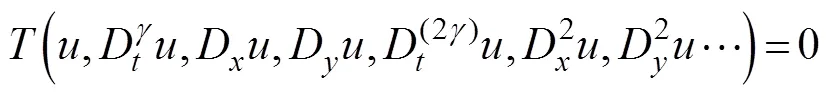
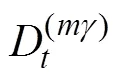
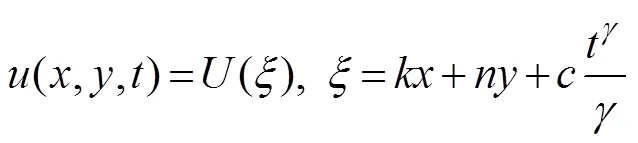
where,, andare arbitrary constants to be examined later. Applying the chain rule (Abdeljawad, 2015) and wave transform (3) in Eq. (2) gives rise to the following nonlinear ordinary differential equation (ODE):
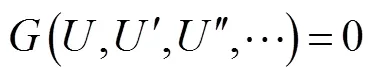
where the prime symbol indicates the integer-order derivative of functionwith respect to. Assume that Eq. (4) has the solution form
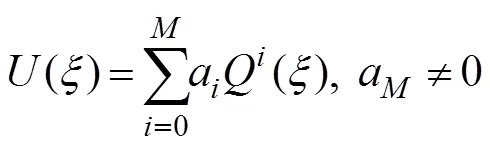
wherea(0≤≤) are constant coefficients to be evaluated later;is a positive integer, which is found by balancing principle in Eq. (4) andQ() holds the ODE in the form
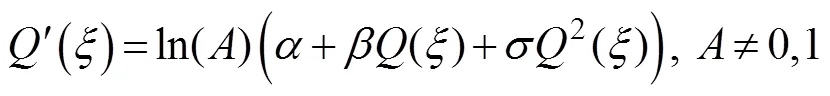
where,, andare constants. The solution set of Eq. (6) is given as follows:
1) When2−4<0 and¹0,
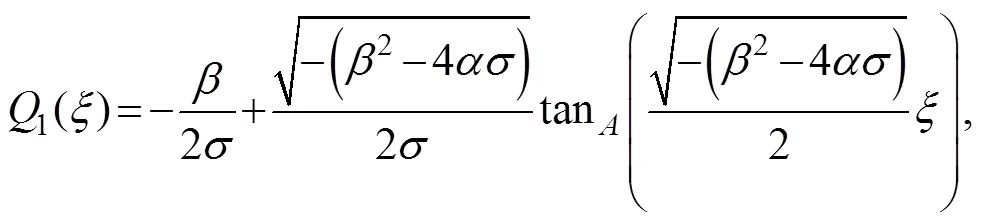
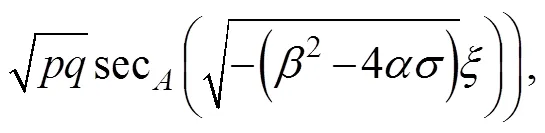
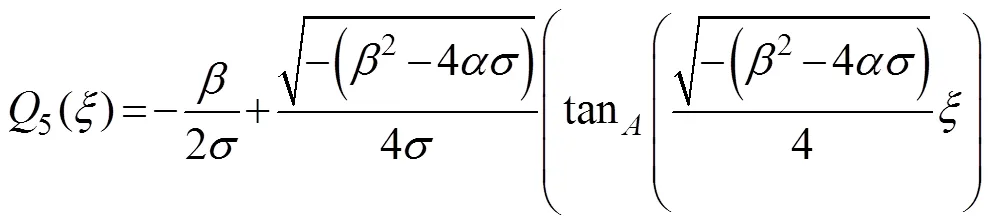
2) When2−4>0 and¹0,
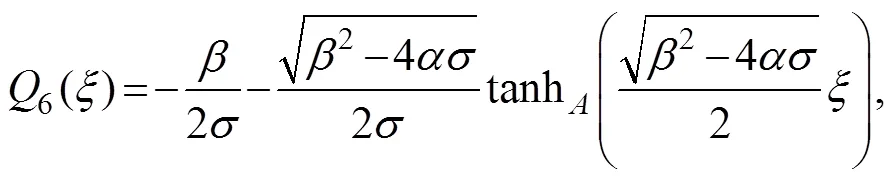
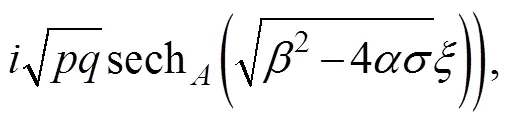
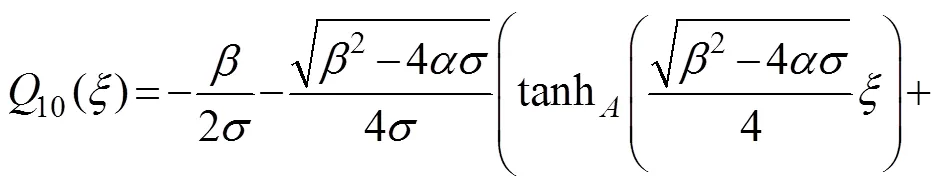
3) When>0 and=0,
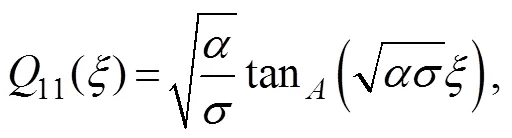
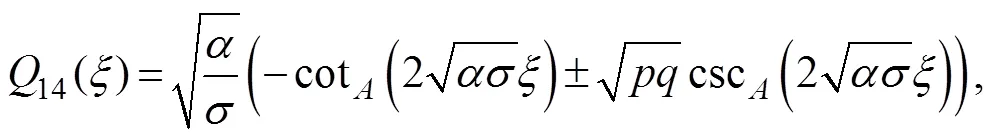
4) When<0 and=0,
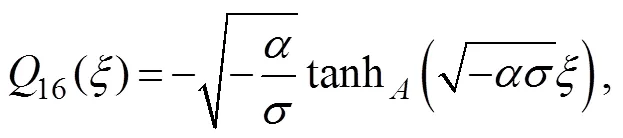
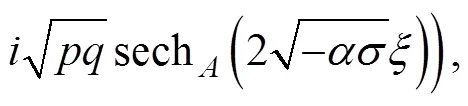
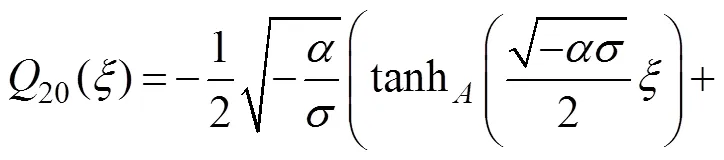
5) When=0and,
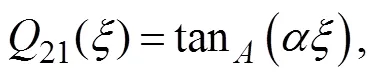

6) When=0and−,
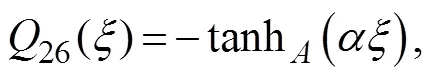

7) When2=4,

8) When=,(¹0), and0,
9) When=0,
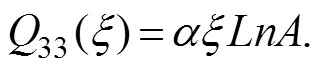
10) When=0,
11) When0 and¹0,

12) When=,(¹0), and0,
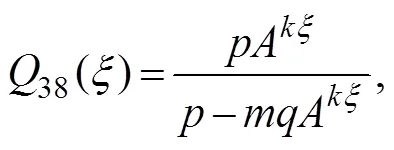
whereis an independent variable, andand, called deformation parameters, are arbitrary constants greater than zero. Substituting Eqs. (5) and (6) into Eq. (4) and vanishing the coefficients ofQ(), we obtain a nonlinear algebraic system inα(=0, 1, ···,)and,,.Then subrogating the acquired values of constants and solution set of Eq. (6) into Eq. (5) by using the wave transform (3), we obtain the analytical solutions for Eq. (2).
Remark 1 The generalized hyperbolic and triangular functions are defined as follows:
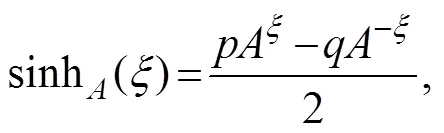
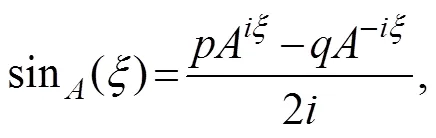
3 Implementation of the Method for the Equation of Shallow Water Waves
Consider the time-fractional pKP equation
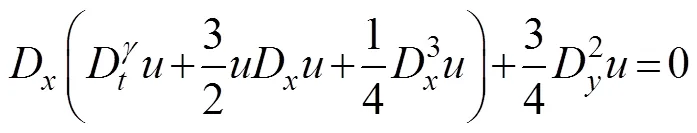
Considering the chain rule (Abdeljawad, 2015) with the help of wave transform (3) and integrating once, we have
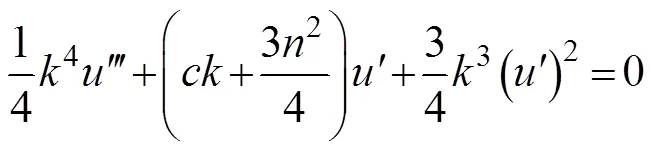
where the prime symbol indicates the integer-order derivative of the functionwith respect to. Assume that Eq. (8) has following the solution:
Using the balancing principle in Eq. (8), we have=1; therefore, the solution can be considered as
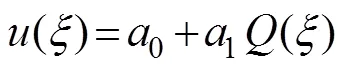
Subrogating Eq. (9) with Eq. (6) into Eq. (8), piling up the coefficients ofQ() and fixing them to zero, we obtain a set of algebraic equations in0,1,,, and. Solving these algebraic equations using the computer software Mathematica, we have
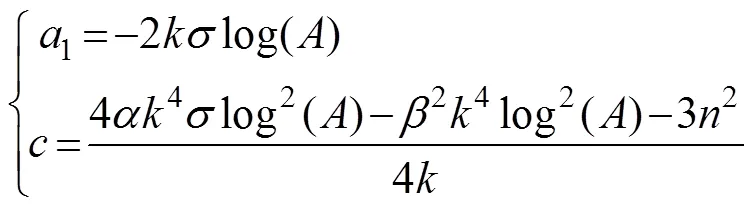
where0,,are free constants.
The solutions of (1) corresponding to (7), (12), (14), and (15) are as follows:
When2−4<0 and¹0,
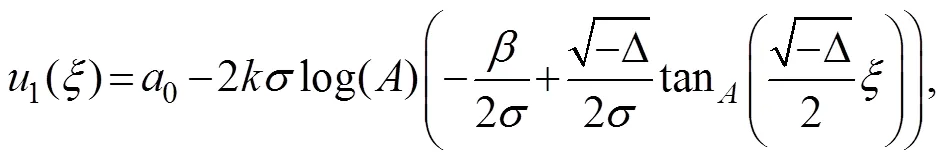
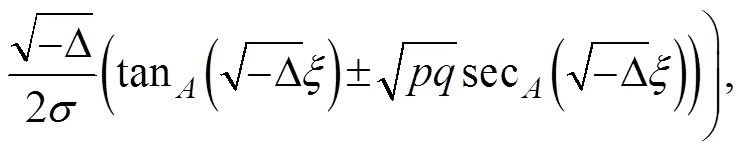
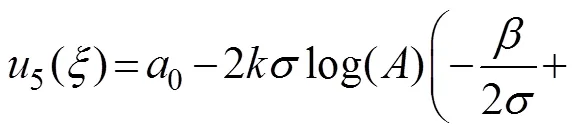
where ∆=2−4and
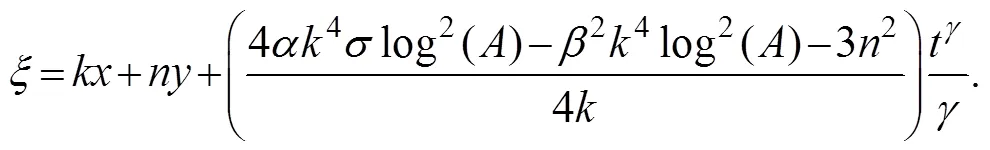
When2−4>0 and¹0,
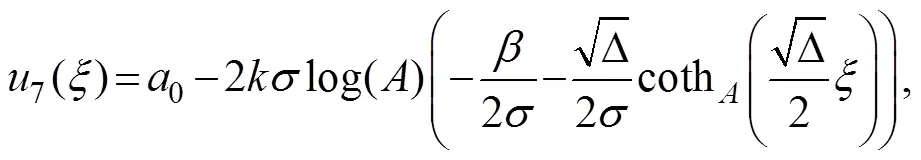
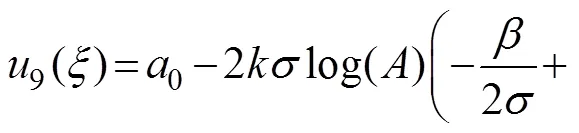
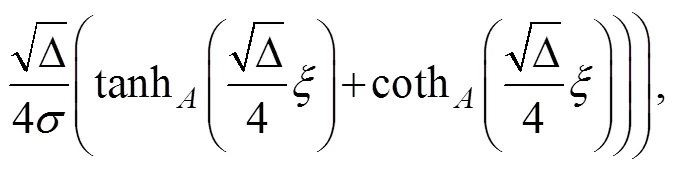
where ∆=2−4and
When>0and=0,
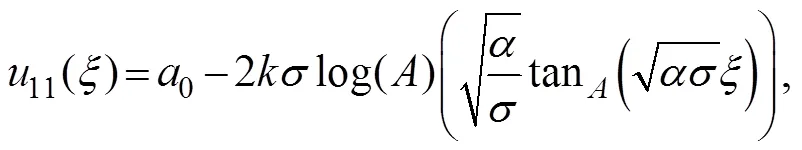
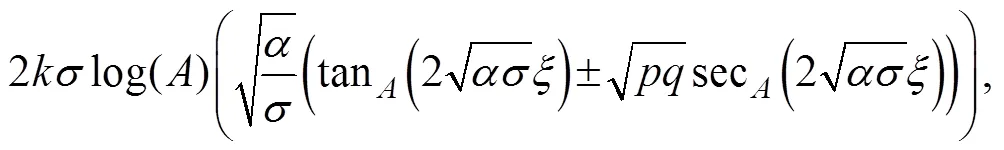
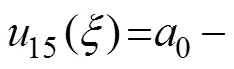
where ∆=−4and
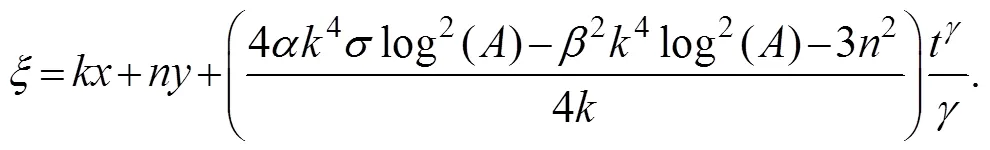
When<0 and=0,
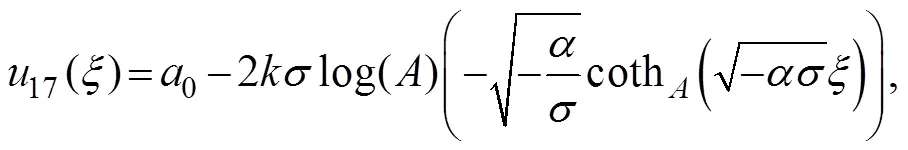
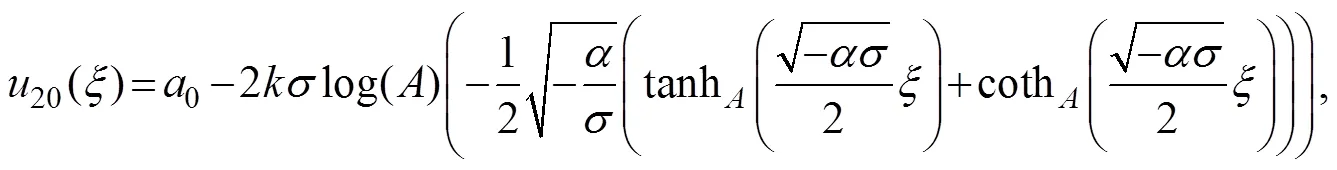
where∆=−4and
When=0and,
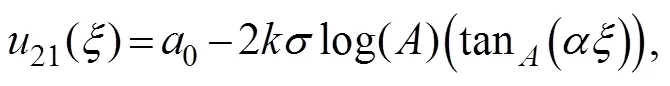
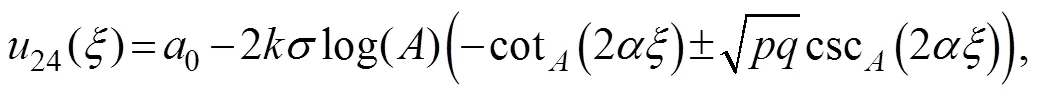
When=0and−,
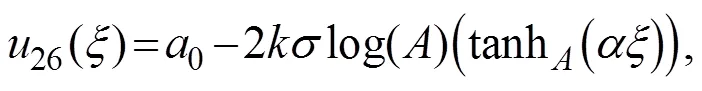

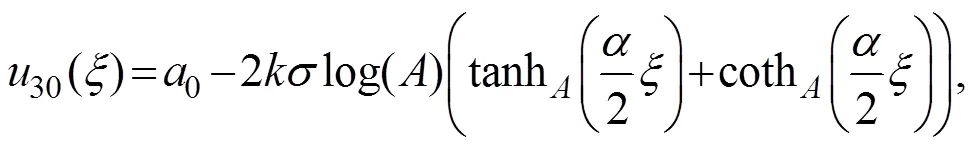
where ∆=42and
When2=4, we get
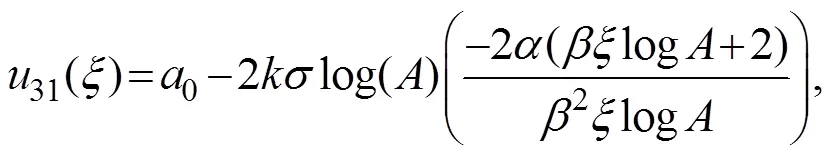
where
For the choise=0,
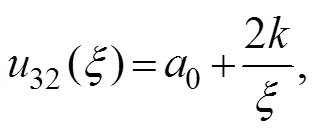
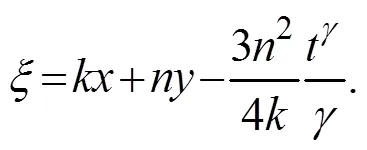
When0 and¹0,
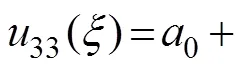
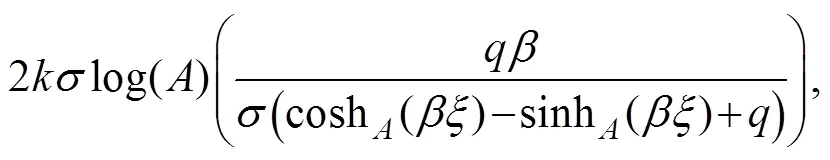
where
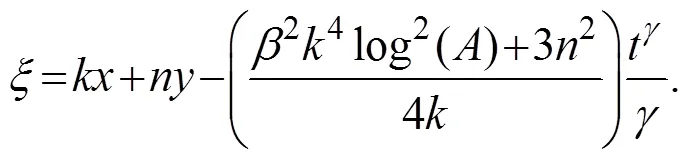
When=,(¹0),=,0,
where ∆=2and
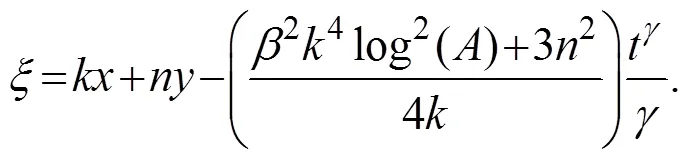
4 Graphical Representation
In this section, 3D and contour plot graphical representations of the solutions6(,,),9(,,),10(,,),16(,,),and17(,,)are given. Different types of graphics are expressed by changing the value of some parameters and the dominant wave propagation directions. Figs.1 to 7 show that the dominant wave propagation direction has a significant effect on the solution and that our solutions are very parameter-sensitive. From the contour plots showing the isobaths, wave surface breaks due to oblique wave interactions can be clearly seen (Figs.1b, 3b, 4b, and 6b). This can also be seen in the 3D graphs such as Fig.2a. Furthermore, the curvature of the wave surface according to the dominance of the wave propagation direction is also noticeable. On the other hand, honeycomb-shaped depth differences can be seen in Fig.7b. Another interesting finding from our solutions is the traveling wave and the corresponding depth gradient formed in Fig.5.

Fig.1 The 3D (a) and contour plot (b) graphics of the solution u6(x, y, t)for β=−5, a0=0, k=10, n=1, A=e−1, α=5, σ=1, p=2, q=−2, μ=0.9, t=0.001.
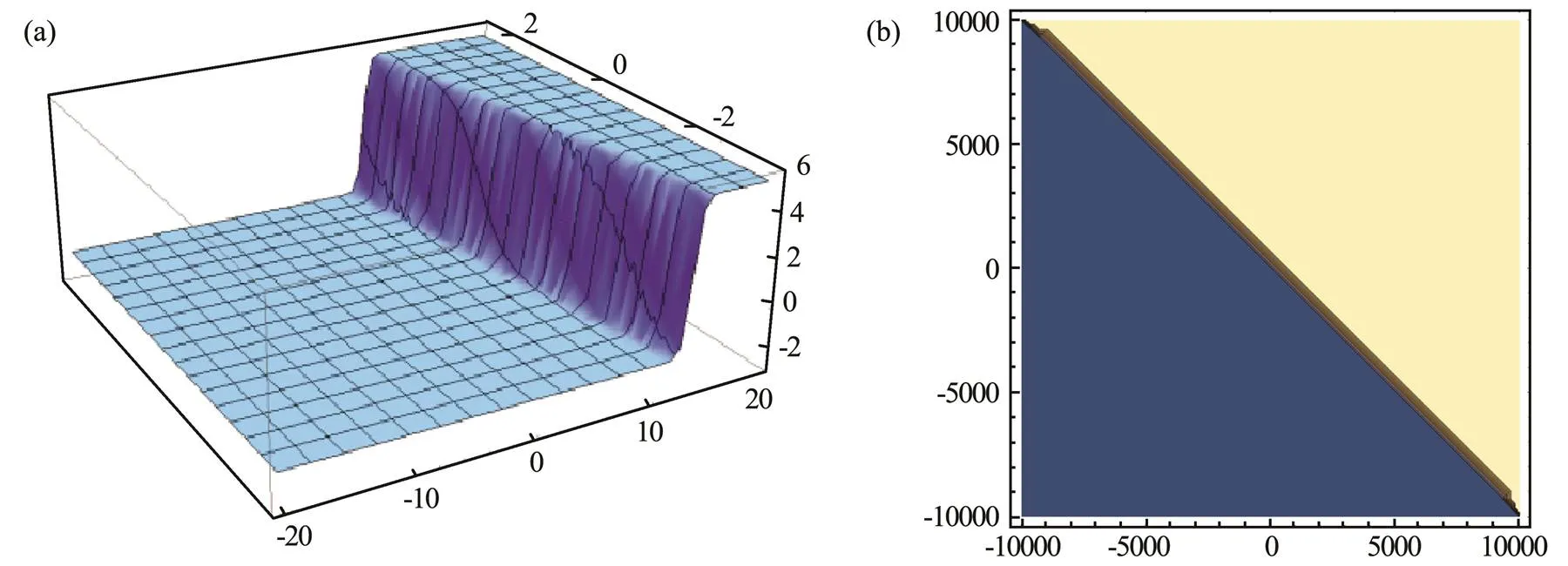
Fig.2 The 3D (a) and contour plot (b) graphics of the solution u9(x, y, t)forβ=1, a0=0, k=1, n=1, A=e2, α=−0.5, σ=1, p=1, q=1, μ=0.5, t=2.
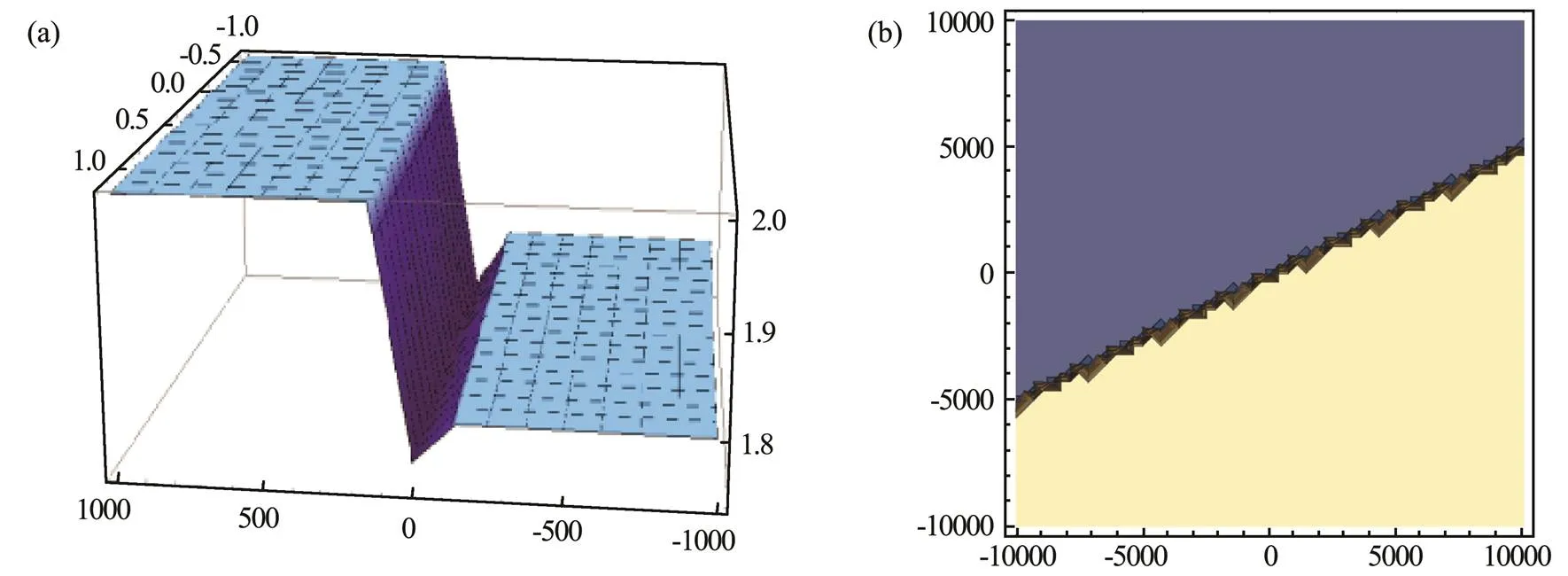
Fig.3 The 3D (a) and contour plot (b) graphics of the solution u10(x, y, t)forβ=0.1, a0=2, k=−1, n=2, A=e, α=−0.001, σ=0.002, p=2, q=1, μ=0.9, t=0.7.
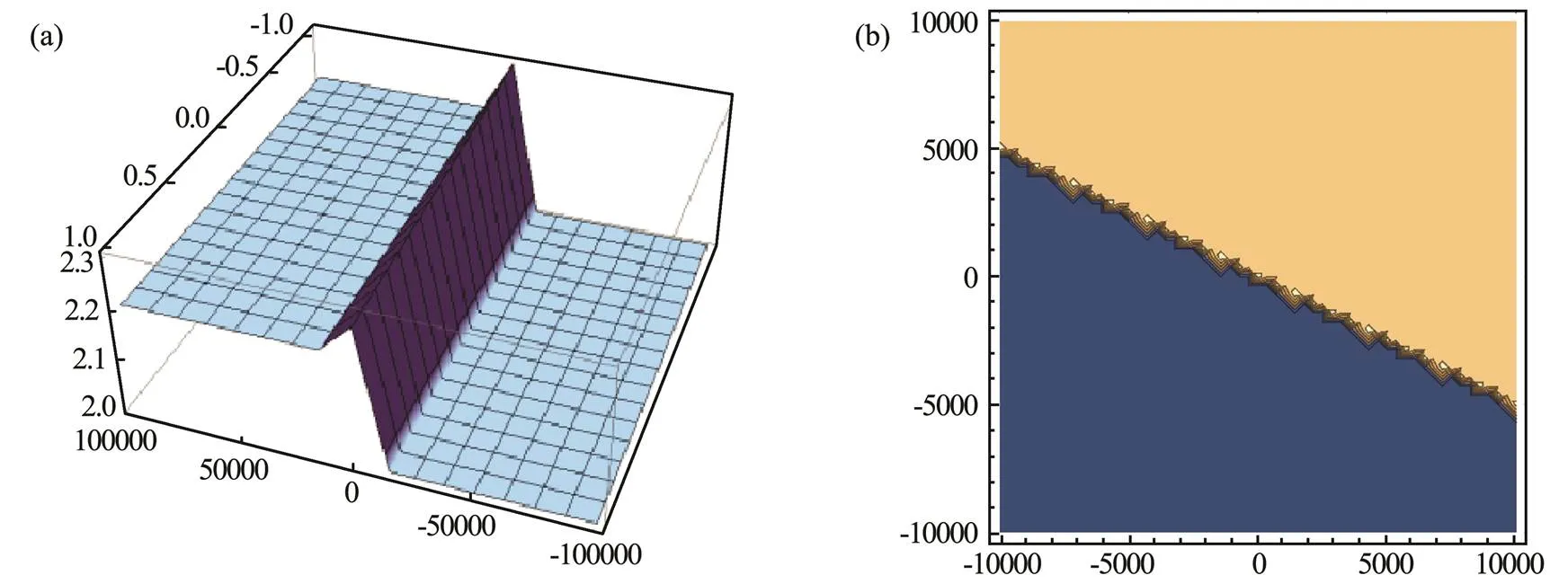
Fig.4 The 3D (a) and contour plot (b) graphics of the solution u10(x, y, t)for β=0.1, a0=2, k=1, n=2, A=e, α=−0.01, σ=0.02, p=2, q=1, μ=0.9, t=0.1.
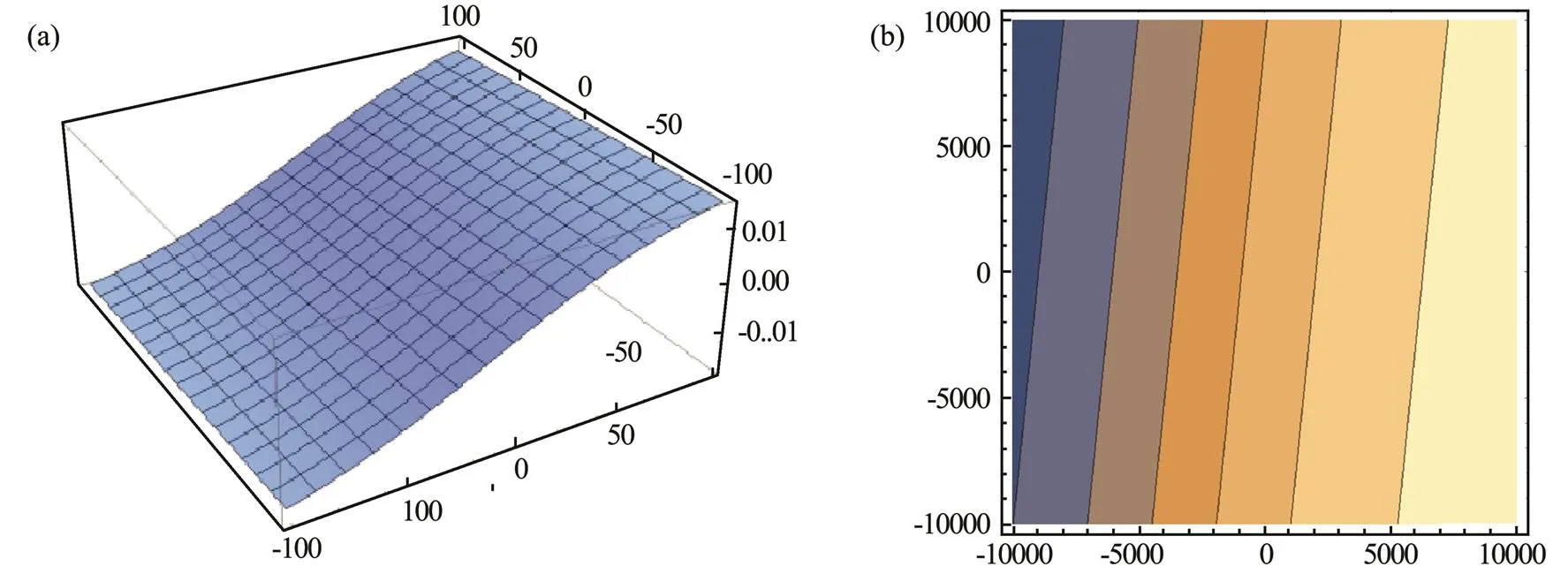
Fig.5 The 3D (a) and contour plot (b) graphics of the solution u16(x, y, t)forβ=0, a0=0, k=1, n=−0.1, A=e, α=−0.0001, σ=1, p=2, q=1, μ=0.5, t=2.
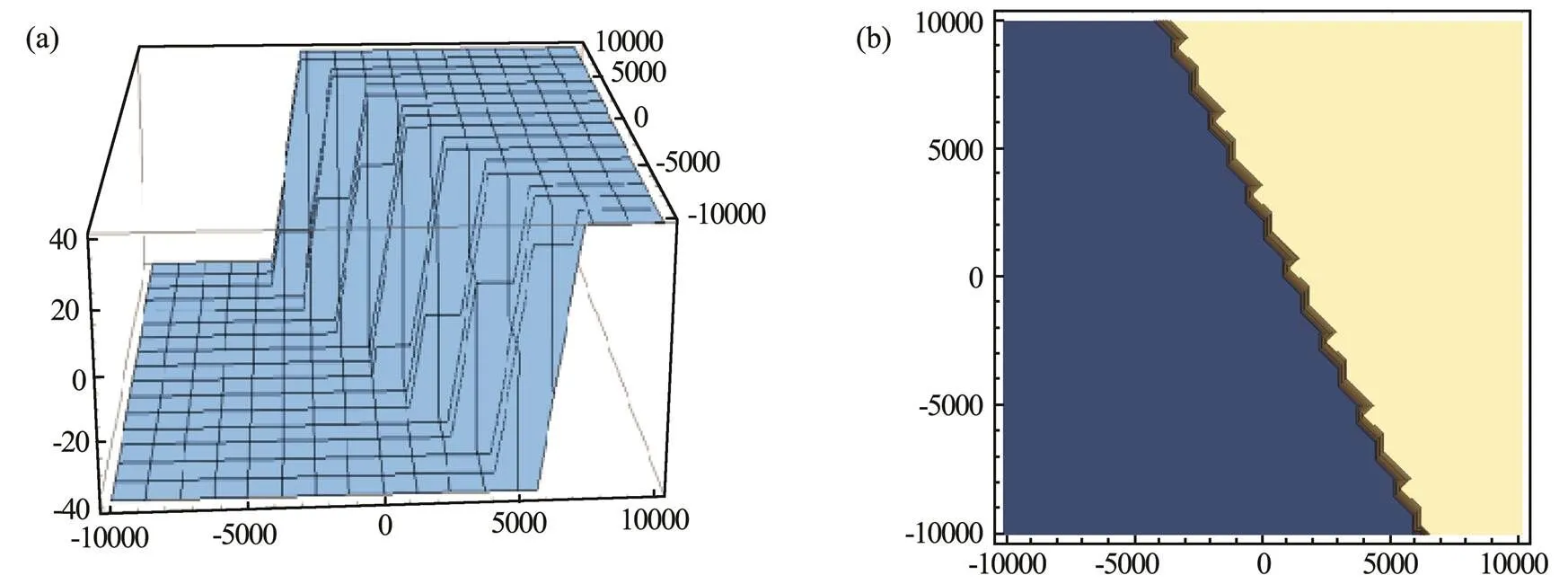
Fig.6 The 3D (a) and contour plot (b) graphics of the solution u16(x, y, t)forβ=0, a0=0, k=2, n=1, A=e, α=−10, σ=10, p=2, q=1, μ=0.5, t=2.
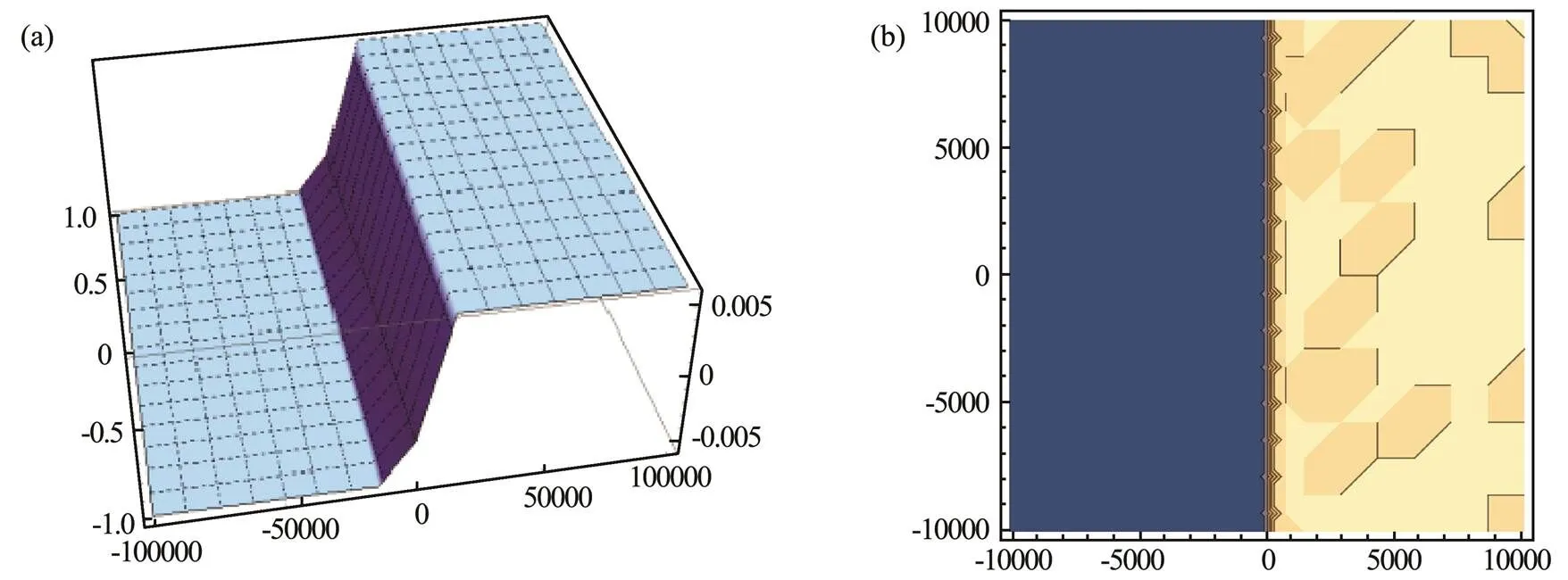
Fig.7 The 3D (a) and contour plot (b) graphics of the solution u17(x, y, t)for β=0, a0=0, k=−1, n=0.0001, A=e3, α=−0.000001, σ=1, p=3, q=1, μ=0.9, t=0.1.
5 Conclusions
In this study, we first obtained the solitary and traveling wave solutions for the time-fractional pKP equation using the new direct algebraic method by means of the conformable derivative. All the solutions were confirmed with the aid of the computer software Mathematica. The results demonstrated that the new extended direct algebraic is reliable, efficient, and can be applied to the solutions of the shallow water models in oceans. Second, 3D and contour plot graphical simulations were obtained to understand which type of model waves arises for the solution of the pKP equation. By applying the new direct algebraic method to solve the pKP equation, the behavior of the obliquely interacting surface waves in two dimensions can be analyzed. These wave solutions can be governed in the computer simulations of coastal and harbor modeling and can help researchers for further research.
Abdeljawad, T., 2015. On conformable fractional calculus., 279: 57-66.
Abdeljawad, T., Al-Mdallal, Q. M., and Jarad, F., 2019. Fractional logistic models in the frame of fractional operators generated by conformable derivatives., 119: 94-101.
Aliyu, A. I., Tchier, F., Inc, M., Yusuf, A., and Baleanu, D., 2019. Dynamics of optical solitons, multipliers and conservation lawsto the nonlinear Schrödinger equation in (2+1)-dimensions with non-Kerr law nonlinearity., 66 (2): 136-142.
Birse, M. C., 1990. Soliton models for nuclear physics, 25: 1-80.
Cenesiz, Y., Kurt, A., and Tasbozan, O., 2017. On the new solutions of the conformable time fractional generalized hirota-satsuma coupled KdV system., 55 (1): 37-50.
Chen, Y. Z., and Liu, P. L. F., 1995. Modified Boussinesq equations and associated parabolic models for water-wave propagation., 288: 351-381.
Epstein, I. R., and Showalter, K., 1996. Nonlinear chemical dynamics:Oscillations,patterns,andchaos., 100 (31): 13132-13147.
Ilie, M., Biazar, J., and Ayati, Z., 2018. The first integral method for solving some conformable fractional differential equations., 50 (2): 55.
Johnson, R. S., 2002. Camassa-Holm, Korteweg-de Vries and related models for water waves., 455: 63-82.
Khalil, R., Al Horani, M., Yousef, A., and Sababheh, M., 2014. A new definition of fractional derivative., 264: 65-70.
Korkmaz, A., Hepson, O. E., Hosseini, K., Rezazadeh, H., and Eslami, M., 2018. Sine-Gordon expansion method for exact solutions to conformable time fractional equations in RLW-class.–, 32 (1): 567-574.
Lan, Z. Z., Gao, Y. T., Yang, J. W., Su, C. Q., and Wang, Q. M., 2016. Solitons, Bäcklund transformation and Lax pair for a (2+1)-dimensional B-type Kadomtsev-Petviashvili equation in the fluid/plasma mechanics., 30 (25): 1650265.
Li, D. S., and Zhang, H. Q., 2003. New soliton-like solutions to the potential Kadomstev-Petviashvili (PKP) equation., 146 (2-3): 381-384.
Mitschke, F., Mahnke, C., and Hause, A., 2017. Soliton content of fiber-optic light pulses., 7 (6): 635.
Mulase, M., 1984. Complete integrability of the Kadomtsev-Petviashvili equation., 54 (1): 57-66.
Osborne, A. R., 2018. Nonlinear Fourier methods for ocean waves., 26: 112-123.
Ovidko, I. A., and Romanov, A. E., 1987. Topological excitations (defects, solitons, textures, frustrations) in condensed media., 104 (1): 13-45.
Pelinovsky, E., Talipova, T., and Kharif, C., 2000. Nonlinear-dispersive mechanism of the freak wave formation in shallow water., 147 (1-2): 83-94.
Rezazadeh, H., Khodadad, F. S., and Manafian, J., 2017. New structureforexactsolutionsofnonlineartimefractionalShar- ma-Tasso-Olver equationconformable fractional derivative., 12 (1): 13-21.
Rezazadeh, H., Tariq, H., Eslami, M., Mirzazadeh, M., and Zhou, Q., 2018. New exact solutions of nonlinear conformable time-fractional Phi-4 equation., 56 (6): 2805-2816.
Stuhlmeier, R., and Stiassnie, M., 2019. Nonlinear dispersion for ocean surface waves., 859: 49-58.
Tasbozan, O., Cenesiz, Y., Kurt, A., and Baleanu, D., 2017. New analytical solutions for conformable fractional PDEs arising in mathematical physics by exp-function method.,15 (1): 647-651.
Tasbozan,O.,Senol,M.,Kurt,A.,andÖzkan,O.,2018.Newsolu- tions of fractional Drinfeld-Sokolov-Wilson system in shallow water waves., 161: 62-68.
Xu, K. K., Li, H. X., and Yang, H. D., 2018. Local-properties-embedding-based nonlinear spatiotemporal modeling for lithium-ion battery thermal process., 65 (12): 9767-9776.
. E-mail: akurt@pau.edu.tr
February 1, 2019;
December 24, 2019;
June 2, 2020
(Edited by Xie Jun)
杂志排行
Journal of Ocean University of China的其它文章
- Taxonomy and Phylogeny of a New Marine Planktonic Ciliate, Strombidium pseudorapulum sp. n.(Protozoa, Ciliophora, Oligotrichia)
- Can Langmuir Circulations Solve the Problem of Insufficient Upper-Ocean Mixing?
- Dynamic Diurnal Changes in Green Algae Biomass in the Southern Yellow Sea Based on GOCI Images
- Transcriptomic Profiling of the Immune Response to Crowding Stress in Juvenile Turbot (Scophthalmus maximus)
- Reducing the Common Environmental Effect on Litopenaeus vannamei Body Weight by Rearing Communally at Early Developmental Stages and Using a Reconstructed Pedigree
- Isolation of Enterococcus faecium with Feeding Attractant Function from Pacific White Shrimp (Litopenaeus vannamei) Intestine
