Review: Energy Methods for Multiaxial Fatigue Life Prediction

Zheng Zhong and Yingya Lu

(1. School of Science, Harbin Institute of Technology, Shenzhen, Shenzhen 518055, Guangdong,China;2. United Automotive Electronic Systems Co., Ltd., Shanghai 201206, China)

Abstract: Fatigue fracture of materials and structures is one of the most common failure modes in engineering applications. Under multiaxial non-proportional loading condition, a large number of materials show non-proportional hardening characteristics, which results in a significant reduction of fatigue life. In this paper, a review on energy methods for multiaxial fatigue life prediction has been carried out. The energy methods are divided into three categories: energy based models without considering the loading path effect, energy based models combined with the critical plane method, and energy based models considering the loading path effect. Among these categories, energy based models considering the loading path effect are introduced in detail since they involve the non-proportional hardening effect in multiaxial fatigue.

Keywords: multiaxial fatigue, life prediction, plastic work, non-proportional factor

1 Introduction

Fatigue phenomena are ubiquitous in airplanes, automobiles, ships, pressure vessels, and electromechanical devices. Statistics show that more than 80% of engineering structure failures are caused by fatigue[1]. The process of low cycle fatigue is a process of dissipation of energy in essence. Mechanical energy input by load is mainly absorbed or dissipated as heat, acoustic emission, atomic vibration, and so on. So the fatigue resistance of a metal can be characterized in terms of its capacity to absorb and dissipate plastic strain energy[2]. In practical applications, due to the complexity of structural geometry, some local positions may be under the multiaxial loading condition, even if the structure is under uniaxial loading, which makes multiaxial fatigue one of the most common failure forms. The uniaxial fatigue criteria are often generalized to calculate the fatigue life under multiaxial proportional loading condition. However, under multiaxial non-proportional (NP) loading conditions, many materials demonstrate additional hardening effect, since the axes of principal strains rotate with the cyclic loading process. In this case, the stresses are related not only to the strains, but also to the history of the loading path[3-4]. This phenomenon was first observed by Taira et al.[5], and then explained respectively by Lamba and Sidebottom[6-7]and Kanazawa et al.[8]The NP hardening effect significantly reduces the fatigue life under the multiaxial NP loading condition, compared with the multiaxial proportional loading condition. Therefore, the NP hardening effect must be considered in the prediction of multiaxial fatigue life.

The equivalent strain methods[9-15]may give the same estimated life for different NP loading paths on the condition that the paths have the equal equivalent strain amplitude. So the equivalent strain methods are not able to consider the effect of the NP hardening effect on the fatigue life calculation. Although the critical plane methods[16-23]can consider the NP hardening effect and the continuum damage mechanics based method can consider the mechanism of damage evolution[24-25], they require complex incremental plastic analyses based on the multiaxial stress-strain relationship that is not easy to obtain. Comparatively, the energy methods not only have clear physical significance but also can avoid the complex incremental plastic analysis, since the energy contains the interaction of stress and strain. In addition, the energy methods can theoretically deal with all kinds of loads so that they have wide applicability.

2 Energy Based Models

2.1 Energy Based Models without Considering Loading Path Effect

Manson-Coffin Equation is a milestone for the calculation of low cycle fatigue life, which first correlated the fatigue life with the strain[26-27]. However, low cycle fatigue life is affected not only by the strain but also by the stress. Some research[28-29]indicate that the tensile mean stress would contribute to the opening of crack surface and reduce the friction, while the shear stress would lead to the mechanical self-lock of irregular crack surface and increase the friction. Therefore, the strain-life model using the strain as a failure parameter cannot fully reflect the fatigue failure mechanism. In contrast, the energy-life model can better reflect the fatigue failure mechanism by considering both the stress and the strain.

Morrow[2]presented an energy-life model based on the assumption that the accumulative plastic work is the main reason of irreversible damage. At about the same time, Esin et al.[30-31]proposed that the cyclic hysteretic energy could be used as a parameter to describe the fatigue damage of materials. Garud[32]extended Morrow’s model from uniaxial fatigue to multiaxial fatigue by introducing a shear work weight factor reflecting the difference between the shear plastic work and the tensile plastic work. A practical energy-life model was then obtained for multiaxial fatigue life prediction as

Nf=A(Wc)r

(1)

(2)

whereWc,ξ,Δσ,Δεp,Δτ,Δγpare respectively the plastic work per cycle, the shear work weight factor, the normal stress range, the normal plastic strain range, the shear stress range, and the shear plastic strain range, andA,r,n′are material constants.

Lefebvre et al.[33-34]estimated approximately the multiaxial cumulative plastic work per cycle according to von Mises equivalent stress and equivalent strain for a multiaxial fatigue criterion:

(3)

Considering the amplitude effect of loading path, Kliman and Bily[35]studied the variation of plastic work under block loading and their sensitivity to material parameters. They suggested that the cyclic strain hardening index is unnecessary to be considered as a variable when the stress amplitude is employed to calculate fatigue life, and then proposed a plastic work model that can deal with random loads.

The plasticity work model has successfully described the relationship between energy and fatigue life. But when the load level is small, the plastic strain is small so that the plastic work is difficult to calculate accurately. Therefore, Ellyin et al.[36-38]proposed an energy-life model based on the total strain energy per cycle, which is called the total strain energy density model. The total strain energy is defined as the sum of the plastic strain energy ΔWpand the positive elastic strain energy ΔWe+:

ΔW=ΔWp+ΔWe+

(4a)

(4b)

whereσais the stress amplitude andEis the elasticity modulus.

Koh[39]pointed out that Ellyin’s model requires the loading covering negative value, otherwise the hysteresis loop will not intersect with horizontal axis and then the positive elastic strain energy will be incorrect. Therefore, Koh improved Ellyin’s model by the stress amplitude and the mean stress.

Roostaei et al.[40]indicated that the influence of the average stress should be taken into account so that they modified the total strain energy density model by adding the average stress into the elastic strain energy as

(5)

whereσmis the mean stress andσmaxis the maximum stress.

Nearly all of these methods evaluate the fatigue life through strain or stress amplitude. Lefebvre’s model is capable in general but is not able to distinguish the tensile and shear damage since it just considers equivalent stress and strain. In contrast, Garud’s model recognizes the tensile and shear damage by introducing a shear work weight factor. If the plastic strain is tiny, Lefebvre’s model and Garud’s model will give an inaccurate estimation of the plastic work, while Ellyin’s model and Roostaei’s model avoid the situation by summing the elastic and plastic strain energy as the damage parameter.

2.2 Energy Based Models Combined with Critical Plane Method

The critical plane method focuses on the priority plane of fatigue failure, and discusses the stress, the strain, and their mutual influence on this plane. Since the critical plane methods have clear physical significance and can obtain both fatigue life and crack initiation direction at the same time, they becomes the most popular method of multiaxial fatigue life prediction. For example, the critical plane strain energy density model[41-49]was established by combining the energy based method with the critical plane method and plays an increasingly important role in fatigue life analysis.

The most well-known critical plane energy parameter is the SWT parameter proposed by Smith et al.[22]who selected the product of the strain amplitude and the maximum tensile stress as the damage parameter. Socie[23]modified the SWT parameter by choosing the maximum principal strain amplitude plane as the critical plane and defining the damage parameter as the product of the maximum principal strain amplitude Δε1/2 and the maximum normal stressσn，maxon the maximum principal strain amplitude plane as the energy parameter as follows:

(6)

Since this model introduces the maximum normal stress on the maximum principal strain amplitude plane, it can consider the influence of the mean stress and the NP hardening effect.

Liu[41]proposed a virtual strain energy model, which is an extension of uniaxial energy-life model combined with the critical plane method:

ΔW=ΔWe+ΔWp≅ΔσΔε=

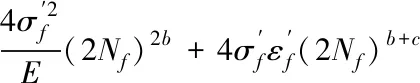
(7)

where ΔWe,Δεare respectively the elastic strain energy, and the strain range. The total work is the sum of the tensile work and the shear work. Liu[41]also proposed that the plane with the maximum tensile work and the plane with the maximum shear work can be taken as the critical plane respectively for the tensile failure mode and the shear failure mode. Chu et al.[42-43]employed the similar parameters to seek a plane with the maximum sum of tensile work and shear work as the critical plane. The difference is that they use the maximum stress to replace the stress amplitude so that the influence of mean stress can be considered:

(8)

whereτn,maxis the maximum shear stress.

Glinka et al.[44-45]suggested the sum of the elastic-plastic strain energy density on the critical plane serve as the fatigue damage parameter:

(9)

They further pointed out that the model of Chu et al.[42-43]might neglect the mean stress effect when the positive strain amplitude is zero and the normal stress is constant under an NP loading path, and proposed just to consider the shear plastic work and the mean stress as follows:

(10)

The model of Glinka et al.[44-45]considers the opening and sliding of crack surface and reveals that a positive mean normal stress helps the crack opening and a mean shear stress tends to overcome the reverse sliding friction. Since the model of Glinka et al.[44-45]is based on the shear work, it may be more reasonable to use the shear parameters of the strain life equation than the tensile parameters.

(11)

Varvani-Farahani[47]proposed to employ the plane where the Mohr’s circle of stress or strain is largest as the critical plane and considering the impact of the axial mean stress.

Chen, Xu and Huang[48]thought that all the stress and strain components had a non-negligible influence on the fatigue damage. Thus they put forward two fatigue life models according to the failure modes: for the tensile failure material, the critical plane is chosen as the maximum principal strain range plane; for the shear failure material, the critical plane is chosen as the maximum shear strain range plane.

Since the SWT model and the model of Glinka et al. are suitable for tensile failure and shear failure respectively, Gan[49]combined their characteristics to obtain the following damage parameters:

(12)

(13)

where Δτ1is the maximum shear stress range on the maximum normal strain amplitude plane, and Δγ1is the maximum shear strain range on the maximum shear strain amplitude plane.

The former corresponds to the form of tensile failure. It inherits the SWT parameter as the main damage parameter and introduces a shear component to consider the influence of both the tensile mean stress and the shear component. The latter corresponds to the form of shear failure. The shear work on the maximum shear strain amplitude plane is chosen as the main damage parameter, and a tensile component is also included to modify the damage. If the influence of the mean shear stress needs to be considered, Δτ1can be replaced by the maximum shear stressτn,maxon the critical plane.

2.3 Energy Based Models Considering Loading Path Effect

The traditional energy based model usually does not consider the influence of the loading path effect on the fatigue life. As described in Section 2.1, as long as the strain amplitude is the same, those methods will give the same fatigue life, no matter what strain loading path is used and whether the loading history is the same. This is not consistent with experimental observations. In fact, under a multiaxial NP loading path, the fatigue life will be significantly reduced because of the NP hardening effect. For the multiaxial low cycle fatigue, fatigue life must be path-dependent since plastic strain is path-dependent. Therefore, considering the loading path effect in the energy life model is a necessary step to effectively predict the multiaxial low cycle fatigue life.

2.3.1Aneffectiveplasticworkmodel

Lu et al.[50]proposed an effective plastic work model to consider the loading path effect. In this model, NP hardening can be reflected by the NP factorFNPas

(14)

whereσeq,σ0, andσOPare respectively von Mises equivalent stress amplitudes for a measured loading path, the pure tensile path, and the circular path under the similar strain level.

In practical application,FNPcan be calculated according to the shape of loading path as follows:

(15)

whereTis the period of one cycle, π is the ratio of the circumference of a circle to its diameter,εI(t) andεImaxare respectively the absolute value of maximum principal strain at timetand during one cycle,ψ(t) is the angle ofεI(t) andεImax.

By introducing the NP factor to consider the loading path effect, the plastic work in the traditional energy model can be extended into the effective plastic work:

Weff=(1+αwFNP)W0

(16)

whereαwis a material constant reflecting material sensitivity to an NP path, andW0denotes uniaxial plastic work or multiaxial proportional plastic work.

The material dependent constantαwis introduced by Lu et al.[50]as the specific value of the plastic work under 90° out-of-phase loadings,WOP, to that under uniaxial or proportional loading,W0, for the similar high strain level, which reflects the material sensitivity to the non-proportionality of the loads, i.e.:

(17)

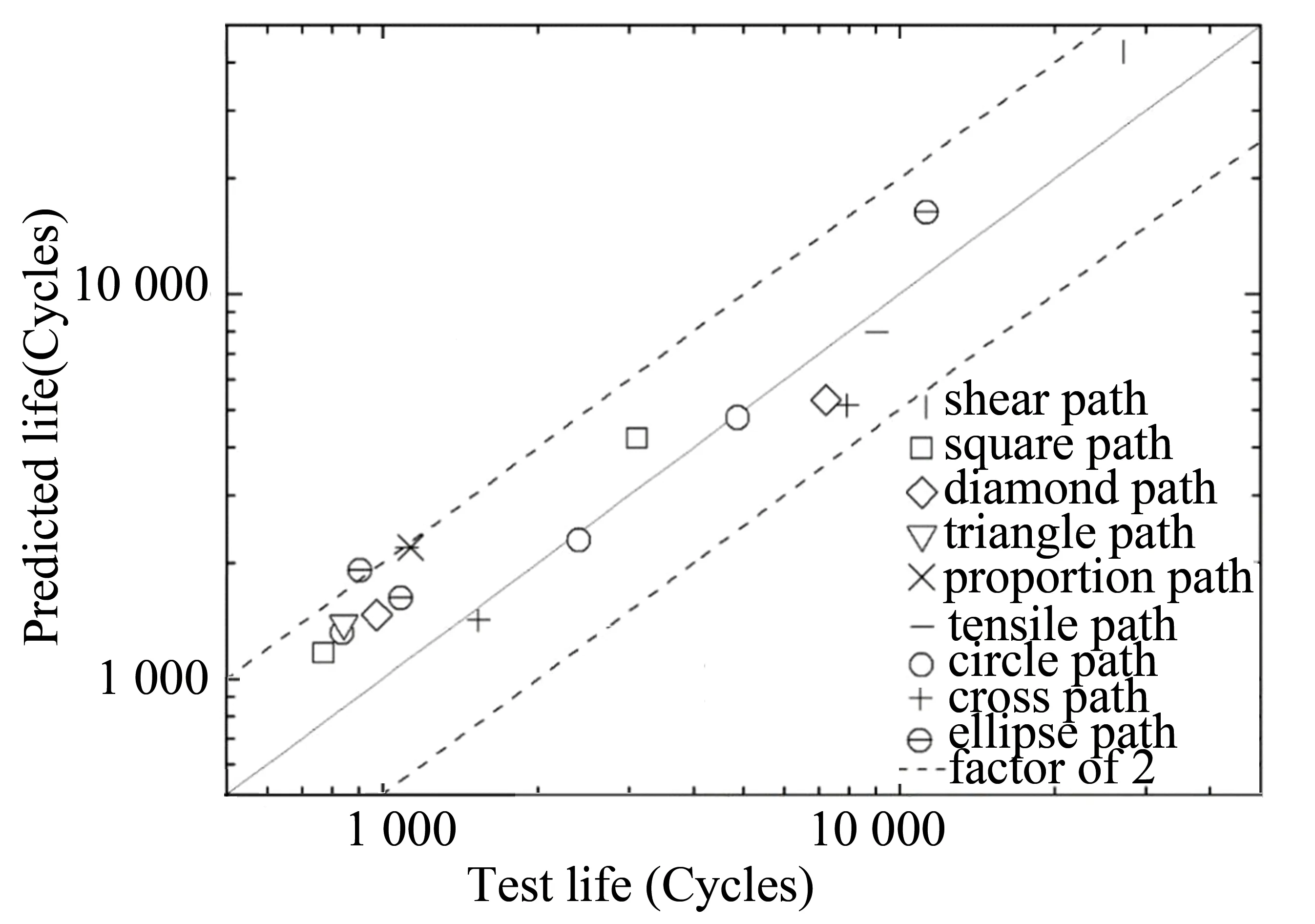
For uniaxial or proportional loading,FNP=0 andWeff=W0; for 90° out-of-phase loadings,FNP=1 andWeff=WOP; for general multiaxial NP loading, 0

The multiaxial fatigue life can then be calculated through replacing the plastic workWcbyWeff, which yields

Nf=A[(1+αwFNP)W0]r

(18)

For a uniaxial or proportional condition, (FNP=0,Weff=W0), Eq. (18) degenerates to Garud’s model described in Eq. (1). For general multiaxial NP condition, (0≤FNP,W0≤Weff), the effective plastic work is enlarged to reflect the influence of the NP hardening, which reduces fatigue life.

2.3.2Aneffectiveplasticworkmodelbasedonuniaxialwork

Similar to the model of Lu et al.[50], Zhu et al.[51]estimated the effective plastic work based on uniaxial work. In the effective plastic work model, a reference work is taken as the plastic work under uniaxial or proportional loading paths. Note that the plastic work under proportional loading path in the form of Eq. (2) is accurate, while the uniaxial work is approximate as the reference work. When the reference work is taken as the uniaxial work, it is more reasonable to modify the uniaxial work by the Moment of Inertia method[52-53]as the effective plastic work.

The Moment of Inertia method calculates the moment of inertiaIXXandIYYof the loading path relative to theX-axis and theY-axis by assuming the loading path as a uniform line element with unit mass.IXXandIYYrepresent respectively the contribution of tensile and shear components to the damage. Then the eigenvalue of the loading path can be written as

(19)

wheredis the diameter of the minimum envelope circle of the loading path, andξ=Wt/Wσis the weight factor denoting the ratio of the shear plastic work to the tensile plastic work.

If the uniaxial tensile plastic work is combined with the eigenvalue of the loading path, Zhu et al.[51]suggested that the effective plastic work can be expressed as

Weff=(+αwFNP)·F1·ΔWσ

(20)

For the pure shear path,IYY=0,W0=ξ·Wσ=Wτ; for the uniaxial tensile path,IXX=0,W0=Wσ; for a proportional path,W0=(1+ξ)·Wσ=Wσ+Wτ.

Therefore, it is feasible to use the effective plastic work model based on the uniaxial plastic work to predict the multiaxial fatigue life. The weight factor can be obtained through experiments, and the eigenvalue of the loading path can be obtained by the Moment of Inertia Method, then the effective plastic work can be obtained by them and the uniaxial tensile work. This process avoids computing the shear plastic work.

2.3.3Acumulativedamagemodelbasedontheeffectiveplasticwork

To deal with multiaxial irregular loading path, Lu et al.[54]provided a damage accumulation algorithm based on the effective plastic work. A combined or irregular loading path can be approximately divided into several regular subpaths and then the damage and fatigue life of every subpath can be estimated by the effective plastic work model described in Section 2.3.1 or Section 2.3.2. The total damage of one cycle of the irregular path can be obtained according to the Palmgren-Miner’s cumulative damage theory, and then the fatigue life can be obtained.

To divide an irregular path, the reference point is chosen as the perimeter center of the loading path, and the number of cycle is calculated as the ratio of the central angleθicorresponding to theith divided arc to the entire period (2π):

Ti=θi/2π

(21)

The fatigue damage corresponding to one cycle of the irregular path is

(22)

The failure occurs when

(23)

or

(24)

3 Test Verification

For 316L austenitic stainless steel tubular specimens, fatigue tests under 9 kinds of regular paths and 9 kinds of irregular paths have been performed in MTS809 axial/torsional test system. The test loadings cover the strain amplitudes of 0.4%-0.84%, and the test results are shown in Table 1. The test results of No.1-2, 4 and No.7-9 are used to determine coefficients and the others are used for examining the models. The comparisons between the predicted lives of the effective plastic work model or the cumulative damage model based on the effective plastic work and the test lives are respectively shown in Fig.1 and Fig.2. Since the material parameterαwdescribed in Eq. (17) is determined by the data of 90° out-of-phase loading and uniaxial loading, the predicted lives for most of the circle paths and tensile paths are nearly accurate, as shown in Fig.1. Most of the predictions of the effective plastic work model are conservative. A possible explanation is that the effective plastic work model underestimates the impact of the shear plastic work. In contrast, the predicted lives distribute nearly symmetrically when the cumulative damage model is employed, as shown in Fig.2. It is because the damage accumulation algorithm balances the weight between the tensile and shear damage by calculating the central angle in a normal strain vs. shear strain diagram.

Table 1 Fatigue test results for 16 different loading paths [36]

It can be seen from Fig.1 that all the predicted results of the effective plastic work model fall within the factor of 2 error lines. It can be seen from Fig.2 that all the predicted results of the cumulative damage model based on the effective plastic work fall within the factor of 2 error lines.

Fig.1 Comparison of the predicted lives by the effective plastic work model and the observed fatigue lives[32]

Fig.2 Comparison of the predicted lives by the cumulative damage model and the observed fatigue lives[36]

4 Conclusions

1) The energy methods not only have clear physical significance but also can avoid the complex incremental plastic analysis, since the energy contains the interaction of stress and strain. They are divided into three categories, energy based models without considering the loading path effect, energy based models combined with the critical plane method and energy based models considering the loading path effect.

2) By introducing the NP factor into the traditional energy life model to reflect the path effect, the effective plastic work model can further distinguish the difference between multiaxial proportional loading and multiaxial NP loading. The predicted results are in a good agreement with fatigue test results.

3) The effective plastic work model based on uniaxial plastic work introduces the path eigenvalues computing according to the Moment of Inertia Method, and is able to evaluate the multiaxial fatigue life through the uniaxial work.

4) Combining the effective plastic work model and the Palmgren-Miner’s cumulative damage theory, the fatigue life under the irregular loading path can be estimated, which may help to develop the model for variable amplitude fatigue.