Representative heights for assessing whole-tree values of cell-type proportions in Eucalyptus camaldulensis and E. globulus

Jyunichi Ohshima · Kazuya Iizuka · Futoshi Ishiguri · Shinso Yokota ·Toshihiro Ona

Abstract Eucalyptus camaldulensis Dehnh. and E. globulus Labill. are economically important species for wood and pulpwood materials. Representative heights for assessing whole-tree values of cell-type proportions (vessels, fibers, ray and axial parenchyma percentages) using increment cores were examined by analysis of within-tree variations. Pattern differences were evaluated between trees and species in both radial and axial directions by statistical data analysis (Moses test). In E. camaldulensis,within-tree variation of vessel percentage was generally higher in the upper and outer regions of the trunk. In contrast, E. globulus within-tree variation was unclear. In both species,although no clear pattern of fiber percentages was observed, within-tree variations of ray and axial parenchyma levels were higher in the lower and inner regions.Significant differences in patterns were observed in the axial variation between species for vessel percentages and in the radial variation between trees of E.camaldulensis for ray parenchyma percentages.The representative heights for assessing whole-tree cell-type proportions were 0.8 m above the ground for E. camaldulensis and 2.8 m for E.globulus, regardless of differences in tree height and pattern of within-tree variation of cell-type proportions.

Keywords Eucalyptus·Cell-type proportion·Within-tree variation · Representative height · Quality breeding

Introduction

The Australian native hardwood genus, Eucalyptus, is widely planted throughout the world with over 20 million ha of plantations in more than 100 countries. It has been a successful exotic genus because of many of its species relatively fast growth and tolerance of harsh environments(Myburg et al.2014).Eucalyptus species are processed into solid wood for flooring,construction,outdoor furniture and other products, and paper products for newsprint, facial tissues, and other specialty papers (Raymond 2002; Nolan et al. 2005; Washusen 2011; Albaugh et al. 2013; Henry 2014).

Tree breeding programs are commercially important to improve quality and productivity (Sato 2007). In practical,quality breeding programs, the use of non-destructive increment cores has the advantage of sampling without felling the trees to achieve efficient elite tree propagation(Downes et al. 1997). However, this method is an indirect selection method with limited sampling, using an increment core with a conventional 5-12 mm diameter,whereas the appropriate selection index should relate to wood and/or pulpwood properties for the estimation of whole-tree properties.

Cell-type proportions in wood such as vessels, fibers,and ray and axial parenchyma cells, have been recognized as an important trait for both solid wood and pulp properties in hardwoods (Amidon 1981; Zobel and van Buijtenen 1989;Ona et al.2001).High fiber percentages in Caesalpinia echinata Lam. provide higher musical bow stiffness (Alves et al. 2008). Fiber percentage also significantly relates to the MOR (modulus of rupture) of solid wood in Pseudolachnostylis maprounaefolia Pax. (Uetimane and Ali 2011), to tensile strength in Quercus liaotungensis Koidz. (Zhang and Zhong 1992), and to compression strength in Casuarina equisetifolia Linn.(Chowdhury et al. 2012). Axial parenchyma percentage relates significantly to tensile strength of Q. liaotungensis(Zhang and Zhong 1992). Ray parenchyma correlates to unit shrinkage, total shrinkage, and residual collapse in Eucalyptus urophylla S. T. Blake, E. grandis W. Hill ex Maiden., and E. urophylla × E. grandis (Wu et al. 2006).In addition, cell-type proportions are a crucial anatomical parameter toforecast pulp yields,and high fiber percentage is required for good yields (Pirralho et al. 2014). Vessel elements have a major effect on paper properties (Colley 1973; Colley and Ward 1976; Ogata 1978; Malan et al.1994).Ray and axial parenchyma levels significantly relate to many pulp properties such as yield, unbleached brightness, and Kappa number,1Kappa number is an indication of the residual lignin content or bleachability of wood pulp by a standardized analysis method and estimates the amount of chemicals required during bleaching, being this amount related to the lignin content of the pulp (Tarrés et al.2017).and fiber percentage relates to pulp yield in E.camaldulensis(Ona et al. 2001). Cell-type proportions such as fiber percentages are reported to have high heritability in E. grandis (Malan 1988) and could be used as selection indices for quality breeding.

With the increment core method, there is uncertainty as to which height will best represent the whole-tree value.Investigation of within-tree variation of cell-type proportions is necessary to decide the core-sampling position.Within-tree variation of cell-type proportions in Eucalyptus has been reported by Taylor (1973a, b), Sardinha and Hughes (1979), Malan and Gerischer (1987), Malan(1991), Rao et al. (2002), and Wu et al. (2006). However,there is little information on detailed axial variations of cell-type proportions, including ray and axial parenchyma percentages which correlate with many pulp properties(Ona et al. 2001). Furthermore, the pattern differences of within-tree variation of cell-type proportions have not been thoroughly investigated between trees or between species using statistical data analysis with a quantitative approach.Moreover,the most appropriate core-sampling position has not been reported for cell-type proportions on a whole-tree basis (Downes et al. 1997).

In this paper, the within-tree variation of cell-type proportions in E. camaldulensis and E. globulus were first examined and then the pattern differences in within-tree variations between trees and between species in both radial and axial directions by statistical data analysis. The representative height was determined to indicate whole-tree values of cell-type proportions for core sampling. The effect of height on pattern differences in within-tree variations of cell-type proportions between trees and between species was also studied as part of developing a quality breeding program for solid wood and pulpwood.

Materials and methods

Materials

Two trees each of 14-year-old E. camaldulensis and E.globulus grown at Manjimup, Western Australia, were sampled. The plantation site had annual average temperature of 15.5 °C and rainfall of 1000 mm.The sample trees,#1 and #2 of each species, possessed average growth characteristic (average tree height in the plantation) and superior growth characteristic(higher tree height than other individuals), respectively, under the same silvicultural conditions.Height(H)and diameter at breast height(DBH)were, respectively, E. camaldulensis #1: 15.2 m, 18.8 cm;#2:18.1 m,23.5 cm;E.globulus#1:19.9 m,24.4 cm;and#2: 30.0 m, 23.8 cm.

Debarked discs 6-cm thick were cut from the sample trees at 1-m intervals,starting from 0.3 m above the ground up to a diameter of 8 cm. Two 2-cm width strips were cut from the center of each disc. One strip was divided into 2-cm from pith to bark, and 2 cm (radial) × 2 cm (tangential) × 6 cm (axial) blocks were obtained. These blocks were fixed with ethanol/water and used for the measurement of cell-type proportions.

Measurement of cell-type proportions

Tangential sections 15 μm thickness were cut with a microtome from each block, stained with safranin and mounted in Bioleit (Ohken Shoji, Tokyo, Japan). Images were taken with an Olympus BH-2 light microscope(Olympus, Tokyo, Japan) equipped with a CCD camera CK3800 (Meiji Techno, Saitama, Japan), and incorporated to a personal computer for image analysis. The cell-type proportions were measured using a point counting method according to Taylor (1973b) and Denne and Hale (1999).Twenty-four digital images were taken for a tangential section,and twenty-five grid points arranged symmetrically at 50 μm intervals were drawn on each image using Adobe Photoshop software (Adobe Systems Incorporated, San Jose, CA, USA). The cell types were categorized into vessel, fiber, ray parenchyma and axial parenchyma. The cell-type proportions in each category were calculated as a percentage of a total count (600 points). One pixel was approximately 0.6 μm.

Statistical analysis

The difference of patterns in within-tree variations of celltype proportions was investigated between trees and between species for both radial and axial directions using statistical data analysis (Ohshima et al. 2005a). A linear regression was obtained for radial variation at a fixed distance from the pith and for axial variation at a fixed height above the ground. The slopes for one tree and for one species were sorted as one data set and used to investigate the pattern differences in within-tree variations of cell-type proportions between trees and between species. This was carried out in both radial and axial directions by the Moses test of extreme reactions using the R version 3.1.1(R Core Team 2014). The sample data without data trimming were used. The Moses test is one of the nonparametric tests for two-sample independent sets, and is used in experimental studies which assume that the treatment variables will affect the subjects in a positive or negative manner, creating a polarizing effect (Moses 1952). To compare the pattern differences in within-tree variation,it often happens that one tree or species is normally distributed and the other is polarized at some extreme(Ohshima et al.2005a).In this instance, it is feasible that both samples have the same central tendency of within-tree variation and might not be significantly different by some tests which highlight differences in central tendencies. The Moses test focuses on the differences of extreme tendencies in the distribution tails.If the possibility related to the Moses test is less than the desired significant level, then the two sample sets are not different. This study performed the Moses test at 0.05 significance level.

Whole-tree and disc values of cell-type proportions

The whole-tree and disc values of cell-type proportions were calculated according to Ohshima et al. (2005a). The unmeasured regions between two axially adjacent measured regions were assumed to have the average values of the measured regions. Other unmeasured regions were assumed as the same values of radially adjacent measured regions or the calculated regions described above. Further,each measured or calculated region was considered to own the same quality as its concentric circled section.Based on these, whole-tree and disc values at given heights are weighted values by wood volume. Whole-disc values at 0.8, 1.8, and 2.8 m above the ground are presented as the average values of two adjacent disc values.

Results and discussion

The cell-type data

Data of cell-types are summarized in Table 1. The linear regression lines at specific heights above the ground are plotted for vessel percentage against the distance from the pith for E. camaldulensis #1 (Fig. 1). The slopes of regression lines in both radial and axial directions are summarized in Tables 2 and 3,respectively.The slopes for one tree and for one species were treated as one data set.The Moses test of extreme reactions was performed using the data for pattern differences in within-tree variations of cell-type proportions between trees and between species in both radial and axial directions.

Within-tree variations of vessel percentage

Within-tree variations of vessel percentage for E. camaldulensis and E. globulus are shown in Figs. 2 and 3,respectively. For E. camaldulensis, both #1 and #2 trees had high vessel contents in the upper and outer regions of the trunk, with tree #1 with higher values than #2. There was a pattern of increasing vessel percentage from pith to bark in the radial direction, and from bottom to top in the axial direction. Tree-to-tree variation was small in E.camaldulensis. In contrast, E. globulus trees had no clear pattern of vessel percentages in the trunk and tree-to-tree variation was small. Consequently, significant differences between species were observed for vessel percentages in the axial direction (Table 4).

The pattern of increasing vessel numbers with distance from the pith in the radial direction has been reported for E.globulus(Hudson et al.1998;Ramírez et al.2009),and for E. urophylla, E. grandis, and E. urophylla × E. grandis(Wu et al.2006).In addition,a pattern of increasing vessel percentages from the ground to the top of the tree in the axial direction was reported for E. dalrympleana Maiden.(Hillis 1978). A similar pattern was observed in this study for E. camaldulensis and was not affected by growth rate differences. On the other hand, in the radial direction, no constant pattern of vessel percentage has been reported for E. globulus (Leal et al. 2003), for E. grandis (Taylor 1973a; Malan 1991; Malan and Hoon 1992; Palermo et al.2015) and for E. viminalis Labill. (Nicholls and Phillips 1970). In the axial direction, Taylor (1973b) reported no obvious patterns of vessel percentage with height in E.grandis. Rao et al. (2002) also revealed that vessel percentage was not significantly different between heights above the ground in E. tereticornis Sm. Similarly, there was no clear or consistent pattern observed in this study for E. globulus.

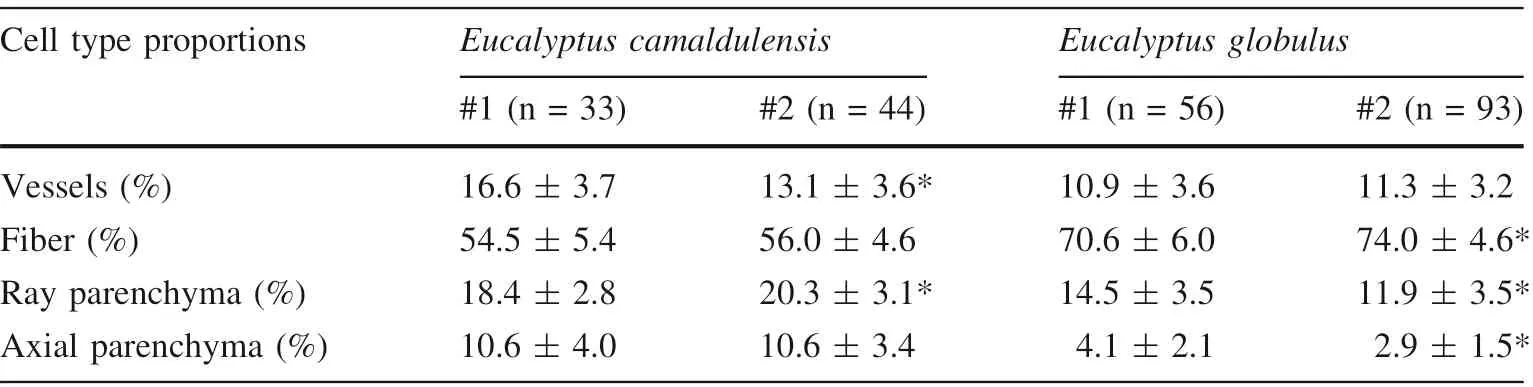
Table 1 Data of cell-type proportions (average ± SD)

Fig. 1 Plots of linear regression lines at given heights for vessel percentage against distances from pith in Eucalyptus camaldulensis tree #1

Within-tree variation of vessel percentages could be the result of variations in either frequency or vessel diameters.The general pattern of vessel diameters in E.camaldulensis was similar to that in Ohshima et al.(2004).Consequently,vessel percentage is considered to depend mainly on diameter. No clear pattern of vessel percentage was observed in E. globulus. In contrast to E. camaldulensis,vessel numbers are thought to reflect vessel frequency,possibly due to the larger variations of frequencies compared to diameters as reported previously (Ohshima et al.2004).

A higher percentage of vessel tofiber cells tends to increase vessel picking2Vessel picking is a problem for pulp and paper production of hardwood. Vessel elements are picked from the surface of paper during the printing process and deposited on the printing surface,causing ink-free spots on the printed page (Hudson et al. 1998).in E. deglupta Blume (Colley and Ward 1976). The results suggest that paper from E.camaldulensis #2 may have better printability than E.camaldulensis #1 because of less vessel picking. On the other hand, vessel percentage had no significant relationship with the MOE (modulus of elasticity) of clear wood and veneer of E. nitens H. Deane & Maiden (McKenzie et al. 2003). Vessel percentage in Eucalyptus species only appears to affect pulp products.

Within-tree variations of fiber percentage

Within-tree variations of fiber percentage in E. camaldulensis and E. globulus are shown in Figs. 4 and 5,respectively. In E. camaldulensis, both trees had no consistent pattern of fiber percentage in radial and axial directions although at 4.0 m above the ground for tree #1 and 3.0 m for#2 there was particularly higher fiber content than in other regions of the trunk. However, tree-to-tree variation was small (Table 4). In comparison, E. globulus#1 had high fiber percentage in the upper and inner regions of the trunk,while#2 had high fiber percentage only in the outer regions. No clear pattern of fiber content for both trees was observed in the upper regions of the trunk in the radial direction; however, different patterns between trees were observed in lower regions. Decreasing fiber percentage was observed from pith to bark in the radial direction for tree #1, whereas increasing fiber levels were observed for tree #2. Although no clear pattern of fiber percentage was observed in the inner regions of the trunk in the axial direction, relatively uniform variation was observed in the outer regions.

Sardinha and Hughes(1979)reported no definite pattern of fiber percentage in the trunk for E. saligna Sm. Similarly, there was no clear pattern of fiber percentage in the radial direction for E. viminalis (Nicholls and Phillips 1970), E. urophylla (Wu et al. 2006), and E. grandis(Palermo et al. 2015), and in the axial direction for E.grandis (Taylor 1973b) and E. tereticornis (Rao et al.2002). Our results are in general agreement with these reports for both species except for the lower regions of E.globulus. On the other hand, a pattern of decreasing fiber percentage from pith to bark in the radial direction was reported in E.grandis(Wu et al.2006),similar to the lower regions of E. globulus #1. In contrast, a slight increase of fiber contents in the radial direction was reported for E.grandis (Taylor 1973a), similar to the lower regions of E.globulus #2. Taylor and Wooten (1973) noted that the increase in one cell type must necessarily be accompanied by the decrease of at least another. This suggests that the patterns of within-tree variation of fiber percentage in both species are affected by the increase of vessel percentage and the decrease of ray and axial parenchyma numbers.

Table 2 Slopes of linear regression lines from pith to bark at given heights in radial variation

Table 2 continued

Table 3 Slopes of linear regression lines from bottom to top at given distances from pith in axial variation

Fiber percentage is positively correlated to mechanical strength in Q. liaotungensis and C. equisetifolia (Zhang and Zhong 1992;Chowdhury et al.2012).Consequently,E.globulus was expected to have superior wood strength compared with E.camaldulensis because of its higher fiber content. In fact, E. globulus had higher strength properties compared to E. camaldulensis because MOR and MOE in E. camaldulensis and E. globulus were 96.7-110.0 MPa and 9.7-11.0 GPa, and 139.0-154.0 MPa and 19.4-21.7 GPa,respectively(Hillis 1978).These findings support our results.

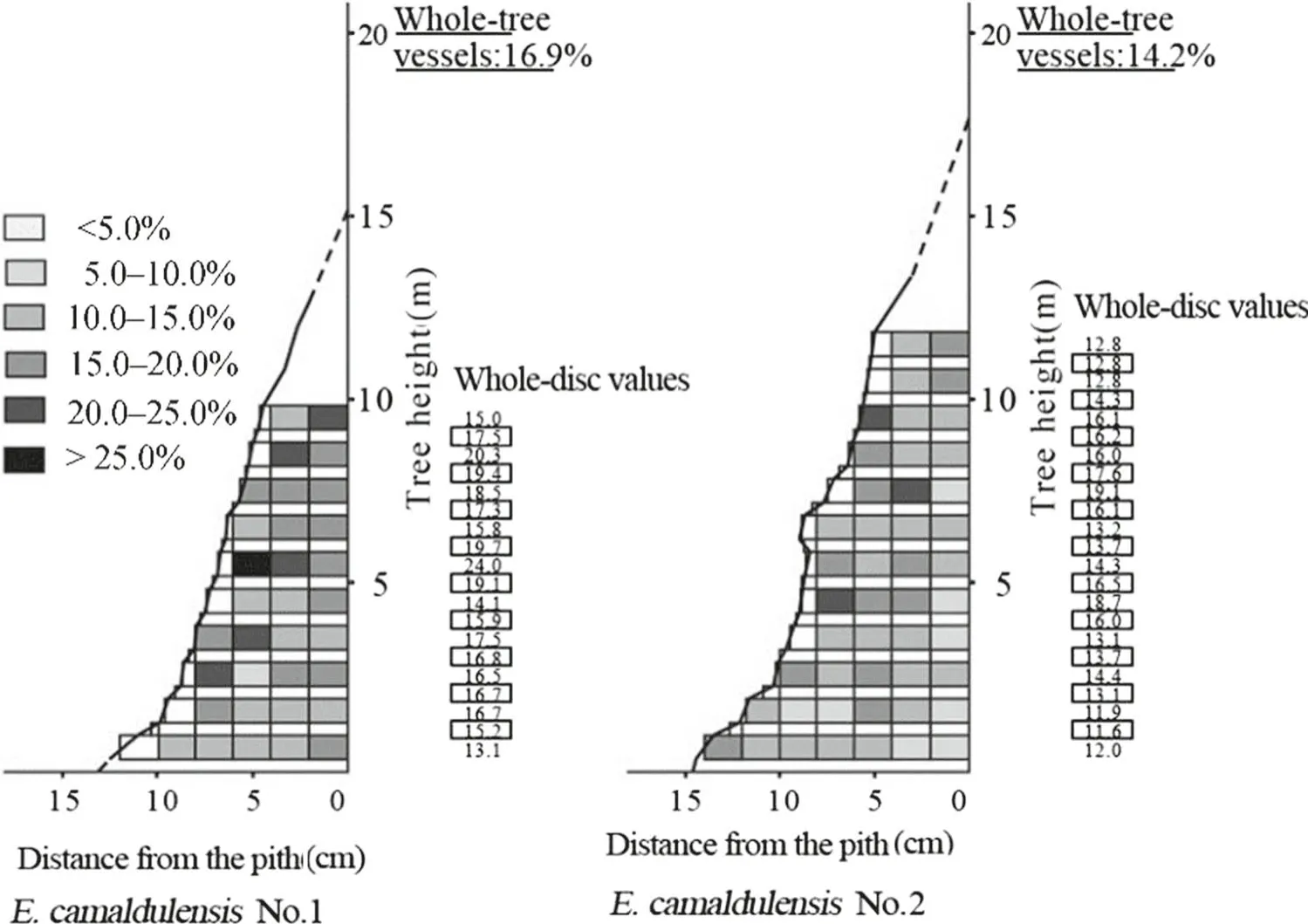
Fig. 2 Within-tree variation of vessel percentage in E.camaldulensis. Note: Unboxed digits represent whole-disc values calculated based on measured disc values at sampling heights; boxed digits represent whole-disc values calculated as the average values of measured disc values at two adjacent sampling heights

Fig. 3 Within-tree variation of vessel percentage in E.globulus(see note in Fig. 2)

Within-tree variation of ray parenchyma

Within-tree variations of ray parenchyma content in E.camaldulensis and E. globulus are shown in Figs. 6 and 7,respectively. Both E. camaldulensis trees had high ray parenchyma percentages in the lower and inner regions of the trunk. The lower regions of tree #2, in particular,showed high ray parenchyma numbers compared to theother regions of the trunk. No clear patterns of ray parenchyma percentages were observed in the radial and axial directions for tree #1, whereas a decreasing pattern of ray parenchyma content occurred from pith to bark in the radial direction, and from the bottom to the top in the axial direction for tree #2. From these observations, significant differences between trees were recognized for ray parenchyma in the radial direction of E. camaldulensis(Table 4). Similar to E. camaldulensis, E. globulus had high ray parenchyma contents in the lower and inner regions of the trunk. No clear patterns of ray parenchyma were observed in the radial direction for tree #1, while a decreasing pattern was observed from pith to bark in the radial direction for tree #2. A decreasing pattern was observed from bottom to the top in the axial direction for both trees. Consequently, for both species, the lower and inner regions of the trunk had higher ray parenchyma percentages compared to other regions.

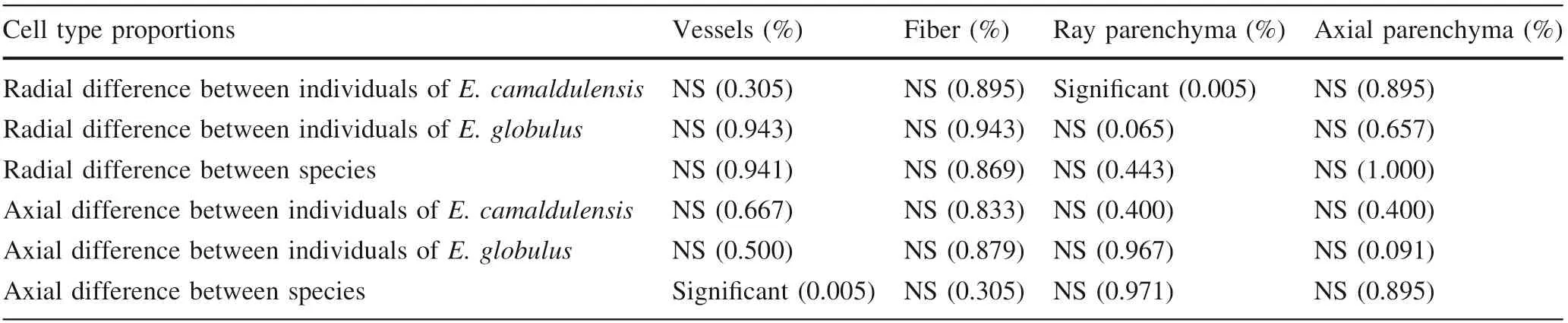
Table 4 Statistical differences of pattern in within-tree variations of cell-type proportions between trees and between species by the Moses test(P ＜0.05) and its probability

Fig. 4 Within-tree variation of fiber percentage in E.camaldulensis (see note in Fig. 2)

Previous studies on Eucalyptus showed no distinct patterns of ray parenchyma contents in both radial and axial directions (Taylor 1973a, b; Malan and Gerischer 1987;Rao et al. 2002; Palermo et al. 2015). Our results are similar to these studies for E. camaldulensis #1 and the radial direction of E.globulus#1.Wu et al.(2006)reported that ray parenchyma decreased radially from the pith toward the bark for E. urophylla, E. grandis, and E. urophylla × E. grandis, which is similar to our results for E.camaldulensis #2 and E. globulus #2. In addition, ray parenchyma tended to decrease from the bottom towards the top in the axial direction for other hardwoods such as Neolamarckia cadamba (Roxb.) Bosser (Ismail et al.1995).Our results for E.camaldulensis#2 and E.globulus#1 and #2 are in agreement with this study.

Fig. 5 Within-tree variation of fiber percentage in E. globulus(see note in Fig. 2)

Fig. 6 Within-tree variation of ray parenchyma percentage in E. camaldulensis (see note in Fig. 2)

Fig. 7 Within-tree variation of ray parenchyma percentage in E. globulus (see note in Fig. 2)

A high amount of ray parenchyma cells has adverse effects on wood strength (Zobel and van Buijtenen 1989).Ona et al. (2001) reported that ray parenchyma contents significantly correlated to pulp yield (negatively) and to pulp digestibility (positively). The results suggest that the upper and outer regions of the trunk will have higher board strength, higher pulp yield, and better digestibility than other regions.

Within-tree variation of axial parenchyma percentage

Within-tree variations of axial parenchyma cells in E.camaldulensis and E. globulus are shown in Figs. 8 and 9,respectively. In both species, the trees had high axial parenchyma cells in the lower and inner regions of the trunk.The lower regions of E.globulus#1,in particular,had high values compared to #2. In the radial direction, a weak pattern of decreasing axial parenchyma content from pith to bark was observed for both species. In the axial direction, no clear pattern was observed in E. camaldulensis,while a weak pattern of decreasing axial parenchyma percentage occurred from bottom to the top in E. globulus.

In the radial direction, decreasing patterns from pith to bark were reported for E. urophylla, E. grandis, and E.urophylla × E. grandis (Taylor 1973a, Wu et al. 2006).Similar patterns were observed in our samples.In the axial direction, no clear pattern was reported for E. grandis(Taylor 1973b) and E. tereticornis (Rao et al. 2002). Our results for E. camaldulensis agree with these studies. A weak pattern of decreasing axial parenchyma was reported for Alnus glutinosa (L.) Gaertn. (Vurdu and Bensend 1980), which is similar to our results for E. globulus.

Hillis (1990) reported that, in many fast growing Eucalyptus species,the first 10-15 years of growth constitutes juvenile wood.This suggests the sample trees in this study had predominantly juvenile wood. Bendtsen (1978) and Zobel and van Buijtenen (1989) also indicated that wood properties are quite variable in the juvenile zone and more constant in the mature zone. Consequently, within-tree variation of cell-type proportions in this study is thought to be influenced by the transition from juvenile to mature wood, and to be more constant with increasing tree age.

Axial parenchyma is significantly related to pulp yield(negatively) and to digestibility (positively) (Ona et al.2001), and thus the upper and outer regions of the trunk may have higher pulp yields and better digestibility than for other regions with similar levels of ray parenchyma.

Fig. 8 Within-tree variation of axial parenchyma percentage in E. camaldulensis (see note in Fig. 2)

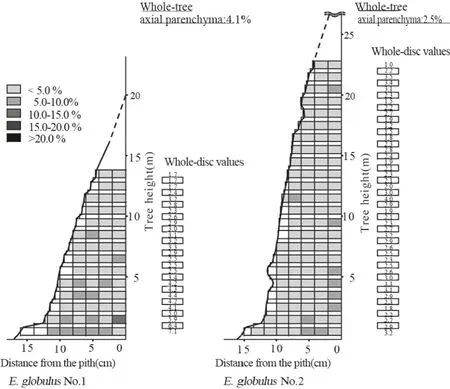
Fig. 9 Within-tree variation of axial parenchyma percentage in E. globulus (see note in Fig. 2)

Appropriate heights for sampling using an increment core

Heights to determine the whole-tree values of cell-type proportions were examined below 3.3 m by increment cores using an automatic drill as part of a test for practical quality breeding (Ona et al. 1996). Representative heights were defined as the height possessing the whole-disc value within the whole-tree value, ± 99% confidence limits by student t test according to Ohshima et al. (2005b). The whole-disc values of cell-types below 3.3 m in E. camaldulensis and E.globulus are summarized in Tables 5 and 6,respectively.

Common representative heights between trees are: E.camaldulensis, for vessels, 1.8-3.3 m; fiber, 0.8 m; ray parenchyma cells, 0.8, 1.3 and 2.8 m; axial parenchyma,0.8-1.8 m;in E.globulus,for vessels,heights were 0.8 and 1.8 m; fiber, none; ray parenchyma, 2.8 m; axial parenchyma, 2.8 and 3.3 m. Consequently, common representative heights for cell-type proportions are 0.8 m above the ground for E. camaldulensis (except for vessels), and 2.8 m for E. globulus (except for vessels and fibers).

No significant representative height for fiber levels was found for E. globulus, possibly due to the large difference between 99%confidence intervals of whole-tree values and whole-disc values derived from relatively small within-tree variations. On the other hand, when vessel and fiber percentages were grouped together to estimate common representative heights for cell-type proportions,the heights for vessel and fiber percentages were 2.8 and 3.3 m in E.globulus, respectively (Table 6). Therefore, the most appropriate heights to take increment cores are 0.8 m above the ground for E. camaldulensis, and 2.8 m for E.globulus. As noted previously, vessel percentage may be calculated as [100 - the other cell-type proportions]. The representative heights were not affected by differences in height growth and within-tree variations between trees.

Table 5 Whole-tree and disc values of cell-type proportions at given heights representing whole-tree values in E.camaldulensis

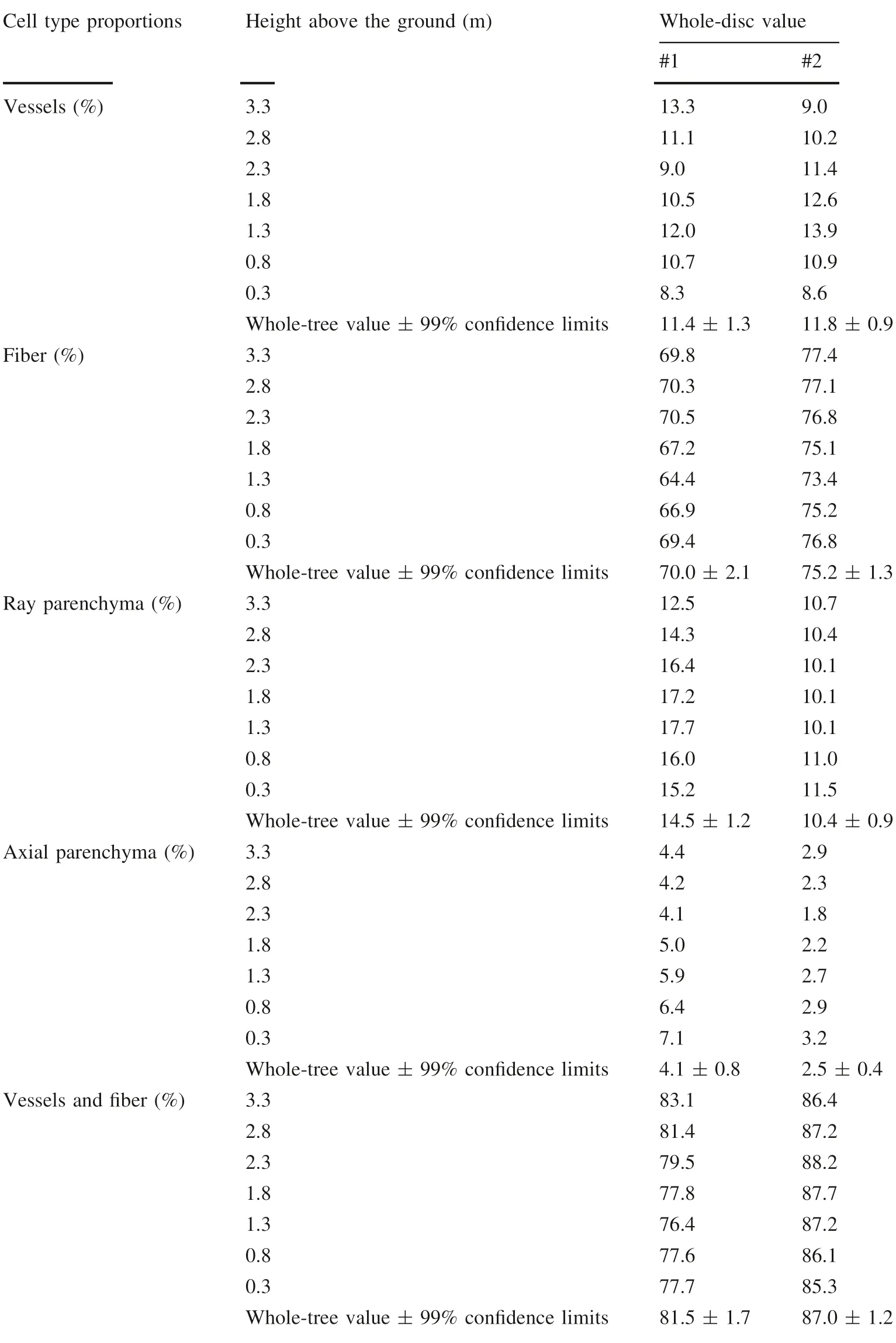
Table 6 Whole-tree and disc values of cell-type proportions at given heights representing whole-tree values in E.globulus

The representative height for sampling by increment cores is a major research subject in breeding programs,and is reported as breast height for Eucalyptus (Jorge et al.2000; Igartua et al. 2003; Schimleck et al. 2006; Muneri et al. 2011). However, Hudson et al. (2001) suggested that the representative height(whole-tree value ± 5%),against a percentage of tree height,was 10%(1.5 m)in E.globulus for all fiber dimensions, and 10% (1.38 m) in E. nitens for fiber length, coarseness, diameter, and lumen diameter.Raymond et al. (2001) also identified 1.1 m in E. globulus and 0.9 m in E. nitens as the recommended sampling height for predicting pulp yields. Although the results for E. camaldulensis were similar to those for E. nitens by Raymond et al.(2001),those of E. globulus were different from these reports. Previous results using the same trees showed the sample height for pulpwood productivity from properties analyzed chemically was 2.8 m above the ground for E. globulus (Ona et al. 1996). Including the results presented here, the most appropriate height for sampling by increment core is 2.8 m above the ground for

E. globulus.

Consequently, the use of a single increment core taken at the correct height is feasible for estimating whole-tree values of cell-type proportions. These measurements and determinations are to be encouraged for Eucalyptus breeding programs for the improvement of wood and pulp properties.

Conclusion

Within-tree variations of cell-type proportions and representative heights were examined to determine whole-tree values using E. camaldulensis and E. globulus. Cell-type proportions, including vessels, ray parenchyma, fiber and axial parenchyma were used as selection indices for the improvement of wood and pulp properties.

Within-tree variations of vessel percentage were higher in the upper and outer regions of the trunk of E. camaldulensis. There was no distinct pattern for E. globulus.Within-tree variations of fiber content had no clear pattern for both species,while those for ray and axial parenchyma were higher in the lower and inner regions of the trunk for both species. No significant pattern differences were observed between trees and between species in both radial and axial directions, excluding the axial variation between species for vessels and the radial variation for ray parenchyma between trees of E. camaldulensis.

The representative heights for estimating whole-tree values at ± 99% confidence limits were 0.8 m for E.camaldulensis and 2.8 m for E. globulus. Consequently,measurements using a single increment core taken at these heights could be used for breeding programs to improve wood and pulp quality of E.camaldulensis and E.globulus.

AcknowledgementsThe authors appreciate the Department of Conservation and Land Management (Western Australia) for sample supplements and Mr. K. Adachi, Faculty of Agriculture, Utsunomiya University, Japan, for his assistance with measurements.