Large-eddy simulation and linear acoustic modeling of entropy mode oscillations in a model combustor with coolant injection

Man ZHANG,An LIANG

AECC Commercial Aircraft Engine Co.,LTD,Shanghai 200241,China

KEYWORDS

Abstract Lean-burn combustor is particularly susceptible to combustion instability and the unsteady heat release is usually considered as the excitation of the self-maintained thermoacoustic oscillations.The transverse coolant injection is widely used to reduce the temperature of burnt gas,but on the other hand,it will introduce temperature fluctuation inside the combustor.Therefore,it is necessary to consider the influence of the coolant injection on combustion instability,and evaluate its dynamic feature.In this paper,Large-Eddy Simulation(LES)of the self-excited pressure oscillations in a model combustor with coolant injection is carried out.The analysis of transient flow characteristics and the identification of the pressure modes confirm that one of the low frequency pressure oscillations is related to entropy fluctuations,which is known as rumble combustion instability.The LES results show that transient coolant injection is another excitation of temperature fluctuation other than unsteady combustion.The amplitude of the entropy mode oscillation increases with increasing coolant air mass whereas the change of its frequency is insignificant.According to the major feature of entropy wave oscillation caused by coolant injection,a compact coolant injection model is proposed and applied in the One Dimensional(1D)Acoustic Network Method(ANM).Key correlations used in the model match well with LES data in low frequency range.This means that the coolant injection model is a complex one reflecting the interaction of the fluctuating coolant mass,pressure and temperature.Finally,the combustion instability frequencies and modes predicted by acoustic network method are also in good agreement with LES results.

1.Introduction

Lean combustion technology can significantly reduce the burnt gas temperature and the thermal NOxemissions.Therefore,it has been widely used in industrial applications,such as aero-engine combustors and ground-based gas turbine combustors.1,2However,lean combustion is prone to combustion instability problems,which is susceptible to synchronized pressure oscillations and can heavily damage combustion facilities.

According to the work of Lieuwen and Yang,3the basic principle of combustion instability is that the standing acoustic waves in the chamber are in phase with the unsteady heat release.However,there are different variances in the mechanism of thermo-acoustic instability for different frequency ranges.For instance,Dowling and Stow,4Zhu et al.,5,6and Eckstein and Sattelmayer7,8studied one kind of pressure oscillation mode in combustion chamber under low-power working conditions.The amplitude of the pressure oscillations of this mode is slightly weaker than that of the standing wave mode,but the corresponding time of the oscillation is in the same scale of flow transport time.For this kind of oscillation mode,entropy fluctuations are generated by the unsteady heat release and transport with flow.At the converging boundary of the choked nozzle,they are converted into pressure wave and propagate in opposite direction compared to the flow direction.The upstream propagating pressure wave leads to velocity fluctuation of the inlet air and fuel and aggravates the change of heat release rate.If this feedback loop is established,the self-excited rumble combustion instability can be maintained.

Previous studies mainly indicated that entropy waves are generated by the unsteady combustion heat release.Recently,Motheaul et al.9used Large-Eddy Simulation(LES)to study the combustion instability in a real configured aero-engine combustor.In their work,combustion instability of entropy mode was simulated and the coolant air injection was claimed to excite the entropy wave.Therefore,the pressure oscillation of entropy mode is more obvious compared to the former reports.Ichihashi et al.10,11investigated the combustion instability in a real industrial single swirler combustor.Among their results,the measurement of transient heat release shows that the coolant injection is involved in the combustion oscillation loop,and enhances the self-excited pressure oscillations.

In the previous works,coolant injection directly or indirectly contributes to unstable combustion.However,as demonstrated by Motheaul et al.,9the coolant injection is an important excitation of the entropy fluctuation in the dilution zone.

In steady flow,the coolant jet is utilized to dilute burnt gas and reduce the temperature at the inlet of the high-pressure turbine.Lefebvre and Ballal,12and Lieuwen and Yang13,14summarized the empirical correlation which is deduced from Bernoulli equation and widely used in industry,written as

where mc,Acand ρcare the mass flow rate,area and density of the coolant air,respectively,Cdis the effective coefficient,and panand pchstand for the static pressure in combustor annuls and the chamber,respectively.In general,about 65%of the total air mass is utilized for burning in a lean-burn combustor,25%of the air is designed for the liners cooling,and roughly 10%(or less)of air is assigned for dilution.15Although the development trend is to reduce the mass of the dilution air in the ultra-lean burn combustor in order to offer sufficient air for lean-burn and cool down the combustor wall,16dilution injection is still a conservative and effective approach to adjust the specific radial thermo-loading distribution in high-pressure turbines.

In transient flow,on the other hand,dilution jet is pulsed and influenced by the chamber pressure fluctuations.17Thus the diluted gas temperature in combustor also varies and the entropy wave is introduced.However,this effect on combustion instability is not directly and fully considered in the previous prediction methods.Until now,most interests are mainly focused on the averaged temperature and sound speed before and after the dilution hole.For example,the thermo-acoustic network model developed by Hsiao et al.18and Eckstein and Sattelmayer7,8is representation for this category.

In order to improve the prediction capability of the combustion instability caused by entropy wave,Motheaul et al.9proposed an acoustic boundary condition for solving the Helmholtz equation.This approach correlates density and acoustic velocity fluctuation in the flame region.Furthermore,the forwarded acoustic wave,which is reflection of the entropy wave choked exit,is modified via additional boundary conditions in order to enclose transport source term in Helmholtz equation.With this approach,the calculated frequencies of entropy combustion instability contain the interaction between entropy oscillation and acoustic wave.However,this method needs the density correlation as the input parameter which must be obtained from other LES calculations.

In this context,the work aims to study entropy mode oscillations with coolant injection and develop a fast and reliable prediction method for industry application.Thus,LES and One Dimensional(1D)Acoustic Network Method(ANM)are carried out.Firstly,LES is used to study self-excited oscillation in a model single swirler combustor.The frequency,mode characteristics and the transient physical process of the entropy mode oscillation are analyzed in detail.Subsequently,an analytical model is proposed to correlate the coolant injection with temperature and pressure fluctuations.Based on this model,a thermo-acoustic network including the influence of the transverse jet is established and validated by LES results.Furthermore,the predicted combustion instability frequency and pressure modes are both compared with the LES.

In the following part,this paper consists of four sections:LES method and the studied combustor are introduced first,the analysis of the LES results is presented next,the derivation of the coolant injection model is addressed in the third part,and the conclusion is drawn finally.

2.Computational setup

2.1.Brief description of LES method

The turbulent reacting flow is governed by the Navier-Stokes equations and the multi-species transport equation coupled with reaction sources,which are filtered through the spatial cells:

Eqs.(2)–(4)are the conservative laws for mass,momentum and energy where subscript i,j and k(see Eqs.(6),(7))stand for the different coordinate directions in Cartesian coordinate system.In Eq.(2),t stands for time,is the filtered density,and~u is the filtered velocity.In Eq.(3),δijis the Kronecker delta function,¯P is the filtered pressure,and~τijis the filtered stress tensor,which is modeled as

where μ is the molecular viscosity,and it is assumed to vary with temperature according to the Sutherland law.τSGSin Eq.(3)is the Sub-Grid Scale(SGS)stress tensor,which is expressed as

where Δ is the filter width and G is a filter kernel,which is modeled by Dynamic Smagorinsky-Lilly model.19,20

In Eq.(4),~h is the resolved total enthalpy,andis the resolved heat flux vector.In Eq.(5),andare the mass fraction and diffusion velocity of species m.is the production rate of species m,which is modeled with Dynamically Thickened Flame Model(DTFM).21–23Kerosene surrogate C10H20is used as fuel,and its burning rate is modeled with two-step scheme proposed by Franzelli et al.24

Here Prtand Sctare turbulent Prandtl number and Schmidt number,respectively.The other SGS terms such asandare neglected in this work.

2.2.Setup of test cases

A model laboratory-scale combustor studied by Pantangi et al.25is used in our work.This combustor consists of a radial air swirler,a centric fuel nozzle and a squared flame tube with a choked exit nozzle.Prevaporized gaseous kerosene is injected from the centric fuel nozzle and burnt with the surrounding air.The inlet of numerical domain is extended to the upstream of the fuel injector and swirler.In this manner,the detailed specifying of the turbulent fluctuations of in flow is neglected since it may affect the predictions.A mass flow inlet boundary condition is used for fuel injection system,while a pressure inlet boundary condition is employed for air supplement system to stabilize the proper combustion pressure inside the chamber,and a supersonic outlet boundary condition is imposed on the outlet nozzle.Non-reflecting wave boundary conductions are imposed on both of the fuel inlet and air inlet in order to get rid of the possible upstream disturbances introduced by the waves.An overview of the studied combustor is shown in Fig.1(a),and a sketch of the air swirler with grid resolution is displayed in Fig.1(b).

Details regarding the swirler geometry and operating conditions are listed in Table 1.In addition,measurements have been carried out downstream of the fuel injection nozzle,and the measured data has also been compared with LES results in this paper.With the aim to study the characteristics of the thermo-acoustic oscillation generated by the mixing jet,two squared holes(see Fig.1(c))are made symmetrically on the center of side wall of the flame tube.The ratio of coolant air to overall mass flow rate is denoted as MR and totally five different mass flow rates from the dilution holes are investigated,corresponding to the range of MR from 10%to 35%,as shown in Table 2.

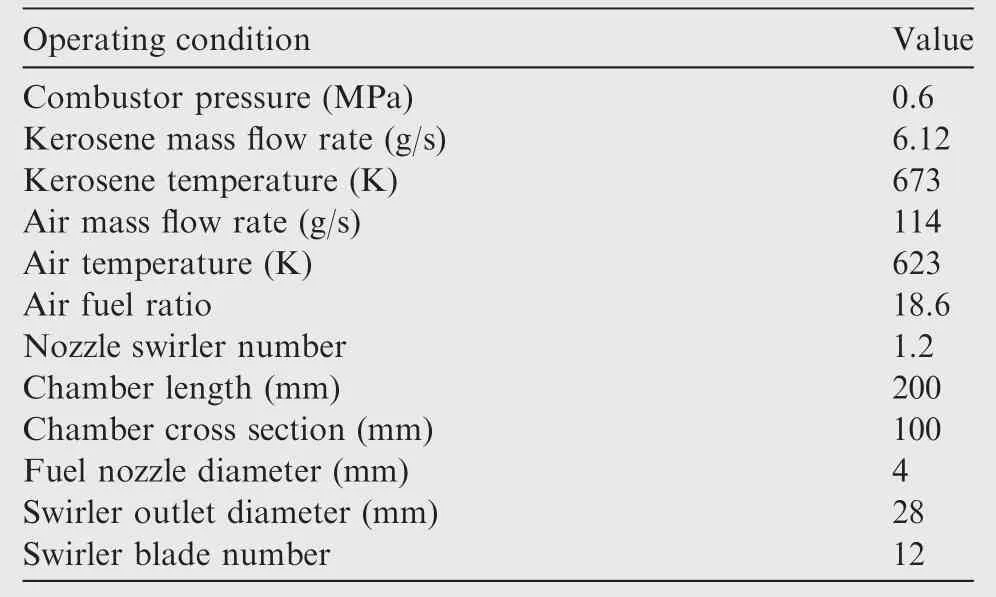
Table 1 In flow conditions and geometry information of a prevaporized combustion.25

Table 2 Nomination of studied cases.

2.3.Grid refinement and LES model validation

The structured grids(see Fig.1(b))are used for spatial resolution and the initial meshes are proposed according to the preliminary estimation of the distinction between combustion and the dilution region.The grid refinement is implemented by optimizing the resolved turbulence kinetic energy spectrum at the monitored point utilizing Komogorov’s-5/3 decay law(using fTfor turbulent frequency).After several iteration of modification,the refined mesh has approximately 9.0 million cells,as shown in Fig.2.

For further validation of LES results,mean velocity(¯uafor axial,¯urfor radial and¯utfor tangential velocity components)and Turbulent Kinetic Energy(TKE)along radial direction R at 5,10,15 and 20 mm downstream of the fuel injector are compared with experimental data from the study of Pantangi et al.,25as illustrated in Fig.3.LES results show good agreement with experimental results,which indicates that LES is applicable for this study.

3.LES result analysis

3.1.Time averaged flow field characteristics

Fig.3 Time averaged velocity and fluctuations at different locations from LES and measurement.

Fig.4 Mean velocity contour and streamlines on axial cross section of combustor.

Fig.5 Mean temperature contour on axial cross section of combustor.

Figs.4 and 5 show the mean axial velocity¯uacontour and mean temperature¯T contour on the cross section of the combustor with and without dilution.From Fig.4,it can be seen that the dilution jets cut off the central recirculation zone in the combustion chamber,and part of the dilution air is drawn into the upstream recirculation zone.Due to the influence of dilution injection,the temperature field also shows a discontinuity along the axial direction inside the combustor,which can be clearly identified in Fig.5.

Fig.6 depicts the distribution of transverse-sectionaveraged mean temperature along the normalized axial direction(axial direction x divided by chamber length L)of the combustor for different cases.It shows that burning zone is close to the front end of the flame tube.In Case 0 without coolant injection,the averaged temperature of the combustion zone reaches up to 2600 K.And it decreases gradually for increasing cooling air mass rate,whereas the temperature field after the coolant injection position is more evidently influenced.When the coolant air mass rate ratio is less than 20%,the dropping of the temperature in burning zone is less than 50 K,and the decrease is less than 200 K in dilution zone.For the cases with 35%and higher mass rate ratio of cooling air,the temperature in the burning zone decreases to 2000 K,and it goes down to 1700 K in dilution zone.This result reveals that there is a large amount of injection air swept into the burning zone,where the combustion has been influenced by the diluted air in burning zone.Since the mass rate ratio of coolant air between 10%and 20%performs similarly in temperature fields,Case 3 is used in the following part for in-depth analysis.

Fig.6 Mean temperature distribution of different cases along axial direction of combustor.

3.2.Dynamic pressure oscillations and acoustic modes identification

The temporal evolution of the dynamic pressure oscillations are recorded on the monitoring probe(see Fig.2)and then Fast Fourier Transform (FFT)approach is applied to convert these oscillations into frequency domain.The dynamic pressure spectrum of different test cases are listed in Fig.7.

As shown in Fig.7,three discrete frequencies can be resolved for the cases with mass rate ratio less than 20%.The lowest frequency is close to 90 Hz,and it does not change significantly with varying coolant mass.For the frequency around 140 Hz,it does not appear if there is no coolant injection and it can be clearly identified in the frequency spectrum when the coolant mass ratio exceeds 10%.If the coolant mass ratio keeps increasing up to 35%,it becomes the dominated frequency in the lower frequency range,whereas in the high frequency range,the pressure fluctuation around 2100 Hz can be recognized.The amplitude of this frequency is decreasing for increasing coolant mass ratio and its value drops down to 1785 Hz when the coolant mass ratio is equal to 35%(Case 5).

The pressure modes(represented by amplitude p′and normalized phase angle Φ/π)of the identified discrete frequencies for Case 0 and Case 3 are plotted in Figs.8 and 9,respectively.

For the lowest oscillation frequency,the variance of the amplitude and phase distribution along the axial direction is quite small,and thus the whole combustion chamber exhibits a global oscillation.This characteristic is similar to the mode of Helmholtz oscillation,which is related to the volume of the combustion chamber and the fuel nozzle area.The Helmholtz character frequency can be calculated as

Fig.7 Dynamic pressure spectrum for different cases.

Fig.8 Pressure modes of Case 0.

where fHstands for Helmholtz character frequency,is the speed of sound in background flow,Anozis the area of the fuel nozzle,Vchis the volume of the combustion chamber,and lnozis the length of the fuel nozzle.For the oscillation around 2000 Hz,the amplitude and phase present the typical feature of the first-order standing wave.The natural frequency of a 1D cavity with wall boundary at both ends can be written as

Fig.9 Pressure modes of Case 3.

Table 3 Predicted frequency with LES and analytical method.

where lchis the length of combustion chamber and n is the mode number.In Eq.(12),the transverse dimension is neglected due to the large aspect ratio of the combustor.According to Eqs.(11)and(12),the predicted Helmholtz frequency and natural frequency can be summarized in Table 3 together with LES results.

The pressure mode around 144 Hz has unique performance.The pressure amplitude within the first half of the combustor axial distance decreases with increasing distance from the nozzel,while it is almost constant after the dilution hole.The phase of the pressure fluctuation shows no significant change in the combustor.It is suspected that this frequency is related to entropy mode oscillation.In order to verify it,the convection time of flow from the dilution hole to the exit nozzle is calculated and it is about 0.0075 s,which is very close to the inverse of 144 Hz.Furthermore,the amplitude of the monitored fluctuated density ρ′is compared to the isentropic values(using fluctuated acoustic pressure p′divided by the square of background sound speed¯C2),as shown in Fig.10.

Fig.10 Tonitored density oscillation amplitude and calculated isentropic density mode of Case 3 at frequency of 144 Hz.

It can be seen from Fig.10 that the monitored density fluctuation along the axial of combustion chamber is much larger than the isentropic one,and their difference is contributed by the entropy wave.Moreover,the generation of entropy wave is located in the vicinity of the combustion zone and the dilution hole,and thus the coolant injection is another excitation of entropy fluctuation other than the unsteady combustion.Thus,the pressure fluctuation of 144 Hz is believed to be influenced by entropy wave.

3.3.Transient reacting flow characteristics

Fig.11 shows the transient temperature and vortex structure on the axial cross section of the combustion chamber with and without dilution,and Fig.12 shows the instantaneous fluctuated density distribution.In Fig.11,λ2method is used as the vortex core line detection algorithm.

It is shown in Figs.11 and 12 that the coolant injection is the origin of temperature fluctuation in the center of the combustor,as well as the density.In the case without dilution,the dominant highly transient flow structures including vortex shedding and density fluctuating mainly happen near the air swirler,but when the dilution takes place,it develops downstream towards the dilution zone.

For a better understanding of the flow dynamics caused by the coolant injection,Fig.13 shows the profile of mass weighted average density fluctuation as a function of time and its frequency f spectrum of Case 3 at the position of a quarter of tube length downstream from the dilution hole.

It can be found from Fig.13 that the density fluctuation has two primary frequencies in the downstream region from the dilution hole.On the basis of the analysis in Section 2.2,it can be identified that the first mode is related to the Helmholtz mode oscillation,and the second one corresponds to the entropy oscillation.It is also noted that the density oscillation corresponding to the isentropic acoustic pressure fluctuation is too weak to be recognized compared to the other low frequency oscillations.The instantaneous snapshots of the cross-sectional density fluctuations in different time series(see Fig.13(a))are displayed in Fig.14.Due to swirling motion of the flow inside the combustion chamber,the density fluctuation also shows a spiral pattern,which indicates that there is positive and negative density pulsation appearing alternately along the center axis.However,the cross-sectional-averaged density fluctuation shows a primary oscillation mode which corresponds to the 144 Hz oscillation.

Fig.11 Contour of transient temperature and iso-surface of vorticity without and with dilution(λ2= –5.5 × 108 s–2).

Fig.12 Contour of instantaneously fluctuated density without and with dilution.

Fig.13 Mass weighted average density fluctuation versus time and its frequency spectrum L/4 downstream from dilution hole.

Fig.14 Illustration of density fluctuation downstream from dilution hole.

4.Construction of acoustic network model involving coolant injection

The acoustic network transfer unit is applied to construct the whole combustion chamber with coolant injection.Because the pressure fluctuation of LES results has one-dimensional characteristic along the axial direction,the combustion chamber is simplified as a one-dimensional cavity with an acoustic choked nozzle.The non-homogeneity in transverse direction caused by coolant mixing is not considered in our calculation.The simplified combustor geometry used for acoustic analysis is shown in Fig.15.The following derivation of acoustic network is based on the assumption of planar wave decomposition.

4.1.Derivation of main formula

4.1.1.Basic equations and transfer units

The pressure,velocity and density fluctuation with uniform background flow can be written as

Fig.15 Acoustic domain of combustor with coolant injection.

where u′is the fluctuated velocity,A+and A-mean the amplitude of downstream and upstream propagating pressure respectively,AEis the entropy wave amplitude,ω is the annular frequency,i is imaginary unit,k±stand for the acoustic wave number for both downstream and upstream propagation,and kEis entropy wave number,which can be expressed as

where Ma is the Mach number.

Combining Eqs.(13)–(15),the solution of p′,u′and ρ′can be written as matrix,that is,

where Γ,P and Π are the transfer matrix.

If the conservation of acoustic flux across the two connected units is taken into account,the matching conditions can be written as

where subscript N and N+1 represent the N-th and N+1-th acoustic units,subscript 1 denotes the end location of the unit,and subscript 0 denotes the start location of the unit.The transfer matrix G for the N-th unit can be calculated as

where γ is specific heat ratio,and ANis the cross-sectional area of unit N.Unsteady heat release is not considered in this study.

4.1.2.Coolant injection model

In order to introduce the density fluctuation induced by coolant injection into the acoustic network,it is necessary to construct the relationship among the coolant mass fluctuation,combustor pressure and temperature fluctuation.If we linearize Eq.(1)and assume that the pressure fluctuation in combustor annuls is zero,we will have

The conservation of energy before and after the coolant injection can be addressed like

where cpis the specific heat at constant pressure and subscript N+c means the transfer unit after coolant hole.As the results of temperature fluctuation are ignored in the upstream burning zone,it has thatandUsing this assumption to linearize Eq.(25),we have

Additionally,the thermodynamic state equation is

and combining Eqs.(24)–(27),we can achieve

The density fluctuation has the isentropic and nonisentropic part and can be written as

Substitute Eq.(28)into Eq.(29),and the density fluctuation after the coolant injection hole can be finally expressed as

Its matrix form is

where the coolant injection matrix Bsis

4.1.3.Boundary condition of acoustic domain

The combustor inlet is supposed to be the wall boundary,and the acoustic condition u′=0 is applied at this position.If it is written in matrix form,

where inlet acoustic boundary matrix B0is

The combustor exit nozzle is choked,and the compact acoustic choked boundary condition is given by Marble and Candel,26like

If expressed in matrix,it is

where outlet acoustic boundary matrix BEis

Finally,combine Eqs.(18),(22),(31),(33)and(36),and the whole system can be written as

where subscript M is the total number of acoustic units.The system frequency is the eigen solution of Eq.(38).

4.2.Validation of key model

In the above mentioned thermo-acoustic network,key procedure is to rebuild the relationship among coolant injection,pressure and temperature fluctuation,as shown in Eqs.(24)and(28),which is verified by LES results.Fig.16(a)compares the coolant mass fluctuation calculated from LES and the transformed fluctuating pressure using Eq.(24),which shows good agreement with fluctuating coolant mass.Fig.16(b)plots the difference between the calculated pressure fluctuation and the derived temperature fluctuation from Eq.(28),and the dimensionless temperature fluctuation also shows good agreement with the transformation of the fluctuated pressure.

Fig.16 Validation of key model.

From Fig.16,it can be noticed that the characteristics of the low frequency fluctuation among the fluctuating coolant mass,pressure and temperature are very well captured by the coolant injection model given in Eqs.(24)and(28).The high frequency composition observed in Fig.16,which may be caused by high frequency acoustic waves,can be generated by the three-dimensional effect of the flow field as well.They are not our concern in the coolant injection model.However,as the entropy wave oscillation frequency is about 100 Hz,the proposed fluctuation correlation is applicable in low frequency range.

4.3.Comparison of instability frequencies and modes

All studied cases are analyzed with the acoustic network method proposed in this paper.The obtained instability frequencies and typical pressure modes are also compared with LES results,as shown in Fig.17.

As depicted in Fig.17,unstable frequency above 2000 Hz is the standing wave frequency,which can be predicted by the conventional thermo-acoustic network method.The oscillation frequency of entropy wave is close to 100 Hz,and the predicted results can be obtained only when the injection model is taken into account.Furthermore,the spatial distribution of the corresponding typical pressure modes shows that the result calculated with the acoustic network is in good agreement with LES calculation,especially in the downstream region of the combustor.

Fig.17 Comparison of LES and ANM results.

5.Conclusions

Large-eddy simulation and linear acoustic method are used to analyze the entropy combustion instability excited by the transverse coolant injection in a model combustor.A new coolant injection model,which involves the correlation among the fluctuating coolant mass,pressure and temperature,has been derived based on one dimensional conservation equations,and used for combustion instability prediction.The main conclusions in this paper are summarized as the following two aspects.

(1)The LES results indicate that the coolant injection has unsteady feature in the combustor,which can enhance the entropy combustion instability other than the unsteady heat release.The amplitude of the entropy mode oscillation increases with the increasing coolant air mass,while the frequency remains constant which is close to the inverse of the time scale corresponding to the flow transport from the dilution hole to the combustor exit nozzle.

(2)The correlation among the fluctuating coolant mass,pressure and temperature is validated by LES results.The comparison shows that in low frequency range,the proposed model can perform good simulation of the major unsteady feature of the coolant injection and its influence on the temperature field.By combining the coolant injection model and the acoustic network method,the combustion instability frequencies and modes are successfully predicted and agree well with the LES results.

Acknowledgement

The funding of the National Natural Science Foundation of China(No.51776191)is gratefully acknowledged.