基于本构模型的颗粒饲料成型特性研究
2017-12-20孔丹丹王红英
陈 啸,孔丹丹,王红英,方 鹏
基于本构模型的颗粒饲料成型特性研究
陈 啸,孔丹丹,王红英※,方 鹏
(中国农业大学工学院,农业部国家农产品加工技术装备研发分中心,北京 100083)
为研究饲料原料挤压成型特性,构建了非线性黏弹塑性颗粒饲料成型本构模型,针对主要饲料原料小麦,通过分析成型试验因素对其本构模型系数及其表征的流变学特性的影响,研究物料特性、加工参数等对小麦原料成型特性的影响规律,并分析了模型系数与颗粒成型质量的相关性关系。黏弹塑性本构模型由牛顿黏滞体(Newton viscous dashpot element)、应变硬化弹簧元件(strain hardening spring element)和库伦摩擦元件(Coulomb friction element)构成,基于小麦原料的实际成型试验完成模型构建;模型验证结果显示:数值结果中各应力区间模型决定系数R在0.99以上,模型值与试验值较为吻合,相对误差总平均值为3.378%,并通过χ检验进一步证明模型有效性;应用本构模型对小麦原料成型特性的分析结果显示,表征其流变学特性的模型系数表现出明显的应力函数规律,黏性系数绝对值随着挤压过程的进行逐渐减小,弹性模量值逐渐增大,集成塑性系数值则表现为先增后降,而各成型试验因素对模型系数产生了显著规律性影响,与宏观试验现象比较吻合;通过Pearson相关性分析可知颗粒成型密度、颗粒成型率、颗粒成型硬度等成型质量指标分别与黏性系数、塑性模量与弹性模量间达到了最高的相关系数,数值皆在0.80以上且为极显著相关性水平(<0.001),为基于本构模型对颗粒成型质量进行分析和控制提供依据。研究结果为颗粒饲料挤压成型特性研究提供了一种新方法和新角度,也为颗粒饲料的高效低耗生产和产品质量的改善提供基础数据和理论参考。
黏弹性;挤压;模型;饲料原料;颗粒成型特性;材料流变学
0 引 言
颗粒饲料生产过程中,饲料原料在粉碎、混合、调质后进行的挤压成型加工是一个重要工序。物料颗粒粒子在这一过程中持续受压并相互重叠搭接,颗粒体系由松散态逐渐固化黏结成型,表现出力学特性及粘弹塑性等成型特性的规律性变化;而颗粒饲料产品易产生裂纹,在降低生产效率的同时导致产品质量不稳定,这往往是因为对颗粒成型特性认识不够深入。因此,从材料流变学角度研究颗粒饲料成型特性,对饲料原料在挤压成型过程中表现出的力学特性及弹塑性、黏性[1]等流变特性进行探讨是极有必要的,对于分析颗粒饲料成型规律、优化成型工艺与设备具有理论指导意义。
目前,基于农业物料流变学对挤压成型特性的研究多集中在生物质领域:相关学者针对秸秆等不同生物质原料特性和应用目的及不同流变理论,构建了热黏塑性本构模型[2]、黏弹性本构模型[3]、Burgers本构模型[4]等进行研究;郑晓等则针对芝麻[5]、菜籽[6]等物料的挤压压榨过程,采用理论经验混合方法构建了蠕变黏塑性本构模型;卢立新等[7]提出表征果实非损伤条件下跌落冲击力学特征的非线性黏弹性本构模型,可确定类似果实动态流变特征参数;国外相关领域中,相关学者分别针对高纤维生物质原料[8]、花旗松[9]、小麦秸秆[10]等基于流变模型进行了质量指标预测、流变参数变化规律和应力松弛特性等的研究。目前颗粒饲料成型研究多集中在宏观加工参数的影响及加工工艺优化等方面,而应用流变学方法研究颗粒饲料成型特性则尚未见报道,且与上述生物质领域相关研究相比,一是饲料原料与生物质物料特性差别大[11-12];二是由于生物质成型颗粒应用目的,其研究多集中在应力松弛、蠕变,与饲料颗粒成型特性的研究重点有所不同;三是对于本构模型与加工过程、颗粒质量的规律性研究较少,未充分体现模型应用价值。而根据目前颗粒饲料原料应用现状[13-14],小麦替代玉米作为性价比更高的能量饲料来源具有较好的应用前景,而其在颗粒饲料加工过程中表现出的成型特性则有待探究。
由此,本文在分析颗粒饲料成型过程特点的基础上,构建了表征成型过程流变学特性的非线性黏弹塑性本构模型;以小麦这一主要饲料原料为研究对象,基于本构模型数值结果,分析其在挤压成型过程中表现出的黏弹性、塑性等流变学特征,进而研究物料特性、加工参数等试验因素对物料流变学特征及成型特性的影响规律;并由此分析本构模型各系数与成型颗粒质量的相关关系,为颗粒饲料挤压成型特性研究提供一种新方法,也为颗粒饲料的高效低耗生产和产品质量的改善提供理论参考。
1 材料与方法
1.1 试验材料与试验设计
试验对象小麦采集自北京首农畜牧发展有限公司饲料分公司,品种为德高1号,产地河南,初始含水率13%左右。
根据颗粒饲料成型加工过程主要影响因素[15],选取挤压成型试验因素及试验水平如下:以物料含水率(12%、15%、18%)、颗粒粒度(粉碎过Φ1.5、Φ2.0、Φ2.5 mm筛片孔径)作为物料参数,成型温度(60、75、80、85 ℃)、挤压载荷(2、3、4 kN)作为成型加工参数,设计为完全随机化4因素全面试验,重复试验3次[16]。
针对挤压成型后的颗粒产品裂纹、质量变化等问题,使用颗粒成型密度、颗粒成型率及颗粒成型硬度等质量指标进行分析研究;其中成型密度、成型率计算方法见式(1)、(2),成型硬度使用谷物硬度计测定(GWJ-1,托普仪器有限公司)。

式中为颗粒成型密度,g/cm3,为物料质量,g;为模孔内半径,cm;为物料初始高度,cm;为压杆位移,cm。

式中为颗粒成型率,%,m为成型颗粒质量,g。
1.2 挤压成型试验方法
使用试验用小型粉碎机(JFSD-100,上海沪粤明科学仪器有限公司)按试验安排对物料进行粉碎;待物料降至室温(25 ℃)时,测定粉碎物初始含水率[17],进而由初始含水率进行计算并对样品自然晾干或赋水达到目标含水率,并测定粉碎样品颗粒粒度情况[18](表1);封存制备好的样品于密封袋中并置于4 ℃试验用冷藏柜中保存。
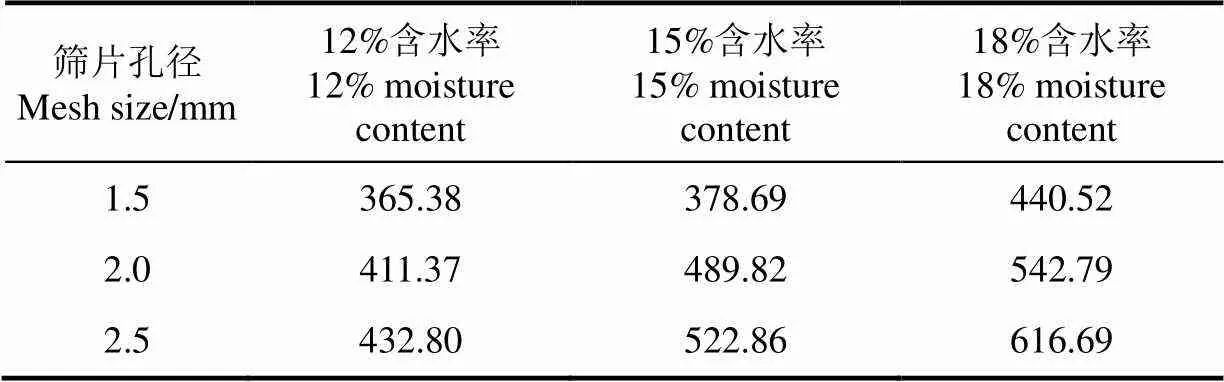
表1 不同筛片孔径、含水率物料颗粒粒度
结合相关文献研究方法[19-22],使用自行设计制作的挤压成型单孔闭式试验台完成挤压成型试验,通过设计加工公差使压杆直径尺寸略小于模孔直径,从而形成间隙便于排出模孔内空气从而降低对试验的影响;试验台搭载电子万能试验机(RGM 100 kN,深圳瑞格尔仪器有限公司)采集加载过程中的压力、时间、位移数据,通过陶瓷加热线圈和加载PID程序算法的智能温控器实现温度控制;根据物料密度范围和模孔容积将试验用物料质量定为15 g,通过预试验及参考文献[21-22],确定预热保温时间、压杆挤压速度分别为120 s和30 mm/min;试验时向模孔中缓慢均匀填料,将模孔及内容物预热至目标温度并进行保温后,压杆对物料进行挤压;试验完成后通过卸料挡板将成型颗粒从模孔中完整取出并测定各项质量指标;挤压成型试验台示意图如图1。

图1 挤压成型试验台示意图
2 黏弹塑性本构模型的建立
本构模型(constitutive model)是表征和描述材料受载荷作用时表现的变形行为和流变特性[23]的数学物理模型[24],可综合反映材料宏观力学性能和响应机理,并体现物料在工程应用过程中的本质属性。
2.1 挤压成型阶段的划分
在颗粒挤压成型过程的初始阶段,粉碎物粒子在惯性力的作用下得到重新排列[25],当其相对位置不再发生显著变化时,粒子间连接初步建立[26],之后的物料成型规律可认为由其本身黏弹塑性产生,物料亦可视为连续介质[2, 27]。由此,将挤压成型过程分为惯性变形阶段和黏弹塑性成型阶段[28],并采用应力-时间梯度法进行划分,将应力梯度值保持为正值的起点作为黏弹塑性成型阶段的开始(图2);由于惯性变形阶段中原料粒子松散且多处于无规则运动,物料成型特性受其初始状态、间隙气体等诸多随机因素影响而较不稳定,故本文主要在发生有效变形的黏弹塑性成型阶段进行本构模型的构建。
2.2 非线性黏弹塑性本构模型的构建
作为典型生物材料,饲料原料在挤压成型过程中表现出诸多复杂特性,如非线性、流变性、率相关性等[25],其黏弹塑性变形不仅与现时载荷有关,也与载荷作用时间有关[29],因此使用简单的线弹性体系进行表征是不准确的;而从连续介质力学理论出发,使用针对非线性材料、以流变学理论模型和试验数据为基础的唯象法[30-31]对本构模型进行构建,由此应用高分子物理学中黏弹塑性等概念,给出非线性流体应力、应变及应变速率间的关系,并以本构方程中的模型系数如黏度、模量等表征材料的特性,可在探讨系数物理意义的同时认识成型过程的本质规律[31-32]。

注:筛片孔径2.0 mm,成型温度80 ℃,挤压载荷3 kN,物料含水率15%。
2.2.1 流变特性与流变元件
传统弹塑性理论主要基于区分弹塑性的屈服理论,而饲料原料成型过程中,黏弹塑性变形是同时发生的,各阶段材料特性的不同使某种流变特性占主导地位[33];采用应变强化弹簧元件(strain hardening spring element)进行弹塑性表征,将弹性变形与塑性变形进行耦合,使用幂强化方式描述塑性变形的非线性特性,得到弹塑性本构方程为:

式中为该元件中相应应变响应,无量纲,σ1为弹塑性应力,MPa;为弹性模量(elastic modulus, MPa),为塑性模量(plastic modulus, MPa),为应变硬化指数(strain hardening exponent, 无量纲)。其中,弹性模量在本文的应用中代表物料受力压缩产生变形的难易程度,该系数的增加说明材料当前条件下可压缩变形量的降低,刚度增加,可以表征颗粒混合物在挤压成型过程中表现出的可压缩性的强弱。

依据近似本构关系[34](式中σ、ε为相对应应力,MPa及应变响应,无量纲),将塑性模量和应变强化指数写为集成塑性系数(combined plastic exponent, 无量纲):1/n,其越高表明发生的塑性应变程度越高。而从材料学角度看,塑性应变的提高表明撤去外力后可保持的变形量的提高,这对颗粒饲料成型质量控制有积极的指导意义。
与弹塑性相比,物料的黏性具有较为明显的率相关性和时间依赖性,由此将表征黏性的牛顿黏滞体(Newton viscous dashpot element)中的应变响应修正为其对加载时间的变化率,其本构方程为:

式中σ2为黏性应力,MPa;为黏性系数(viscous coefficient, MPa·s),为应力作用时间,s。本文中的黏性主要表征的是颗粒饲料成型过程中粒子间相互联结、黏合的能力,反映当前状态下材料受外力作用产生变形而不发生显著破坏的性能。
结合单孔成型试验条件和库伦摩擦定律可知,由物料与模孔壁面摩擦产生的剪切应力应为物料壁面摩擦系数、径向应力转换系数和轴向作用力的乘积,则表征摩擦特性的库伦摩擦元件(Coulomb friction element)本构方程为:

式中σ3为摩擦损耗应力,MPa;σ为摩擦损耗因子(frictional loss factor, MPa)。摩擦损耗因子可反映在挤压过程中物料的能量耗散特性,其来源主要包括颗粒间或颗粒与模孔壁面的摩擦和黏结等。
2.2.2 模型整体结构
与高分子、金属或陶瓷材料的流变学特点相比,饲料原料作为生物材料,其差异性主要表现为受压过程中黏弹塑性应变响应的模糊性[35],故传统流变学模型并不适用于颗粒饲料挤压成型过程,本文流变元件的并联式耦合方式(图3)可适应这一特点,则饲料原料非线性黏弹塑性本构方程为:

式中为黏弹塑性本构模型总应力,MPa。
2.3 非线性黏弹塑性本构模型的数值实现
为分析本构模型表征的随挤压成型过程变化的饲料原料流变学特性,将黏弹塑性成型试验过程按应力加载路径依次均分为5个应力区间,基于各应力区间的成型试验数据求取黏弹塑性本构模型数值结果;应用软件Matlab 2014a实现应力-时间梯度法划分成型阶段,以及SPSS 20.0非线性回归模块获得本构模型数值结果,数值分析方法采用序列二次编程法和Levenberg-Marquardt法,其平方和收敛性、参数收敛性皆设置为10-8;数据分析与作图等使用SPSS 20.0、Microsoft Excel 2010和OriginPro 9.1等软件完成。

图3 非线性黏弹塑性本构模型元件图
3 结果与分析
3.1 本构模型数值结果与验证
分析非线性黏弹塑性本构模型数值结果(表2),可知各黏弹塑性成型区间中模型决定系数R都在0.99以上;图4显示模型值与试验值吻合度较高,应力区间1-5中模型值与试验值的平均相对误差[36]分别为3.883%、1.798%、2.992%、1.496%和6.721%,总平均值为3.378%;根据式(8)进一步计算各区间内数据的χ值,依次为0.011、5.547、0.280、8.921和35.962,均远小于自由度为130、显著性为0.001时χ标准值(χ0.001(130)=184.37),综合以上判据说明本文构建的黏弹塑性本构模型精度较高,可较好地表征小麦原料成型过程中表现出的黏弹塑性等材料流变特性,由此可对其成型特性展开研究。

式中V为对应应力区间中模型值,V为相应试验值,为相应应力区间内数据数量。
由表2数据可以看出,随着挤压过程的进行,黏弹塑性本构模型系数及其表征的小麦原料成型流变特性呈规律性变化,表现出明显的应力函数特征:弹性模量随应力的增加而增大,说明物料的变形性能随挤压的进行逐渐减弱,物料整体压缩性逐渐下降至成型终点,而成型物料材料刚度则显著上升;耦合塑性模量和应变强化指数得到的集成塑性指数首先呈增大趋势,说明随着挤压应力的增高材料可保持的塑性变形量逐渐升高,而在高应力区间中出现了突降的现象;黏性系数绝对值逐渐增大说明物料颗粒间的黏结力随着挤压过程的进行逐渐增强,粒子间连接键逐步加固,而其为负值主要因为在黏性特性产生应变响应的过程中常伴有能量耗散的现象。

注:为降低前一应力区间的影响,将各区间应力值、应变响应值减去该区间初始值后使用;图中Vtest-1、Vmodel-1至Vtest-5、Vmodel-5分别代表随挤压过程进行,依次应力区间中的试验值、模型值。
3.2 试验因素对小麦原料成型流变特性的影响
为分析加工参数、物料特性参数等对小麦原料成型过程中表现出的流变学特性的影响[37],从而研究其成型特性规律,本节选取不同试验条件组合,调整目标因素水平对比研究其对本构模型参数的影响;此时其他试验因素水平相应固定为物料含水率15%,粉碎筛片孔径2.0 mm,成型温度80 ℃,挤压载荷3 kN。

表2 小麦物料挤压成型黏弹塑性本构模型数值结果
注:表中为小麦物料在试验条件为物料含水率15%、粉碎筛片孔径Φ2.0 mm、成型温度80 ℃、挤压载荷3 kN下求得;使用Duncan法进行多重比较,同列肩标上的不同小写字母表示组间差异显著(<0.05)。
Note: The data of these numerical results are from trail of moisture content is 15%, mesh size is 2.0 mm, forming temperature is 80 ℃, compression load is 3 kN; and these results are compared by Duncan’s multiple comparisons, different letters on shoulder indicate significant difference among groups (<0.05)
3.2.1 弹性模量
由图5可见,随着应力的增大,不同成型温度下的小麦物料弹性模量值差异显著增加,且在高温区段温度的升高会降低弹性模量,这说明在高温引入的热量作用下成型过程中颗粒粒子运动加剧,提高小麦原料受力压缩后产生的变形量,并在一定程度上降低物料刚度。随着物料由软橡胶态(典型弹性模量值3.4 MPa)[38]向硬橡胶态转化,小麦物料含水率的升高降低了其在挤压过程中的弹性模量,或因水分作为饲料原料成型时的重要软化剂,其含量的增加可以改善材料可压缩性和变形程度[39]。根据粉碎样品颗粒粒度试验数据(表1)可知,随着筛片孔径的降低,颗粒粒度随之下降。由图线可知随着挤压过程的进行,低颗粒粒度物料弹性模量处于较低水平。而随着挤压额定载荷的逐渐升高,小麦物料的弹性模量依次增高,表现出外力对物料成型可压缩性的突出影响。

注:其他试验因素水平为:图a,物料含水率15%,筛片孔径2.0 mm,挤压载荷3 kN;图b,筛片孔径2.0 mm,成型温度80 ℃,挤压载荷3 kN;图c,物料含水率15%,筛片孔径2.0 mm,成型温度80 ℃;图d,物料含水率15%,成型温度80 ℃,挤压载荷3 kN。
3.2.2 集成塑性系数
图6显示,含水率为15%、18%的小麦物料在挤压成型过程中呈现相似的高水平集成塑性系数值,说明物料含水率的升高可以引起较大的塑性应变,表现为当前状态下撤出外界应力后,材料可保持的变形量升高,体现出物料含水率对成型过程的积极作用[40]。较高的挤压载荷(4 kN)同样显著增大了集成塑性系数(<0.05),而中低水平时该系数值则较为接近,且颗粒粒度越小的物料具有的集成塑性系数值越大,说明提高挤压载荷和降低颗粒粒度可提高挤压成型残留变形量。同时,在高温高应力区间中,成型温度对集成塑性系数影响规律表现为正向趋势。

注:其他试验因素水平为:图a,筛片孔径2.0 mm,成型温度80 ℃,挤压载荷3 kN;图b,物料含水率15%,筛片孔径2.0 mm,成型温度80 ℃;图c,物料含水率15%,筛片孔径2.0 mm,挤压载荷3 kN;图d,物料含水率15%,成型温度80 ℃,挤压载荷3 kN。
3.2.3 黏性系数
图7显示,随着颗粒粒度的降低,小麦物料黏性系数绝对值逐渐增大,表现出颗粒粒子间结合力的显著提高,原因或为颗粒物粒径的减小增大了粒子间接触面积,使其能够更充分地进行水热交换反应以建立更为牢固的连接键[21]。成型温度对黏性系数绝对值的正向影响规律表明,更高的热量在软化颗粒粒子的同时,可以通过达到淀粉的玻璃化转变温度,激活物料中蛋白质等黏结剂作用等过程,促进粒子间的相互作用,显著提高物料颗粒的结合能力,因此成型颗粒能够更好地抵抗外力的破坏作用[41]。中高挤压载荷下的黏性系数绝对值相对处于较高水平,说明载荷的增加有利于物料黏性特性的发挥。而随着挤压过程的进行,含水率越高的小麦物料黏性系数绝对值反而越小,这说明过高含量的水分造成的颗粒间滑移会阻碍其结合作用。
3.2.4 摩擦损耗因子
由图8各图线变化趋势可以看出,试验因素对摩擦损耗因子的影响规律性并不明显,该值在成型过程中处于正负波动的状态,原因或为粉状小麦物料受载压缩过程中不断膨胀反弹的间断性变化[42];而其数值数量级与挤压应力、弹塑性模量等相比较小,表明在本文试验条件下,因颗粒间及颗粒与壁面摩擦产生的能量耗散现象并不显著。

注:其他试验因素水平为:图a,物料含水率15%,成型温度80 ℃,挤压载荷3 kN;图b,物料含水率15%,筛片孔径2.0 mm,挤压载荷3 kN;图c,物料含水率15%,筛片孔径2.0 mm,成型温度80 ℃;图d,筛片孔径2.0 mm,成型温度80 ℃,挤压载荷3 kN。

注:其他试验因素水平为:图a,物料含水率15%,筛片孔径2.0 mm,挤压载荷3 kN;图b,物料含水率15%,筛片孔径2.0 mm,成型温度80 ℃;图c,筛片孔径2.0 mm,成型温度80 ℃,挤压载荷3 kN;图d,物料含水率15%,成型温度80 ℃,挤压载荷3 kN。
3.3 本构模型系数与成型质量指标相关性分析
为探讨小麦原料成型流变特性与颗粒成型质量指标的关系[43],通过Pearson相关性分析得到小麦原料各试验条件下三项成型颗粒质量指标与挤压成型黏弹塑性本构模型系数两两间的相关关系(表3),通过比较发现,颗粒成型密度、颗粒成型率、颗粒成型硬度分别与黏性系数、塑性模量与弹性模量间的相关系数为最高,数值均大于0.80,并都达到了极显著的相关性水平(<0.001),可说明模型系数与相应质量指标达到了较高的相关关系;其中塑性模量对成型材料内部残余应力、变形恢复的影响较为显著,会导致颗粒成型后内部应力变化,引起膨胀变形从而产生裂纹甚至断裂,这或是其与颗粒成型率相关性较为显著的部分原因[25];由此可为使用黏弹塑性本构模型系数从材料流变学角度对颗粒产品成型质量进行分析和控制提供理论依据[44]。

表3 小麦成型本构模型系数与质量指标相关性分析
注:表中肩标**表示该相关性系数达到了极显著相关性水平(<0.001),肩标*表示达到显著性相关性水平(<0.05)。
Note: Two * on the shoulder of correlation data means it reaches extreme significant correlation level (<0.001), while one * means it reaches significant correlation level (<0.05), no * means it is not significantly correlated.
4 结 论
1)为研究颗粒饲料成型特性,基于流变学建模理论,构建了表征颗粒饲料挤压成型流变特性的非线性黏弹塑性本构模型,由应变硬化弹簧、牛顿黏滞体和库伦摩擦元件并联构成;以小麦原料为研究对象的本构模型数值验证结果显示,各应力区间模型决定系数R在0.99以上,相对误差总平均值为3.378%,模型值与试验值吻合度较高,并进一步通过χ检验综合说明该本构模型的精度和有效性。
2)在阐述模型系数的应力函数特性基础上,分析了各试验因素对表征小麦原料成型流变特性的本构模型系数的影响规律:弹性模量随含水率的升高逐渐降低,较高的挤压载荷显著增大了集成塑性系数,黏性系数绝对值则受到成型温度的正向规律影响,而摩擦损耗因子受各试验因素的影响并不明显等。
3)分析了颗粒成型质量指标与本构模型系数间的相关关系,其中颗粒成型密度、颗粒成型率、颗粒成型硬度分别与黏性系数、塑性模量与弹性模量间的相关系数为最高,皆大于0.80的同时都达到了极显著相关性水平(<0.001),反映了材料流变学特性与颗粒成型质量间的高度相关关系。
基于本文黏弹塑性本构模型分析的其他饲料原料(玉米、豆粕等)在颗粒饲料成型过程中表现出的成型特性,与本文分析的小麦成型特性规律是相似的,由此该模型及其分析方法是通用而具有一定可行性的,可以为颗粒饲料成型特性的研究提供一种新角度,并为通过该本构模型从材料流变学角度对颗粒成型过程、产品成型质量等进行分析和控制提供理论依据和基础数据,从而为颗粒饲料的高效低耗生产和产品质量的改善做出贡献。
[1] Molenda M, Montross M D. Mechanical properties of corn and soybean meal[J]. Transactions of American Society of Agricultural Engineers,2002, 45(6): 1929-1936.
[2] 孙启新,陈书法,董玉平. 秸秆类生物质成型热黏塑性本构模型构建[J]. 农业工程学报,2015,31(8):221-226. Sun Qixin, Chen Shufa, Dong Yuping. Establishment of thermo viscoplastic constitutive model for straw biomass briquetting[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2015, 31(8): 221-226. (in Chinese with English abstract)
[3] 霍丽丽,赵立欣,田宜水,等. 生物质颗粒燃料成型黏弹性本构模型[J]. 农业工程学报,2013,29(9):200-206. Huo Lili, Zhao Lixin, Tian Yishui, et al. Viscoelastic constitutive model of biomass pellet[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2013, 29(9): 200-206. (in Chinese with English abstract)
[4] 李汝莘,耿爱军,赵何,等. 碎玉米秸秆卷压过程的流变行为试验[J]. 农业工程学报,2012,28(18):30-35. Li Ruxin, Geng Aijun, Zhao He, et al. Rheologic behavior of chopped corn stalks during rotary compression[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2012, 28(18): 30-35. (in Chinese with English abstract)
[5] 郑晓,林国祥,尹芳,等. 芝麻、花生在压榨过程中非线性黏弹塑性模型与蠕变模拟[J]. 农业工程学报,2006,22(9):29-33. Zheng Xiao, Lin Guoxiang, Yin Fang, et al. Nonlinear viscous-elastic-plastic model and creep simulation of sesame and peanut[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2006, 22(9): 29-33. (in Chinese with English abstract)
[6] 郑晓,林国祥,李智,等.菜籽与菜籽仁的非线性黏弹塑性本构模型[J].农业机械学报,2005,36(11):87-91,101. Zheng Xiao, Lin Guoxiang, Li Zhi, et al. Nonlinear viscous-elastic-plasticity constitutive model of rapeseed and rapeseed kernel[J]. Transactions of the Chinese Society for Agricultural Machinery, 2005, 36(11): 87-91,101. (in Chinese with English abstract)
[7] 卢立新,王志伟. 跌落冲击下果实动态本构模型的构建与表征[J]. 农业工程学报,2007,23(4):238-241. Lu Lixin, Wang Zhiwei. Dynamic nonlinear viscoelastic model for the fruits under dropping impact[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2007, 23(4): 238-241. (in Chinese with English abstract)
[8] Song Xiaoxu, Zhang Meng, Pei Z J, et al. Ultrasonic vibration-assisted (UV-A) pelleting of wheat straw: A constitutive model for pellet density[J]. Ultrasonics, 2015, 60: 117-125.
[9] Lam Pak Sui, Lam Pak Yiu, Sokhansanj Shahab, et al. Mechanical and compositional characteristics of steam- treated Douglas fir () during pelletization[J]. Biomass and Bioenergy, 2013, 56: 116-126.
[10] Chen Longjian, Liao Na, Xing Li, et al. Description of wheat straw relaxation behavior based on a fractional-order constitutive model[J]. Agronomy Journal,2013, 105(1): 134-142.
[11] Holm Jens K, Henriksen Ulrik B, Hustad Johan E, et al. Toward an understanding of controlling parameters in softwood and hardwood pellets production[J]. Energy & Fuels, 2006, 20(6): 2686-2694.
[12] Holm Jens K, Henriksen Ulrik B, Wand Kim, et al. Experimental verification of novel pellet model using a single pelleter unit[J]. Energy & Fuels, 2007, 21(4): 2446-2449.
[13] 中国饲料工业协会信息中心. 2015年全国饲料工业生产形势简况[J]. 中国饲料,2016,(10):1-2.
[14] 中国饲料工业协会全国饲料工作办公室. 中国饲料工业年鉴[M]. 北京:中国商业出版社,2016.
[15] 曹康. 中国现代饲料工程学[M]. 上海:上海科学技术文献出版社,2014.
[16] Li Y, Puri V M, Manbeck H B. Elastic-viscoplastic cyclic constitutive model parameter determination and evaluation for wheat en-masse[J]. Transactions of the ASAE, 1990, 33(6):1984—1995.
[17] GB 5009.3-2016,食品安全国家标准食品中水分的测定[S].
[18] ANSI/ASAE S319.4 FEB2008,Method of Determining and Expressing Fineness of Feed Materials by Sieving[S].
[19] 黄晓鹏,万芳新,黄建龙,等. 基于挤压模拟试验的苜蓿草颗粒成型工艺参数优化[J]. 农业工程学报,2011,27(11):354-358. Huang Xiaopeng, Wan Fangxin, Huang Jianlong, et al. Parameter optimization of granulated alfalfa pelleting process based on extrusion simulation experiment[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2011, 27(11): 354-358. (in Chinese with English abstract)
[20] 吴劲锋,黄建龙,张维果,等. 苜蓿草粉制粒密度与挤出力模拟试验[J]. 农业机械学报,2007,38(1):68-71. Wu Jingfeng, Huang Jianlong, Zhang Weiguo, et al. Simulated experiment and model of pelletizing density and extruding force for alfalfa powder[J]. Transactions of the Chinese Society for Agricultural Machinery, 2007, 38(1): 68-71. (in Chinese with English abstract)
[21] Adapa P K, Tabil L, Schoenau G. Compression characteristics of selected ground agricultural biomass[J/OL]. Agricultural Engineering International:CIGR EJournal, 2009, 1347.
[22] Sokhansanj S, Mani S, Tabil L G. Evaluation of compaction equations applied to four biomass species[J]. Canadian Biosystems Engineering, 2004, 46(3): 55-61.
[23] 李瀚如,潘君拯. 农业流变学概论[M]. 北京:农业出版社,1990.
[24] Macosko Christopher W. Rheology: Principles, Measurements and Applications[M]. Canada: WILEY-VCH Inc, 1994.
[25] Faborode M O, Ocallaghan J R. A rheological model for the compaction of fibrous agricultural materials[J]. Journal of Agricultural Engineering Research, 1989, 42(3): 165-178.
[26] 董磊,盖超,董玉平.生物质液压成型影响因素分析[J].农业机械学报,2011,42(7):139-143. Dong Lei, Gai Chao, Dong Yuping. Numerical study on process and influencing factors of biomass hydraulic briquetting[J]. Transactions of the Chinese Society for Agricultural Machinery, 2011, 42(7): 139-143. (in Chinese with English abstract)
[27] 孙清,白红春,赵旭,等. 蜂窝状生物质燃料固化成型有限元分析[J]. 农业机械学报,2009,40(2):107-109. Sun Qing, Bai Hongchun, Zhao Xu, et al. Finite element analysis of honeycomb biomass fuel press briquettin[J]. Transactions of the Chinese Society for Agricultural Machinery, 2009, 42(2): 107-109. (in Chinese with English abstract)
[28] Faborode M O, Ocallaghan J R. Theoretical-analysis of the compression of fibrous agricultural materials[J]. Journal of Agricultural Engineering Research, 1986, 35(3): 175-191.
[29] Osobov V I. Theoretical principles of compressing fibrous plant materials[J]. Trudy Viskhom, 1967, 4(55): 221-265.
[30] Phan-Thien Nhan. Understanding Viscoelasticity[M]. London: Springer-Verlag Berlin Heidelberg, 2013.
[31] 杨挺青. 黏弹性理论及其应用[M]. 北京:科学出版社,2004.
[32] 张义同. 热黏弹性理论[M]. 天津:天津大学出版社,2002.
[33] 韩晓玲,翟之平,贺向新,等. 矿物质微量元素舔块压制成型过程的数值模拟[J]. 农业工程学报,2012,28(3):50-54.Han Xiaoling, Zhai Zhiping, He Xiangxin, et al. Numerical simulation of mineral microelement licking block press forming[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2012, 28(3): 50-54. (in Chinese with English abstract)
[34] Kovalchenko M S. A rheological model of pressing of powders[J]. Soviet Powder Metallurgy and Metal Ceramics, 1990, 29(9): 753-756.
[35] 范林,王春光,王洪波,等.揉碎玉米秸秆可压缩性研究[J].农业机械学报,2008,39(11):76-80. Fan Lin, Wang Chunguang, Wang Hongbo, et al. Study on the compressibility of maize straw rubbed[J]. Transactions of the Chinese Society for Agricultural Machinery, 2008, 39(11): 76-80. (in Chinese with English abstract)
[36] 刘汝宽,肖志红,张爱华,等. 基于松弛试验的光皮树果实冷态压榨流变模型[J]. 农业工程学报,2015,31(16):278-283. Liu Rukuan, Xiao Zhihong, Zhang Aihua, et al. Rheological model for swida wilsoniana fruits in process of cold pressing based on stress-relaxation tests[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2015, 31(16): 278-283. (in Chinese with English abstract)
[37] 冯坤,蔡纪宁,陈杰,等. 螺杆挤压连续汽爆玉米秸秆流变性分析[J]. 农业工程学报,2011,27(增刊2):11-14. Feng Kun, Cai Jining, Chen Jie, et al. Rheological analysis of corn straw pretreated by screw extrusion and continuous steam explosion[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2011, 27(Supp.2): 11-14. (in Chinese with English abstract)
[38] Mohsenin N N. Physical Properties of Plant and Animal Materials[M]. New York: Gordon and Breach Science Publishers, 1986.
[39] Kaliyan Nalladurai, Morey R. Vance. Constitutive model for densification of corn stover and switchgrass[J]. Biosystems Engineering, 2009, 104(1): 47-63.
[40] Nathier-Dufour Nathalie, Angue Yasmine, Devaux Marie- Francoise, et al. Influence of wheat meal variability upon compacting behaviour during pelleting[J]. Animal Feed Science and Technology, 1995, 51(3): 255-268.
[41] 郭康权,赵东,查养社,等.植物材料压缩成型时粒子的变形及结合形式[J]. 农业工程学报,1995,11(1):138-143. Guo Kangquan, Zhao Dong, Cha Yangshe, et al. Particles deformation and combination model of the biomass materials in the compressing and forming process[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 1995, 11(1): 138-143. (in Chinese with English abstract)
[42] Peleg Kalman. A rheological model of nonlinear viscoplastic solids[J]. Journal of Rheology, 1983, 27(5): 411-431.
[43] 王菊霞,崔清亮,李红波,等. 基于流变特性的不同品种苹果果皮质地评价[J]. 农业工程学报,2016,32(21):305-314. Wang Juxia, Cui Qingliang, Li Hongbo, et al. Evaluation on peels texture of different apple cultivars based on rheological properties[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE). 2016. 32(21): 305-314. (in Chinese with English abstract)
[44] Ren S. Thermo-Hygro Rheological Behavior of Materials Used in the Manufacture of Wood-Based Composites[D]. U.S: Oregon State University, 1991.
陈 啸,孔丹丹,王红英,方 鹏. 基于本构模型的颗粒饲料成型特性研究[J]. 农业工程学报,2017,33(23):267-275. doi:10.11975/j.issn.1002-6819.2017.23.035 http://www.tcsae.org
Chen Xiao, Kong Dandan, Wang Hongying, Fang Peng. Analysis of forming properties based on pellet feed forming constitutive model[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2017, 33(23): 267-275. (in Chinese with English abstract) doi:10.11975/j.issn.1002-6819.2017.23.035 http://www.tcsae.org
Analysis of forming properties based on pellet feed forming constitutive model
Chen Xiao, Kong Dandan, Wang Hongying※, Fang Peng
(100083)
In the feed industry, through crushing, mixing and conditioning, feedstuffs are pelleted by mechanical equipment to obtain pellet products. In this compression process, the feed material particles are overlapped and staggered with each other, and the granular system is changed from the loose state into the curing bond with squeeze flow and the gap flow occurs synchronously. The mechanism for the pelleting process appears to be a regular relationship of stress and strain and other mechanical properties. Therefore, it is necessary to learn the stress-strain relationship and rheology properties of the feedstuffs during the compression process, and it is of theoretical significance to analyze the forming rules of granules and optimize the pelleting process and equipment. In this paper, based on characteristics and nonlinear properties of wheat as a feedstuff, a nonlinear visco-elasto-plastic constitutive model for characterization of rheological properties was constructed. Based on the experiment, and establishment and verification of the numerical model, the rheological properties such as viscoelasticity and plasticity of the feedstuff were analyzed by the model coefficients, and the influence of material properties and processing parameters on the rheological properties is studied. Also, the relationship between the rheological coefficients of the materials and the quality of the pellet is learned, which provides a new methodology for the study on the compression process of pellet feed. According to the process of pelleting, the factors in compression trials were selected including moisture, particle size (i.e., mesh size) and forming temperature, compression load. During the initial stage of the compression process, air between the particles was extruded by pressure and relative positions of particles are rearranged under the action of the inertial force. With the process progressing, the gap gradually decreases to infinitely small and the relative positions of the particles cease to change significantly, after which the deformation of the material can be considered as caused by its own viscoelastic properties, and therefore the compression process is divided into inertial deformation stage and visco-elasto-plastic compression stage for better description. According to the theory of rheology, the feedstuffs properties exhibited during the compression process are summarized as elastic-plastic, viscous and particle-wall friction, and represented by the strain hardening spring element, Newton viscous dashpot element, and Coulomb friction element, respectively. The elastic modulus shows the flexibility of the elastic element or the difficulty of compression. It turns out that the larger the coefficient, the more difficult the deformation and the stronger the stiffness of the material, which can stand for the strength and deformation properties. The improvement of the plastic strain showed by combined plastic exponent, which is coupled by plastic modulus and strain hardening exponent, indicates that the deformation can be maintained after the removal of the compressive stress, which has a special meaning on the quality control of the pellet feed. The viscous coefficient is mainly characterized by the ability of the particles to be bonded in the process of pelleting, thus reflecting the capacity of the formed pellet to stay stable under external force without significant damage in the current state. Meantime, the frictional loss factor can reflect the characteristics of energy dissipation, which mainly includes the friction and adhesion between the particles or the particles and the die wall. The compression stage was divided into 5 even ranges in order to study the various material properties along with the process. The numerical results of the constitutive model of wheat were obtained under 15% material moisture content, 2.0 mm mesh size, 80 ℃ forming temperature and 3 kN compression load, which showed that the determination coefficients (2) in all stress ranges were beyond 0.99 and the mean relative error values were 3.883%, 1.798%, 2.992%, 1.496%, and 6.721% in the ranges of 1-5, respectively. The comparative curve showed a good fit between the actual test value and model value; besides, the2examination showed that the2values of the data in each range were much smaller than the standard values in the degree of freedom of 130 and the significance of 0.001. Based on the above criteria, this constitutive model possesses a good performance that can be used to characterize the rheological properties of wheat. The constitutive model coefficients and the rheological properties of the feedstuff were characterized by regular changes, which showed obvious stress function: The elastic modulus increased with the increase of stress, indicating the deformation performance of the material was gradually weakened and the overall compressibility of the material decreased step by step to reach the end of forming, and the stiffness increased significantly. The increases of combined plastic exponent showed that the plastic deformation of the material increased gradually with a sudden downside in the last range. The absolute value of the viscosity coefficient increased gradually, which means that the bonding force among the material particles is enhanced with the progress of the forming, and the connection between the particles is strengthened therewith. For the influence of various forming factors, results were showed as follows: The increase in forming temperature can improve the elastic modulus, which means a better compression deformation capacity; with preferable softening, and bonding effect on the compressibility, higher moisture content can improve the combined plastic exponent and viscosity coefficient at the same time; the decrease of the particle size increases the absolute value of the viscosity coefficient, indicating its significant positive effect on the particle bonding force. The correlation relations between the pellet quality index and the constitutive model coefficients show that the pellet density, the pellet forming ratio and the pellet hardness were correlated with the viscous coefficient, plastic modulus, and elastic modulus respectively (<0.001), which owned the highest correlation coefficients that were all above 0.80, illustrating the close relationship between pellet product quality and feedstuff rheological properties. The nonlinear visco-elasto-plastic constitutive model constructed in this paper can provide a new methodology and perspective for the research of the process of feed pelleting, and provide theoretical basis for analyzing the particle forming characteristics, and product quality from the view of material rheology, which give contribution to the efficient and low-cost pellet production and the improvement of product quality.
viscoelasticity; extrusion; models; feedstuff; pellet forming properties; material rheology
10.11975/j.issn.1002-6819.2017.23.035
S816.8
A
1002-6819(2017)-23-0267-09
2017-08-09
2017-10-18
公益性行业(农业)科研专项(201203015)
陈 啸,北京人,博士生,主要从事饲料加工工艺技术研究。北京 中国农业大学工学院,100083。Email:chenxiaocau@gmail.com.
王红英,江西人,教授,博士生导师,主要从事饲料加工工艺技术与设备及饲料产品质量评价研究。北京 北京市海淀区清华东路17号,100083。Email:hongyingw@cau.edu.cn
中国农业工程学会会员:王红英(ED41200500S)
