超低比转速离心泵关阀启动瞬态特性分析

王 勇，陈 杰，刘厚林，邵 昌，张 翔

（1. 江苏大学国家水泵及系统工程技术研究中心，镇江 212013；2. 西华大学流体及动力机械教育部重点实验室，成都 610039）

超低比转速离心泵关阀启动瞬态特性分析

王 勇1，陈 杰1，刘厚林1，邵 昌1，张 翔2※

（1. 江苏大学国家水泵及系统工程技术研究中心，镇江 212013；2. 西华大学流体及动力机械教育部重点实验室，成都 610039）

为探究超低比转速离心泵关阀启动瞬态特性，该文以一台比转速为25的超低比转速离心泵为研究对象，在关死点工况下对稳态和关阀启动瞬态过程进行数值模拟，并与试验结果进行对比，研究表明：在关死点稳态工况下性能曲线与试验测得结果变化趋势相同，最大偏差小于5%，验证了数值模拟的准确性；关阀启动过程，不同启动加速度下启动过程的末期均出现一个冲击扬程；在相同转速时，稳态过程中间截面的静压分布、相对速度流线分布和进口管路内相对速度与关阀启动瞬态过程分布趋势存在差异。关阀启动瞬态过程内部流场的发展总体上滞后于关死点稳态过程内部流场。研究结果可为进一步研究超低比转速离心泵启动的瞬态过程特性提供参考。

离心泵；计算机仿真；试验；关死点工况；稳态特性；瞬态特性

0 引 言

超低比转速离心泵（简称超低比速泵）一般是指比转速小于等于30的离心泵，具有小流量、高扬程的特点，在航空航天、石油化工和农业灌溉等国民经济领域有着广泛的应用[1-6]。通常超低比速泵运行工况基本稳定，其流量、转速和压力等参数保持不变或者在一定范围内缓慢变化，但超低比速泵除了在稳态工况下运行之外，还需短期在各种各样的瞬态工况下运行，如启动过程，往往伴随着转速、流量和压力等参数的剧烈变化，严重时将造成机组设备冲击破坏。因此，研究泵开启过程的瞬态特性，对系统的安全和稳定运行具有重要意义。

目前已有学者对非设计工况下离心泵内部瞬态流动特性进行了研究[7-9]。Dazin等[10]发现采用角动量方程和能量方程可以很好的预测离心泵瞬态运行过程中的叶轮扭矩、扬程和功率。Farhadi等[11]建立了适用于预测离心泵启动过程瞬态特性的数学模型，该模型将整个系统内部的湍动能考虑在内，预测精度较高。Rochuon等[12]提出POD方法（proper orthogonal decomposition method）在提取瞬态流场的主导模式方面是有效的。李贵东等[13]基于Eulerian-Eulerian非均相流模型对离心泵内部流场进行三维瞬态数值模拟，结果表明当初始气相体积分数逐渐增大时，叶轮流道内流动紊乱，气液两相流动不均匀。张玉良等[14]研究低比转速离心泵流量突然减小瞬态过程的外特性和内流场，发现变工况过程结束后的稳定流量越小，瞬态效应愈发明显。王玉川等[15]基于RNG k-ε湍流模型和滑移网格，对不同工况下离心泵内部瞬态流场进行数值模拟，模拟得到的扬程和效率曲线与试验结果吻合较好。韩伟等[16]以导叶式离心泵为研究对象，研究过渡过程动静叶栅内固液两相流的瞬态流动特性，研究表明：动叶栅流道内的涡持续产生、合并、破碎和耗散，使得动叶进口处的流动滞止，导致动叶进口逐渐产生旋涡。

Thanapandi等[17-18]以较低的启动加速度对不同阀门开度的离心泵进行试验研究，研究发现在启动加速度很低的情况下，启动过程与准稳态理论基本相符。Wu等[19-24]研究发现快速启动使离心泵出现较高的扬程峰值，阀门开启过程中的流体流动加速效应使得其外特性曲线整体上位于稳态计算结果之下，流量瞬态增加过程的性能曲线低于稳态过程的性能曲线。刘竹青等[25]采用数值模拟手段研究双吸离心泵关阀启动过程的瞬态特性，研究发现全回路三维模型用来模拟泵启动过程得到的瞬态扬程相比于局部边界的数值模拟结果更为接近试验值。袁建平等[26]针对离心泵启动过程瞬态内部流场和结构场进行了双向流固耦合联合求解，获得了离心泵启动过程中瞬时效应对叶片应力和应变的影响规律。

综上所述，尽管国内外学者对离心泵瞬态过程做了大量研究工作，但是对超低比速泵的瞬态特性研究还较少，因此开展对超低比速泵启动过程瞬态特性的研究显得尤为重要。本文以一台比转速ns=25的超低比转速离心泵为研究对象，在关死点工况下对其分别进行稳态和瞬态关阀启动过程的数值计算，并与试验结果进行对比，分析不同启动加速度对瞬态启动过程中超低比转速泵非定常特性的影响。最后对启动时间为2 s的关阀启动过程内部流场进行分析并与稳态过程关死点工况的内流场进行对比，为深入研究超低比转速离心泵启动的瞬态过程特性提供参考。

1 超低比转速泵瞬态特性测试系统

1.1 试验测试系统

试验用超低比速泵主要参数如下：设计流量Qd=12.5 m3/h，扬程Hd=74 m，额定转速nd=2 950 r/min，比转速ns=25，叶轮入口直径Dj=68 mm，叶轮出口直径D2=228 mm，叶片出口宽度b2=7 mm，叶片数z=6，叶片出口安放角β2=40°，蜗壳基圆直径D3=245 mm。本试验在国家水泵及系统工程技术研究中心实验室闭式试验台上进行，图1为超低比速泵瞬态特性测试试验台结构示意图。该试验台包括真空泵1、真空罐3、电磁流量计5、模型泵7、稳压罐9和管路阀门等。

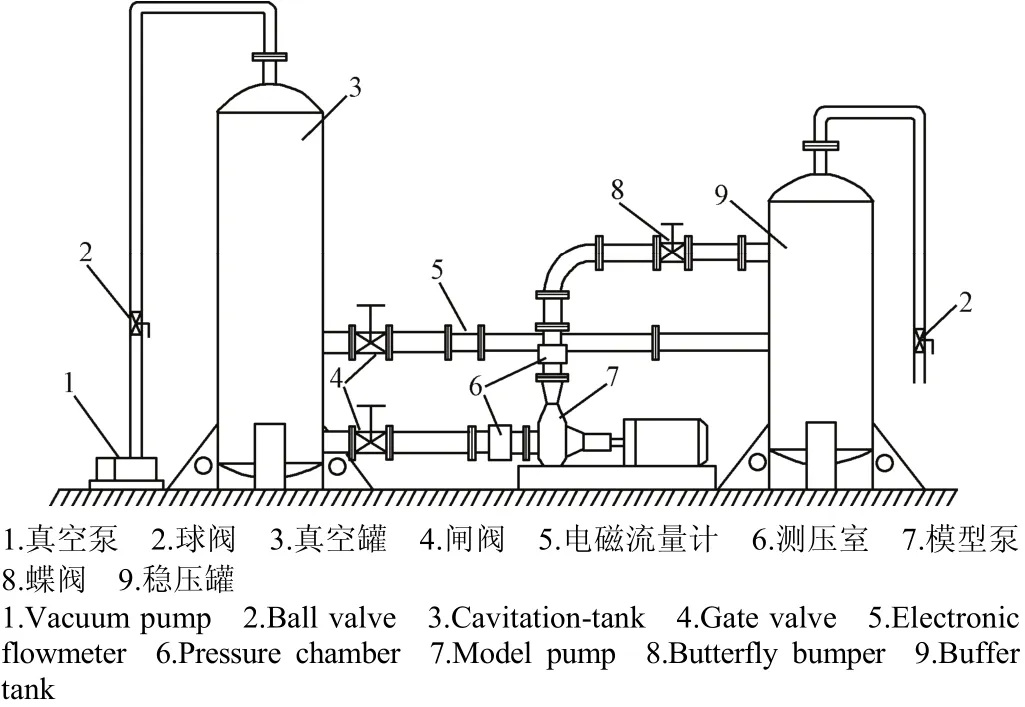
图1 瞬态特性测试试验台结构示意图Fig.1 Schematic diagram of transient characteristic experimental set-up

1.2 瞬态试验测量装置

1.2.1 压力脉动的测量

用于进口压力脉动测量的传感器型号为HM90-H10（武汉环宇高科测控有限公司），工作频率为0～2 kHz，量程为0～300 kPa，用于出口压力脉动测量的传感器型号为HY6305（武汉环宇高科测控有限公司），工作频率为0～2 kHz，量程为0～1 MPa，输出信号为大小4～20 mA的电流信号，精度为±0.25%。分别在离心泵进出口管道上一倍管径处进行打孔，孔径为10 mm，安装压力脉动传感器，用于测量模型泵进出口处的压力脉动。

1.2.2 电机瞬态转速测量装置

采用霍尔转速传感器对超低比转速离心泵启动过程中的转速变化过程进行监测。图2所示为CZ400型霍尔转速传感器安装示意图（上海传振电子科技有限公司，量程为0～20 Hz，精度为±1%，方波电压脉冲输出）。将霍尔传感器安装在可调节支架上，并将支架固定在离心泵底座上，同时在离心泵联轴器处裸露轴上安装一个感应磁铁，通过调节传感器上的安装螺母，使得传感器正对感应磁铁，并保持两者距离为0.5～3 mm，每当感应磁铁扫过霍尔转速传感器时，传感器便输出一个方波信号，2个脉冲方波间隔为一个叶轮旋转周期，从而得出此时刻叶轮的转速，当感应磁铁连续扫过传感器时，便可以得到一个时间段内电机轴转速的变化过程，从而拟合出超低比速泵启动过程叶轮转速的变化曲线，为后期关阀启动过程的数值模拟提供参考。

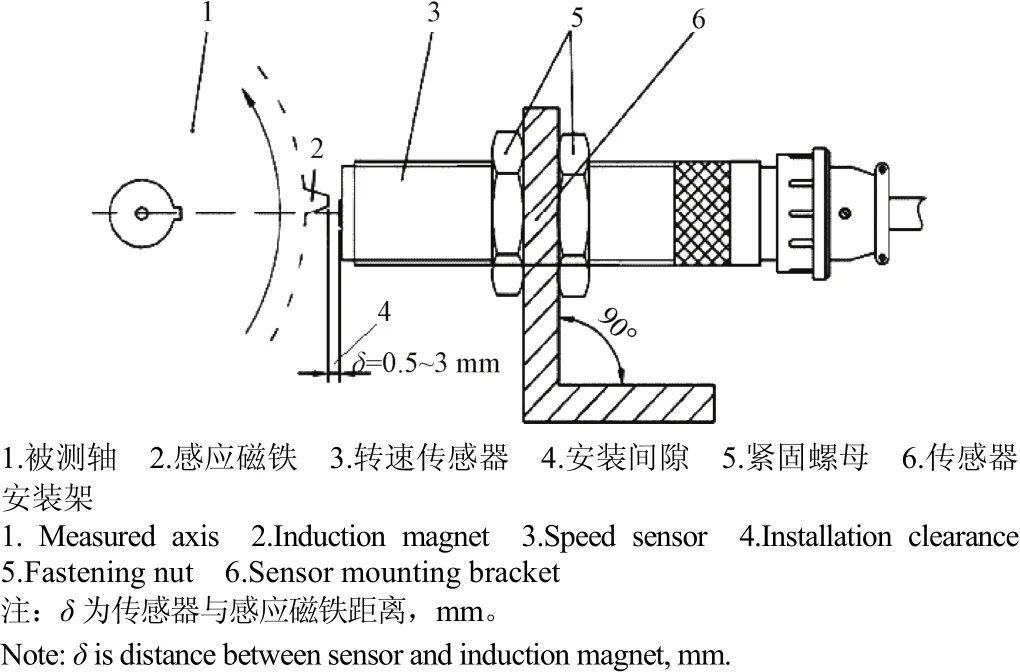
图2 传感器安装示意图Fig.2 Schematic diagram of sensor installation

2 数值计算模型及参数设置

2.1 计算域建模及网格划分

采用三维造型软件Pro/E 5.0对超低比转速模型泵整个流场计算域进行三维建模，计算域包括：进口延伸段、吸入室、叶轮水体、蜗壳水体和出口延伸段。其中进口延伸长度为离心泵进口直径的5倍，出口延伸长度为蜗壳出口直径的5倍，以保证流动的充分发展。采用商用软件ANSYS-ICEM 14.5对计算域进行网格划分，为了保证较高的网格质量和边界层网格尺寸，对所有计算域采用六面体结构化网格，经过网格无关性验证，最终网格数量为176.7万，模型泵计算域三维造型如图3所示。

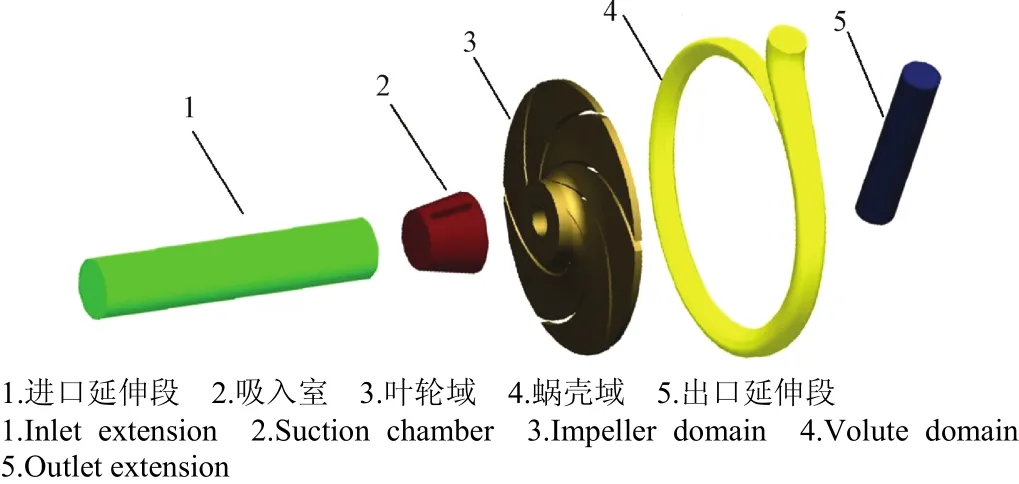
图3 计算域三维造型Fig.3 Three-dimension model of computational domain

2.2 湍流模型

SSTk-ω模型整合了k-ε模型和k-ω模型，在自由流区和边界层外层使用k-ε模型，在近避面区采用k-ω模型，在混合区通过一个加权函数F1来表示2种模型，并通过函数F2来修正函数F1在剪切流计算时的误差[27-28]。

Menter等[29]对SST k-ω湍流模型适用性的研究表明：该湍流模型能够较好的处理近壁面与自由流区的流动，对流场细节的处理能力较好。因此本文在超低比速泵稳态过程的数值计算中采用SST k-ω湍流模型完成雷诺方程组的封闭。

2.3 边界条件

采用商用软件ANSYS CFX 14.5全隐式耦合技术对方程组进行求解，计算模型边界条件设置为总压进口和质量流量出口，系统参考压力设置为0，固壁面边界设置成无滑移壁面，壁面粗糙度设置为20 μm。

求解过程中，关死点稳态过程求解时，选取时间步长为∆t=1.122 33×10-4s，即叶轮每旋转2°为1个时间步长，总计算步数为1 080步，即叶轮旋转6圈；关阀启动过程求解时，对不同启动加速度下的求解取相同的分析频率f=2 000 Hz，对应的时间步长分别为∆t=0.001 s、∆t=0.001 5 s、∆t=0.002 s，计算总时间分别为2、3和4 s。

2.4 关死点流量

离心泵在关死点处运行时，一般认为此时的流量为0。吴贤芳[30]在对离心泵关死点工况进行数值模拟时，认为离心泵在关死点工况下运行时，离心泵的内部流动在很小的流量下循环，此流量大致与口环泄漏量相近，并通过式（1）求解口环泄漏量。

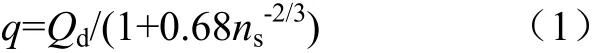
式中q为扣环泄流量，kg/s；Qd为设计流量，m3/h；ns为比转速。Dyson等[31]认为，口环泄漏量的大小约为泵设计流量的1%～5%。本文在计算时，取这个很小的流量为0.01Qd=0.035 kg/s，在对关阀启动过程进行求解时，认为关死点流量足够小时，这个很小的流量在整个启动过程中可以看作一个不变常数。

3 结果对比与分析

3.1 关死点工况稳态扬程

图4所示为模型泵不同转速下关死点处扬程的模拟值与试验值对比图。

由图4可知，数值模拟得到的扬程-转速曲线与试验结果的变化趋势相同，数值模拟结果均略高于试验结果，随着转速的增加，模型泵关死点扬程逐渐增大；额定转速处关死点扬程的模拟值为76.91 m，试验测得关死点扬程为74.02 m，模拟值与试验值的绝对偏差为3.9%，其余工况最大偏差均小于5%。因此，本文对超低比转速离心泵关死点工况的数值计算方法具有一定的准确性。

3.2 关阀启动过程瞬态扬程

为了研究启动加速度对超低比转速模型泵关阀启动过程瞬态特性的影响，分别在启动加速度为154.38、102.92和77.19 rad/s2，即加速时间分别为2、3和4 s共3种不同启动加速度时，对超低比转速离心泵关阀启动过程进行数值计算。图5为关阀启动过程扬程曲线的计算结果和试验结果。

图4 关死点扬程-转速曲线模拟值与试验值Fig.4 Head-speed curve of simulation and experiment in shut-off condition

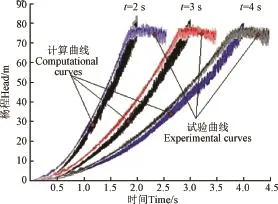
图5 瞬态模拟和试验结果Fig.5 Transient computational and experimental results

由图5可知，随着启动过程发展，即转速的线性增加，扬程逐渐增大，扬程在启动初期增加缓慢，随后随时间增加扬程增加速率逐渐增大，扬程脉动幅度逐步加剧，3种不同启动加速度下启动过程的末期，扬程均达到最大值。当加速时间为2、3和4 s时，数值计算得到扬程的峰值分别为83.31、81.62和80.13 m；3种加速时间下试验测得的扬程峰值分别为80.05、79.28和78.53 m，相对偏差分别为4.07%、2.95%和2.04%，均在5%以内，表明对超低比转速离心泵关阀启动过程的数值模拟方法具有一定的准确性。模型泵关死点处的扬程计算值为76.91 m，3种不同启动加速度下，启动完成时的瞬态扬程分别比稳态扬程高出8.32%、6.13%和4.19%，这表明关阀启动过程中扬程的变化具有明显的瞬态效应，启动过程结束时会产生一个明显的冲击扬程，且随着启动加速度的增大，这个冲击扬程也逐渐增大，表明启动加速度对超低比速泵关阀启动过程的瞬态特性有明显的影响。

3.3 内部流场结果对比与分析

通过上述的研究结果发现，随着启动加速度的减小，启动过程中的瞬态效应逐渐削弱。因此，为了深入分析超低比速泵关阀启动过程中的瞬态特性，本文选取启动加速度最大的一组启动方案，分析加速时间t=2 s时关阀启动瞬态和关死点稳态过程中内流场的演化过程。

3.3.1 静压分布

图6分别为关死点稳态过程与启动总时间为t=2 s下关阀启动瞬态过程不同时刻泵中间截面的静压分布云图。

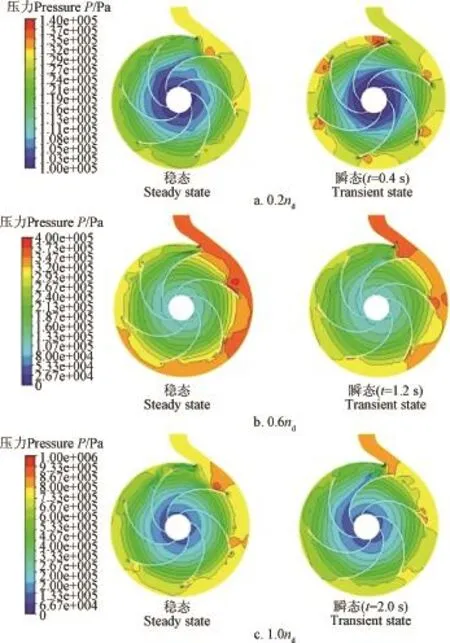
图6 稳态与瞬态中间截面静压分布Fig.6 Static pressure of steady state and transient state in middle section

由图6可知，不同转速工况下，静压最低处均位于叶轮进口区域，叶轮流道出口靠近蜗壳附近断面中间区域出现高压区。随着叶轮旋转对流体做功，叶轮流道内静压随着半径的增大逐渐增大，除靠近隔舌的叶轮流道外，其余流道内压力分布均匀，隔舌处压力梯度较大，表明在关死点工况，蜗壳隔舌结构对泵内静压分布有重要影响。随着转速的增加，泵内静压逐渐增加，泵进出口压差逐渐增大，不同转速下叶轮流道内静压分布趋势相似。不同时刻静压最低处均位于叶轮进口区域，随着叶轮旋转对流体做功，叶轮流道内静压随着半径的增大逐渐增大，叶轮流道内压力分布均匀，隔舌处压力梯度较大，不同时刻静压分布趋势相似。当t=0.4 s时，叶轮出口靠近叶片工作面处出现高压集中区，随着转速的增加，这个高压区逐渐消失，泵内静压逐渐增加，泵进出口压差逐渐增大，泵内静压分别逐渐分布均匀。

由图6可知，在相同转速时，稳态过程泵内静压分布与关阀启动过程泵内静压明显不同，在启动过程初期，静压分布差别最大，随着转速的增加，泵内静压分布的差别逐渐减小。

3.3.2 相对速度分布

图7为关死点稳态过程与启动总时间为t=2 s下关阀启动瞬态过程不同时刻泵中间截面的相对速度分布与流线。

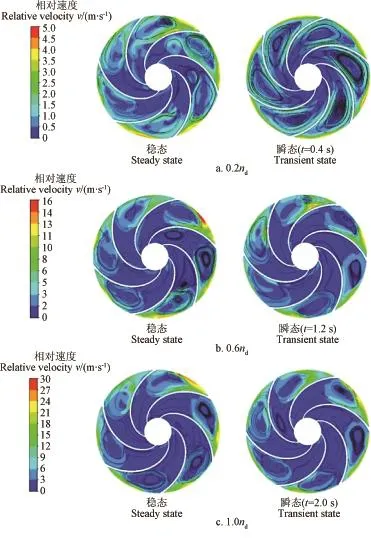
图7 稳态与瞬态中间截面相对速度分布Fig.7 Relative velocity of steady state and transient state in middle section

由图7a可知，不同转速下，叶轮流道内均存在大面积的低速区，叶轮出口处相对速度最大，叶轮流道内存在数量不等，大小不一的漩涡，叶轮内流动损失很大，随着转速的增加，叶轮内相对速度逐渐增大，低速区面积逐渐减小，漩涡区的范围和数量逐渐减小，叶轮内相对速度分布逐渐变的均匀。不同时刻叶轮出口处相对速度最大，叶轮流道内同样存在数量不等，大小不一的漩涡，当启动初期t=0.4 s时，漩涡区几乎充满整个叶轮流道，叶轮内流动损失很大，随着转速的增加，叶轮内相对速度逐渐增大，低速区面积逐渐减小，漩涡区的范围和数量逐渐减小。

由图7可知，在相同转速时，稳态过程时泵内相对速度大于关阀启动过程泵内相对速度，在启动过程初期，相对速度流线分布的差别最大，随着转速的增加，泵内部流场的差别逐渐减小。

3.4 进口管路内速度分布

图8为关死点稳态过程与启动总时间为t=2 s下关阀启动瞬态过程不同时刻进口管路轴截面的速度分布与流线图。

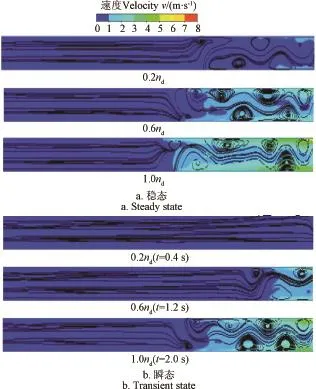
图8 稳态与瞬态过程进口管路内速度分布Fig.8 Velocity of steady state and transient state in inlet pipe

由图8a可知，不同转速下，进口管路内速度沿流动方向逐渐增大，进口管路靠近来流处速度流线分布均匀，在靠近吸水室进口处出现大量类似卡门涡街的对称分布漩涡区，随着转速的增加，进口管路内速度逐渐增加，在吸水室进口处速度最大，漩涡区范围逐渐扩大，漩涡数量逐渐增加，当漩涡区充满进口管路约一半时，漩涡区范围不再扩大。由图8b可知，在启动初期t=0.4 s时，进口管路内速度流线分布均匀，当t=1.2 s时，靠近吸水室进口出出现漩涡区，随着转速的增加，漩涡区范围向来流方向扩大，漩涡数量增加，漩涡区分布与稳态过程相似，呈对称分布。

对比图8a与8b可以看出，在相同转速时，稳态过程进口管路内速度大于关阀启动过程进口管内的速度，漩涡区的范围及漩涡数量均大于同一时刻瞬态过程。

基于对关死点稳态过程和启动过程内流场的分析发现：在相同转速时，关阀启动过程内部瞬态流场的发展总体上滞后于关死点处稳态过程内部流场。

4 结 论

本文对一台超低比速泵在关死点工况进行稳态和关阀启动瞬态过程进行了数值模拟，并与试验结果进行对比，分析了不同启动加速度对瞬态冲击扬程的影响，同时对比了关死点工况稳态过程与瞬态过程扬程和内流场的区别，得到以下结论：

1）关死点工况下扬程的数值模拟结果与试验结果偏差均在5%以内，表明本文采用的数值计算方法是可行的。

2）关阀启动过程中，3种启动加速度下启动完成时的瞬态扬程分别比稳态扬程高出8.32%、6.13%和4.19%，这表明关阀启动过程中扬程的变化具有明显的瞬态效应，启动过程结束时会产生一个明显的冲击扬程，且冲击扬程随启动加速度的增大而增大。

3）相同转速时，启动过程初期的静压分布差别最大；启动过程蜗壳流道内的漩涡区明显多于稳态过程；稳态过程进口管路内相对速度、漩涡区的范围及漩涡数量均大于瞬态过程。随着启动过程中转速增加，这些差异逐渐缩小。

4）相同转速时，关阀启动过程内部瞬态流场的发展总体上滞后于关死点处稳态过程内部流场。

[1] 刘元义，衣振芹. 超低比转速离心泵的研究[J]. 机械工程与自动化，2008(2)：198－200. Liu Yuanyi, Yi Zhenqin. Study on super-low specific speed centrifugal pump[J]. Mechanical Engineering & Automation, 2008(2): 198－200. (in Chinese with English abstract)

[2] 郭维，白东安. 超低比转速离心泵内流场计算及分析[J].火箭推进，2007，33(2)：26－30. Guo Wei, Bai Dongan. Inner flow field computation and analysis of super low-specific-speed centrifugal pump[J]. Journal of Rocket Propulsion, 2007, 33(2): 26－30. (in Chinese with English abstract)

[3] 齐学义，刘永明，胡家旺，等. 提高超低比转速离心泵效率的方法[J]. 水电能源科学，2011，29(2)：118－120. Qi Xueyi, Liu Yongming, Hu Jiawang, et al. Method of improving efficiency of low specific speed centrifugal pump[J]. Water Resources and Power, 2011, 29(2): 118－120. (in Chinese with English abstract)

[4] 闻建龙，沙毅，李传君，等. 无过载超低比转速离心泵水力设计[J]. 江苏大学学报：自然科学版，2003，24(6)：21－24. Wen Jianlong, Sha Yi, Li Chuanjun, et al. Hydraulic design of non-overload super-low specific speed centrifugal pump[J]. Journal of Jiangsu University :Natural Science Edition, 2003, 24(6): 21－24. (in Chinese with English abstract)

[5] 张德胜，施卫东，陈斌，等. 低比转速离心泵内部流场分析及试验[J]. 农业工程学报，2010，26(11)：108－113. Zhang Desheng, Shi Weidong, Chen Bin, et al. Turbulence analysis and experiments of low-specific-speed centrifugal pump[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2010, 26(11): 108－113. (in Chinese with English abstract)

[6] 齐学义，孔源，田亚斌，等. 基于CFD技术的超低比转速离心泵叶轮的优化设计[J]. 兰州理工大学学报，2010，36(4)：52－55. Qi Xueyi, Kong Yuan, Tian Yabin, et al. Optimization design of impeller for centrifugal pumps with super-low specific-speed by means of CFD technology[J]. Journal of Lanzhou University of Technology, 2010, 36(4): 52－55. (in Chinese with English abstract)

[7] 付燕霞，袁寿其，袁建平，等. 离心泵小流量工况下的内部流动特性[J]. 排灌机械工程学报，2014，32(3)：185－190. Fu Yanxia, Yuan Shouqi, Yuan Jianping, et al. Internal flow characteristics of centrifugal pump at low flow rates[J].Journal of Drainage and Irrigation Machinery Engineering (JDIME), 2014, 32(3): 185－190. (in Chinese with English abstract)

[8] Liu H L, Wang K, Kim H B, et al. Experimental investigation of the unsteady flow in a double-blade centrifugal pump impeller[J]. Science China Technological Sciences, 2013, 56(4): 812－817.

[9] GonzáLez J, FernáNdez J, Blanco E, et al. Numerical simulation of the dynamic effects uue to impeller-volute interaction in a centrifugal pump[J]. Journal of Fluids Engineering, 2002, 124(2): 348－355.

[10] Dazin A, Caignaert G, Bois G, et al. Transient behavior of turbomachinery: Applications to radial flow pump startups[J]. Journal of Fluids Engineering, 2007, 129(11): 1436－1444.

[11] Farhadi K, Bousbia-Salah A, D’Auria F. A model for the analysis of pump start-up transients in Tehran Research Reactor[J]. Progress in Nuclear Energy, 2007, 49(7): 499－510.

[12] Rochuon N, Trébinjac I, Billonnet G. An extraction of the dominant rotor-stator interaction modes by the use of proper orthogonal decomposition (POD)[J]. Journal of Thermal Science, 2006, 15(2): 109－114.

[13] 李贵东，王洋，郑意，等. 气液两相条件下离心泵内部流态及受力分析[J]. 排灌机械工程学报，2016，34(5)：369－374. Li Guidong, Wang Yang, Zheng Yi, et al. Unsteady internal flow and thrust analysis of centrifugal pump under gas-liquid two- phase flow conditions[J]. Journal of Drainage and Irrigation Machinery Engineering, 2016, 34(5): 369－374. (in Chinese with English abstract)

[14] 张玉良，肖俊建，崔宝玲，等. 离心泵快速变工况瞬态过程特性模拟[J]. 农业工程学报，2014，30(11)：68－75. Zhang Yuliang, Xiao Junjian, Cui Baoling, et al. Simulation of transient behavior in prototype centrifugal pump during rapid regulating flow rate[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2014, 30(11): 68－75. (in Chinese with English abstract)

[15] 王玉川，谭磊，曹树良，等. 离心泵叶轮区瞬态流动及压力脉动特性[J]. 机械工程学报，2014，50(10)：163－169. Wang Yuchuan, Tan Lei, Cao Shuliang, et al. Characteristics of transient flow and pressure fluctuation in impeller for centrifugal pump[J]. Journal of Mechanical Engineering, 2014, 50(10): 163－169. (in Chinese with English abstract)

[16] 韩伟，岳婷，李仁年，等. 动静叶栅内固液两相过渡过程的数值研究[J]. 排灌机械工程学报，2015，33(2)：111－115. Han Wei, Yue Ting, Li Rennian, et al. Numerical analysis of transition process of solid-liquid two-phase in rotor-stator cascades[J]. Journal of Drainage and Irrigation Machinery Engineering (JDIME), 2015, 33(2): 111－115. (in Chinese with English abstract)

[17] Thanapandi P, Prasad R. Centrifugal pump transient characteristics and analysis using the method of characteristics[J]. International Journal of Mechanical Sciences, 1995, 37(1): 77－89.

[18] Thanapandi P, Prasad R. A quasi-steady performance prediction model for dynamic characteristics of a volute pump[J]. Proceedings of the Institution of Mechanical Engineers Part a Journal of Power & Energy, 1994, 208(11): 47－58.

[19] Wu D Z, Jiao L, Wang L Q. Experimental study on cavitation performance of a centrifugal pump during starting period[J]. Journal of Engineering Thermophysics, 2008, 29(10): 1682－1684.

[20] Wu D, Wang L, Hao Z, et al. Experimental study on hydrodynamic performance of a cavitation centrifugal pump during transient operation[J]. Journal of Mechanical Science and Technology, 2010, 24(2): 575－582.

[21] Li Z, Wu P, Wu D, et al. Experimental and numerical study of transient flow in a centrifugal pump during startup[J]. Journal of Mechanical Science and Technology, 2011, 25(3): 749－757.

[22] Wu D, Peng W, Li Z, et al. The transient flow in a centrifugal pump during the discharge valve rapid opening process[J]. Nuclear Engineering & Design, 2010, 240(12): 4061－4068. [23] Li Zhifeng, Wu Dazhuan, Wang Leqin, et al. Numerical simulation of the transient flow in a centrifugal pump during starting period[J]. Journal of Fluids Engineering, 2010, 132(8): 081102.

[24] 武鹏，吴大转，李志峰，等. 离心泵流量突增过程瞬态流动研究[J]. 工程热物理学报，2010(3)：419－422. Wu Peng, Wu Dazhuan, Li Zhifeng, et al. Study of transient flow in centrifugal pump during flow impulsively increase process[J].Journal of Engineering Thermophysics, 2010(3): 419－422. (in Chinese with English abstract)

[25] 刘竹青，朱强，杨魏，等. 双吸离心泵关阀启动过程的瞬态特性研究[J]. 农业机械学报，2015，46(10)：44－48. Liu Zhuqing, Zhu Qiang, Yang Wei, et al. Transient characteristics of double-suction centrifugal pump during starting period under shut-off condition[J]. Transactions of the Chinese Society for Agricultural Machinery, 2015, 46(10): 44－48. (in Chinese with English abstract)

[26] 袁建平，夏水晶，宗伟伟，等. 基于流固耦合的离心泵启动过程瞬态叶片动应力特性[J]. 振动与冲击，2016，35(12)：197－202. Yuan Jianping, Xia Shuijing, Zong Weiwei, et al. Transient stress characteristic during centrifugal pumps start-up based on fluent-structure interaction[J]. Journal of Vibration and Shock, 2016, 35(12): 197－202. (in Chinese with English abstract)

[27] Menter F R. Two-equation eddy-viscosity turbulence models for engineering applications[J]. AIAA Journal, 1994, 32(8): 1598－1605.

[28] Menter F R. Zonal. Two-equation k-ω turbulence model for aerodynamic flows[J]. AIAA Paper, 1993: 1993－2006.

[29] Menter F R, Kuntz M, Langtry R. Ten years of industrial experience with the SST turbulence model[J]. Turbulence, Heat and Mass Transfer 4, 2003.

[30] 吴贤芳. 离心泵关死点性能的研究[D]. 镇江：江苏大学，2013. Wu Xianfang. Research on Characteristics of Centrifugal Pumps at Shut-off Condition[D]. Zhenjiang: Jiangsu University, 2013.

[31] Dyson G, Teixeira J. Investigation of closed valve operation using computational fluid dynamics[C]// ASME 2009 Fluids Engineering Division Summer Meeting, 2009: 1－9.

Transient characteristic analysis of ultra-low specific-speed centrifugal pumps during startup period under shut-off condition

Wang Yong1, Chen Jie1, Liu Houlin1, Shao Chang1, Zhang Xiang2※
(1. National Research Center of Pumps, Jiangsu University, Zhenjiang 212013, China; 2. Key Laboratory of Fluid and Power Machinery, Ministry of Education, Xihua University, Chengdu 610039, China)

In order to explore the characteristics of the ultra-low specific-speed centrifugal pump during startup period under shut-off condition, an ultra-low specific-speed centrifugal pump with the specific-speed of 25 was chosen as the research object. Unsteady numerical calculation under shut-off condition and transient condition was conducted for the ultra-low specific-speed centrifugal pump. The accuracy of numerical simulation was verified by experimental contrast. Based on the numerical results, the influence of different starting acceleration on the transient impact head, the difference between steady state and transient impact head, and the internal flow field were analyzed. The results showed that: The variation tendency of performance curve on shut-off condition under steady state condition was similar with the experiment, and along with the increase of rotational speed, the head under shut-off condition increased gradually. When the start was completed, the transient head was 8.32%, 6.13% and 4.19% higher than the steady state head, respectively, at 3 different starting accelerations, which indicated that the valve head change during the startup process had a significant transient effect. There was a significantly higher impact head at the end of startup processes with 3 different start accelerations, and with the increase of start acceleration, the impact head was also increased. With the rotation of the impeller acting fluid, the static pressure increased gradually with the increase of the radius. In addition to the impeller flow close to the tongue, the pressure distribution in the other channels was uniform, and the pressure gradient of the tongue was larger, which indicated that the volute tongue structure had an important influence on the distribution of the static pressure in the pump. With the increase of rotation speed, the static pressure increased gradually, and the pressure difference between the inlet and outlet of the pump increased gradually. When the time was 0.4 s, a high pressure concentration area occurred at impeller outlet near the blade pressure surface, and along with the increase of rotation speed, the high pressure concentration area gradually disappeared. The pump pressure distribution gradually grew uniform with pump pressure increasing. At the same speed, static pressure distribution, absolute speed streamline, and relative speed streamline shaft section of inlet pipe during stable process were different from the distribution during transient startup process. The differences were narrow with the increase of rotational speed. At the same speed, the development of inside transient flow field during transient startup process generally lagged after stable condition. At the same speed, static pressure of steady state and startup period under shut-off condition was obviously different. At the beginning of startup period, the static pressure distribution difference was the maximum, and with the increase of speed, the static pressure distribution difference gradually decreased. The relative velocity of steady state and startup period under shut-off condition was obviously different. At the beginning of the startup process, the difference of the streamline distribution of relative velocity was the biggest; with the increase of rotating speed, the difference of pump flow field decreased gradually. The relative velocity of inlet pipe in the process of steady state was larger than that in the process of startup, and the ranges of vortex region and vortex number were greater than the transient process at the same time. Based on the above results, it was found that with the decrease of starting acceleration, the transient effect was weakened. The research results provide the reference for the further study of the characteristics of transient process of the ultra-low specific-speed centrifugal pump.

centrifugal pump; computer simulation; experiment; shut-off condition; steady characteristic; transient characteristic

10.11975/j.issn.1002-6819.2017.11.009

TH311

A

1002-6819(2017)-11-0068-07

王 勇，陈 杰，刘厚林，邵 昌，张 翔. 超低比转速离心泵关阀启动瞬态特性分析[J]. 农业工程学报，2017，33(11)：68－74.

10.11975/j.issn.1002-6819.2017.11.009 http://www.tcsae.org

Wang Yong, Chen Jie, Liu Houlin, Shao Chang, Zhang Xiang. Transient characteristic analysis of ultra-low specific-speed centrifugal pumps during startup period under shut-off condition[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2017, 33(11): 68－74. (in Chinese with English abstract) doi：10.11975/j.issn.1002-6819.2017.11.009 http://www.tcsae.org

2016-12-20

2017-04-23

江苏省产学研联合创新资金—前瞻性联合研究项目（BY2015064-10）；江苏省“六大人才高峰”高层次人才项目（GBZB-017）；江苏高校优势学科建设工程资助项目；流体及动力机械教育部重点实验室（西华大学）开放课题（szjj2016-068）

王 勇，男，吉林白山人，博士，副研究员，主要研究方向为泵设计理论与方法。镇江 江苏大学国家水泵及系统工程技术研究中心，212013。Email：wylq@ujs.edu.cn

※通信作者：张 翔，博士，讲师，主要研究方向为水力机械数值计算。成都 西华大学流体及动力机械教育部重点实验室610039。

Email：zhangxiang@mail.xhu.edu.cn