关于对偶Steiner多项式的根的注记

张德燕，马统一

（1.淮北师范大学数学科学学院，安徽淮北235000；2.同济大学数学系，上海200092；3.河西学院数学与统计学院，甘肃张掖734000）

关于对偶Steiner多项式的根的注记

张德燕1，2，马统一3

（1.淮北师范大学数学科学学院，安徽淮北235000；2.同济大学数学系，上海200092；3.河西学院数学与统计学院，甘肃张掖734000）

受凸体的Steiner多项式的启发，定义了星体的对偶Steiner多项式，并利用对偶Aleksandrov-Fenchel不等式讨论了对偶Steiner多项式的根.进而，得到了关于对偶Steiner多项式的根的一些不等式，这些不等式恰好是关于Steiner多项式的根的不等式的对偶形式.

Steiner多项式；对偶Steiner多项式；对偶Aleksandrov-Fenchel不等式

1 引言

记Kn是所有凸体（n维欧氏空间Rn中的紧凸集）的集合，Bn是n维单位球体，Kno表示所有有非空内部的凸体的集合，则Kno⊆Kn.我们用V（·）表示n维体积.对任意的凸体K，E∈Kn，ρ是一个非负实数，则V（K+ρE）可以表示成ρ的n次多项式：

若E=Bn，（1.1）式是经典的Steiner公式［2］，Wi（K；Bn）通常简记为Wi（K），是经典的第i阶均值积分.

一般地，凸体K关于E的相对内半径r（K；E）和相对外半径R（K；E）定义为：

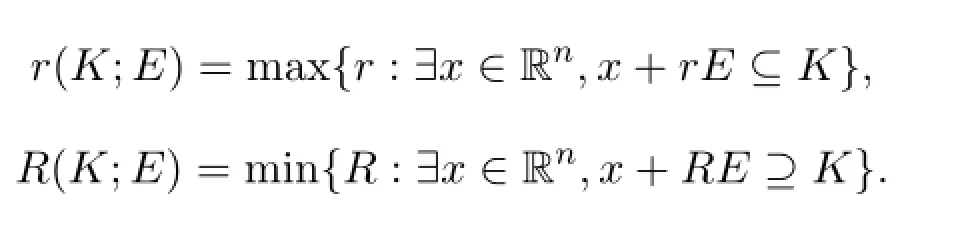
定义1.1［3］记C为复数域.设s∈C，称关于变量s的多项式

为（相对）Steiner多项式.

在Brunn-Minkowski理论中，Steiner多项式是一个重要的研究课题，尤其是对Steiner多项式根的讨论已受广泛关注［37］.当n=2时，Steiner多项式有两个负实根，其中一个不大于-R（K；E），另一个不小于-r（K；E）.在文献［8］中Teissier提出是否可将此结果推广到n＞2的情形？同时文献［7，9］中提出了下面的猜想：

猜想记Re（s）为一个复数s的实部.设K，E∈Kn，γi（i=1，···，n）是Steiner多项式f（K，E，s）的n个根，且满足Re（γ1）≤···≤Re（γn），则

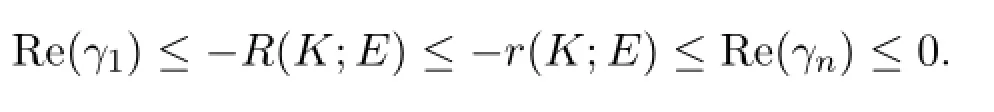
上面已经提到n=2时该猜想正确.对n=3的情形，文献［5］中已经证明对于一些特殊的凸体该猜想正确.对任意维数n，文献［3］中证明了：设K∈Kn，E∈Kno，γi（i=1，···，n）是Steiner多项式f（K，E，s）的n个根，则

（i）若K是m维的且m≥1，则非零根γi满足

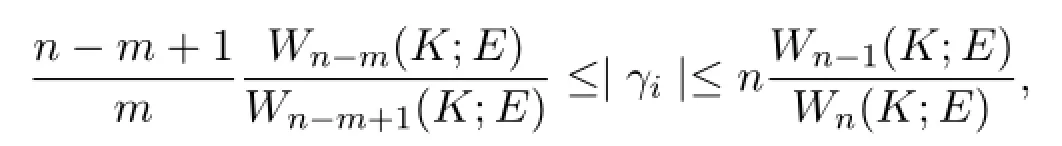
其中上界是最优的，且r（K；E）/n≤|γi|≤nR（K；E）.

（ii）|Re（γ1）|+···+|Re（γn）|≥nr（K；E）.

（iii）若Re（γi）≤0，i=1，···，n，则

相比较凸体理论，它的对偶理论即星体理论在凸几何中也扮演着重要的角色.在本文中我们对星体K，E定义对偶Steiner多项式g（K，E，s）：

在第三节中将讨论对偶Steiner多项式g（K，E，s）的根，并利用对偶的Aleksandrov-Fenchel不等式［10］得到了与对偶Steiner多项式的根有关的不等式，见本文的定理3.1，它恰好是上面提到的文献［3］中的结论（i）-（iii）的对偶形式.

2 准备工作及引理

记Sn-1是Rn中的单位球面.设Rn中的一个紧子集K关于原点是星形的，它的径向函数ρK=ρK（·）：Rn｛0｝→R定义为

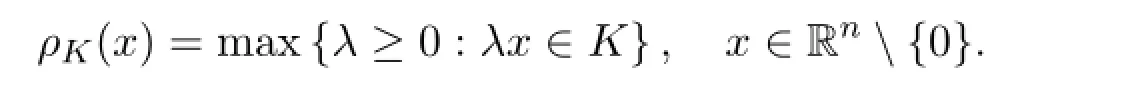
若ρK是正定的连续的，则K叫做一个关于原点的星体（简称星体）.用Sn记作Rn中全体星体的集合.

设K∈Sn，c是一个实数，则Minkowski数乘cK定义为

从径向函数的定义不难得到：若K是一个星体，c≥0，则

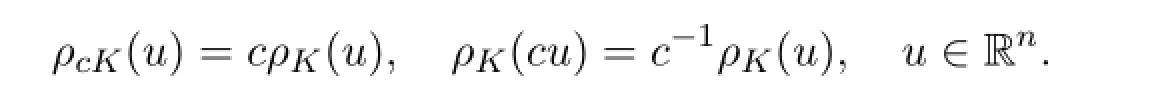
我们称两个星体K，L是互为膨胀的，若ρK（u）/ρL（u）是与u∈Sn-1无关的常数.

对于α，β≥0，星体K，L的径向线性组合［10］定义为

若Kj∈Sn（1≤j≤n），对偶混合体积［10］定义为

其中dS（u）是Sn-1上的n-1维体积元.设s，t是两个和不超过n的非负整数，K，L是两个星体，是n-s-t个星体的星体组（C1，···，Cn-s-t）.记是对偶混合体积

.对任意的i，0≤i≤n，若

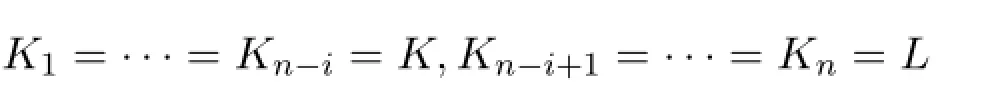
下面列举来自于文献［10］中对偶混合体积的一些基本性质，以备下文所用.

性质2.1［10］设K，Ki，Li∈Sn（1≤i≤n），则

仿照凸体的相对Steiner公式，我们引入星体的对偶相对steiner公式.

引理2.1 设K，E∈Sn，则对任意的实数λ≥0，有

我们称（2.4）式为K相对于E的对偶Steiner公式，简称对偶Steiner公式.

为星体K相对于星体E的对偶Steiner多项式，简称对偶Steiner多项式.

3 主要定理及其证明

这一节中我们讨论对偶Steiner多项式的根的性质，首先需要下面的一个引理.

［1］Schneider R.Convex Bodies.The Brunn-Minkowski Theory［M］.Encyclopedia of Mathematics and its Applications 44.Cambridge：Cambridge University Press，1993.

［2］Steiner J.Über parallel Flächen［J］.Ges.Werke，1882，2：245-308.

［3］Henk M，Hernández Cifre M A.Notes on the roots of Steiner polynomials［J］.Rev.Mat.Iberoamericana，2008，24（2）：631-644.

［4］Henk M，Hernández Cifre M A.On the location of roots of the Steiner polynomials［J］.Bull.Braz.Math. Soc.，2011，42（1）：153-170.

［5］Hernández Cifre M A，Saorín E.On the roots of the Steiner polynomial of a 3-dimensional convex body［J］. Adv.Geom.，2007，7：275-294.

［6］Jetter M.Bounds on the roots of the Steiner polynomial［J］.Adv.Geom.，2011，11：313-317.

［7］Sangwine-Yager J R.Bonnesen-Style inequalities for Minkowski relative geometry［J］.Transactions of the American Mathematical Society，1988，307（1）：373-382.

［8］Oda T.Convex Bodies and Algebraic Geometry.An Introduction to the Theory of Toric Varieties［M］. Berlin：Springer-Verlag，1988.

［9］Sangwine-Yager J R.Mixed Volumes，in：Handbook of Convex Geometry［M］.Amsterdam：North-Holland，1993.

［10］Lutwak E.Dual mixed volumes［J］.Pacific J.Math.，1975，58：531-538.

［11］Marden M.Geometry of Polynomials［M］.2nd ed.USA：American Mathematical Society，1966.

Notes on roots of the dual Steiner polynomial

Zhang Deyan1，2，Ma Tongyi3
（1.School of Mathematical Sciences，Huaibei Normal University，Huaibei235000；2.Department of Mathematics，Tongji University，Shanghai200092；3.College of Mathematics and statistics，Hexi University，Zhangye734000）

Motivated by the Steiner polynomial for convex bodies，the dual Steiner polynomial for star bodies is defined.Furthermore，roots of the dual Steiner polynomial are discussed by applying the dual Aleksandrov-Fenchel inequality，and some inequalities involving roots of the dual Steiner polynomial are obtained，where these inequalities are just dual forms of those of the Steiner polynomial.

The Steiner polynomial，the dual Steiner polynomial，the dual Aleksandrov-Fenchel inequality

O186.5

A

1008-5513（2016）02-0111-08

10.3969/j.issn.1008-5513.2016.02.001

2015-04-07.

国家自然科学基金（11161019，11561020）；安徽省高校自然科学研究重点项目（KJ2016A635）；甘肃省科技计划项目（145RJZG227）.

张德燕（1980-），博士，讲师，研究方向：微分几何与凸几何分析.

2010 MSC:52A20，52A39