Estimation of boundary-layer flow of a nanofluid past a stretching sheet: A revised model*

Naeema ISHFAQ， Zafar Hayat KHAN， Waqar Ahmad KHAN， Richard J. CULHAM

1. School of Mathematical Sciences， Peking University， Beijing 100871， China，

E-mail:sanam_143_6@hotmail.com

2. Department of Mathematics， University of Malakand， Dir （Lower）， Khyber Pakhtunkhwa， Pakistan

3. Department of Mechanical and Industrial Engineering， College of Engineering， Majmaah University， Al Majma′ah， Saudi Arabia

4. Department of Mechanical Engineering， University of Waterloo， Waterloo， Ontario， Canada



Estimation of boundary-layer flow of a nanofluid past a stretching sheet: A revised model*

Naeema ISHFAQ1， Zafar Hayat KHAN2， Waqar Ahmad KHAN3， Richard J. CULHAM4

1. School of Mathematical Sciences， Peking University， Beijing 100871， China，

E-mail:sanam_143_6@hotmail.com

2. Department of Mathematics， University of Malakand， Dir （Lower）， Khyber Pakhtunkhwa， Pakistan

3. Department of Mechanical and Industrial Engineering， College of Engineering， Majmaah University， Al Majma′ah， Saudi Arabia

4. Department of Mechanical Engineering， University of Waterloo， Waterloo， Ontario， Canada

The previous model for the boundary layer nanofluid flow past a stretching surface with a specified nanoparticle volume fraction on the surface is revisited. The major limitation of the previous model is the active control of the nanoparticle volume fraction on the surface. In a revised model proposed in this paper， the nanoparticle volume fraction on the surface is passively controlled， which accounts for the effects of both the Brownian motion and the thermophoresis under the boundary condition， whereas the Buongiorno's model considers both effects in the governing equations. The assumption of zero nanoparticle flux on the surface makes the model physically more realistic. In the revised model， the dimensionless heat transfer rates are found to be higher whereas the dimensionless mass transfer rates are identically zero due to the passive boundary condition. It is also found that the Brownian motion parameter has a negligible effect on the Nusselt number.

boundary layer flow， nanofluid， stretching sheet， Brownian motion， thermophoresis

Introduction

Buongiorno［1］developed a model for nanofluid that includes both the Brownian motion and thermophoresis effects. This model was employed by Kuznetsov and Nield［2］and Nield and Kuznetsov［3］to examine the influence of nanoparticles on the free convection past a vertical plate. They employed boundary conditions with respect to the nanoparticle fraction akin to the temperature. Later on， Khan and Pop［4］employed the same model to investigate the laminar flow generated by the stretching of a flat surface. They studied the effects of Brownian and thermophoresis parameters on the dimensionless heat and mass transfer rates using the same approach as used in Refs.［2，3］. This means that the nanoparticle fraction on the wall can be specified arbitrarily， which is not realistic physically. Most recently， Kuznetsov and Nield［5，6］developed a physically realistic type of boundary condition which accounts for the effect of both Brownian and thermophoresis parameters. According to this new type of boundary condition， there is zero nanoparticle flux on the surface and the particle fraction values are adjusted accordingly. The model developed in Kuznetsov and Nield［5，6］was employed by Khan et al.［7，8］.

Pal and Mandal［9］studied the magnetohydrodynamic boundary layer flow of an electrically conducting convective nanofluids induced by a non-linear vertical stretching/shrinking sheet with viscous dissipation， thermal radiation， and Ohmic heating. Their results reveal that by increasing the value of the Hartman number the velocity will decrease， whereas a reverse effect is found in the temperature profiles. Das［10］investigated numerically the boundary layerflow of a nanofluid over non-linear permeable stretching sheet with prescribed surface temperature in the presence of partial slip. Sandeep et al.［11］analyzed the flow， the heat and the mass transfer behavior of Jeffrey， Maxwell and Oldroyd-B nanofluids over a permeable stretching sheet in the presence of transverse magneticfield， thermophoresis， Brownian motion and suction/injection. They found that the friction factor of the Maxwell nanofluid is less important as compared with the Oldroyd-B and Jeffrey nanofluid. Chandrasekar and Kasiviswanathan［12］applied a variational technique to the MHD， the radiative nanofluid flow over a non-isothermal stretching sheet with Brownian motion and thermophoresis effects by Gyarmati's principle. The heat and mass transfer effects were investigated and analyzed by this technique. Ganga et al.［13］analyzed the effects of the space and temperature dependent internal heat generation/absorption on the magnetohydrodynamic boundary layer flow of the water based nanofluid over a stretching sheet with different nanoparticles. The influences of the nanoparticle volume fraction， the magnetic field，the Prandtl number， the non-uniform heat source/sink，the local skin friction coefficient and the reduced Nusselt number were investigated for different nanoparticles. Khan et al.［14］investigated the problem of the oblique hydromagnetic stagnation point flow of the electrically conducting optically dense viscous incompressible nanofluid of a variable viscosity over a convectively heated stretching sheet in the presence of thermal radiation. They analyzed the effects of various controlling parameters on the dimensionless velocity，temperature， nanoparticles concentration， skin friction，Nusselt and Sherwood numbers. Makinde et al.［15］investigated the combined effects of the thermal radiation， the thermophoresis， the Brownian motion， the magnetic field and the variable viscosity on the boundary layer flow， the heat and the mass transfer of an electrically conducting nanofluid over a radially stretching convectively heated surface. Their results reveal that the heat transfer rate is reduced with the increase of the viscosity and the nanofluid parameters whereas the mass transfer rates are enhanced with the increase of the Brownian motion parameter and the Lewis number. Ahmad［16］studied a classical non-Newtonian fluid in the presence of nano-particles over a nonlinear stretching sheet. The correlation expressions for the skin friction， the Nusselt number and the Sherwood number were developed by performing a linear regression on the obtained numerical data. Mabood and Khan［17］obtained an analytical solution of an unsteady two-dimensional MHD nanofluid flow with heat and mass transfer over a heated surface. They conducted a detailed study illustrating the influences of the magnetic， unsteady， suction/injection and nanofluid parameters， on the dimensionless velocity， temperature， concentration as well as on the skin friction coefficient， and the reduced Nusselt and Sherwood numbers.

In this study， the model of the nanofluid flow past a stretching sheet in Ref.［4］ is revised with the assumption that the value of nanoparticles at the wall are no longer given.

1. Formatting mathematical model

The present analysis closely follows the work of Ref.［4］ and so only a brief outline is given here. A two-dimensional steady boundary layer flow of a nanofluid past a stretching surface is under consideration. The sheet is linearly stretched with linear velocity uw（x）=ax ， whereais a constant and a steady uniform stress is accompanied by a pair of forces of equal magnitude and opposite directions along thex-axis so that the sheet is stretched with the origin kept fixed. The flow takes place aty≥0， whereyis the coordinate measured normal to the stretching surface. It is assumed that on the stretching surface， the temperatureTkeeps a constant value Twand the nanoparticle fractionC takes the value Cwfor the actively controlled nanoparticles on the surface whereasCis passively controlled by the effects of thermophoresis for the passively controlled nanoparticles. The ambient values， attained asy tends to infinity， ofT and C are denoted by T∞and C∞， respectively.

The basic steady conservation equations of mass，momentum， thermal energy and nanoparticles for the nanofluids can be written in Cartesian coordinates x andy as （see Kuznetsov and Nield［5，6］）

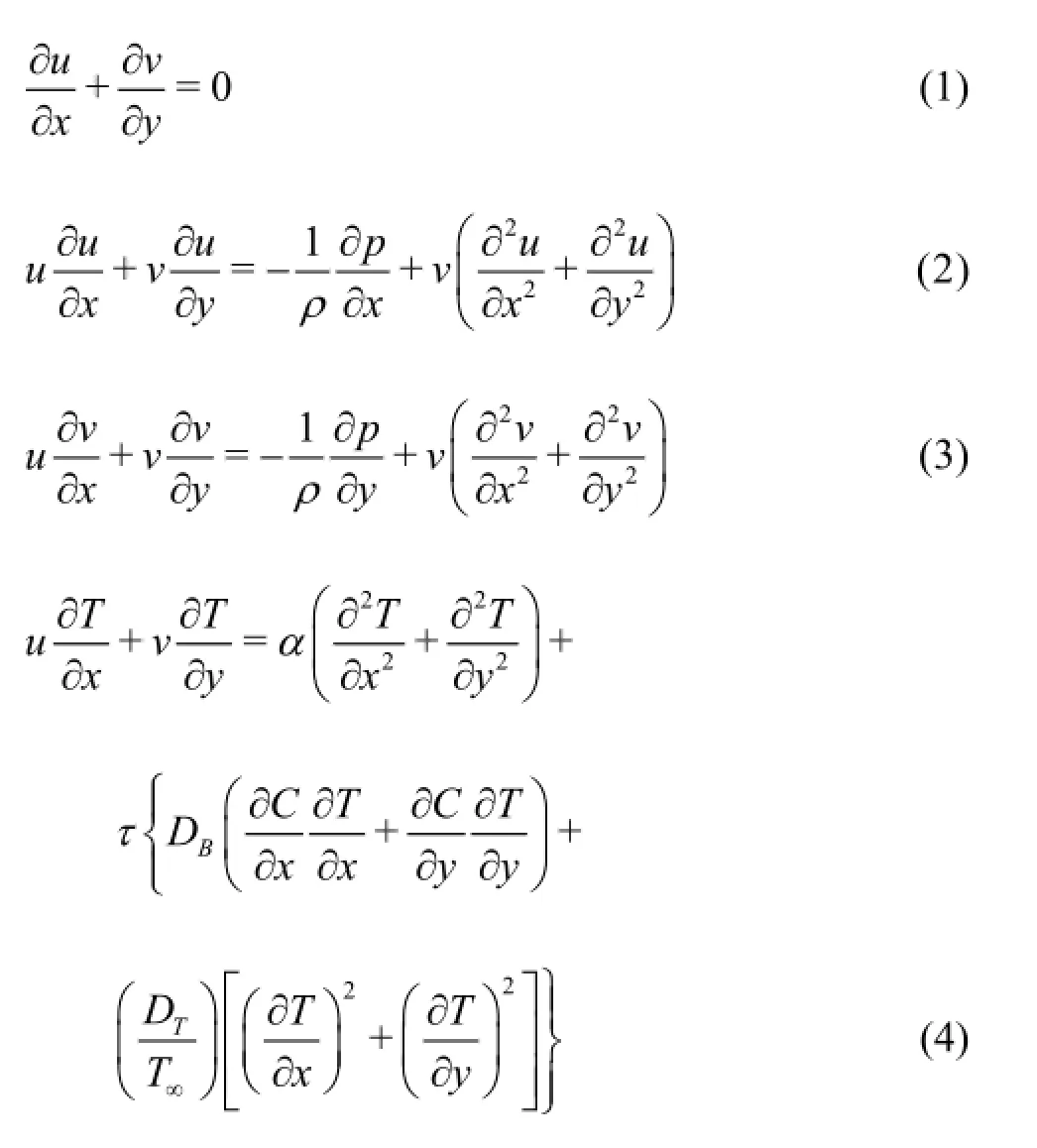
Table 1 Comparison of results for the Nusselt number when Nb　=　Nt=0

with boundary conditions

Hereuandv are the velocity components along the axes xandy ， respectively，p is the fluid pressure，ρis the density of the nanofluid，νis the kinematic viscosity of the nanofluid，αis the thermal diffusivity of the nanofluid，a is a positive constant，DBis the Brownian diffusion coefficient， and DTis the thermophoretic diffusion coefficient.

A similarity solution of Eqs.（1）-（5） with the boundary conditions （6） takes the following form:

where the stream functionψis defined in the usual way as u=∂ψ/∂y and v=-∂ψ/∂x. To determine the similarity solution （7）， we have taken into account that the pressure in the outer （inviscid） flow isp=p0（constant）. Substituting （7） into Eqs.（2）-（5）， we obtain the following ordinary differential equations:

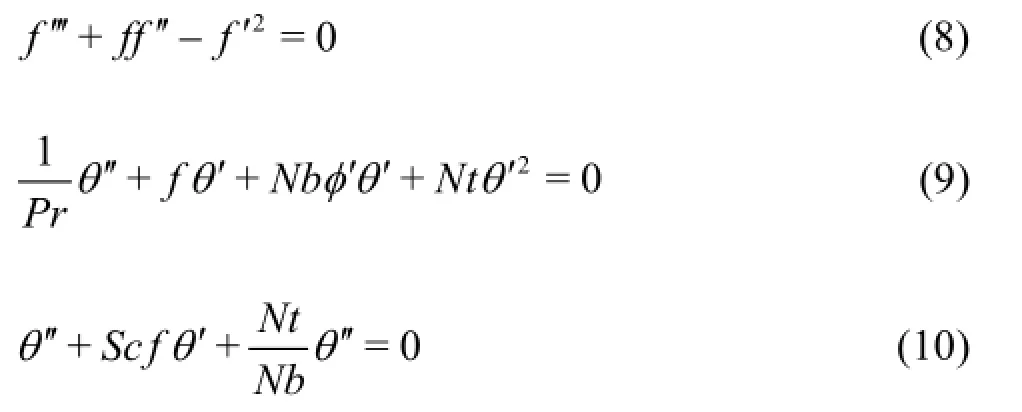
with the boundary conditions

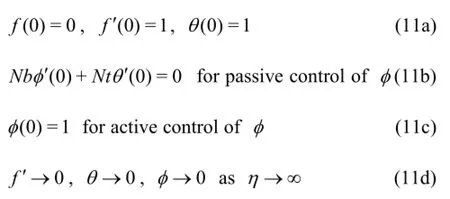
where primes denote differentiations with respect to ηand the four parameters are defined as:

HerePr，Sc，NbandNt denote the Prandtl number， the Schmidt number， the Brownian motion andthe thermophoresis parameters， respectively. It is important to note that this boundary value problem reduces to the classical problem of the flow and heat and mass transfer due to a stretching surface in a viscous fluid whenNbandNt approaches to zero in Eqs.（9）and （10）.

Of practical interest， in this study， is the Nusselt number Nux， which is defined as

where qwis the heat flux andkis the effective thermal conductivity. Using （7）， we obtain

It is important to note that with the new boundary condition the Sherwood number， which represents the dimensionless mass flux， is identically zero， i.e.

2. Numerical scheme

The nonlinear ordinary differential Eqs.（8）-（10）subject to the boundary conditions （11） are solved numerically using an efficient Runge-Kutta fourth order method along with the shooting technique. The asymptotic boundary conditions given by Eq.（11） are replaced by using a value of 15 for the similarity variable ηmax. The choice of ηmax=15and the step size∆η=0.001， ensure that all numerical solutions approach the asymptotic values correctly. For validating the proposed scheme， a comparison of the Nusselt number with the data in Refs.［4，8，9］ is shown in Table 1 for both active and passive controls ofφin the special case when Nb=Nt=0. Therefore， we are confident that the applied numerical scheme is very accurate.

3. Results and discussions

Since there is no change in the momentum and energy equations and the related boundary conditions，the dimensionless velocity and temperature profiles will remain the same as obtained with the previous model［4］. The only change is in the boundary condition of the surface nanoparticle volume fraction. The effects of the nanofluid parameters and the Schmidt number on the rescaled nanoparticle volume fraction for water-based nanofluids are shown in Figs.1（a）-1（c） for active control （as the previous model） and passive control （as the revised model） of nanoparticles at the surface.

Fig.1 Variation of volume fraction with nanofluid parameters and Schmidt number for both active and passive controls of nanoparticles at the surface

It is noticed that in the active control model， the rescaled nanoparticle volume fraction φ （η）assumes the maximum on the surface and decreases to zero in the related boundary layer. No appreciable effect of nanofluid parameters could be observed on the rescaled nanoparticle volume fraction profiles.

It is quite interesting to observe in the passive control model that the similarity variableφ （η）overshoots and attains negative values in the neighbourhood of the surface， see Figs.1（a）-1（c）. This interestingbehaviour is also observed by Kuznetsov and Nield［5，6］. In fact， it is due to the zero nanoparticle flux condition（accounting for both Brownian motion and thermophoresis parameters） on the surface， which means that the nanoparticle flux at the surface is suppressed.

Fig.2 Variation of Nusselt numbers for both active and passive controls of nanoparticles on the surface

The variations of the dimensionless heat transfer rates against the dimensionless numbersSc，Pr ， and the nanofluid parametersNtandNb are shown in Figs.2（a） and 2（b）. In Fig.2（a） and Table 3， the values of the Prandtl numbers for different base fluids are given in Table 2.

4. Estimation of the nusselt number

A linear regression estimate Nuestof the Nusselt number for the case Pr =6.2and Sc=10， is presented， which incorporates the effects of the Brownian motionNband the thermophoresis parameterNt. The linearly estimated equation using the least square method is obtained as

The calculations are performed for 256 sets of values ofNb， andNt in the range ［0.1，0.2，0.3，0.4，0.5］. This correlation is valid in the range ［0，0.5］with the maximum error of 1.66%. For more accurate estimation， a quadratic regression is performed for the following relation

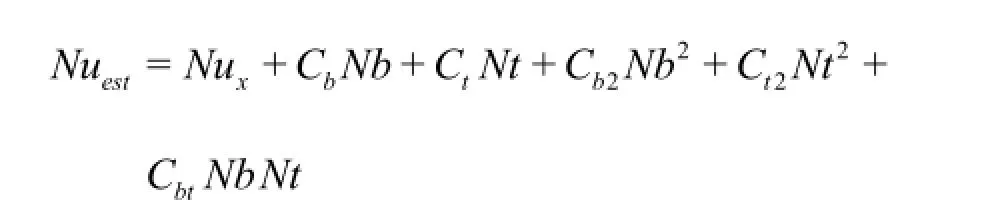
Both the linear and quadratic estimations indicate that the Nusselt number is a decreasing function of the Brownian motion Nband the thermophoresis parameterNt. This decreasing behaviour corresponds to an increase in the thermal boundary layer thickness. The interesting aspect of these correlations is that the coefficient ofNb is negligibly small and has very little effect on the Nusselt number. The same conclusion was drawn by Kuznetsov and Nield［3，4］.

5. Conclusion

We have revisited the boundary layer flow of nanofluid past a stretching sheet. The major improvement in the reformulation is the introduction of a more realistic boundary condition on the surface. We have assumed the zero nanoparticle flux on the surface，which accounts for the effects of both Brownian motion and thermophoresis in the boundary condition. The self-similar numerical solutions are analysed under the influence of the different governing parameters. The dependence of the Nusselt number on the Brownian motion and thermophoresis parameters is estimated using both linear and quadratic correlations. It is found that under the assumption of passively controlled nanoparticle volume fraction on the surface， the Brownian motion parameterNb has negligible effect on the heat transfer rate. This is in accordance with the conclusion made by Kuznetsov and Nield［3，4］.

Table 2 Prandtl numbers for different base fluids under consideration［19，20］

Table 3 Variation of Nusselt number against Nt for different base fluids with Sc =10and Nb=0.1

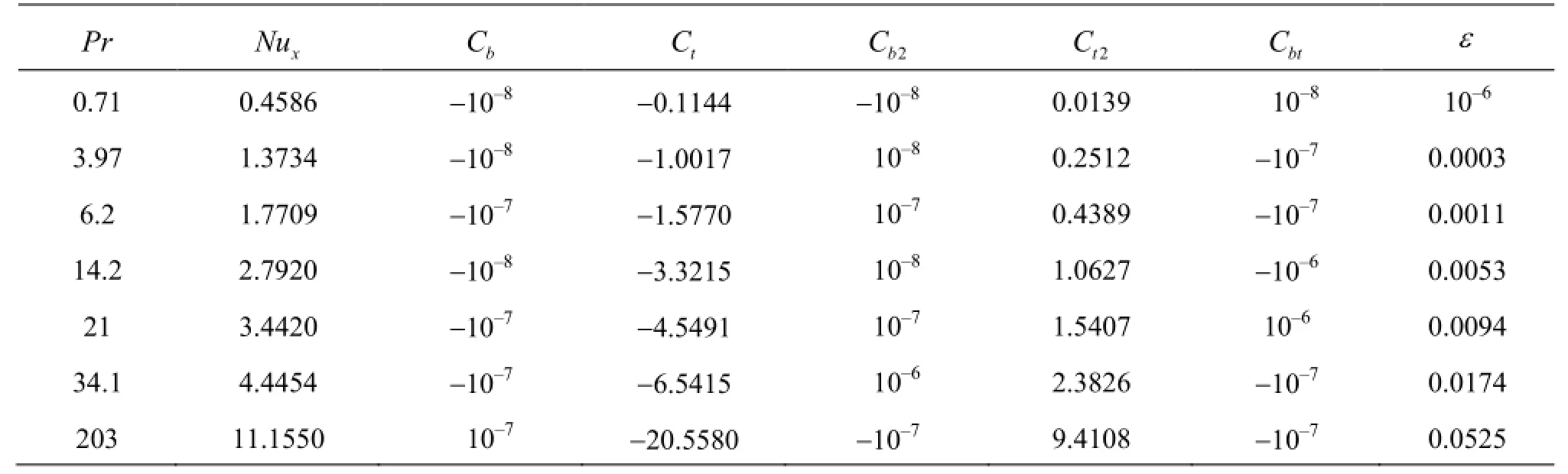
Table 4 Quadratic regression coefficients and error bound for the estimated Nusselt number with variations in Prandtl numberPrfor Sc=10

Table 5 Quadratic regression coefficients and error bound for the estimated Nusselt number with variations in Schmidt numberScfor Pr=6.2

References

［1］ BUONGIORNO J. Convective transport in nanofluids［J］. Journal of Heat Transfer， 2006， 128（3）: 240-250.

［2］ KUZNETSOV A. V.， NIELD D. A. Natural convective boundary-layer flow of a nanofluid past a vertical plate［J］. International Journal of Thermal Sciences， 2010， 49（2）: 243-247.

［3］ NIELD D. A.， KUZNETSOV A. V. The Cheng-Minkowycz problem for natural convective boundary layer flow in a porous medium saturated by a nanofluid［J］. International Journal of Heat and Mass Transfer， 2009，52（25-26）: 5792-5795.

MH7A细胞为永生化的RA关节滑膜细胞，购自广州吉妮欧公司。用高糖DMEM+10%胎牛血清于5%CO2，37℃的培养箱培养。购买时为第3代，培养3代后用于后续实验研究。

［4］ KHAN W. A.， POP I. Boundary-layer flow of a nanofluid past a stretching sheet［J］. International Journal of Heat and Mass Transfer， 2010， 53（11）: 2477-2483.

［5］ KUZNETSOV A. V.， NIELD D. A. The Cheng-Minkowycz problem for natural convective boundary layer flow in a porous medium saturated by a nanofluid: A revised model［J］. International Journal of Heat and Mass Transfer， 2013， 65（25-26）: 682-685.

［6］ KUZNETSOV A. V.， NIELD D. A. Natural convective boundary-layer flow of a nanofluid past a vertical plate: A revised model［J］. International Journal of Thermal Sciences， 2014， 77: 126-129.

［7］ KHAN Z. H.， KHAN W. A. and POP I. Triple diffusive free convection along a horizontal plate in porous media saturated by a nanofluid with convective boundary condition［J］. International Journal of Heat and Mass Transfer， 2013， 66（1）: 603-612.

［8］ WANG C. Y. Free convection on a vertical stretching surface［J］. Zamm-Journal of Applied Mathematics and Mechanics/Zeitschrift für Angewandte Mathematik und Mechanik， 1989， 69（11）: 418-420.

［9］ PAL D.， MANDAL G. Hydromagnetic convective-radiative boundary layer flow of nanofluids induced by a nonlinear vertical stretching/shrinking sheet with viscous-Ohmic dissipation［J］. Powder Technology， 2015， 279: 61-74.

［10］ DAS K. Nanofluid flow over a non-linear permeable stretching sheet with partial slip［J］ Journal of the Egyptian Mathematical Society， 2015， 23（2）: 451-456.

［11］ SANDEEP N.， RUSHI KUMAR B. and JAGADEESH KUMAR M. S. A comparative study of convective heat and mass transfer in non-Newtonian nanofluid flow past a permeable stretching sheet［J］. Journal of Molecular Liquids， 2015， 212（1）: 585-591.

［12］ CHANDRASEKAR M.， KASIVISWANATHAN M. S. Analysis of heat and mass transfer on MHD Flow of a nanofluid past a stretching sheet［J］. Procedia Engineering， 2015， 127: 493-500.

［13］ GANGA B.， SARANYA S. and VISHNU GANESH N. et al. Effects of space and temperature dependent internal heat generation/absorption on MHD flow of a nanofluid over a stretching sheet［J］. Journal of Hydrodynamics，2015， 27（6）: 945-954.

［14］ KHAN W. A.， MAKINDE O. D. and KHAN Z. H. Nonaligned MHD stagnation point flow of variable viscosity nanofluids past a stretching sheet with radiative heat［J］. International Journal of Heat and Mass Transfer， 2016，96: 525-534.

［15］ MAKINDE O. D.， MABOOD F. and KHAN W. A. et al. MHD flow of a variable viscosity nanofluid over a radially stretching convective surface with radiative heat［J］. Journal of Molecular Liquids， 2016， 219: 624-630.

［16］ AHMAD A. Flow of Reiner Philipp off based nanofluid past a stretching sheet［J］. Journal of Molecular Liquids，2016， 219: 643-646.

［17］ MABOOD F.， KHAN W. A. Analytical study for unsteady nanofluid MHD Flow impinging on heated stretching sheet［J］. Journal of Molecular Liquids， 2016， 219: 216-223.

［18］ KANDASAMY R.， LOGANATHAN P. and PUVI ARASU P. Scaling group transformation for MHD boundary-layer flow of a nanofluid past a vertical stretching surface in the presence of suction/injection［J］. Nuclear Engineering and Design， 2011， 241（6）: 2053-2059.

［19］ BEJAN A. Convection heat transfer［M］. 3rd Edition. New York， USA: John Wiley， 2004.

［20］ DAUBERT T. E.， DANNER R. P. Physical and thermodynamic properties of pure chemicals［M］. New York，USA: Hemisphere Publishing Corporation， 1989.

10.1016/S1001-6058（16）60663-7

October 6， 2014， Revised April 27， 2015）

* Project supported by the National Natural Science Foundation of China （Grant No. 11271023）.

Biography: Naeema ISHFAQ （1986-）， Female，

Ph. D. Candidate

Corresponging author: Zafar Hayat KHAN，

E-mail: zafarhayyatkhan@gmail.com

2016，28（4）:596-602