Tomographic PIV investigation on coherent vortex structures over shark-skin-inspired drag-reducing riblets

Shao-Qiong Yang·Shan Li·Hai-Ping Tian·Qing-Yi Wang,3·Nan Jiang



Tomographic PIV investigation on coherent vortex structures over shark-skin-inspired drag-reducing riblets

Shao-Qiong Yang1,2·Shan Li1·Hai-Ping Tian1·Qing-Yi Wang1,3·Nan Jiang1,2

©The Chinese Society of Theoretical and Applied Mechanics;Institute of Mechanics,Chinese Academy of Sciences and Springer-Verlag Berlin Heidelberg 2015

AbstractNature has shown us that the microstructure of the skin of fast-swimming sharks in the ocean can reduce the skin friction drag due to the well-known shark-skin effect.In the present study,the effect of shark-skin-inspired riblets on coherent vortex structures in a turbulent boundary layer（TBL）is investigated.This is done by means of tomographic particle image velocimetry（TPIV）measurementsinchannelflwsoveranacrylicplateofdrag-reducing riblets at a friction Reynolds number of 190.The turbulent flws over drag-reducing riblets are verifie by a planar time-resolved particle image velocimetry（TRPIV）system initially,and then the TPIV measurements are performed. Two-dimensional（2D）experimental results with a dragreduction rate of around 4.81%are clearly visible over triangleribletswithapeak-to-peakspacings+of14,indicating from the drag-reducing performance that the buffer layer within the TBL has thickened;the logarithmic law region has shifted upward and the Reynolds shear stress decreased. A comparison of the spatial topological distributions of the spanwise vorticity of coherent vortex structures extracted at differentwall-normalheightsthroughtheimprovedquadrant splitting method shows that riblets weaken the amplitudes of the spanwise vorticity when ejection（Q2）and sweep （Q4）events occur at the near wall,having the greatest effect on Q4 events in particular.The so-called quadrupole statistical model for coherent structures in the whole TBL is verified Meanwhile,theirspatialconditional-averagedtopological shapes and the spatial scales of quadrupole coherent vortex structures as a whole in the overlying turbulent flw over riblets are changed,suggesting that the riblets dampen the momentum and energy exchange between the regions of near-wall and outer portion of the TBL by depressing the bursting events（Q2 and Q4）,thereby reducing the skin friction drag.

KeywordsTurbulent boundary layer（TBL）·Coherent vortexstructure·Flowcontrol·Dragreduction·Shark-skininspired riblet·Tomographic particle image velocimetry（TPIV）

1　Introduction

Since 1967,when Kline et al.［1］at Stanford University observed the low-/high-speed streaks and burst events in the turbulent boundary layer（TBL）,scientists have changed their conventional knowledge on turbulence as not completely random motions of fluiparticles.Then the term coherentstructurewasgraduallyacceptedbyresearchersand is now roughly define as a pseudo-ordered structure with the basic characteristics of repeated intermittency,extended self-similarity,and a multiscale nature in time and space dimensions［2-5］.Overthedecades,muchresearchoncoherent vortex structures have been reported for experiments ［6-12］and numerical simulations［13-18］.A model of hairpin vortices or hairpin vortex pockets for coherent structuresin TBLs or other turbulent flws was initially proposed by Adrianetal.［6］,whichhasbeenconsideredasthe“structural skeleton”of TBLs,i.e.,the main flw structures for turbulence（Fig.1b）.Recently,a new statistical model,called a quadrupole（Fig.1a）,for coherent structures in TBLs was presented by Jiang et al.［19-21］.It was later extracted and quantifie by Chen et al.［14］in the direct numerical simulation（DNS）data of a turbulent channel flw using their velocity-vorticity correlation structure（VVCS,see Fig.1c）. However,whetherthequadrupolestructuresare“real”structures in turbulent flws remains an open question.

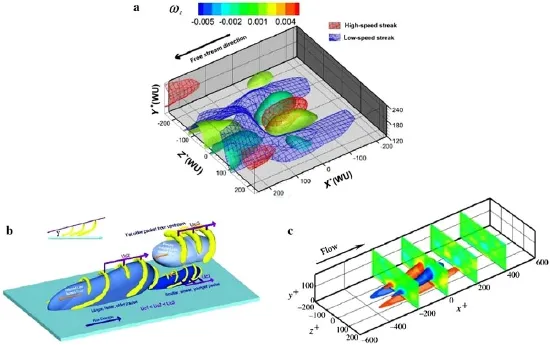
Fig.1　Models for coherent vortex structures in turbulent flws［6,14,20,21］

Fig.2　Potential applications of shark-skin-inspired drag-reducing riblets in transportation,energy,and competitive sports（the silky shark picture is adapted from Bechert and Bartenwerfer［29］）

It is a well-known lesson from nature that the microstructure of the skin of fast-swimming sharks in the ocean can reduce the skin friction drag in certain turbulent-flw conditions as a result of the well-known“shark-skin effect”. Since the 1980s,many researchers have started to manufacture and test these kinds of shark-skin-inspired surfaces or their simplifie model,known as riblets,which are small surface protrusions aligned with the flw direction.（More informationcanbefoundinrecentreviewpapers［22-24］and references therein.）Meanwhile,the drag reduction mechanismofribletshasbeenexploredindetailaswell（althoughit is not yet thoroughly understood）for their potential applicationintransportations,energy,andcompetitivesports（Fig.2）over the past several decades.Two main theories for the mechanism of drag reduction by riblets have been proposed and discussed:the second vortex theory and the protrusion heighttheory.Thesecondvortextheoryfocusesonthebehaviorsofcrossflw.Thus,investigatorswhosupportthistheory ［25-28］believethatribletshaveadrag-reducingeffectwhen the paired second streamwise vortices are generated within or near the riblet valleys as the second vortex pairs dampen the spanwise movement of the primary streamwise vortices during sweep events（Q4）;the drag-reduction effect deteriorates if the generated second vortices separate and shed. The protrusion height theory,on the other hand,in which a so-called protrusion height is the distance between the effectivevelocityprofil originandtheriblettip［29］,empha-sizes the determination of apparent flw origins based on the velocity profile and the relationship between the scales of coherent turbulent structures near riblets and riblet spacing ［s+,dimensionless peak-to-peak spacing in wall units（WU）scaled with inner variables］.Proponents of this theory［29-33］suggest that smaller coherent vortex structures in valleys undertheinvisibleoriginpointaremainlysuppressedbyviscosity,i.e.,a greater thickness of the viscous sublayer of the TBL,and a smaller velocity gradient,which decreases the overall wall drag friction.In addition,when the multiscale coherent structures in a scale are greater than s+,i.e.,above the invisible starting point,the riblets will greatly increase the flui drag friction owing to the cross-stream translation and consequent effects of coherent structures.The aforementioned drag-reducing riblets have the best shark-skin effect or optimal drag-reduction benefi when their peak-topeak spacing s+is at an optimum value of 17［34］.The riblets become ineffective and can even become a dragincreasing device as a result of streamwise vortices and their behaviors in the spanwise direction based on the two previously discussed theories.However,García-Mayoral and Jiménez［34,35］believedthatsuchabreakdownisduetothe onset of a Kelvin-Helmholtz instability in the TBL over the drag-increasing riblets,which introduces a kind of Kelvin-Helmholtz-like roller structure.These interesting spanwise roller structures were recently clearly observed by Yang et al.［36］for the firs time in wind tunnel experiments utilizing a smoke flw visualization technique.

In the present study,the effect of shark-skin-inspired riblets on coherent vortex structures in the TBL is experimentally investigated by means of planar and tomographic particle image velocimetry（TPIV）measurements inchannel flwsoveranacrylicplateofdrag-reducingriblets.TheTPIV system and experimental setups for the planar PIVand TPIV measurements,together with a data analysis method（the improved quadrant splitting method,IQSM［20］）for detecting and extracting a new statistical model of quadrupole coherent structures from a database of three-dimensional three-component（3D3C）velocity vectors,are presented in Sect.2.Results and discussion on the drag-reduction mechanism for shark-skin-inspired riblets are presented in Sect.3 from the perspective of their influenc on the topologies of quadrupole coherent structures.Lastly,several conclusions are summarized in Sect.4.

2　Experimental apparatus and methods

2.1TPIV system

Particle image velocity has evolved into a dominant method for velocimetry in experimental fluimechanics and has made a significan contribution to our understanding of real turbulent flws［37］.Furthermore,TPIV was recently introduced by Elsinga et al.［38］as a promising new technique to measure the instantaneous 3D3C velocity fielbased on optical tomography and the PIV method.Its principle in detail and research/engineering applications have been reviewed by Westerweel et al.［37］and Gao et al.［39］.Here we briefl introduce the TPIV system（Fig.3）at the Fluid Dynamics Laboratory of Tianjin University（FDLTU）and its operating principle.The TPIV system consists of fi e different kinds of subsystem:（1）a laser illumination system （Warwickshire,England,UK,Litrondualpowerlasers,LDY-304-PIV）;（2）animagepairrecordingsystem（cameras,three SpeedSense9072imagers）;（3）asynchronouscontrolsystem （synchronizer,timer box）;（4）tracer particles（Skovlunde, Denmark,Dantec polyamid seeding particles,PSP-50）; （5）a data analysis and visualization system（DynamicStudio base package and Tecplot）.The working principle of this TPIV system is schematically illustrated in Fig.3.First, tracer particles,PSP-50 immersed in water channel flw, are illuminated by the LDY-304-PIV laser.Image pairs are then recorded simultaneously from three viewing directions using the three SpeedSense 9072 imagers.A Scheimpflu adapter mounted between the imager and the beam is used to keep the Scheimpflu condition among the image plane, lens plane,and mid-object plane.In addition,to avoid a large angle of refraction,a water prism is installed in the system that is able to ensure that the particle images have better focus in each imager.Then a separate multicamera calibration procedure needs to be followed to determine the mapping between image coordinates and the physical object space of the measurement volume.Third,based on the mapping,the three recorded pairs of images are reconstructed into a pair of volumetric particle images to obtain the 3D particle displacement using a tomographic algorithm （multiplicative algebraic reconstruction technique,MART ［38］）.Finally,the velocity vector fiel of object flws can be obtained by directly applying a cross-correlation analysis （e.g.,least-squares matching,LSM［40］）because the reconstructedvolumesare3Darraysoftheintensityoftheparticle distribution at two exposures.

2.2Experimental setups

PIVexperimentswereperformedinalow-speedwaterchannel with a test section of 0.38m×0.25m×5.32m（height ×width×length）at the FDLTU.The TBL flws were fully developedoveranacrylicsmoothplateandatriangularriblet plate separately.Both plates had the same dimensions of 0.01m×0.138m×1.2m（thickness×width×length）, and longitudinal riblet grooves with a peak-to-peak physical spacing,s,of 1.6mm were aligned with the flw direction. A tripwire with a diameter of 6mm was placed 115mmdownstream of the leading edge of the plates to trip the flw into fully developed turbulent flws.The PIV measurements were typically taken at the streamwise distance,x, around 575mm downstream of the tripwire.The free stream velocity of the TBL flw was 0.155m/s.The water temperature was kept at（22±0.5）◦C and the pressure at 1.01× 105Pa when the experiments were conducted,giving a kinematic viscosity（ν）and density（ρ）of the water of 9.6×0−7m2/s and 997.8kg/m3,respectively.A schematic diagram of the planar and 3D3C experimental setups are shown in Fig.4.

Fig.3 TPIV system at Fluid Dynamics Laboratory of Tianjin University

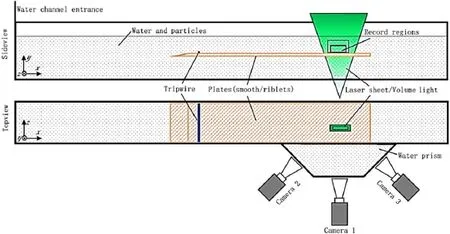
Fig.4 Schematic diagram of experimental configuratio

To verify the basic flws and the drag-reduction performance over ariblet plate,the planar PIVmeasurements were firs carried out using camera 1,one of three cameras of the TPIV system.In the experiments,the sampling frequency was 500Hz in single-frame mode,and a total of 8001 frames of images were recorded at a view of the x-y plane.［NB:we will use（x,y,and z）for streamwise,wall-normal,and spanwise directions and（u,v,w）for their corresponding velocity components of the TBL flws.］After a standard 2D calibration,the recorded region for planar PIV measurements was around86.2mm×53mm（x×y）.ThenTPIVmeasurements were conducted in the same flw conditions after a separate standard 3D calibration.The 3D calibration process will be elaborated on in Sect.2.3.The recorded region of the flws forTPIVmeasurementswasapproximately60mm×45mm ×30mm（x×y×z）.

2.3Multicamera calibration and data processing

Multicameracalibrationwasappliedtogeneratethemapping of points from the object measurement volume space to the imageplanes,wheremultiplecameras（thethreeSpeedSense 9072 imagers in the system）were calibrated simultaneously. To do this,a 3D calibration target（100mm×100mm,down to30mm×30mm）wasusedintheexperiments.Inwhatfollows,thetermposeisusedtodescribeaspecifi locationand orientation of the 3D calibration target in the measurement space.The firs target pose（the reference pose）aligned with thestreamwisedirectionandfacedcamera1;thenthesecond tofift posesmaintainedthesameorientationasthefirs pose butwereseparatelymovedforwardandbackwardtwiceevery 5mm to identify the coordinate system of the image planes. Next,the target was rotated clockwise and counterclockwise from the reference pose fi e times of each 4◦,respectively. In all 15 poses of the target,one corresponding image was recordedbyeachcamera.Therefore,3imageensembleswith 15 acquired calibration images in each ensemble from the 3 cameras were obtained for the 3D multicamera calibration. The calibration procedure was completed by applying the multicamera calibration method of DynamicStudio 3.40 to the three image ensembles.The calibrated area is−45mm ≤x≤45mm;−7.5mm≤y≤45mm;−16.76mm≤z≤16.5mm,withanaveragereprojectionerrorof0.471604pixelsforCamera1,0.423087pixelsforCamera2,and0.560013 pixels for Camera 3.

After the multicamera calibration,2500 pairs of particle images of the object flws over smooth and riblet plates were separately recorded by each camera of the TPIV system.The sampling frequency of all three cameras was set to 500Hz.Each of the three groups of 2500 image pairs from three different views of the cameras were used to reconstruct 2500 pairs of volumetric particle images.Time series of 3D3C velocity vector field in the TBLs over both plates were eventually obtained by simultaneously selecting the three groups of 2500 volumetric image pairs and the multicamera calibration file when the LSM method was conducted.The voxel reconstruction space was−30mm≤x≤30mm;0mm≤y≤45mm;and−15mm≤z≤15mm,and each 3D3C velocity fielconsisted of 51×37×16（x×y×z）grid points/vectors,which means an increment of 1.44mm in the spanwise and 1.08mm in the streamwise and wall-normal directions.

Animprovedsplittingquadrantmethod,i.e.,theImproved splittingquadrant method（ISQM）,wasinitiallyproposedby Yang et al.［20］to detect and extract the spatial topologies of the coherent structures in the TBLs.This method applied themultiscalespatiallocallyaveragedstructurefunction［41］of the streamwise velocity component at different scales to the database of 3D3C velocity vector field measured by the TPIV system.The ISQM describes the bursting events of coherent structures in the TBLs with four quadrants of the fluctuatio velocity components at the u′-v′plane,namely, the first-quadran event（Q1,u′＞0,v′＞0）,the secondquadrant event（Q2,u′＜0,v′＞0）,the third-quadrant event （Q3,u′＜0,v′＜0）,andthefourth-quadrantevent（Q4,u′＞0,v′＜0）.Specificall,the Q2 and Q4 bursting events are also referred to as ejection and sweep events,respectively, which are the most important contributions to the Reynolds shearstress［8］andtheturbulentskinfrictiondrag［42］.More details on the ISQM is given in Ref.［20］.

3　Results and discussion

3.1Planar PIV measurements

To verify object TBL flws and the drag-reduction benefi over a riblet plate,all 8001 frames of images recorded by camera 1 of the PIV system were analyzed using Dynamic-Studio 3.40 in the following steps:adaptive correlation with interrogation windows of 32 pixels×32 pixels and an overlap rate of 50%;UV scatter plot range validation and the last stepofmovingaveragevalidation.Timeseriesof80002D2C instantaneous velocity vectors were eventually obtained in which each grid point of the vector corresponded to every 0.55272mmincrement.Thefreestreamvelocitieswerefi ed at 0.155m/s.The thicknesses of the TBL flws at the measured region were approximately the same（θ≈21mm）. More flw parameters are summarized in Table 1,where the skin friction velocity,skin friction,and friction coeffi cient were calculated using a mean-velocity-profil method ［43］in the near-wall region of the TBLs over these twoplates.To use this method,the instantaneous streamwise components of the velocity vectors were initially averaged in time and then in space along the streamwise direction. Then,Newton iteration approximation and a conjugate gradient technique were applied to the data of logarithmic law （log-law）mean streamwise velocity profiles Subsequently a moderate drag-reducing rate of 4.81%was obtained by performing subtraction between the different friction coefficient of the TBLs over the riblet plate and its smooth counterpart.As the systematic uncertainty of the planar PIV system is the same for the measurements of two plates, whichcannotaffecttheevaluationofthedrag-reductionrate, therefore,a real shark-skin effect of 4.81%over riblets is credible.

A log-law plot of the space-time-averaged dimensionless streamwise velocity profile and Reynolds shear stress distributions is shown in Fig.5 against y+for both plates at a peak-to-peakspacings+of14.Thecharacteristicsofthedrag reductionareclearlyvisible.Theslopeofthelogarithmiclaw over riblets remained the same as that of the smooth plate. However,in the riblet scenario,the intercept of the law was increased,identifying an upward-shifting trend of the mean velocity profil and a thickened buffer layer［26］of the TBL. The virtual origin of the riblets was obtained by Bechert and Bartenwerfer（Fig.17 in Ref.［29］）depending on the shape. In Fig.5,the protrusion height of 0.14s+was subtracted to replotthecorrespondingvaluesfortheribletsherein.Smaller wall-normal gradients of the mean streamwise velocity indicate a smaller wall friction,although U+at the buffer layer of the TBL over the riblet plate is slightly larger than that over its smooth counterpart.In addition,the Reynolds shear stress is related to the Q2 and Q4 events,which significantl contributes to the skin friction drag near the wall.Figure 5 showsamuchlowerReynoldsshearstressbelow y+of120in the TBL flw over riblets.Moreover,the peak location of the Reynoldsshearstressmovedbackwardfromaround y+of18 to 120.It should be stressed that in this paper,the Reynolds shearstressisratherlargenearriblettips［35］,whilethecorresponding value of the smooth plate is relatively small（Fig.5 inRef.［6］）,althoughitwasnotresolvedinthisstudyowingto the planar PIV resolution.In addition,a reduction of the skin friction is a direct phenomenon and a direct consequence of the wall surface,i.e.,riblets,while the Reynolds shear stress isanindirecteffectfromtheshark-skin-likeribletssuppressingthebursting（suchassweepevents）ofcoherentstructures in the TBL.Therefore,the relative low drag-reduction rate of less than 5%was obtained for the whole TBL over the riblets,which is not as much as that high indicated by the difference in the Reynolds shear stress at a larger distance from the wall over both plates.In conclusion,there is a drag reduction of the shark-skin-inspired riblets in the TBL flw, and it is necessary and feasible to study the shark-skin effect on the coherent vortex structures over the riblets by TPIV, like the topological changes of the coherent structures below y+of 120 in the TBL flw.

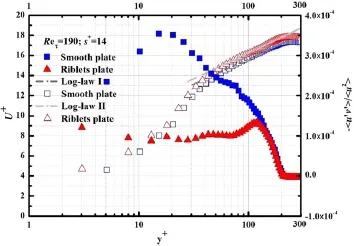
Fig.5　Space-time-averaged dimensionless streamwise velocity and Reynolds shear stress distributions against y+scaled with inner variables of the TBLs over the smooth and riblet plates measured by the planar PIV

Table 1　Basic flw and drag-reduction parameters of TBLs over smooth plate and riblets measured by planar PIV

3.2TPIV measurements

Each of the 2500 3D3C instantaneous velocity vectors over both the smooth and riblet plates measured by TPIV were firs averaged in time,and the streamwise velocity contours are shown in Fig.6a,b.There is a more intuitive view on the thickened sublayer,which is the drag-reduction feature inthe TBL flw over riblets.Then the time-averaged streamwise components of the velocity vectors were averaged inspace.Comparisons for the streamwise velocity profile of the TBLs over both plates separately measured by planar PIV and TPIV systems are plotted in Fig.6c,d.Their good collapse with less than 6%maximum error shows that the time series of the 3D3C instantaneous velocity vectors are reliable for further analysis.Similar observations on errors shownhereinreflectin themeanvelocitydifferencebetween smoothandribletplatespresentasystematictrendfrom−2% to 6%for y+＜75 at the near-wall region,which implies that the error might be caused by the difference between the planarPIVandTPIV.ThecorrespondingresolutionsofTPIV measurements are 13WU（1.44mm,nondimensionalized by the skin friction velocity uτof 8.671×10−3m/s in the planar PIV measurement over the riblet plate）in the spanwise and 9.75WU（1.08mm）in the streamwise and wall-normal directions,respectively.

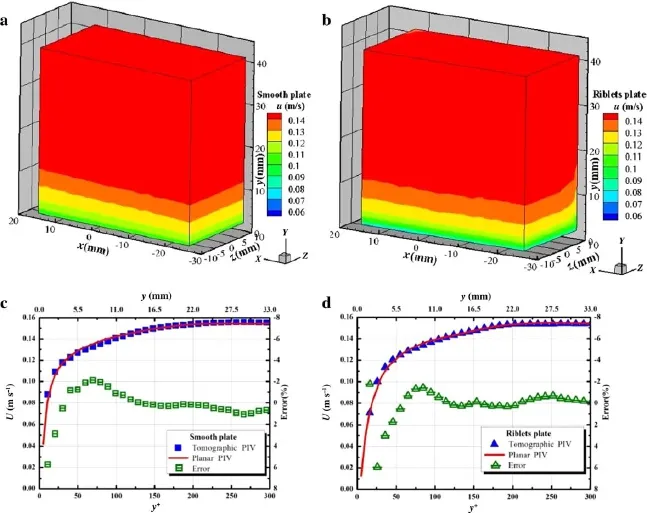
Fig.6　Velocitiesof object TBL flws.［Time-averaged streamwise velocity contours of TBLs over smooth plate a and riblets b measured by TPIV; space-time-averaged streamwise velocity profile of TBLs over smooth plate c and riblets d separately measured by planar PIV and TPIV systems］

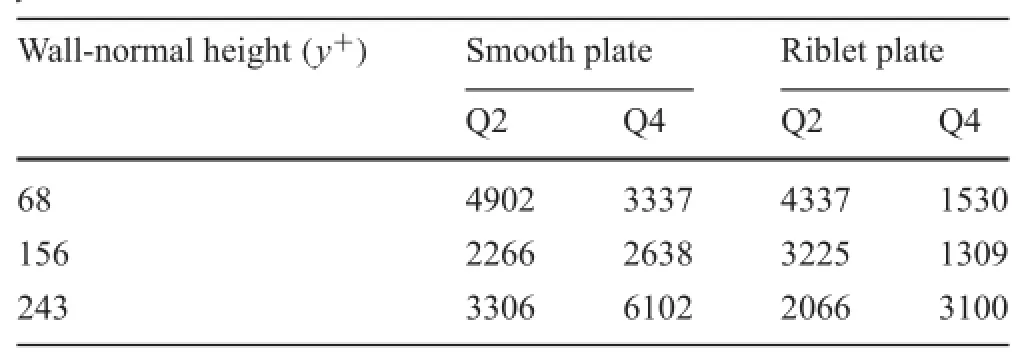
Table2　Statisticsofburstingeventsforejection（Q2）andsweep（Q4）of TBL flws over smooth plate and riblets at fourth scale with increasing y+

To study the shark-skin effect on coherent vortex structuresoverriblets,thedatabaseoftimeseriesof3D3Cvelocity vector field was further analyzed using the ISQM when the wall-normalheightsofthedetectingcenter y+arearound68 （inner layer）,156（log-law layer）,and 243（outer layer）.Statistical values of bursting events for Q2 and Q4 of the TBL flws over the smooth plate and its riblet counterpart at the fourth scale are given in Table 2.Note that only the fourth scale（corresponding to 195WU scaled with inner variables）is discussed in this paper owing to the limitation of 16 vector grid points（24）in the spanwise direction.The frequencies of Q4 events at all three wall-normal heights are reduced by half because of the shark-skin-inspired riblets,whereas the frequency of Q2 events at y+≈156 show an increase of 30%for the riblet case,which may be explained as follows: （1）riblets demonstrate a shark-skin effect at the inner layer oftheTBLwherethehairpinvortexlegsorquasi-streamwisevortices are located in general by effectively suppressing Q2 and Q4 bursting events,which can be seen by the Reynolds shear stress distributions in Fig.5 as well;（2）Q4 events have a greater contribution to skin-friction drag［26］,and so riblets exert a shark-skin effect in the whole TBL by suppressing Q4 events in particular;（3）riblets may not have a shark-skin effect on flws at the log-law layer and the outer layer since Q2 events may occur at random where there are more hairpin vortex heads intheselayers［6］.Inaddition,Q2 events eject low-speed flui away from the wall while Q4 events sweep high-speed flui toward the near-wall surface, so it makes sense that the riblets have a more direct effect on Q4 events than Q2 bursting events.Therefore,more details on the changes in the topological shapes and spatial scales of coherent structures in the TBL at these three normal heights over the riblets should be explored when Q4 bursting events happen.

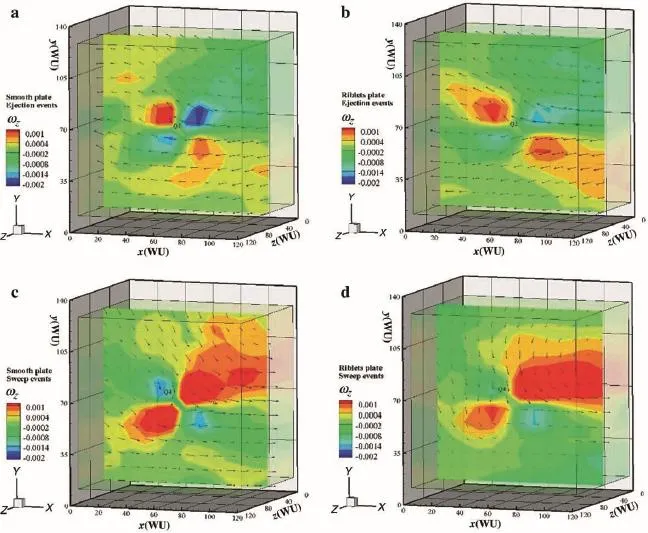
Fig.7　Slices of bursting events for Q2（up）and Q4（down）of the TBL flws over the smooth plate（left）and riblets（right）at the fourth scale when the wall-normal height of the detecting center y+≈68.The contour in eachslice indicates the conditional-averaged spanwise vorticity,while vectors are fluctuatin velocities

Spanwise slices of bursting events for Q2 and Q4 of the TBL flws over the smooth plate and riblets at the fourth scale are plotted in Fig.7,where the wall-normal height of the detecting center,y+,is approximately 68.At the detectingcenter,theISQMsuccessfullycapturesthecharacteristics of bursting events for Q2:ejecting the low-momentum flui （u′＜0）away from the wall（v′＞0）（Fig.7a,b）,and Q4:sweeping the high-momentum flui（u′＞0）toward the wall（v′＜0）（Fig.7c,d）.Contours in the slices indicate conditional-averaged spatial topologies of the spanwise vorticity in the TBLs over the smooth and riblet plates.The clearquadrupolestructuresmentionedinSect.1areshownat the slice centers.The shark-skin-inspired riblets weaken the amplitudes of the spanwise vorticity when Q2 and Q4 events occur,but no marked changes in the spatial conditionalaveraged topological shapes of the coherent structures were observed at the planar slices,which are the same as the previous planar PIV measurements［44］over riblets.However, their topologies in scale size were changed by riblets,which is illustrated more clearly in Fig.8.

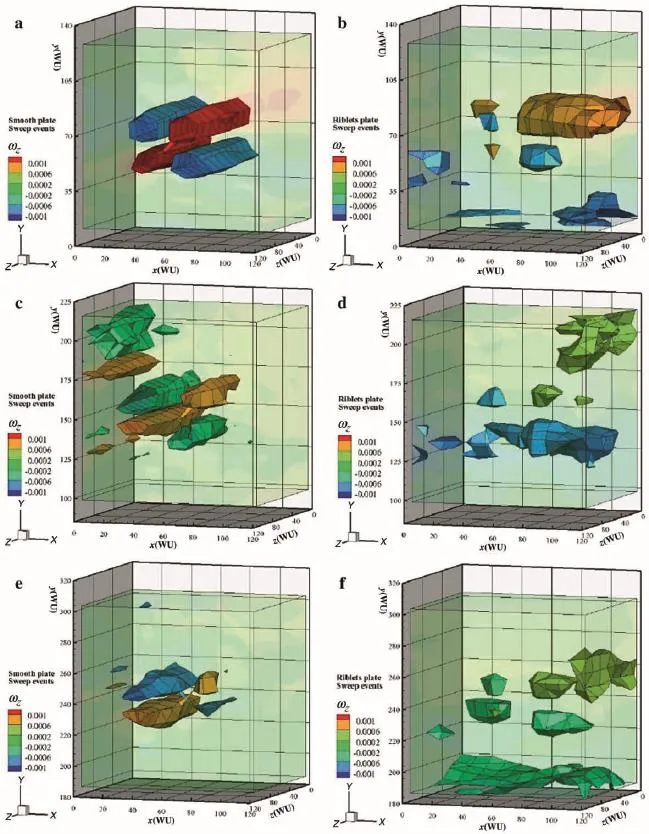
Fig.8　Conditional-averaged spanwise vorticity isosurfaces of Q4 bursting events in TBL flws over smooth plate（left）and riblets（right）at fourth scale when the wall-normal height of the detecting center y+≈68（up）,156（middle）,and 243（down）

Figure 8 shows conditional-averaged topologies of the coherentstructureswithspanwisevorticityisosurfacesinthe TBL flws over the smooth plate（left）and riblets（right）at the fourth scale when the Q4 bursting events were detected and extracted at different wall-normal heights of y+≈68 （a-b）,156（c-d）,and 243（e-f）.It seems that the shark-skin effect on the coherent vortex structures over the shark-skininspired riblets may exist in the whole TBL,not only on the vorticity amplitudes,but also on their spatial scales.The control mechanism that is suppressing the structures in the log-law/outerlayers,whichoriginatesfromthestrongmanipulationofriblets,issomewhatsimilartotheresultsofXuetal. ［45］providedthatthesequadrupolestructuresareconsidered one kind of large-scale motion and the riblets an opposition control device.However,this effect was not reflecte by the planar PIV result in Fig.5,where the Reynolds stress profile along the wall-normal direction of both plates are different only below y+of 120 in the TBL flw,which can be attributed to the three-dimensional characteristics of the coherent vortex structures at the log-law/outer layers in the TBL.In particular,as shown in Fig.8a,a classic standard quadrupole coherent structure with an alternatingly positive and negative spanwise vorticity is clearly visible over the smooth plate,and there may be a better two-dimensionality for it at the inner layer of the TBL.In Fig.8b,the core region of the corresponding structure over the riblets in scale size is smaller and to some extent becomes more three-dimensional apart from a smaller amplitude of spanwise vorticity for the topological structure.The smaller spanwise vorticity of the core regions of the quadrupole coherent structure means that less induced high-momentum flui is swept toward the wall,which inhibits the energy and momentum exchange, thereby reducing the skin friction drag because all momentum transferred parallel to the surface of an object results in a corresponding increase in drag［22］.Meanwhile,as y+increases up to 156 at the log-law layer and 243 at the outer layer,the shark-skin effect of riblets on the quadrupole coherent structures shows some similarity to the spatial conditional-averaged topological changes that occur at the innerlayeroftheTBL.Ribletsweakentheamplitudeofspanwise vorticity for the topological quadrupole structure and enlarge their length scales at the streamwise direction near thedetectingcenter（Fig.8c-f）,althoughseveralsmallersecondary vortices were induced upstream.Smaller secondary vortices may reveal that the shark-skin effect of riblets has been weakened.

3.3Discussion

The drag-reduction rate of 4.81%for riblets in this study is comparatively lower than the optimal value of around 10%［22］owing to the choice of s+,which was limited by the free-stream velocity of the water channel at the FDLTU.In the future,the optimum value for s+of 17 ［34］should be achieved for a better understanding of the drag-reducing mechanism of the shark-skin-inspired riblets. Moreover,since only a minimum 9.75WU of the resolution in the wall-normal direction was obtained by TPIV and it was not possible to resolve the linear viscous sublayer region sufficientl,in the future,fine resolution to the Kolmogorov microscale should be achieved（＜5WU［20］）by altering the density of particles and the setting positions of the volumetric light source.A fine resolution would make it possible to resolve smaller-scale coherent structures in the TBLandthoseclosetothewallbelow y+of68.Furthermore, the quadrupole structure of the TBL itself is a new statistical model for coherent structures;therefore,its dynamics and formation mechanism also call for further investigation.

4　Conclusions

In summary,planar PIV and TPIV measurements were performed to study the effect of shark-skin-inspired riblets on quadrupolecoherentvortexstructuresinTBLflwsataTBL thickness-based friction Reynolds number of 190.The planar PIV experimental result reveals a drag-reduction rate of around 4.81%over the riblet plate,with peak-to-peak spacings+of14.Thedrag-reducingcharacteristicsareshownby the thickened buffer layer in the TBL,the upward shifted log-law region,and the decreased Reynolds shear stress, confirminthe shark-skin effect on riblets.The results of the TPIV measurements verifiethe existence of quadrupole coherent vortex structures,which were detected and extracted by the ISQM at three different wall-normal heights in the TBL flws over both smooth and riblet plates,respectively.The riblets weakened the amplitudes of the spanwise vorticity when Q2 and Q4 events occurred at the near wall, having especially more depressing effects on the Q4 events. However,the shark-skin effect of the riblets seemed to extend to the log-law layer and the outer layer owing to the three-dimensional characteristics of the quadrupole coherent vortex structures in the TBL.Lastly,the riblets reduced the skin friction drag by changing the spatial conditionalaveragedtopologiesandtheirspatialscalesofthequadrupole coherent structures as a whole in the overlying turbulent flw and by depressing the downwash of high-momentum fluiduring Q4 events,which eventually dampened the momentum and energy exchange of the flw over the sharkskin-inspired riblet plate.

AcknowledgmentsThe authors would like to thank Philip Cohen at the University of Nottingham for his invaluable suggestions for writing the manuscript.The project was supported by the National Natural Science Foundation of China（Grants 11332006,11272233, and11411130150）,thefoundationfrom theChinaScholarship Council （CSC）（Grant201306250092）,andtheFoundationProjectforOutstanding Doctoral Dissertations of Tianjin University.

References

1.Kline,S.J.,Reynolds,W.C.,Schraub,F.A.,et al.:The structure of turbulent boundary layers.J.Fluid Mech.30,741-773（1998）

2.Cantwell,B.J.:Organized motion in turbulent flw.Annu.Rev. Fluid Mech.13,457-515（1981）

3.Robinson,S.K.:Coherent motions in the turbulent boundary layer. Annu.Rev.Fluid Mech.23,601-639（1991）

4.Lumley,J.L.,Yaglom,A.M.:Acenturyofturbulence.FlowTurbul. Combust.66,241-286（2001）

5.Wallace,J.M.:Highlightsfrom50yearsofturbulentboundarylayer research.J.Turbul.13,1-70（2013）

6.Adrian,R.J.,Meinhart,C.D.,Tomkins,C.D.:Vortex organization in the outer region of the turbulent boundary layer.J.Fluid Mech. 422,1-54（2000）

7.Adrian,R.J.:Hairpin vortex organization in wall turbulence.Phys. Fluids 19,41301（2007）

8.Tang,Z.Q.,Jiang,N.,Schröder,A.,et al.:Tomographic PIV investigation of coherent structures in a turbulent boundary layer flw. Acta Mech.Sin.28,572-582（2012）

9.Schröder,A.,Geisler,R.,Staack,K.,etal.:EulerianandLagrangian viewsofaturbulentboundarylayerflwusingtime-resolvedtomographic PIV.Exp.Fluids 50,1071-1091（2011）

10.Elsinga,G.E.,Kuik,D.J.,van Oudheusden,B.W.,et al.:Investigation of the three-dimensional coherent structures in a turbulent boundarylayerwithTomographic-PIV.45thAIAAAerospaceSciences Meeting and Exhibit,Reno,Nevada（2007）

11.Ganapathisubramani,B.,Longmire,E.K.,Marusic,I.:Characteristicsofvortexpacketsinturbulentboundarylayers.J.FluidMech. 478,35-46（2003）

12.Pan,C.,Wang,J.J.,Zhang,C.:Identificatio of Lagrangian coherent structures in the turbulent boundary layer.Sci.China Phys. Mech.Astron.52,248-257（2009）

13.Xu,C.X.,Deng,B.Q.,Huang,W.X.,et al.:Coherent structures in wall turbulence and mechanism for drag reduction control.Sci. China Phys.Mech.Astron.56,1053-1061（2013）

14.Chen,J.,Hussain,F.,Pei,J.,et al.:Velocity-vorticity correlation structure in turbulent channel flw.J.Fluid Mech.742,291-307 （2014）

15.Wu,X.H.,Moin,P.:Forest of hairpins in a low-Reynolds-number zero-pressure-gradient flat-plat boundary layer.Phys.Fluids 21, 91106（2009）

16.Wu,X.H.,Moin,P.:Direct numerical simulation of turbulence in a nominallyzero-pressure-gradientflat-plat boundarylayer.J.Fluid Mech.630,5-41（2009）

17.Lesieur,M.,Begou,P.,Briand,E.,etal.:Coherent-vortexdynamics in large-eddy simulations of turbulence.J.Turbul.4,1-24（2003）

18.Zhang,D.,Luo,J.,Zhou,H.:Dynamicmodelofcoherentstructure in the wall region of a turbulent boundary layer.Sci.China Phys. Mech.Astron.46,291-299（2003）

19.Tian,H.P.,Yang,S.Q.,Cheng,L.,etal.:Antisymmetricquadrupole mode of coherent structures in wall-bounded turbulence.Theor. Appl.Mech.Lett.3,52002（2013）

20.Yang,S.Q.,Jiang,N.:Tomographic TR-PIV measurement of coherent structure spatial topology utilizing an improved quadrant splitting method.Sci.China Phys.Mech.Astron.55,1863-1872 （2012）

21.Tian,H.P.,Jiang,N.,Huang,Y.X.,et al.:Study on local topology model of low/high streak structures in wall-bounded turbulence by tomographic time-resolved particle image velocimetry.Appl. Math.Mech.Engl.Ed.36,1121-1130（2015）

22.Dean,B.,Bhushan,B.:Shark-skinsurfacesforfluid-dra reduction inturbulentflw:areview.Philos.Trans.R.Soc.A368,4775-4806 （2010）

23.Luo,Y.:Recent progress in exploring drag reduction mechanism of real skin surface:a review.J.Mech.Med.Biol.15,1530002 （2015）

24.Saravi,S.S.,Cheng,K.:A review of drag reduction by riblets and micro-textures in the turbulent boundary layers.Eur.Sci.J.9,62-81（2013）

25.Goldstein,D.B.,Tuan,T.C.:Secondary flw induced by riblets.J. Fluid Mech.363,115-151（1998）

26.Choi,K.S.:Near-wall structure of a turbulent boundary layer with riblets.J.Fluid Mech.208,417-458（1989）

27.Karniadakis,G.E.,Choi,K.S.:Mechanisms on transverse motions in turbulent wall flws.Annu.Rev.Fluid Mech.35,45-62（2003）

28.Goldstein,D.,Handler,R.,Sirovich,L.:Direct numerical simulation of turbulent flw over a modelled riblet covered surface.J. Fluid Mech.302,333-376（1995）

29.Bechert,D.W.,Bartenwerfer,M.:The viscous flw on surfaces with longitudinal ribs.J.Fluid Mech.206,105-129（1989）

30.Lee,S.J.,Lee,S.H.:Flow fiel analysis of a turbulent boundary layer over a riblet surface.Exp.Fluids 30,153-166（2001）

31.Suzuki,Y.,Kasagi,N.:Turbulentdragreductionmechanismabove a riblet surface.AIAA J.32,1781-1790（1994）

32.Jung,Y.C.,Bhushan,B.:Biomimetic structures for fluidrag reduction in laminar and turbulent flws.J.Phys.Condens.Matter 22,35104（2010）

33.Bixler,G.D.,Bhushan,B.:Fluid drag reduction with shark-skin riblet inspired microstructured surfaces.Adv.Funct.Mater.23, 4507-4528（2013）

34.García-Mayoral,R.,Jiménez,J.:Drag reduction by riblets.Philos. Trans.R.Soc.A 369,1412-1427（2011）

35.García-Mayoral,R.,Jiménez,J.:Hydrodynamic stability and breakdown of the viscous regime over riblets.J.Fluid Mech.678, 317-347（2011）

36.Yang,S.Q.,Choi,K.S.,Jiang,N.:Flow visualization on Kelvin-Helmholtz-like roller structures in turbulent boundary layer over riblets.Chin.J.Theor.Appl.Mech.47（3）,529-533（2015）（in Chinese）

37.Westerweel,J.,Elsinga,G.E.,Adrian,R.J.:Particle image velocimetry for complex and turbulent flws.Annu.Rev.Fluid Mech.45,409-436（2013）

38.Elsinga,G.E.,Scarano,F.,Wieneke,B.,etal.:Tomographicparticle image velocimetry.Exp.Fluids 41,933-947（2006）

39.Gao,Q.,Wang,H.P.,Shen,G.X.:Review on development of volumetric particle image velocimetry.Chin.Sci.Bull.58,4541-4556 （2013）

40.Westfeld,P.,Maas,H.,Pust,O.,et al.:3-D least squares matching for volumetric velocimetry data processing.In:Proceedings of the 15th International Symposium on Applications of Laser Techniques to Fluid Mechanics.Lisbon,Portugal,5-8 July,2010

41.Liu,W.,Jiang,N.:Three kinds of velocity structure function in turbulent flws.Chin.Phys.Lett.21,1989-1992（2004）

42.Huang,L.,Choi,K.S.,Fan,B.,et al.:Drag reduction in turbulent channel flw using bidirectional wavy Lorentz force.Sci.China Phys.Mech.Astron.57,2133-2140（2014）

43.Fan,X.,Jiang,N.:Skin friction measurement in turbulent boundary layer by mean velocity profil method.Mech.Eng.27,28-30 （2005）.（in Chinese）

44.Li,S.,Yang,S.Q.,Jiang,N.:TRPIV measurement of dragreduction in the turbulent boundary layer over riblets plate.Chin. J.Theor.Appl.Mech.45,183-192（2013）.（in Chinese）

45.Deng,B.Q.,Xu,C.X.,Huang,W.X.,et al.:Effect of active control on optimal structures in wall turbulence.Sci.China Phys.Mech. Astron.56,290-297（2013）

8 February 2015/Revised:7 June 2015/Accepted:8 June 2015/Published online:7 December 2015

✉Shao-Qiong Yang

shaoqiongy@tju.edu.cn

Nan Jiang

nanj@tju.edu.cn

1Department of Mechanics,Tianjin University, Tianjin 300072,China

2Tianjin Key Laboratory of Modern Engineering Mechanics, Tianjin 300072,China

3School of Mechanical and Aerospace Engineering, Nanyang Technological University, Singapore 639805,Singapore