关于近似R-正交的注记
2016-08-13孔亮王念良
孔亮,王念良
(商洛学院 数学与计算机应用学院,陕西商洛 726000)
关于近似R-正交的注记
孔亮,王念良
(商洛学院 数学与计算机应用学院,陕西商洛726000)
在复赋范线性空间,利用范数导数的定义和性质,并运用算子论方法,证明了近似R-正交是近似ρ-正交,给出了弱近似R-正交的定义,并证明了在一定条件下,近似ρ-正交是弱近似R-正交。
R-正交;近似R-正交;弱近似R-正交;近似正交
在20世纪初,R-正交、B-正交、I-正交等正交性定义被相继引入用来研究赋范线性空间的几何性质[1-3]。此后,新的正交性定义被不断引入和研究,比如文献[4]在Banach空间中给出新的正交性定义,文献[5-6]引入了ρ-正交定义。近年来,许多学者推广了以上正交性定义得到相应的近似正交性定义。文献[7-8]在复赋范线性空间中给出了近似B-正交的定义和性质,文献[9-10]在实赋范线性空间中分别引入了近似I-正交和近似R-正交的定义,关于其它各种正交性和近似正交性定义和相关性质已有许多研究[11-16]。受上述定义和结论的启发,本文在复赋范线性空间,证明近似R-正交是近似ρ-正交,给出弱近似R-正交的定义,并证明在一定条件下,近似ρ-正交是弱近似R-正交。
1 预备知识和结论
X在本文中均表示复赋范线性空间,R表示实数集,C表示复数集,U(0;δ)表示在复平面上以原点为中心,以δ>0为半径的邻域。


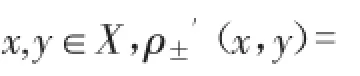
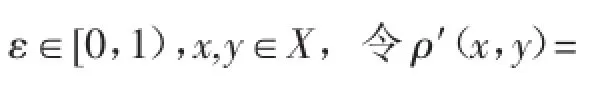
文献[16]在复赋范线性空间中给出了范数导数的性质。


本文利用定义3和定理1,主要得到下面的结论。
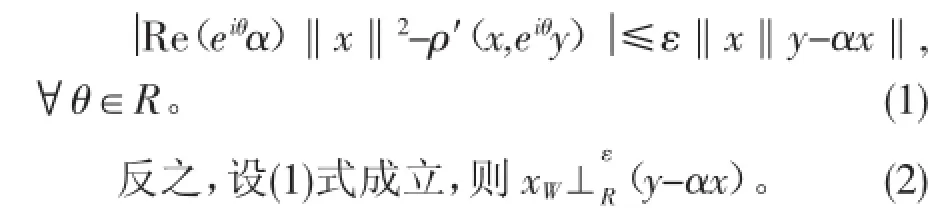
2 结论的证明
为了完成定理2的证明,先给出下面的引理。



反之,设(1)式成立,则由定理1中4)知


证明 在(1)式中令α=0可知结论成立。
证明 在定理3中令θ=0可知结论成立。
证明 在(2)式中令α=0可知结论成立。
[1]ROBERTS B D.On the geometry of abstract vector space[J].TÔhoku Math J,1934,39:42-59.
[2]BIRKHPFF G.Orthogonality in linear metric space[J]. Duck Math J,1935,1(2):169-172.
[3]JAMES R C.Orthogonality in normed linear space[J]. Duck Math J,1945,12(2):291-302.
[4]SAIDI F B.An extension of the notion of orthogonality to Banach spaces[J].J Math Anal Appl,2002,267(1):29-47.
[6]WÓJCIK P.Linear mappings preserving ρ-orthogonality [J].J Math Anal Appl,2012,386(1):171-176.
[8]MOJšKETC B,TURNšEK A.Mapping approximately preserving orthogonality in normed spaces[J].Nonlinear Anal,2010,73(12):3821-3831.
[10]ZAMANI A,MOSLEHIAN M S.Approximate Roberts orthogonality[J].Aequationes Mathematicae,2013,89(3):529-541.
[11]张芳娟,吉国兴.B(H)上保正交性的可加映射[J].陕西师范大学学报(自然科学版),2005,33(4):21-25.
[12]ILIšEVIC D,TURNšEK A.Approximately orthogonality preserving mappings on C*-modules[J].J Math Anal Appl,2006,318(2):433-443.
[13]付向红,黎永锦.Banach空间的非常B-正交性[J].中山大学学报(自然科学版),2008,47(1):116-117.
[14]ALONSOJ,MARTINIH,WUSL.OnBirkhofforthogonality and isosceles orthogonality in normed linear spaces[J]. Aequationes Math,2012,83(1):153-189.
[15]孔亮.ε-近似保内积的某个特定值映射[J].商洛学院学报,2014,28(6):13-15.
[16]陈超群.保持正交的线性映射[D].苏州:苏州大学,2013:3-5.
(责任编辑:李堆淑)
Notes on Approximate R-orthogonality
KONG Liang,WANG Nian-liang
(College of Mathematics and Computer Application,Shangluo University,Shangluo726000,Shaanxi)
In a complex normed linear space,it is proved that approximate R-orthogonality is approximate ρ-orthogonality by the definition and properties of norm derivative and the methods of operator theory.Next the definition of weak approximate R-orthogonality is given.It is proved that approximate ρ-orthogonality is weak approximate R-orthogonality under certain condition.
R-orthogonality;approximate R-orthogonality;weak approximate R-orthogonality;approximate ρ-orthogonality
O177.1
A
1674-0033(2016)04-0003-03
10.13440/j.slxy.1674-0033.2016.04.002
2016-04-26
陕西省自然科学基础研究计划项目(2016JM1034);陕西省教育厅专项科研计划项目(16JK1238)
孔亮,男,陕西商州人,硕士,讲师
