Time-space dependent fractional boundary layer flow of Maxwell fluid over an unsteady stretching surface

Shengting Chen，Liancun Zheng，Bingyu Shen，Xuehui Chen

School of Mathematics and Physics，University of Science and Technology Beijing，Beijing 100083，China

Time-space dependent fractional boundary layer flow of Maxwell fluid over an unsteady stretching surface

Shengting Chen，Liancun Zheng∗，Bingyu Shen，Xuehui Chen

School of Mathematics and Physics，University of Science and Technology Beijing，Beijing 100083，China

A R T I C L EI N F O

Article history：

Accepted 14 September 2015

Available online 28 November 2015

Maxwell fluid

Boundary layer

Fractional derivatives

Unsteady stretching surface

Fractional boundary layer flow of Maxwell fluid on an unsteady stretching surface was investigated. Time-space dependent fractional derivatives are introduced into the constitutive equations of the fluid. We developed and solved the governing equations using explicit finite difference method and the L1-algorithm as well as shifted Grünwald-Letnikov formula.The effects of fractional parameters，relaxation parameter，Reynolds number，and unsteadiness parameter on the velocity behavior and characteristics of boundary layer thickness and skin friction were analyzed.Results obtained indicate that the behavior of boundary layer of viscoelastic fluid strongly depends on time-space fractional parameters.Increases of time fractional derivative parameter and relaxation parameter both cause a decrease of velocity while boundary layer thickness increase，but the space fractional derivative parameter and fractional Reynolds number have the opposite effects.

©2015 The Authors.Published by Elsevier Ltd on behalf of The Chinese Society of Theoretical and Applied Mechanics.This is an open access article under the CC BY-NC-ND license（http：// creativecommons.org/licenses/by-nc-nd/4.0/）.

Much attention has been paid to the study of boundary layer flow induced by continuously stretching sheets submerged in a quiescent or moving fluid due to its important applications in industries（e.g.，copper wire's drawing，annealing，and thinning，aerodynamic extrusion of plastic sheets and fibers，paper production，crystal growing，and glass blowing）.In magnetic field and thermal radiation field，the dissipative boundary layer flow on a nonlinearly stretching sheet was studied by Kumbhakar et al.［1］. With convective boundary condition，the three dimensional radiative flow of Maxwell fluid over an inclined stretching surface was investigated by Ashraf et al.［2］.In a constantly applied magnetic field，the steady mixed convection stagnation point flow of an incompressible Oldroyd-B fluid over the stretching sheet was analyzed by Sajid et al.［3］.Likewise，the problems of unsteady boundary layer were studied widely.Analyses of the unsteady magnetohydrodynamic（MHD）boundary layer flow and heat transfer of an incompressible rotating viscous fluid over a continuouslystretchingsheetwereperformedbyAbbasetal.［4］.Anumerical analysis of the structure of an unsteady boundary layer flow and heat transfer of a dusty fluid over an exponentially stretching sheet subjected to suction was done by Pavithra et al.［5］.The effects of a chemical reaction on an unsteady flow of a micropolar fluid over a stretching sheet embedded in a non-Darcian porous medium were studied by Srinivas et al.［6］.

The viscoelastic materials have the properties of both viscosity andelasticity.ScottBlair［7］proposedafractionalviscoelasticfluid constitutive model using the relation

whereνis a constant，τ（t）is the stress，σ（t）stands for the strain rate，andαis a constant ranging from 0 to 1.

Traditional researches on viscoelastic fluid were carried on in the cases with the governing equations being linear.Caputo and Mainardi［8，9］have shown that results obtained in their analysis were in good agreement with experimental results when fractional derivative is used to describe the viscoelastic materials.El-Shahed et al.［10］obtained exact analytic solutions of a few cases in Navier-Stokes equations with time fractional derivative.By applying the He's homotopy perturbation method（HPM）and variational iteration method（VIM），Khan et al.［11］studied the Navier-Stokes equations with fractional orders.Since viscoelastic fluid shows properties of both elasticity and viscosity，many fractional models have been proposed to characterize the constitutive relationship between viscous stress and the strain rate for viscoelastic materials.MHD flow of an incompressible generalized Oldroyd-B fluid caused by an accelerating plate was studied by Zheng et al.［12］，and they obtained the exact solutions for velocity and shear stress in terms of Fox H-function.A number of the recent works can be also found in Refs.［13-19］.

However，the authors of Refs.［13-19］have ignored the nonlinear term of convection and have dealt with special simple cases where the governing equations are linear.Solutions were obtainedwiththehelpofLaplacetransform，FourierSinetransform and finite Hankel transform.To our knowledge，no report has been made for fractional viscoelastic fluid boundary layer flow with non-linear term of convection considered.

In this paper，the governing equations of fractional viscoelastic fluid induced by an unsteady stretching surface are developed and solvedcoupledwiththeunsteadyboundaryusingtheexplicitfinite difference and L1-algorithm as well as shifted Grünwald-Letnikov formula（approximations for fractional derivatives）.The effects of involved parameters on velocity field，boundary layer thickness，and skin friction are then analyzed and discussed.

Considered an unsteady boundary layer flow of the Maxwell fluid over an unsteady stretched sheet，which can be depicted by the time-space dependent fractional derivatives，the shear stress can be expressed in the following form

By ignoring the pressure gradient，thegoverning equations take the following forms

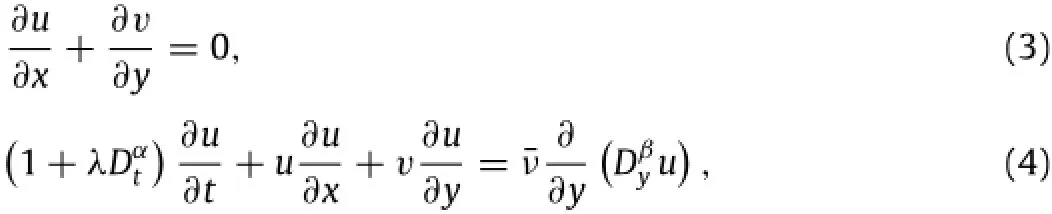
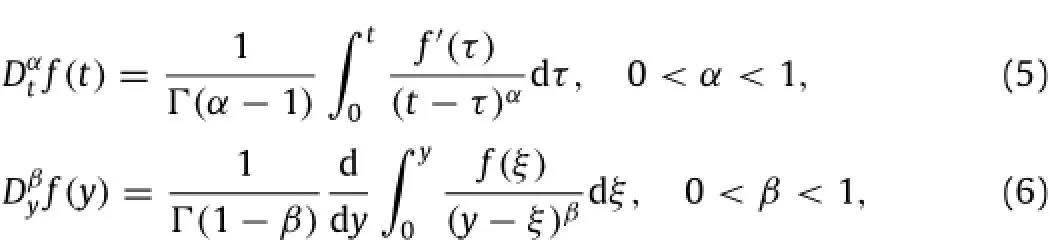
whereΓ（·）is the Gamma function，u andvstand for the horizontal velocity and vertical velocity respectively，¯ν=¯µ/ρis the fractional kinematics viscosity of the fluid（in m1+β/s），¯µis the fractional viscosity coefficient（in kg/m2-β/s），ρis the constant density of the fluid（in kg/m3），andλis the fractional relaxation time（in 1/sα）.

It is assumed that the fluids are static on the plate at first，suddenly the sheet achieves a horizontal velocity Uwalong the xaxis.The shear stress results in the movement of the fluids.The governing equations are given by Eqs.（3）and（4）and satisfy the boundary conditions

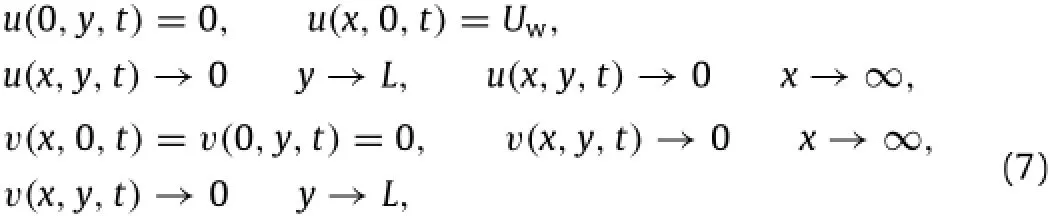
where the unsteady stretching velocity Uwis horizontal and depend on time and space.It is assumed to be

Applying the following non-dimensional quantities

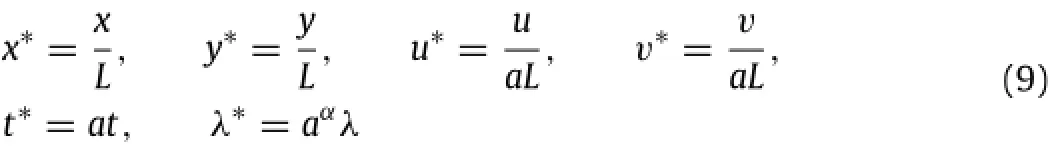
and ignoring the dimensionless mark‘*''for brevity，we can derive the dimensionless motion equations as

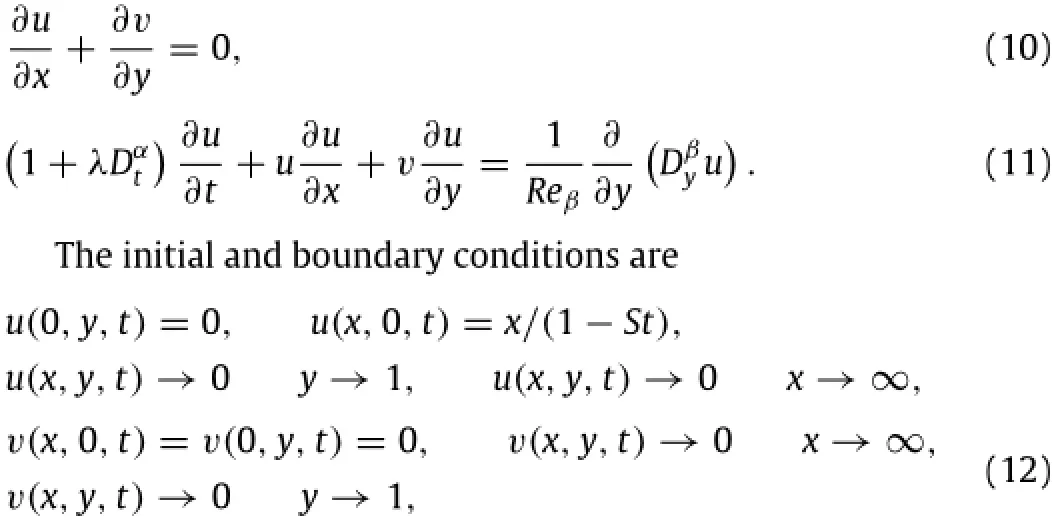
whereS=b/a is the unsteadinessparameter，andis thegeneralfractional Reynoldsnumber.

We first discretize space and time into grid points and time instants，letting xi=ihx（i=0，1，2，···），yj=jhy（j=0，1，2，···），and tn=kτ（k=0，1，2，···），where hx，hyandτare the spatial and temporal steps respectively.

Adopting the L1-algorithm［21］into the unsteady term，we can obtain

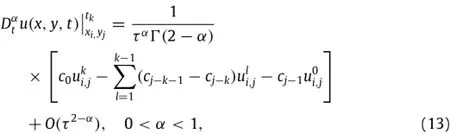
where the diffusion term is approximated using the shifted Grünwald-Letnikov formula［22］

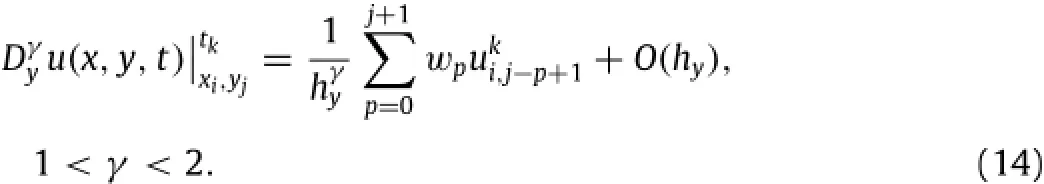
Here the coefficients are defined as

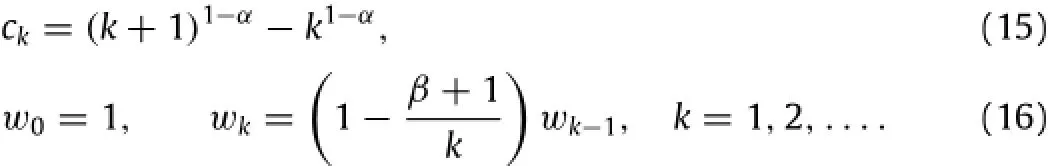
Introducing the Euler backward difference scheme into the first-order time derivative，we have

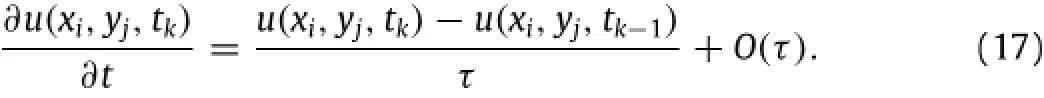
The explicit finite difference approximations for Eqs.（10）and（11）are

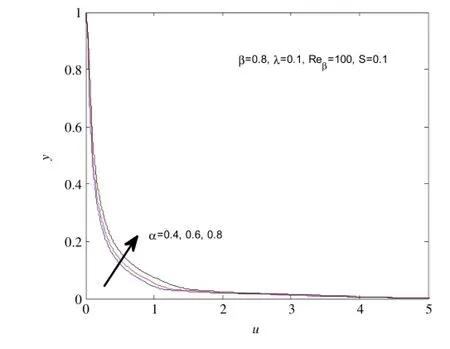
Fig.1.Horizontal velocity profiles for different values of time fractional parameterα.

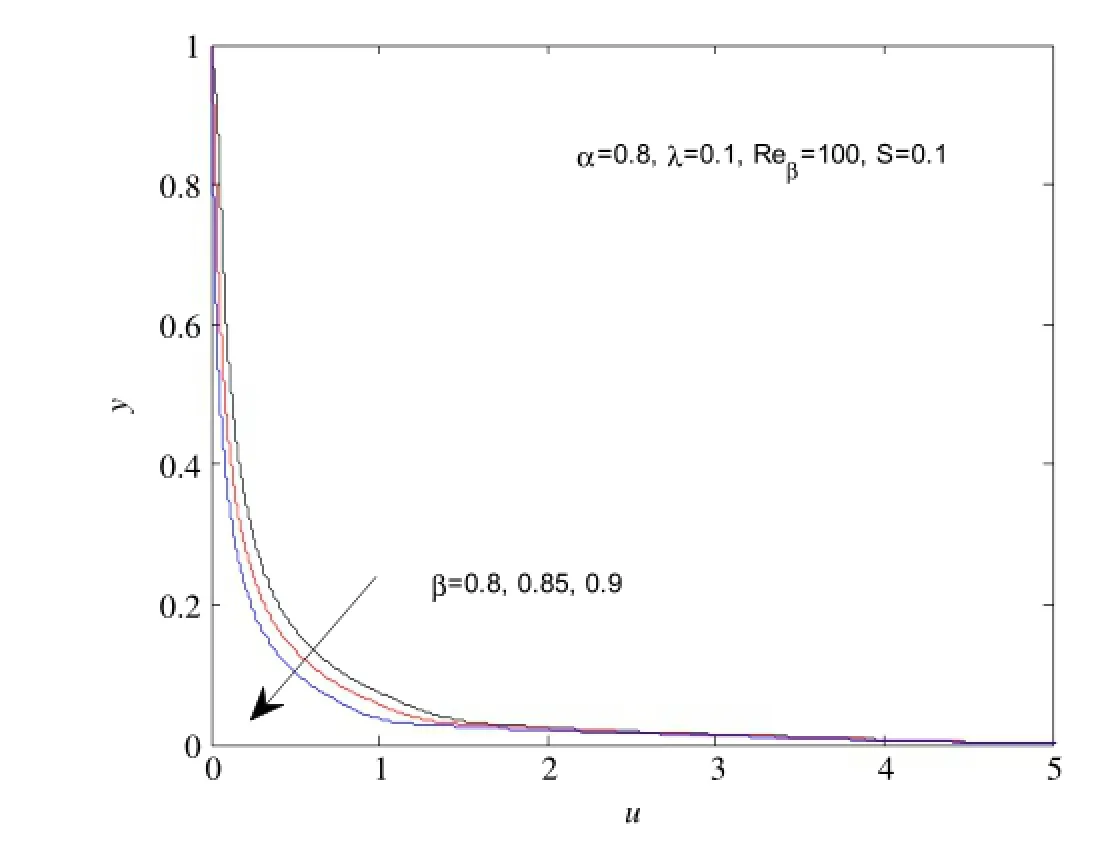
Fig.2.Horizontal velocity profiles for different values of space fractional parameterβ.

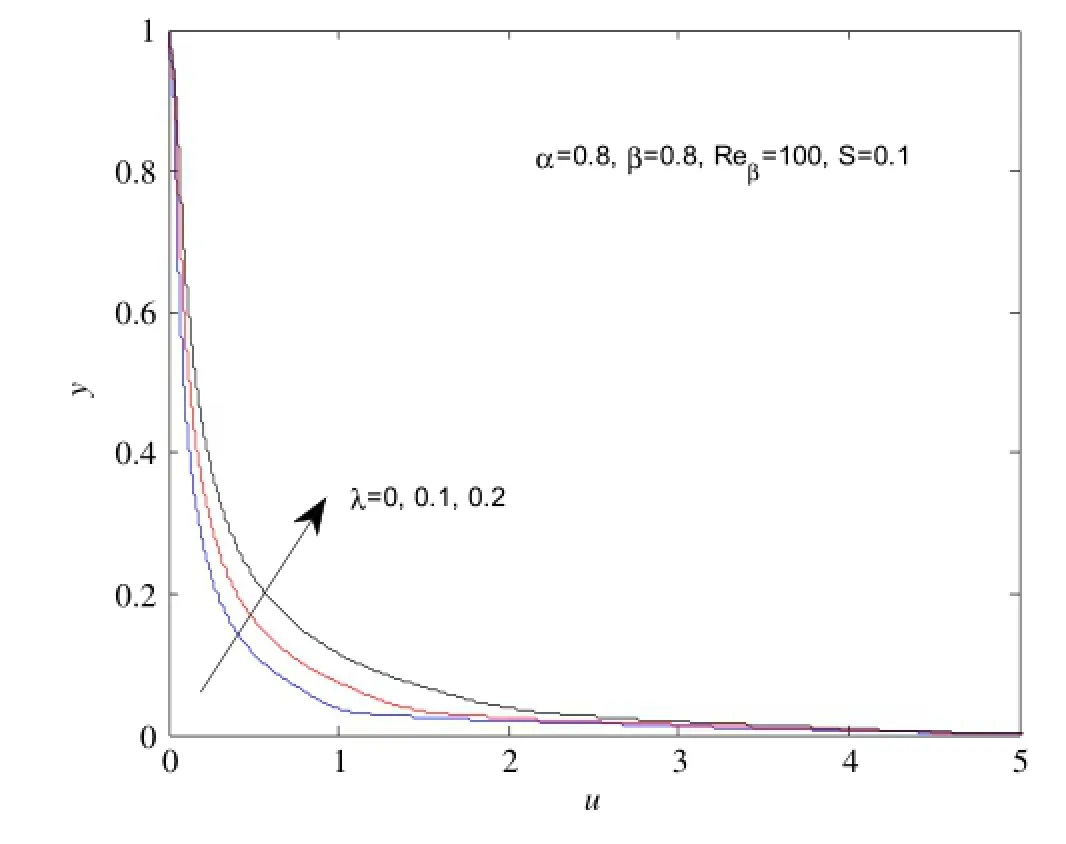
Fig.3.Horizontal velocity profiles for different values of relaxation parameterλ.

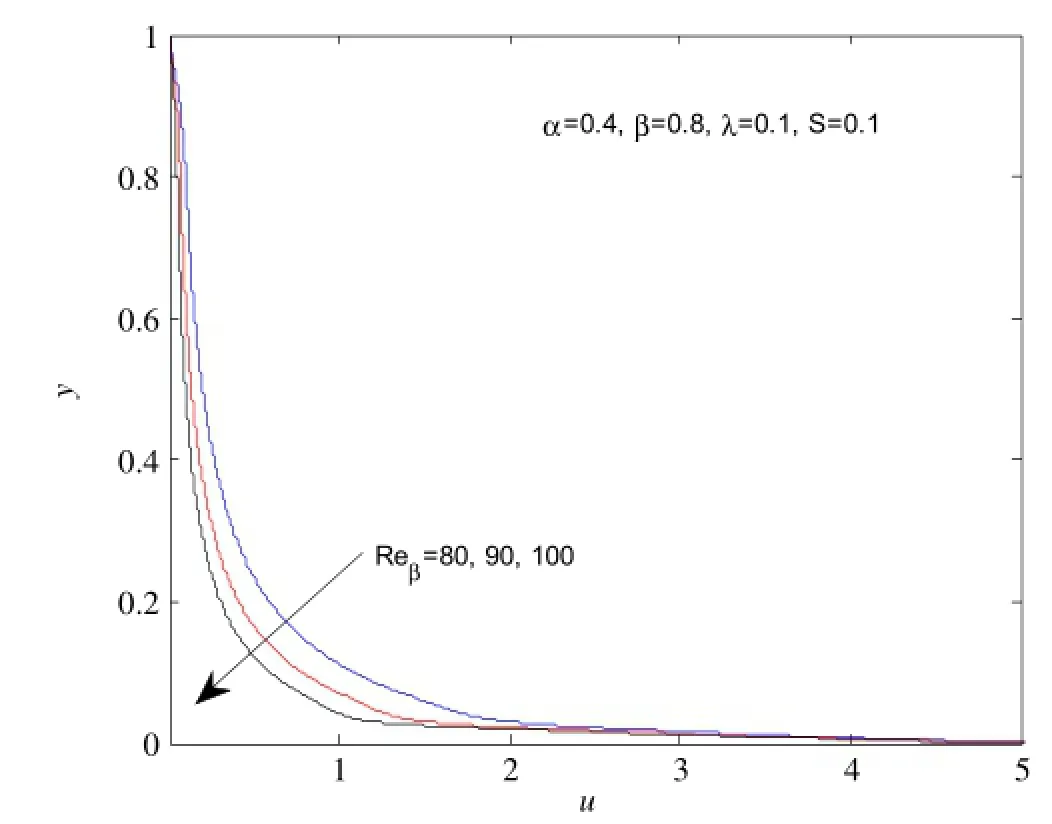
Fig.4.Horizontal velocity profiles for different values of Reynolds number Reβ.

The dimensionless fractional boundary layer equations（10）and（11），coupled with boundary condition（12），are solved by employing the finite difference method and L1-algorithm with shifted Grünwald-Letnikov approximations for time and space fractional derivatives.The boundary layer behavior as well as the effectsofinvolvedparametersonthevelocityfieldandskinfriction are analyzed.Moreover，we should notice that the flow of fluid over an unsteady stretching surface is different from the flow of classical boundary layer.In our case，the values of shear stress and skin friction are negative.

The numerical solutions of fluid velocity u are depicted graphically as functions of boundary layer coordinate y in Figs.1-4 fordifferentfractionalparameters（α，β），relaxationparameter（λ），generalized fractional Reynolds number（Reβ），and unsteadiness parameter（S）.Figure 1 depicts the horizontal velocity profiles for different values ofα.It can be seen from Fig.1 that the horizontal velocity decreases when time fractional parameterα increases，while its decrease causes the increase of the boundary layer thickness.Figure 2 displays an opposite behavior for the velocity field，the increase ofβyields an opposite behavior of horizontal velocity profiles and the boundary layer thickness.

Figure 3 shows the effects of relaxation parameterλon horizontal velocity distribution.It can be shown from Fig.3 that the horizontal velocity decreases and boundary layer thickness increases with the increase of relaxation parameterλ，which can be used to describe the delaying characteristic of viscoelastic fluid. The time required for recovering to normal state increases with the increase ofλ，which causes the increase of boundary layer thickness.Figure 4 depicts the effects of the generalized fractional Reynolds number Reβon horizontal velocity profiles.Obtained results indicate that the horizontal velocity increases（on the contrary，boundary layer thickness decreases）with the increase of generalized fractional Reynolds number Reβ.

Figures 5 and 6 show the influences of unsteadiness parameter（S）on velocity field and skin friction respectively.Velocity profiles at different S are plotted in Fig.5.It is observed that the horizontal velocity increases（on the contrary，the boundary layer thickness decreases）when unsteadiness parameter S increases. This is because the value of S increases with Uwwhen other parameters are all fixed，and it causes the decrease of boundary layer thickness.Figures 6 and 7 indicate that the increasing of unsteadiness parameter S results in the increase of both absolute values of shear stress and skin friction.The absolute value of skin friction monotonously decreases with the increasing of fractional Reynolds number Reβ.

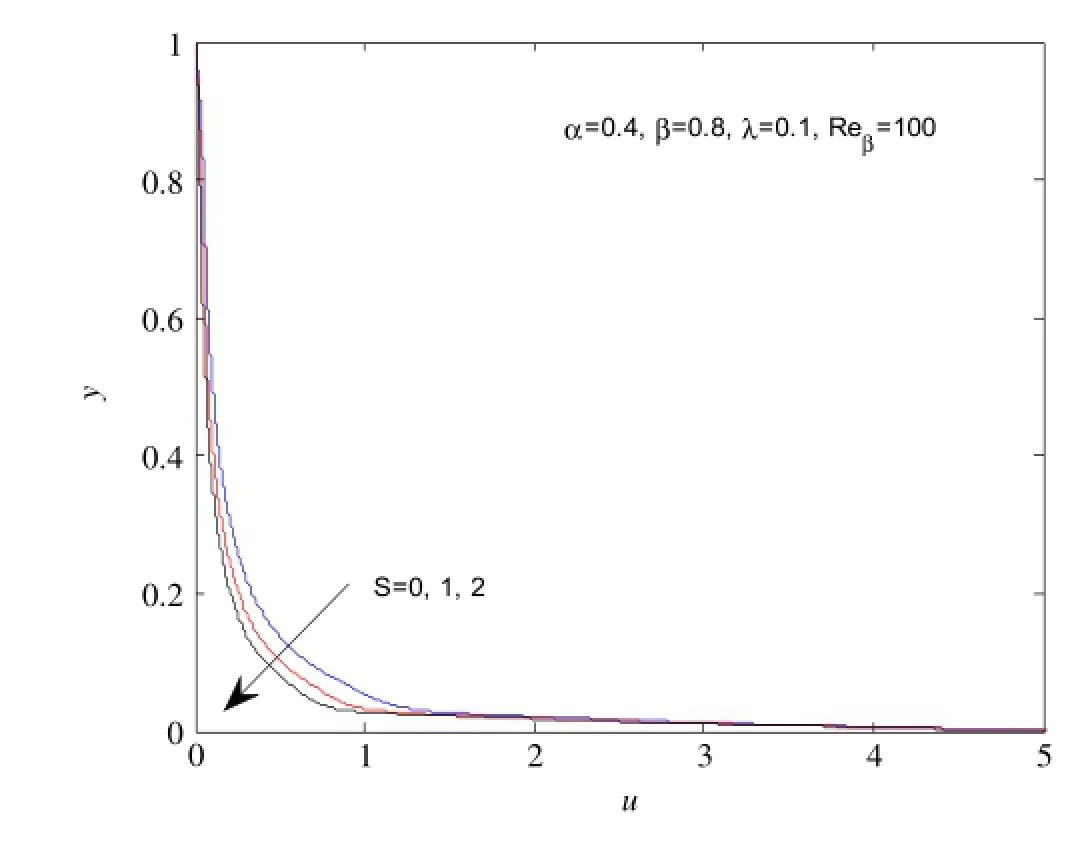
Fig.5.HorizontalvelocityprofilesfordifferentvaluesofunsteadinessparameterS.

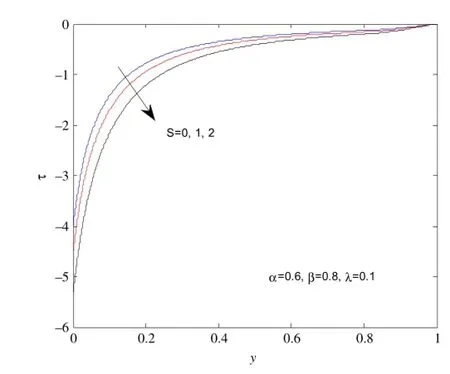
Fig.6.Shear stress for different values of unsteadiness parameter S.

Fig.7.Skin friction profiles for different values of unsteadiness parameter S.

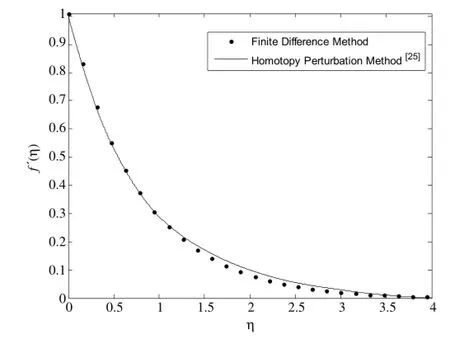
Fig.8.Comparison of velocity profile for classical boundary layer equations（S= 1·2，Re=100）.

Lettingλ=0，α=1，andβ=1 in Eqs.（3）and（4），the current problem reduces to the classical unsteady boundary layer problem as following

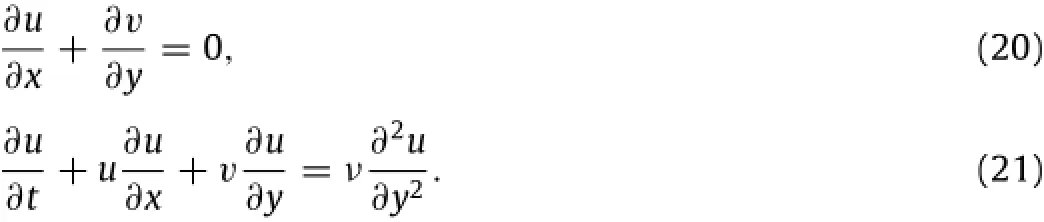
According to Ref.［23］，we have

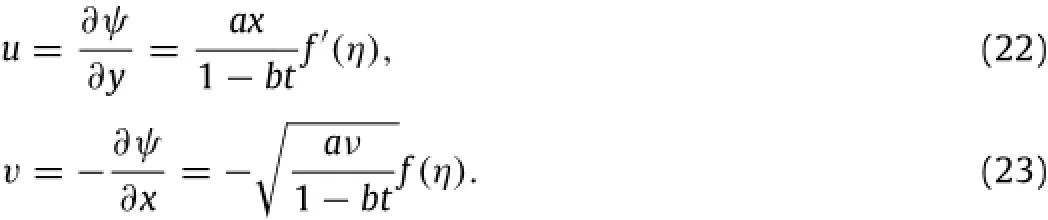
The similarity transformation for a stretching flow is given by

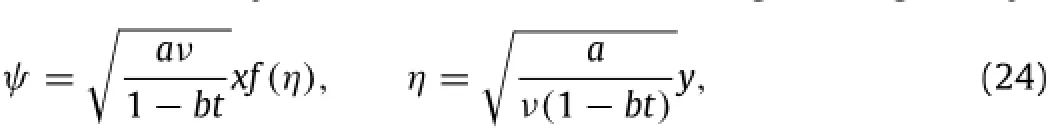
whereψis the stream function which automatically assures the mass conservation in Eq.（3）.The momentum equation can be reduced to

The boundary conditions（7）can be written as

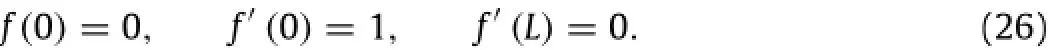
Figure 8 presents the comparison of numerical solution obtained in this paper and analytical result obtained in Ref.［24］. It shows that the obtained numerical result is in good agreement with the analytical result.The reliability and efficiency of the numerical solutions are verified with the comparison.

This paper investigates boundary layer flow of fractional viscoelastic fluid over a stretching surface.The time-space dependentfractionalderivativesareintroducedfirstlyinboundary layer governing equations.Numerical solutions are obtained in explicit expressions with finite difference approximation.The effects of fractional parameters，relaxation parameter，Reynolds number，and unsteadiness parameter on velocity field and skin frictionareanalyzed.Validityoftheproposedmethodisconfirmed by the comparison of obtained numerical result and analytical result.Obtained results indicate that the boundary layer transport behavior of viscoelastic fluid strongly depends on time-space fractional parameters，which construct the basic time and space framework system for the boundary layers transport.

Acknowledgment

The work was supported by the National Natural Science Foundation of China（51476191 and 51406008）.

［1］B.Kumbhakar，P.Sriniivasa Rao，Dissipative boundary layer flow over a nonlinearly stretching sheet in the presence of magnetic field and thermal radiation，Proc.Natl.Acad.Sci.85（2015）117-125.

［2］M.BilalAshraf，T.Hayat，S.A.Shehzad，etal.，Mixedconvectionradiativeflowof three dimensional Maxwell fluid over an inclined stretching sheet in presence of thermophoresis and convective condition，AIP Adv.5（2015）027134.

［3］M.Sajid，B.Ahmed，Z.Abbas，Steadymixedconvectionstagnationpointflowof MHD Oldroyd-B fluid over a stretching sheet，J.Egyptian Math.Soc.23（2015）440-444.

［4］Z.Abbas，T.Javed，M.Sajid，et al.，Unsteady MHD flow and heat transfer on a stretching sheet in a rotating fluid，J.Taiwan Inst.Chem.Eng.41（2010）644-650.

［5］G.M.Pavithra，B.J.Gireesha，Unsteady flow and heat transfer of a fluid-particle suspension over an exponentially stretching sheet，Ain Shams Eng.J.5（2014）613-624.

［6］S.Srinivas，P.B.A.Reddy，B.S.R.V.Prasad，Non-Darcian unsteady flow of a micropolar fluid over a porous stretching sheet with thermal radiation and chemical reaction，Heat Transfer-Asian Res.44（2015）172-187.

［7］G.W.Scott Blair，The role of psychophysics in rheology，J.Colloid Sci.2（1947）21-32.

［8］M.Caputo，F.Mainardi，A new dissipation model based on memory mechanism，Pure Appl.Geophys.91（1971）134-147.

［9］M.Caputo，Vibrations on an infinite viscoelastic layer with a dissipative memory，J.Acoust.Soc.Am.56（1974）897-904.

［10］M.El-Shahed，A.Salem，On the generalized Navier-Stokes equations，Appl. Math.Comput.156（2004）287-293.

［11］N.A.Khan，A.Ara，S.A.Ali，et al.，Analytical study of Navier-Stokes equation with fractional orders using He's homotopy perturbation and variational iteration methods，Int.J.Nonlinear Sci.Numer.10（2009）1127-1134.

［12］L.C.Zheng，Y.Q.Liu，X.X.Zhang，Slip effects on MHD flow of a generalized Oldroyd-B fluid with fractional derivative，Nonlinear Anal.Real World Appl. 13（2012）513-523.

［13］M.Nazar，M.Zulqarnain，M.Saeed Akram，et al.，Flow through an oscillating rectangular duct for generalized Maxwell fluid with fractional derivatives，Commun.Nonlinear Sci.Numer.Simul.17（2012）3219-3234.

［14］M.Jamil，A.A.Zafar，A.Rauf，et al.，Some new exact analytical solutions for helical flows of second grade fluids，Commun.Nonlinear Sci.Numer.Simul. 17（2012）141-153.

［15］L.C.Zheng，C.R.Li，X.X.Zhang，et al.，Exact solutions for the unsteady rotating flows of a generalized Maxwell fluid with oscillating pressure gradient between coaxial cylinders，Comput.Math.Appl.62（2011）1105-1115.

［16］L.C.Zheng，Z.L.Guo，X.X.Zhang，3Dflow of ageneralizedOldroyd-Binducedby a constant pressure gradient between two side walls perpendicular to a plate，Nonlinear Anal.RWA 12（2011）3499-3508.

［17］C.Fetecau，Corina Fetecau，M.Jamil，et al.，Flow of fractional Maxwell fluid between coaxial cylinders，Arch.Appl.Mech.81（2011）1153-1163.

［18］A.Chatterjee，Heat transfer enhancement in laminar impinging flows with a non-Newtonian inelastic fluid，J.Non-Newton.Fluid.211（2014）50-61.

［19］Magdy A.Ezzat，Thermoelectric MHD non-Newtonian fluid with fractional derivative heat transfer，Physica B 405（2010）4188-4194.

［20］I.Podlubny，FractionalDifferentialEquations，AcademicPress，NewYork，1999.

［21］K.B.Oldham，J.Spanier，The Fractional Calculus，Academic Press，New York，1974.

［22］F.Liu，P.Zhuang，V.Anh，et al.，Stability and convergence of the difference methods for the space-time fractional advection-diffusion equation，Appl. Math.Comput.191（2007）12-20.

［23］X.H.Su，L.C.Zheng，X.X.Zhang，DTM-BF method and dual solutions for unsteady MHD flow over permeable shrinking sheet with velocity slip，Appl. Math.Mech.（English Ed.）33（2012）1555-1568.

［24］L.C.Zheng，C.X.Chen，X.X.Zhang，et al.，Analyzing the flow and heat transfer of a power law fluid over an unsteadily stretched surface using a modified homotopy perturbation method，Int.J.Nonlinear Sci.Numer.11（2010）843-849.

25 August 2015

.

E-mail address：liancunzheng@ustb.edu.cn（L.Zheng）.

http：//dx.doi.org/10.1016/j.taml.2015.11.005

2095-0349/©2015 The Authors.Published by Elsevier Ltd on behalf of The Chinese Society of Theoretical and Applied Mechanics.This is an open access article under the CC BY-NC-ND license（http：//creativecommons.org/licenses/by-nc-nd/4.0/）.

*This article belongs to the Fluid Mechanics