转动弹性约束浅拱的2:1内共振非线性模态

曾有艺，易壮鹏，颜东煌



转动弹性约束浅拱的2:1内共振非线性模态

曾有艺，易壮鹏，颜东煌

(长沙理工大学土木与建筑学院，湖南长沙，410114)

采用多尺度法进行直接摄动构造一端转动弹性约束浅拱发生2:1内共振时的非线性模态。在线性频率/模态及形函数中考虑弹性约束边界的影响，分析约束刚度不同时浅拱非线性模态的频率/幅值及时空效应。研究结果表明：转动弹性约束的存在使得频谱上出现模态转向, 模态与形函数不对称；内共振非线性系统存在单模态运动和耦合模态运动；转动弹性约束的存在导致最低2阶模态之间的2:1内共振被激发;非线性模态的频率/幅值具有显著的非线性效应，且不同约束刚度时动力形态呈现不同的时空效应。

转动弹性约束；浅拱；非线性模态；2:1内共振；多尺度法

拱结构[1−3]受力性能良好, 在工程领域应用广泛, 研究人员对各种外激励下的运动稳定性和分岔行为进行了系统研究。在土木工程中，在动力荷载作用下，现代大跨度拱桥或拱形建筑由于基础、土体的共同作用, 整个外部边界对拱结构具有弹性约束作用，基础变形及与相邻结构共同作用引起的附加惯性力将影响精确的动力分析；另一方面, 大跨度系杆拱桥拱脚由于系杆和基础的作用，边界条件考虑为弹性约束更加合理；在机械和航天航空工程中，边界条件对大型复杂结构中弹性约束机械拱臂、曲梁等子结构的动力特性起决定性影响，因而，弹性约束拱的理论研究模型具有广泛的工程背景。基于此，Xu等[4]研究了径向弹性约束拱的动力跳跃特性；Lacarbonana等[5]分析了一端转动弹性约束曲梁的1:1内共振；康婷等[6]研究了水平弹性支撑圆拱的动力特性。非线性模态(NNMs)的概念由Rosenberg[7]提出, 其定义与构造[8−15]是非线性振动研究中的基本问题。内共振系统的耦合非线性模态具有分岔行为，且随着系统参数发生变化，导致模态数目超过系统的自由度数，具有显著的非线性特性, 引起很多研究者的关注。如Nayfeh等[16]采用多尺度法研究了两端固支屈曲梁在发生1:1和3:1内共振条件下的非线性模态；Li等[17]构造了一类3对纯虚根1:2:5双重内共振系统的非线性模态并分析了其分岔特性；Mamandi等[18]研究了非线性弹性地基上轴向压缩简支梁3:1内共振条件下的非线性模态。在此，本文作者针对一端转动弹性约束浅拱, 在线性模态/频率、形函数中考虑约束, 通过多尺度法[9]摄动分析并构造2:1内共振的非线性模态, 分析非线性模态的频率/幅值及时空效应。

1 动力学模型

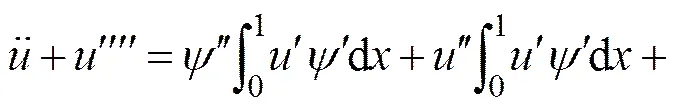
其中：上标“.”和“′”分别表示对和微分；；；；；；为弹性模量；为密度；和分别为惯性矩、面积；为转动半径。边界条件为

=0:=0，；=1:=0，(2)

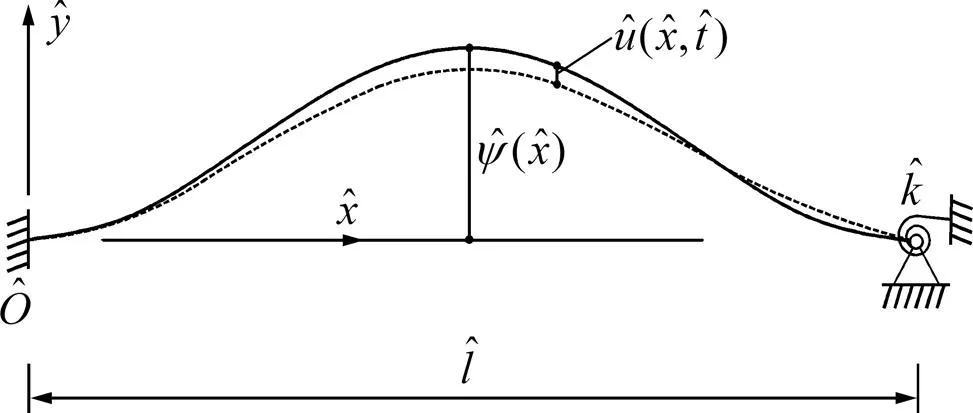
图1 一端转动弹性约束浅拱结构示意图

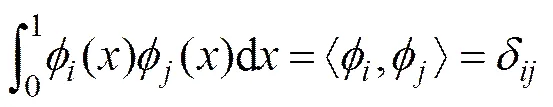
其中：为Kronecker delta。和可由如下特征方程求解：

2 摄动分析解与非线性模态

采用多尺度法近似设定求解, 式(1)的二阶近似解可以表示为

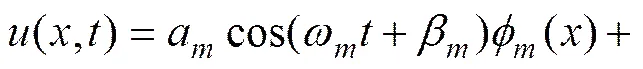
幅值a和a以及频率β和β为下述模态方程的解：

2和3为二次、三次非线性微分算子,且，，是为进一步化简的微分算子。形函数为满足下列边界方程的解：

对于第2种形式的耦合模态响应，由式(9)可得

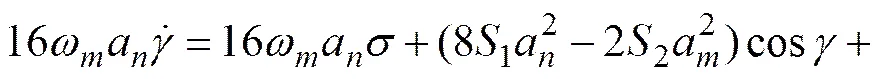
耦合模态响应解的稳定性可由式(9)和式(12)组成的Jacobian矩阵的特征值予以判断。由式(9)有，因此，(=0，1，2，…)。式(12)中，此时，非线性模态可表示为

3 数值分析

取不同值时，采用数值方法计算线性模态和频率, 并以最低2阶模态(=1，=2)之间的2:1内共振为例分析内共振解及非线性模态的时空效应。

3.1 线性模态与频率

图2所示为转动弹性约束浅拱自振特性的分布规律，其中，图2(a)所示为=100时前5阶频率随矢高的变化，其实部和虚线分别表示奇、偶数阶频率。从图2(a)可知：当在(0,20]内单调增加时, 不同阶次模态频率的2:1整数比出现在一些与矢高相关的位置，如1(1~2阶)、2(2~3阶)和3(3~5阶), 在这些位置相关频率之间可能发生2:1内共振；另一方面, 不同阶次频率(1~2阶，3~4阶)在相互接近时发生模态转向。不同转动约束刚度下1~2阶模态之间的转向如图2(b)所示, 结果表明转向模态之间的间隙随着的增大变得越来越小，最终在时变为模态交叉, 这也是转动弹性约束拱与一般固结拱的典型差异之一。此外, 转动弹性约束拱的各阶模态由于边界不对称在跨度范围内出现不对称, 尤其弹性约束端的转角呈现明显的非线性分布。从图2(c)可知：各阶频率的约束端转角随的增大而减小，最终归于0。这些模态的不对称性将导致后续平均方程系数、非线性模态不一致以及内共振的条件出现变化。

(a) 频率分布；(b) 模态转向；(c) 模态弹性约束端转角

3.2 内共振解

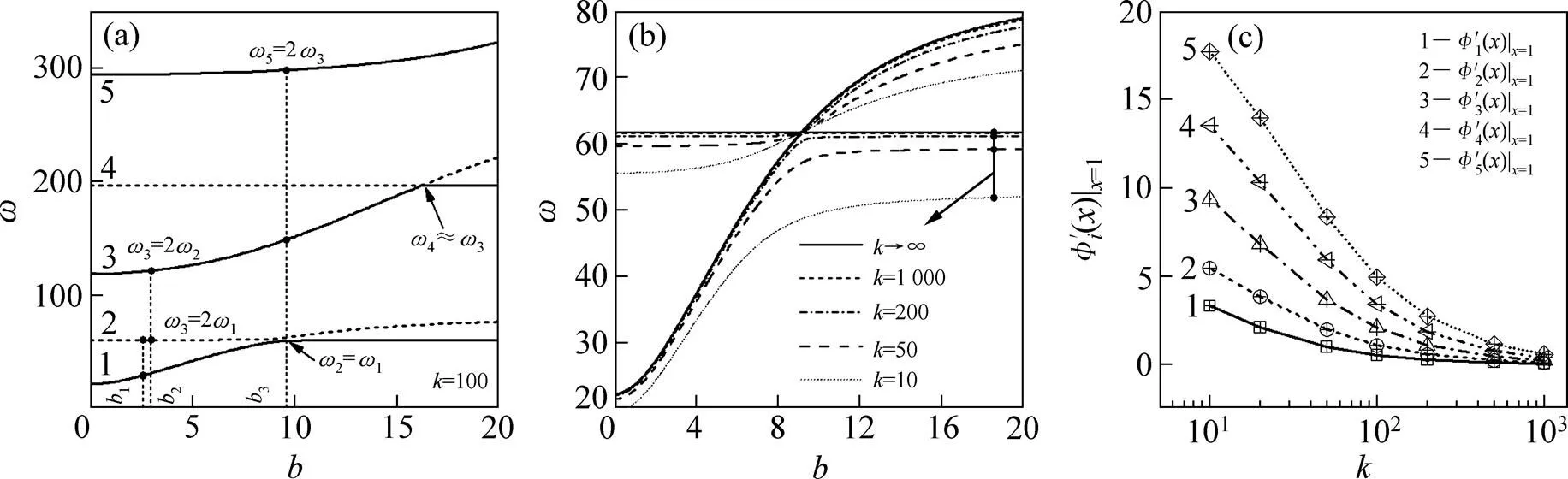
图3 b=b1和k=100时形函数(x=1~6)

图4所示为=1时模态平均方程(9)中系数随的变化规律。由图4(a)可知：1随着的增大而减小,时为0, 即转动弹性约束时不等于0, 刚性约束时等于0。实际上，这是最低两阶模态之间的2:1内共振是否被激发的判定条件: 转动弹性约束时两模态间的2:1内共振被激发; 刚性约束()时, 虽然2阶频率的比值为2:1, 但是不会发生内共振(1=0所致)。这也是浅拱由于转动弹性约束所致动力特性的显著不同之处。二阶非线性系数S与和阶频率、模态及拱轴线形有关。图4(b)表明：这些系数在大于一定值时均趋于稳定, 尤其是当＞1 000时系数变化率很小，与(固结浅拱)非常接近。此外，S在随的变化过程中有1个奇点, 位于内, 在此处S从奇点的左(右)边趋于()，且此奇点刚好发生最低阶3阶模态之间的1:2:4双重内共振，这也进一步表明对浅拱的非线性行为有显著影响。

图4 2:1内共振模态方程系数

图5所示为最低2阶模态之间发生2:1内共振时非线性模态的幅值和频率分布，其中，图5(a)所示为取不同值时单模态解的关系曲线。由式(11)中右边大括号内的项等于0得到每个对应曲线的上支和下支，由单模态解的特征值判断可知2支曲线之间的部分为内共振解的不稳定区, 2支曲线以外的部分则为稳定区。另外,=1 000时上、下支曲线围成的不稳定区域很小, 与时不稳定区域为0、曲线上、下支重合接近, 这与2种情况下非线性系数接近有关。

(a) 单模态；(b)，(c) 耦合模态

图5(b)和图5(c)所示分别为取50，100和1 000时耦合模态解中和关系曲线, 由于, 图5(c)仅给出了与通过线性频率标准化之后的的关系曲线。2阶模态发生内共振的相互作用时, 非线性系数由分别负责软、硬弹簧性质的二、三阶效应2部分组成。从图5(b)可见：对于某一给定的，随着的增大而增大, 且= 1 000时，曲线与时的曲线接近。而从图5(c)可知：取不同值时曲线之间的差异较小, 均呈现软弹簧性质,这是由于在的标准化表达式中, 对于给定的，和在不同时均使得呈软化特性。

3.3 非线性模态的时空效应

对非线性模态的时空效应进行数值描述。图6所示为取50，100和1 000这3种情况下时浅拱在1个周期内的形状变化示意图，其中，为周期。从图6可以看到：变形呈现周期特性和不对称性，动力形态的幅值随着的增大而增大，第1阶模态以及形函数和在动力形态中占据主导地位。这是由于在位置，2:1内共振在第1和第2阶模态之间被激发，低阶模态在模态相互作用过程中占主导。图7所示为不同时下跨中竖向位移、弹性约束端转角位移的时间历程曲线。通过对比可发现：越大时，跨中竖向位移的幅值越大(图7(a))，这与图6所得结论一致。=50和=100时弹性约束端的转角位移(图7(b))较大且较接近；而=1 000时转角位移较小，与时为0接近。这由2种情况下的非线性系数较接近所致, 再一次说明了对转动弹性约束浅拱的重要性。

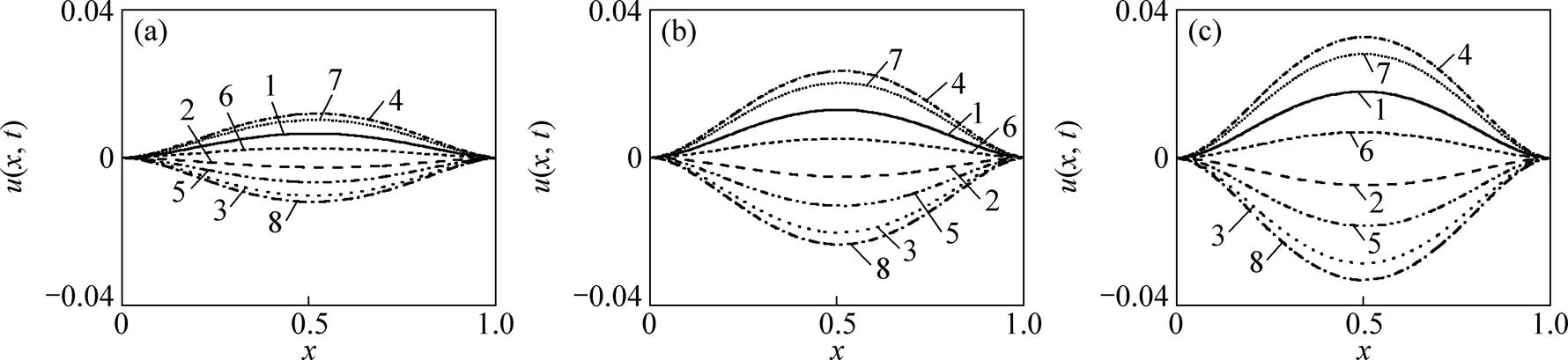
k：(a) 50；(b) 100；(c) 1 000

(a) 跨中竖向位移；(b) 弹性约束端转角

4 结论

1) 转动弹性约束导致线性模态、形函数不对称且模态约束端转角不为0，频谱上相邻阶次的模态以转向的形式靠近。

2) 转动弹性约束使得最低两阶模态之间的2:1内共振被激发，在相同条件下固结边界浅拱未被激发。

3) 在不同转动约束刚度下，非线性模态的时空效应具有不同的动力形态，呈现显著的非线性效应。

[1] ZHOU Liangqiang, CHEN Yushu, CHEN Fangqi. Global bifurcation analysis and chaos of an arch structure with parametric and forced excitation[J]. Mechanics Research Communications, 2010, 37(1): 67−71.

[2] TANG Zhanzhan, XIE Xu, WANG Tong, et al. Study on FE models in elasto-plastic seismic performance evaluation of steel arch bridge[J]. Journal of Constructional Steel Research, 2015, 113: 209−220.

[3] 杨洋, 童根树. 水平弹性支承圆弧钢拱的平面内极限承载力研究[J]. 工程力学, 2012, 29(3): 45−54. YANG Yang, TONG Genshu. Study for in-plane ultimate strength of steel circular arches with horizontal spring supports[J]. Engineering Mechanics, 2012, 29(3): 45−54.

[4] XU Jiangxue, HUANG Hong, ZHANG Peizhen, et al. Dynamic stability of shallow arch with elastic supports: Application in the dynamic stability analysis of inner winding of transformer during short circuit[J]. International Journal of Non-linear Mechanics, 2002, 37(4/5): 909−920.

[5] Lacarbonara W, Arafat H N, Nayfeh A H. Non-linear interactions in imperfect beams at veering[J]. International Journal of Non-linear Mechanics, 2005, 40(7): 987−1003.

[6] 康婷, 白应生, 孙惠香. 水平弹性支撑圆拱的动力特性研究[J]. 力学与实践, 2013, 35(2): 50−55.KANG Ting, BAI Yingsheng, SUN Huixiang. Dynamic characteristics of the horizontal elastic support circular arch[J]. Mechanics in Engineering, 2013, 35(2): 50−55.

[7] Rosenberg R M. The normal modes of nonlinear-degree-of-freedom systems[J]. Journal of Applied Mechanics, 1962, 29(1): 7−14.

[8] Shaw S W, Peirre C. Nonlinear normal modes and invariant manifolds[J]. Journal of Sound and Vibration, 1991, 150(1): 170−173.

[9] Nayfeh A H, Mook D T. Nonlinear Oscillations[M]. New York: John Wiley & Sons Inc, 1979: 10−30.

[10] Vakakis A F. Nonsimilar normal oscillations in a strongly nonlinear discrete system[J]. Journal of Sound and Vibration, 1992, 158(2): 341−361.

[11] 陈予恕, 吴志强. 非线性模态理论的研究进展[J]. 力学进展, 1997, 27(3): 289−300. CHEN Yushu, WU Zhiqiang. Advances in study on theories of nonlinear normal modes[J]. Advances in Mechanics, 1997, 27(3): 289−300.

[12] Lacarbonara W, Rega G, Nayfeh A H. Resonant non-linear normal modes. Part I: Analytical treatment for structural one-dimenensional systems[J]. International Journal of Non-linear Mechanics, 2003, 38(6): 851−872.

[13] Lacarbonara W, Rega G. Resonant non-linear normal modes. Part II: activation orthogonality conditions for shallow structural systems[J]. International Journal of Non-linear Mechanics, 2003, 38(6): 873−887.

[14] Kuether R J, Allen M S. A numerical approach to directly compute nonlinear normal modes of geometrically nonlinear finite element models[J]. Mechanical Systems and Signal Processing, 2014, 46(1): 1−15.

[15] WANG Fengxia. Bifurcations of nonlinear normal modes via the configuration domain and the time domain shooting methods[J]. Communications in Nonlinear Science and Numerical Simulation, 2015, 20(2): 614−628.

[16] Nayfeh A H, Lacarbonara W, Chin C M. Nonlinear normal modes of buckled beams: three-to- one and one-to-one internal resonances[J]. Nonlinear Dynamics, 1999, 18(3): 253−273.

[17] LI Xinye, CHEN Yushu, WU Zhiqiang. Non-linear normal modes and their bifurcation of a class of systems with three double of pure imaginary roots and dual internal resonances[J]. International Journal of Non-linear Mechanics, 2004, 39(2): 189−199.

[18] Mamandi A, Kargarnovin M H, Farsi S. Dynamic analysis of a simply supported beam resting on a nonlinear elastic foundation under compressive axial load using nonlinear normal modes techniques under three-to-one internal resonance condition[J]. Nonlinear Dynamics, 2012, 70(2): 1147−1172.

Nonlinear normal modes of rotationally elastically constrained shallow arch in case of two-to-one internal resonance

ZENG Youyi, YI Zhuangpeng, YAN Donghuang

(School of Civil Engineering & Architecture, Changsha University of Science & Technology, Changsha 410114, China)

The nonlinear normal modes of a shallow arch with one end rotationally elastically constrained in case of two-to-one internal resonance were constructed by using the multi-scale method and direct perturbation. The effects of elastic constraint boundaries were considered in the linear frequencies, modes and shape functions. Then the nonlinear frequencies/amplitudes and space-time evolutions of nonlinear normal modes in shallow arch with different rotational stiffnesses were investigated. The results show that there are veering between two adjacent modes in frequency spectrum and asymmetries in the modes and shape functions due to the existence of rotational stiffness. The internally resonant nonlinear system has both single-mode and couple-mode motions. Also the two-to-one internal resonance between the two lowest modes is activated because of the elastic constraint stiffness in the boundary. Moreover, the frequencies/amplitudes in modulation equations exhibit remarkable nonlinearities, and the dynamic configuration of the nonlinear normal modes for different rotational constraints display various space-time evolutions.

rotational elastic constraints; shallow arch; nonlinear normal modes; 2:1 internal resonance; multi-scale method

10.11817/j.issn.1672-7207.2015.10.048

U311.2

A

1672−7207(2015)10−3922−06

2015−02−10；

2015−04−07

国家自然科学基金资助项目(11002030, 51178059)；长沙理工大学土木工程重点学科基金资助项目(13ZDXK06, 15ZDXK01)(Projects (11002030, 51178059) supported by the National Natural Science Foundation of China; Projects (13ZDXK06, 15ZDXK01) supported by Key Discipline Funds for Civil Engineering of Changsha University of Science and Technology)

颜东煌，博士，教授，博士生导师，从事桥梁结构施工控制与新技术研究；E-mail：yandonghuang@126.com

(编辑 陈灿华)