一个离散可积族的可积耦合及其H am ilton结构
2015-07-28徐秀丽
徐秀丽
(枣庄科技职业学院高级技工部,山东枣庄 277500)
一个离散可积族的可积耦合及其H am ilton结构
徐秀丽
(枣庄科技职业学院高级技工部,山东枣庄 277500)
由位移算子通过二次型恒等式直接得到离散可积族的耦合及其Hamilton结构.这种方法具有普遍性,可应用于其他离散方程族.
可积耦合;二次型恒等式;Hamilton结构
1 引言
近年来,Lattice可积族成为可积系统理论研究的焦点,备受关注.许多非线性Lattice可积族[1-8]及其哈密顿结构的离散路径已经建立[8],同时很多离散可积族的可积耦合的建立方法已被提到[9-14].然而,如何建立一些离散可积族的哈密顿结构仍是非常重要和有趣的课题.郭福奎教授和张玉峰教授提出了二次型恒等式[15-16],为建立连续可积耦合的Hamilton结构提供了理论依据.由于不存在换位运算,我们无法直接通过二次型恒等式直接得到离散可积耦合的Hamilton结构[15].基于以上问题,我们尽力利用位移算子构建类似于连续可积族的换位运算,从而利用二次型恒等式得到离散可积族的Hamilton结构.
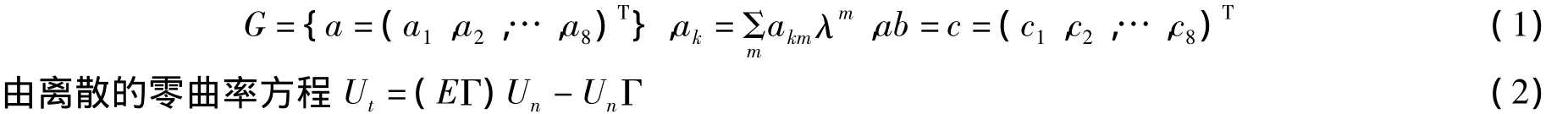
及其静态零曲率方程

若Γ1和ΓV2(3)同阶解满足Γ1=γΓ2,[a,b]T=aTR(b),a,b∈,对称矩阵F=(fij)S×S,要求满足:

由二次型恒等式
构造李代数


其中

易证G满足矩阵乘法具有封闭性[10].a=(a1,a2,…,a8)T,b=(b1,b2,…,b8)T.定义交换算子为
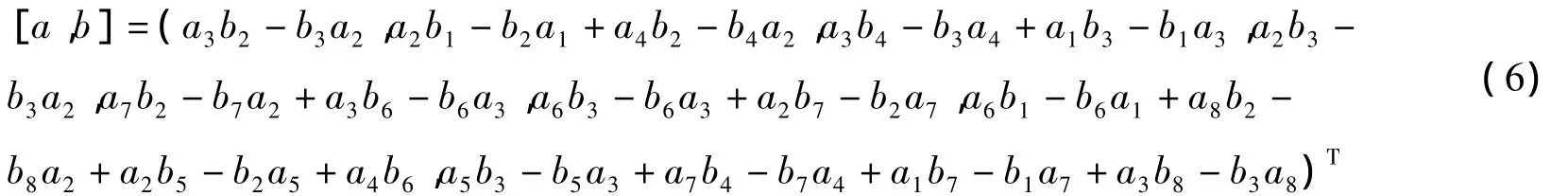
本文通过构造新的loop代数,借助于二次型恒等式得到了离散可积族的可积耦合及其哈密顿结构,这种方法非常新颖,可广泛应用于其他离散可积族.
2 Hamiltonian结构
设计对等谱问题
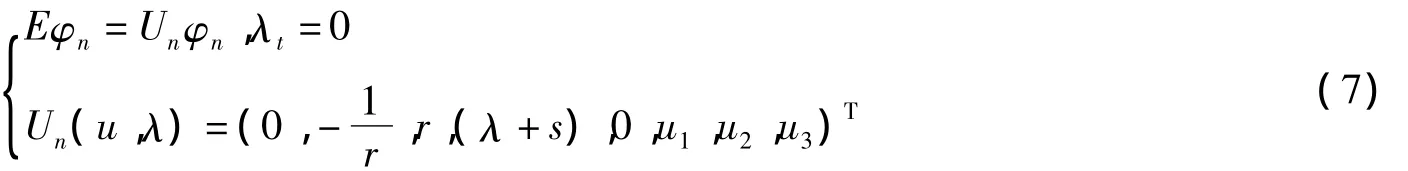

得递推关系

易证
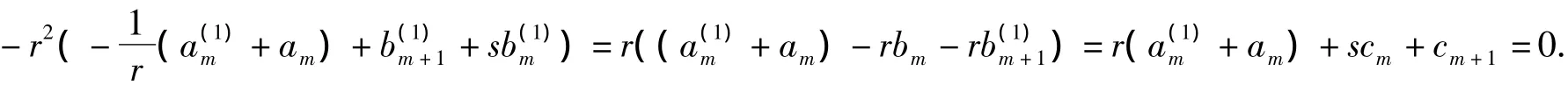
也就是说(9)中的第三个方程可由其他的推出来.
定义

方程(8)可以写成

直接计算得
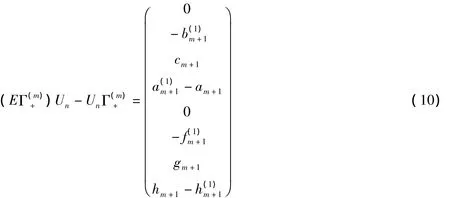
取Γ(m)=Γ(m)+,由零曲率方程Unt-(EΓ(m))Un+UnΓ(m)=0直接计算得

从(6)可得
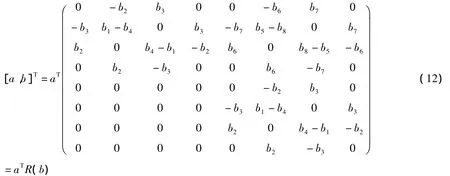
利用二次型恒等式,我们可求得对称矩阵

为建立(11)的哈密顿结构,规定
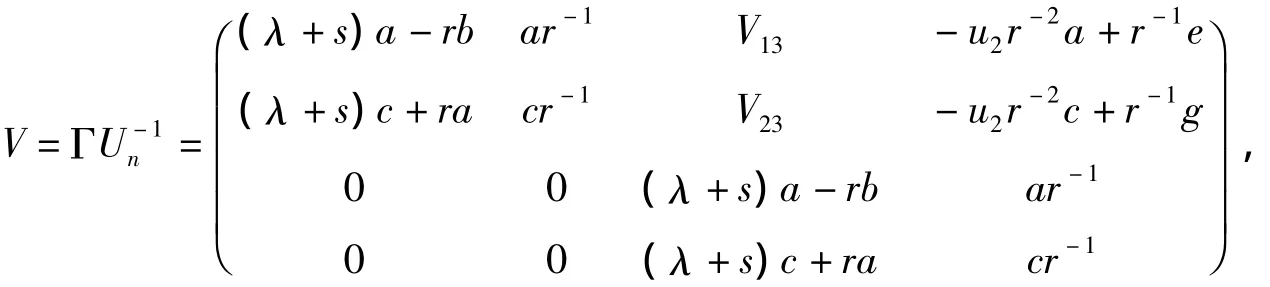
其中
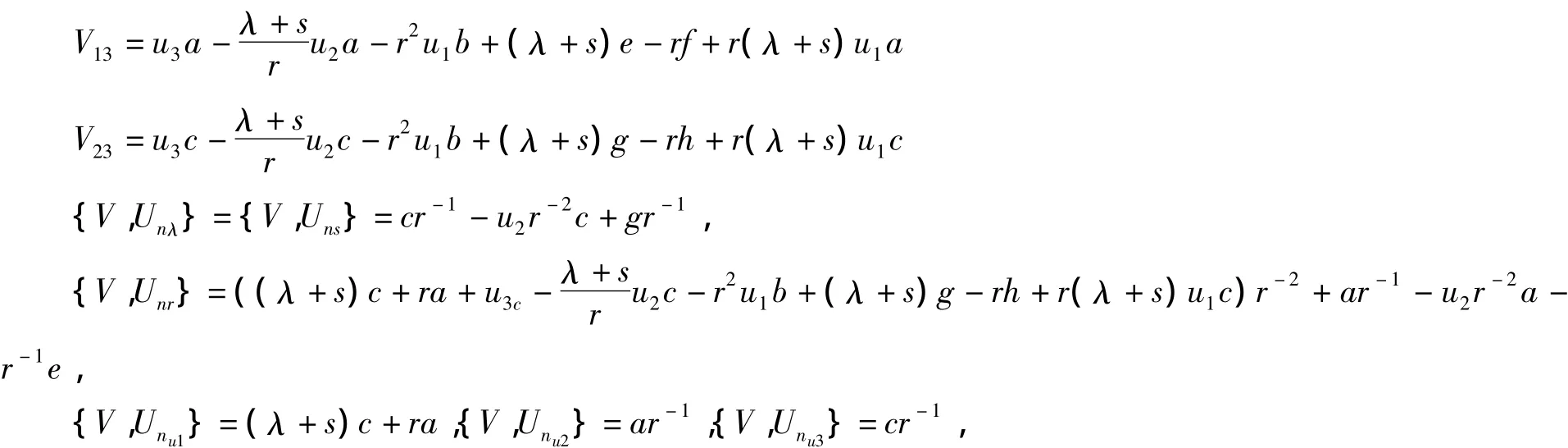
因此,利用二次型恒等式

得

其中

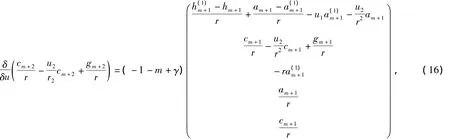
为确定常数γ,在上式两端令,n=0得γ=0,于是有
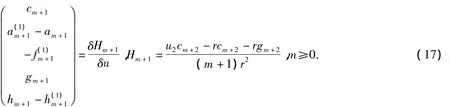
(11)可写成
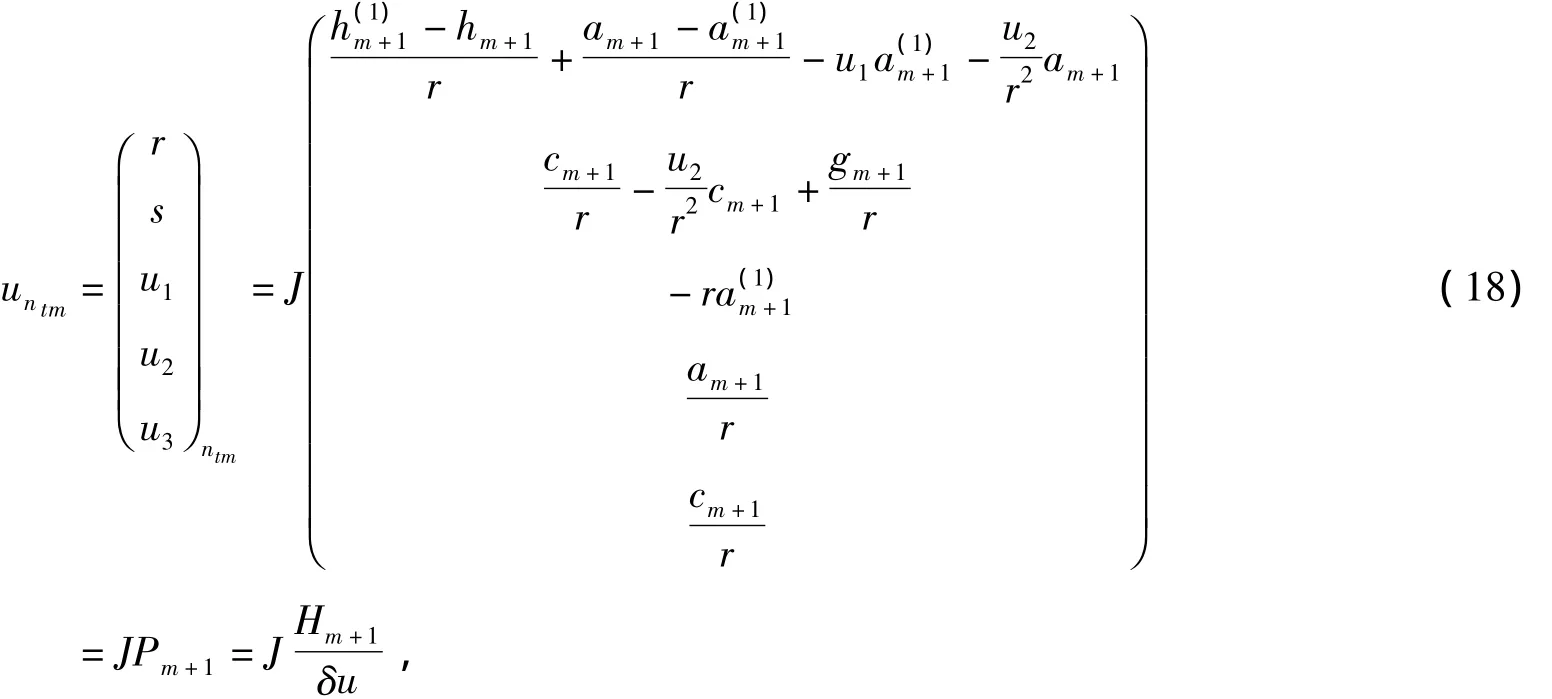

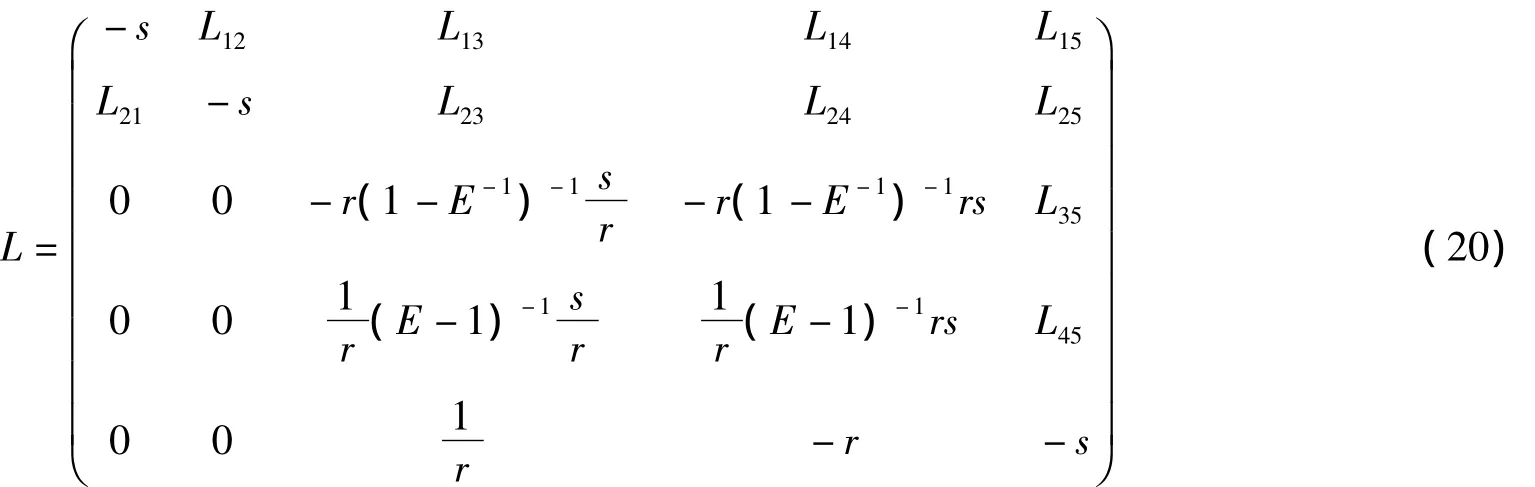
其中

因此,(18)可写成Hamiltonian形式
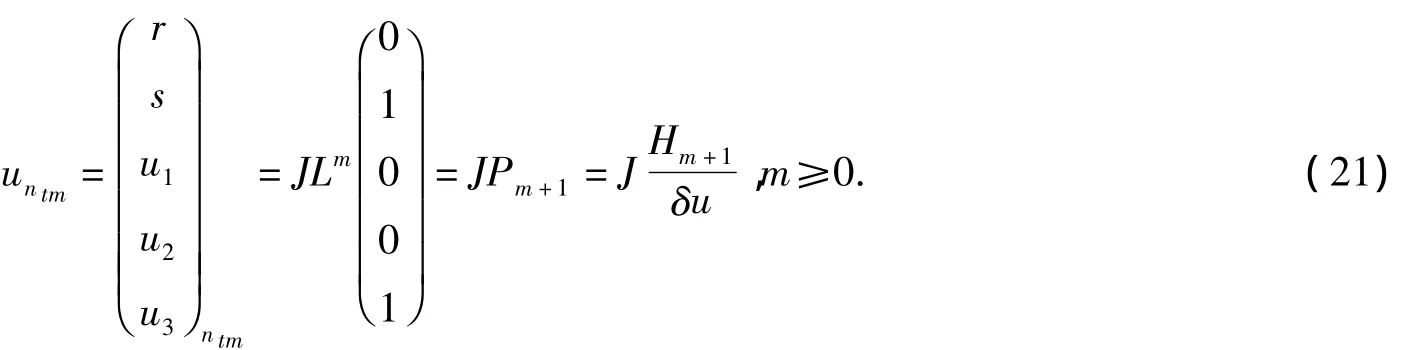
族(21)中的第一个非线性Lattice方程为

[1]Ablowitz Mark,Ladik.Nonlinear differential-difference equations[J].J.Math.Phys.,1975(16):598-603.
[2]Bogoyavlensky Oleg.Integrable discretizations of the KdV equation[J].J.Phys.Lett.A,1988,134(1):34-38.
[3]MaWenxiu,Xu Xixiang.Positive and negative hierarchies of integrable latticemodels associated with a Hamiltonian pair[J].J.Internat Theoret.Phys.,2004,43(1):219-236.
[4]Narita Kazuaki.Soliton solutions for the coupled discrete KdV equations under nonvanishing boundary conditions at infinity[J].J.Phys.Soc.Japan,2002,71(10):2401-2405.
[5]Xu Xixiang.A hierarchy of discrete Hamiltonian equations and its binary nonlinearization by symmetry constraint[J].J.Phys.Lett.A,2004,326(3-4):199-210.
[6]Xu Xixiang,Zhang Yufeng.A hierarchy of Lax integrable lattice equations,Liouville integrability and a new integrable symplectic map[J].J.Commun Theor.Phys.,2004,41(3):321-328.
[7]Tu Guizhang.A trace identity and itsapplication to the theory of discrete integrable systems[J].J.Phys.A:Math.Gen.,1990(23):3903-3922.
[8]Xu Xixiang,Yang Hongxiang,Ding Haiyong.A Liouville integrable lattice soliton equation,infinitelymany conservation laws and integrable coupling systems[J].J.Phys.Lett.A,2006,349(1-4):153-163.
[9]Zhang Yufeng,Fan Engui,Zhang Yongqing.Discrete integrable couplings associated with Toda-type lattice and two hierarchies of discrete soliton equations[J].J.Phys.Lett.A,2006,357(6):454-461.
[10]Ma Wen-Xiu,Xu Xi-Xiang,Zhang Yufeng.Semidirect sums of Lie algebras and discrete integrable couplings[J].J.Math.Phys.,2006,47(5):053501.
[11]Sun Ye-peng,Chen Deng-yuan,Xu Xi-xiang.Positive and negative hierarchies of nonlinear integrable latticemodels and three integrable coupling systems associated with a discrete spectral problem[J].J.Nonlinear Anal.,2006,64(11):2604-2618.
[12]Xia Tiecheng,You Fucai,Chen Dengyuan.A generalized cubic Volterra lattice hierarchy and its integrable couplings system[J].J.Chaos Solitons Fractals,2006,27(1):153-158.
[13]Ding Hai-Yong,Sun Ye-Peng,Xu Xi-Xiang.A hierarchy of nonlinear lattice soliton equations,its integrable coupling systems and infinitelymany conservation laws[J].J.Chaos Solitons Fractals,2006,30(1):227-234.
[14]Guo Fukui,Zhang Yufeng.The quadratic-form identity for constructing the Hamiltonian structure of integrable systems[J].J.Phys.A,2005,38(40):8537-8548.
[15]Guo Fukui,Zhang Yufeng.Expansion of the Lie algebra and its applications[J].J.Chaos Solitons Fractals,2006,27(4):1048-1055.
[16]Zhang Yufeng,Yan Wang.A higher-dimensional Lie algebra and its decomposed subalgebras[J].J.Phys.Lett.A,2006,360(1):92-98.
The Integrable Couplings of a Discrete Integrable Hierarchy and Its Hamiltonian Structure
XU Xiu-li
(Department of Senior Technology,Zaozhuang Vocational College of Science and Technology,Zaozhuang,277500,China)
The integrable couplings of a discrete integrable hierarchy and its Hamiltonian structure are obtained by the quadratic-form identity with shift operator.Thismethod can be used to produce the Hamiltonian structure of the other discrete integrable couplings.
integrable couplings;quadratic-form identity;Hamiltonian structure
O175.8
A
1672-2590(2015)03-0011-07
2015-03-27
徐秀丽(1982-),女,山东枣庄人,枣庄科技职业学院高级技工部讲师.
