偏差依赖于未知函数的高阶泛函微分方程的振动定理
2014-06-27林丹玲
林丹玲
(韩山师范学院数学与应用数学系,广东潮州521041)
偏差依赖于未知函数的高阶泛函微分方程的振动定理
林丹玲
(韩山师范学院数学与应用数学系,广东潮州521041)
考虑高阶泛函微分方程
建立其一切有界解振动的充分条件,推广和改进了已有工作中的相应结果,其中n≥2,偏差变元Δj(j=1,2,…,k)依赖于独立变量t和未知函数x.
高阶微分方程;时滞依赖未知函数;有界解;振动
1 预备知识
考虑时滞依赖于未知函数的高阶泛函微分方程
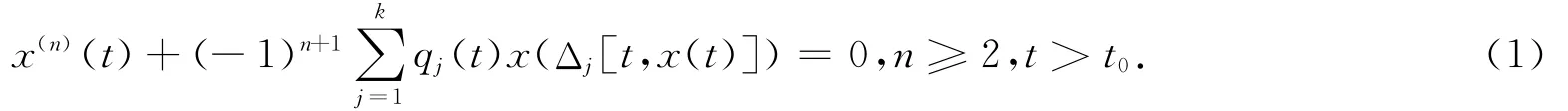
其中偏差变元Δj(j=1,2,…,k)依赖于独立变量t和未知函数x.假设下列条件对j=1,2,…,k成立:(A1)qj(t)∈C([t0,∞),R+),t0>0,R+=[0,∞),且存在j0∈{1,2,…,k},使得qj0(t)>0,t≥t0;(A2)Δj[t,u]∈C([t0,∞)×R,R),且对于每一固定的满足|u|≤D(D为正常数)的u,有Δj[t,u]<t,且
(A3)Δj[t,u1]≤Δj[t,u2],当u1≤u2<0时;Δj[t,u1]≥Δj[t,u2],当0<u1≤u2时;且Δj[t1,u]≤Δj[t2,u],当0≤t1≤t2时.
定义1[1]实值函数φ(t)称为方程(1)的解,如果它在[t0,∞)上定义,有n阶导数并且满足下列条件:
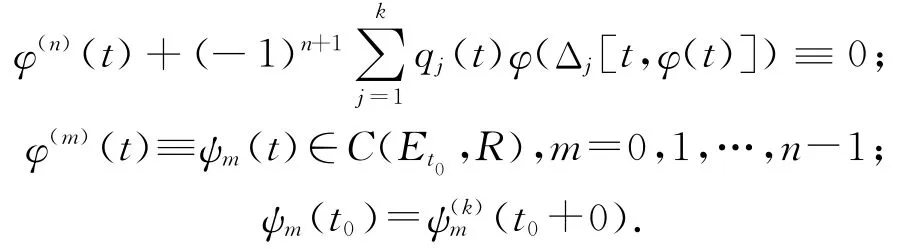
其中ψm(t)是定义在初始集合
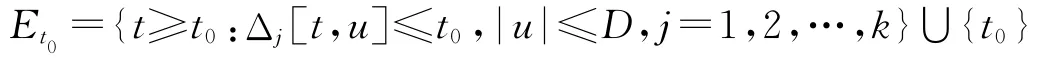
上的函数.本文考虑方程(1)的解集
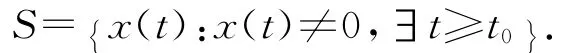
我们假设函数qj(t)和Δj[t,u]使得方程(1)存在属于S的解.
定义2 方程(1)的解称为振动的,如果它有任意大的零点;否则称它为非振动的.
近年来泛函微分方程解的振动性研究取得了丰硕成果[1-6],但其中大多数结果对偏差变元仅依赖于时间t.我们注意到偏差变元同时依赖于状态x(t)和时间t的方程在理论上和实际应用中有重要意义.然而目前文献中此类方程的振动结果还不很多[7-14],已有工作中主要是关于一阶和二阶方程解的振动性和渐近性的研究,而对一般高阶方程的振动定理尚未见到.本文的目的是给出高阶方程(1)的一切有界解的振动定理.
当方程(1)中偏差变元Δj(j=1,2,…,k)不依赖x(t)时,有如下重要特例:
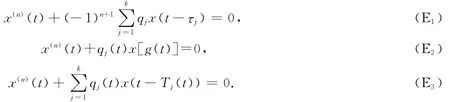
G.Ladas等人在文献[15]中证明了当k=1时,方程(E1)的一切有界解振动的必要充分条件是
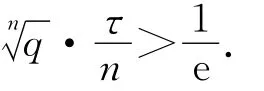
文献[3]给出,若n为奇数,q(t)>0且g(t)≤t非减,则方程(E2)的每一解振动的充分条件是
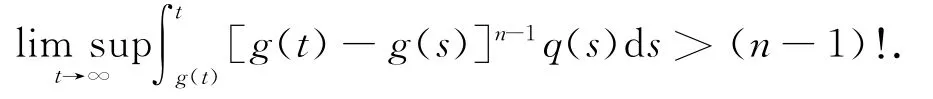
最近,文献[16]证明了,若n为奇数,qj(t)>0且Tj(t)>0,j=1,2,…,k,则方程(E3)的每一解振动的充分条件是
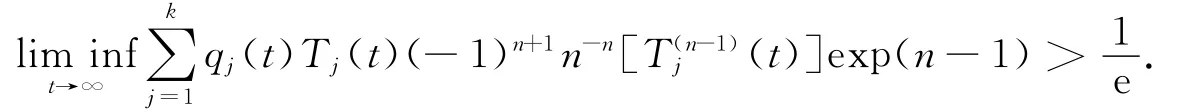
本文得到的结果不仅适用于n为奇数,而且也适用于n为偶数,因此,它部分地推广和改进了文献[3,15-16]中的有关结果.
2 主要结果
为证明本文的定理,我们需要下面两个引理.
引理1[3]设y(t)是R+上的n次可微函数且具有常号,∃t≥0,使得y(n)(t)≠0,在[t1,∞)上满足y(n)(t)y(t)≤0,则:
(ⅰ)存在t2≥t1,使得函数y(j)(t)(j=1,2,…,n-1)在区间[t2,∞)上常号.
(ⅱ)存在整数l,0≤l≤n-1,当n为偶数时,l为奇数;当n奇数时,l为偶数.使得
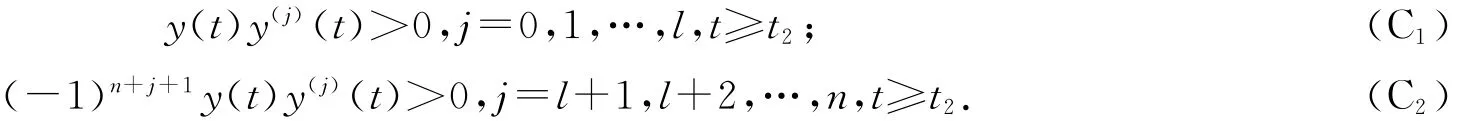
引理2[3]设y,y′,…,y(n-1)在区间(t0,∞)上绝对连续且常号,若y(n)(t)y(t)≥0,则下列结论之一成立:
(ⅰ)y(t)y(j)(t)≥0,j=0,1,…,n.
(ⅱ)存在整数l,0≤l≤n-2,当n为偶数时,l为偶数;当n奇数时,l为奇数.使得

本文主要结果为下述定理.
定理1 设条件(A1),(A2)和(A3)成立.若对每一固定的u(|u|≤D),有

证明 设方程(1)存在有界非振动解x(t),不失一般性,不妨设x(t)>0,t≥t1≥t0.由条件(A2)和(A3),存在t2≥t1,使得
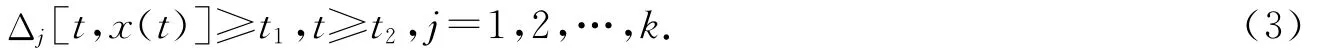
方程(1)可写为
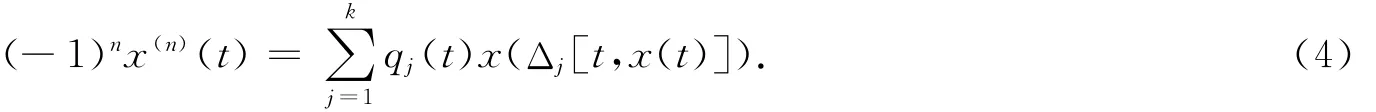
注意到条件(A1),(A3),(3)式和x(t)>0,我们有

因此,存在t3≥t2,使得当t≥t3时,x(j)(t)定号,j=0,1,…,n-1.
先令n为偶数,则x(n)(t)>0.故由引理2,存在t4≥t3和偶数l使得(C3)和(C4)式对t≥t4成立.
首先,设l≥2,则x″(t)>0,t≥t4.故x′(t)单调非减.又因x′(t)>0,此与x(t)的有界性矛盾.因此,l=0,故有
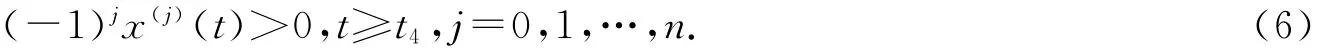
对任意u和v(u≤v)应用Taylor公式,注意到(6)式,我们得到
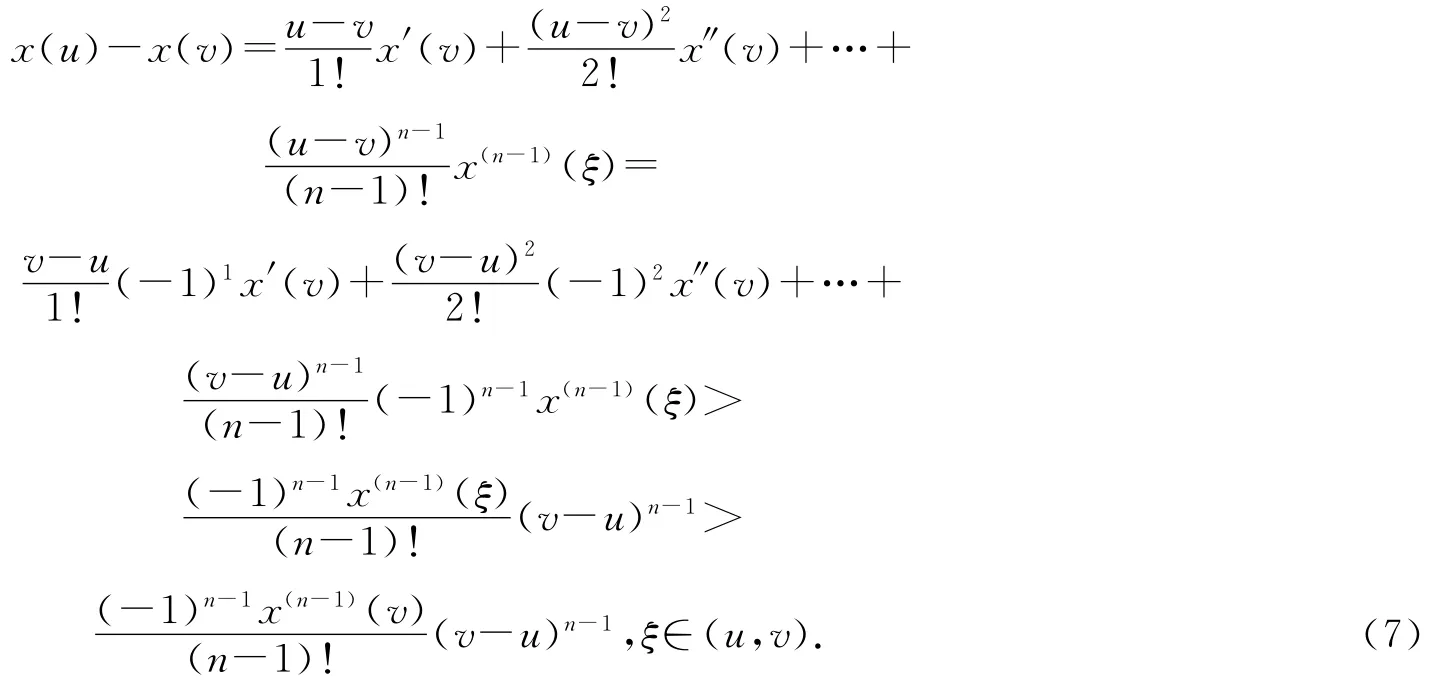
从条件(A2),(A3)和(7)式可以看出,存在t5≥t4,使得对于t≥s≥t5,有
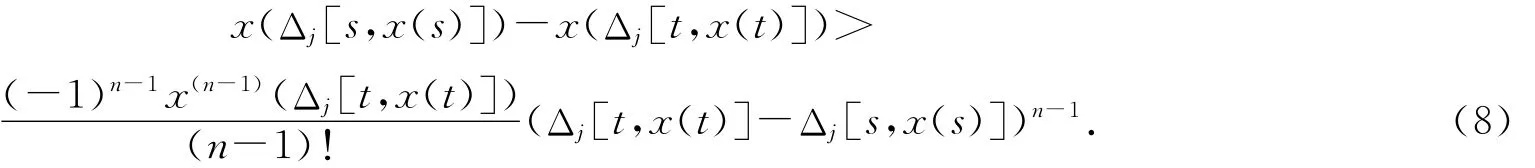
用qj(s)乘(8)式,并对j从1到k求和,注意到条件(A3),得到
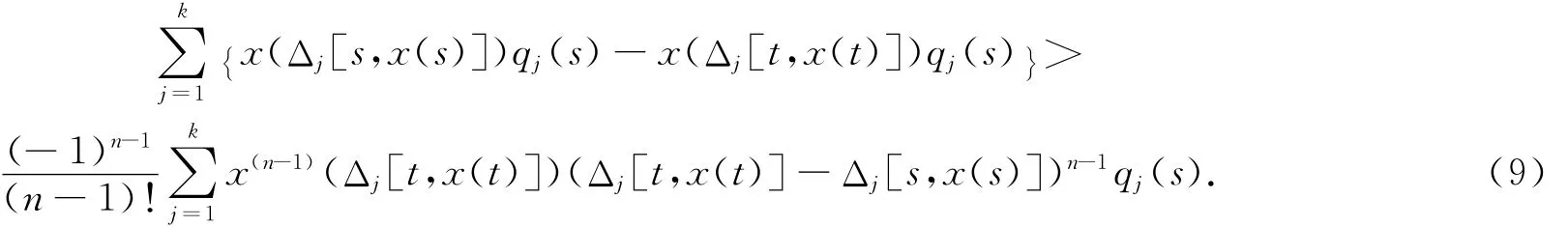
利用(4)和(9)式,并注意到n为偶数,有

从Δ*[t,x(t)]到t关于s对(10)式积分,得
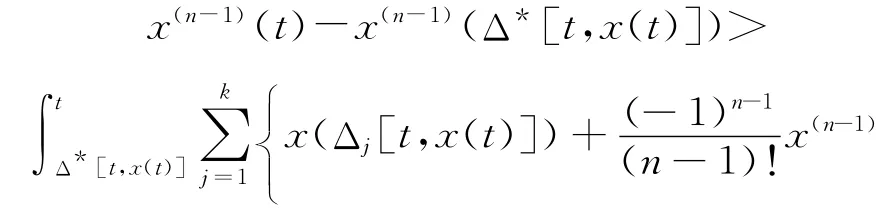
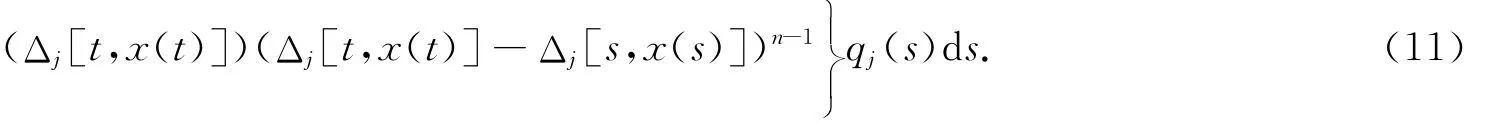
由不等式(5)知,x(n-1)(t)非减.注意到Δ*[t,x(t)]的定义,得到
因此,我们有
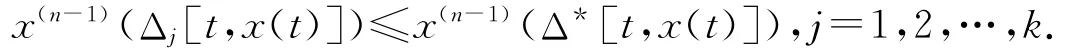
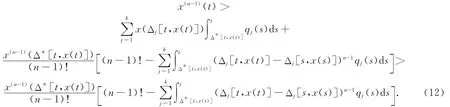
考虑到条件(A3)和表达式(Δj[t,x(t)]-Δj[s,x(s)])n-1中解出x(t)是正的,我们得到如下估计:

利用条件(2)和(13),有
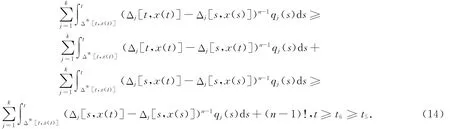
在(14)式中因x(t)非减,并满足条件(A3),可重写为
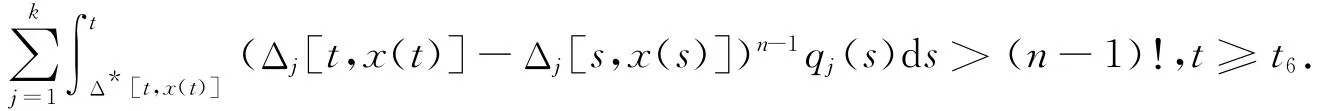
上式等价于

在(6)式中令j=n-1,得到

注意到(15)和(16)式,由(12)式产生

(17)式与(6)式矛盾.因此,当n为偶数时,方程(1)的每一有界解振动.
其次,设n为奇数,则由方程(1)我们得到x(n)(t)<0.故由引理1,存在t2≥t1和偶数l使得(C1)和(C2)对t≥t2成立.如同n为偶数的情况一样,设l≥2,则由(C1)式得x(j)(t)>0,j=0,1,2.这与x(t)的有界性矛盾,因此l=0.由(C2)式,我们有

显然,(18)式即为(6)式.应用Taylor公式和(6)式,同样得到(7)式.因n为奇数,故(-1)n-1=1.于是,(7)式成为

接下来的讨论与n为偶数的情况一样.我们可以得到x(n-1)(t)<0.但此与(18)式矛盾.因此,当n为奇
数时,方程(1)的有界解均振动.定理证毕.例1 考虑二阶方程
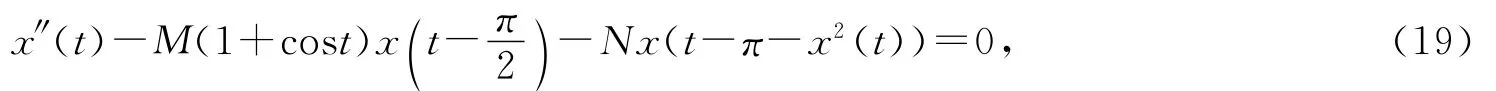
其中M和N为正常数且满足条件

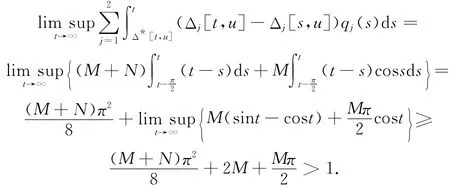
因此,根据定理1,方程(19)的每一有界解振动.
例2 考虑三阶方程

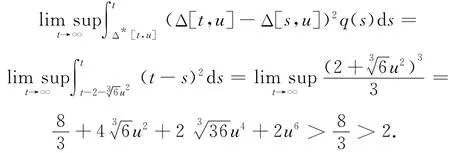
因此,根据定理1,方程(21)的每一有界解振动.
[1] SHEVELOV N.Oscillation of solutions of differential equations with deviating arguments[M].Kiev:Science Press,1978:46-79.
[2] AGARWAL R P,GRACE S R,O'REGAN D.Oscillation theory for second order dynamic equations[M].U K:Taylor &Francis,2003:35-184.
[3] ERBE L H,KONG Q,ZHANG B.Oscillation theory for functional differential equations[M].New York:Marcel Dedder,1995:288-373.
[4] GYORY I,LADAS G.Oscillation theory of delay differential equations with applications[M].Oxford:Clarendon Press,1991:32-57.
[5] LADAS G,LAKSHMIKANTHAM V,ZHANG B.Oscillation theory of differential equations with deviating arguments[M].New York:Marcel Dedder,1987:70-124.
[6] AGARWAL R P,GRACE S R,O'REGAN D.Oscillation theory for difference and functional differential equations[M].Dordrecht:Kluwer,2000:56-108.
[7] BAINOV D,SIMEONOV P.Positive solutions of a superlinear first order differential equations with delay depending on the unknown function[J].J Comput Appl Math,1998,88(1):95-101.
[8] BAINOV D,MARKOVA N,SIMEONOV P.Asymptotic behavior of the nonoscillatory solutions of differential equations of second order with delay depending on the unknown function[J].J Comput Appl Math,1998,91(1):87-96.
[9] DOMOSHNITSKY A,DRAKHLIN M,LITSYN E.On equations of second order with delay depending on solution[J].Nonl Anal,2002,49(5):689-701.
[10] HARTUNG F,TURI J.On the asymptotic behavior of the solutions of a state dependent delay equation[J].Differential and Integral Equations,1995,8:1867-1872.
[11] LI W,ZHANG S.Classifications and existence of positive solutions of higher order nonlinear iterative functional differential equations[J].J Comput Appl Math,2002,139(2):351-367.
[12] LI WANGTONG,DONG ZHONGQI.Classifications and existence of positive solutions of second order nonlinear differential equations with delay depending on the unknown functions[J].Acta Math Sci,2004,24B:403-411.
[13] LUO JIAOWAN.Asymptotic behavior of solutions of second order quasilinear differential equations with delay depending on the unknown function[J].J Comput Appl Math,2006,185(1):133-143.
[14] XU ZHITING.Oscillation and nonoscillation of second order differential equations with delay depending on the unknown function[J].J Comput Appl Math,2008,214(2):371-380.
[15] LADAS G,SFICAS Y G,STAVROULAKIS I P.Necessary and sufficient conditions for oscillation of higher order delay differential equations[J].Trans Amer Math Soc,1984,285:81-90.
[16] EL-ATTAR M A,EL-ABAD E M,EL-ABAD E E.Oscillations of the solutions of retarded differential equations[J].J Fuaay Math,2006,14:451-459.
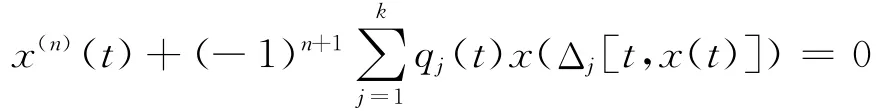
are considered,where n≥2and the deviating argumentsΔj(j=1,2,…,k)depend on the independent variable t as well as on the unknown function x.Sufficient conditions are found under which every bounded solution of the above-mentioned equation is oscillatory.
Oscillation theorem of higher order functional differential equations with deviating arguments depending on the unknown function
LIN Dan-ling
(Department of Mathematics and Applied Mathematics,Hanshan Normal University,Chaozhou 521041,China)
higher order differential equation;delay depending on the unknown function;bounded solution;oscillation
In this paper higher order functional differential equations of the type
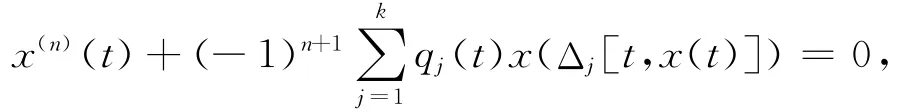
O 175 [学科代码] 110·47
A
(责任编辑:陶 理)
1000-1832(2014)02-0016-06
10.11672/dbsdzk2014-02-004
2013-03-02
国家自然科学基金资助项目(11247310);韩山师范学院科研基金资助项目(LY201302).
林丹玲(1963—),女,副教授,主要从事泛函微分方程研究.
