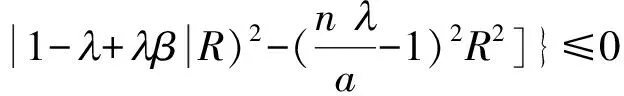推广的非函数的β阶星像性

刘名生

(华南师范大学数学科学学院,广东广州 510631)

刘名生

(华南师范大学数学科学学院,广东广州 510631)

令Hn(p)表示具有如下形式泰勒展开式

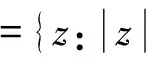
文献[1]和文献[2]分别得到关于M,,α的充分条件，使得由f(z)H1(1)和

(1-)1+Mz

其中0<α<1.SINGH[4]得到如下定理：

OBRADOVIC和OWA[5]得到如下定理：

N(,H1(1):

其中0<α<1,C,-1≤B≤1,A≠B,AR,并且研究了它的从属关系、包含关系、偏差定理和不等式性质.文献[7]研究了某类解析函数子类的性质.一个问题自然提出:是否在适当条件下，这个函数类N(,α,A,B)是星像函数类的子类？

本文的目的是确定关于p,n,,α,β和M的充分条件，使得由

(1-)1+Mz

为了得到本文的主要结果，需要如下引理.

引理1[8]设函数

ω(z)=bnzn+bn+1zn+1+…

引理2 假定0

Q(z)

和

Q(z)pγ(z)1+Mz,

Q(z)1+M1z,

Q(z)pγ(z)1+M1z,

应用文献[5]中引理2.2的方法,可以证明引理2.引理3是文献[9]中引理2.17的特殊情况.

(1)

那么P(z)1+R1z,其中R1=cR/(n-c).

证明令P(z)=1+R1ω(z)，R1=cR/(n-c).则ω(z)在U内解析, 且

ω(z)=bnzn+bn+1zn+1+…

我们要证明P(z)1+R1z,只需证明对所有zU，有.用反证法，假定不成立,则存在z0U{0}使得

根据引理1,存在实数k≥n≥1，使得

z0ω′(z0)=kω(z0).

则

这与式(1)相矛盾.因此可得P(z)1+R1z.

注1 在引理4中令n=1,可得文献[3]中相关结果.当n>1时，引理4改进了文献[5]的引理2.1.

引理5 设≠0 是一个实数,[0,1),P(z)H[1,n]和

P(z),

(2)

其中

M=Mn(,.

(3)

P(z)[1-+((1-β)p(z)+β)]1+Mz,

(4)

(5)

令P=P(z0)=u+iv,则由式(5)可得

2Re{P[1-+(β+(1-β)iρ)]}+1=

(u2+v2)2(1-β)2ρ2+2(1-β)vρ+

(u2+v2)2(1-β)2ρ2+2(1-β)vρ+

定义

G(ρ)=(u2+v2)2(1-β)2ρ2+2(1-β)vρ+

则W-M2≥G(ρ).

因为(u2+v2)2(1-β)2>0,不等式G(ρ)≥0对所有实数ρ成立，如果判别式

由式(2)可得

(6)

应用式(6)、(2)和(3),经过计算可得

因此Δ≤0,由此可得G(ρ)≥0对所有实数ρ成立,或者W≥M2,因此

这与式(4)矛盾,故引理5得证.

定理1 设>0,0<α

(7)

其中幂函数取主值，M=Mn(,pα,β)由式(3)定义,则f).

(8)

由式(7)和式(8)可得

(9)

P(z).

(10)

P(z)[1-+((1-β)p(z)+β)]1+Mz.

(11)

(12)

其中幂函数取主值，且

注2 在推论1和推论2中，令p=n=1,β=0，可得文献[3]中相关结果;在推论1中令β=0,p=1,可得定理B或者文献[5]中定理2.3;在推论1和推论2中，令p=α=1,n=2,β=0，可得定理A或者文献[4]中定理2.

注意到

可得

(13)

其中

M3().

(14)

根据定理1,可得如下定理:

定理2 设>和0

(15)

在推论3中令p=1，可得如下推论:

(16)

注3 推论4改进了定理B或者文献[5]的定理2.3;在推论4中令n=2,α=1,可得一个结果，此结果改进了定理A.

定理3 设≥1 和0

(17)

其中幂函数取主值，和

N=Nn(,α,γ)=

(18)

证明由0<γ≤1和≥1,有(,pα,0),根据定理1,得fS*(p,0).

(19)

由式(17)和式(19),可得

(20)

P(z)1+Nz.

(21)

因此由式(19)～(21)可得

即

P(z)qγ(z)1+Nz.

因为

根据式(21)和引理2,可得Req(z)>0,U.

P(z).

(22)

由式(20)和pα/(n-pα)≥1,可得

(23)

所以由式(22)和式(23)可得

即

P(z)qγ(z).

注4 在定理3中令p==1,可得定理C或者文献[5]中的定理2.4.

[1] LIU Mingsheng. On certain sufficient condition for starlike functions[J]. Soochow J of Math,2003,29(4):407-412.

[2] ZHU Yucan. Some starlikeness criterions for analytic functions[J]. J Math Anal Appl, 2007,335(2):1452-1459.

[3] OBRADOVIC M. A class of univalent functions[J].Hokkaido Math J, 1998,27(2):329-335.

[4] SINGH V. On a class of univalent functions[J].Internat J Math Math Sci,2000,23(12):855-857.

[5] OBRADOVIC M,OWA S. Some sufficient conditions for strongly starlikeness[J].Internat J Math Math Sci,2000,24(9):643-647.

[7] 刘志文, 刘名生. 某类解析函数子类的性质与特征[J]. 华南师范大学学报:自然科学版,2010(3):11-14.

[8] JACK I S. Functions starlike and convex of orderα[J]. J London Math Soc,1971,3:469-474.

[9] PONNUSAMY S,SINGH V. Criteria for strongly starlike functions[J].Complex Variables,1997,34:276-291.

LIU Mingsheng

(School of Mathematical Sciences, South China Normal University, Guangzhou 510631, China)

2012-01-12

教育部博士点基金项目(20050574002)

*通讯作者：刘名生，教授,Email: liumsh@scnu.edu.cn.

1000-5463(2013)01-0014-05

O174.51

A

10.6054/j.jscnun.2012.12.002

【中文责编：庄晓琼 英文责编：肖菁】