Li-Yorke敏感的乘积性和复合性

杨智,朱培勇,吴新星

(电子科技大学数学科学学院,四川 成都 611731)

Li-Yorke敏感的乘积性和复合性

杨智,朱培勇,吴新星

(电子科技大学数学科学学院,四川 成都 611731)

讨论Li-Yorke敏感的乘积性质以及它的迭代不变性.主要证明了Li-Yorke敏感在乘积运算下是保持的,以及在一致连续意义下,它的复合运算也是保持的.同时,举例说明该结论对于一般的连续自映射不成立.

Li-Yorke敏感;乘积映射;复合映射

1 引言及预备知识

广而言之,混沌是指系统在沿着时间维度演化的过程中所表现出来的、其微观个体的状态相对于人们的预测能力而言的不确定性,它是系统演变复杂性的重要表现.数学上的混沌概念首见于文献 [1],他们用点对的邻近和非渐近行为来刻画系统演化的复杂性,后人用Li-Yorke混沌来命名这种复杂性.30多年来数学领域中的混沌受到广泛的关注和深入的研究,基于Li-Yorke混沌的深入研究和学者们对于系统复杂性的不同认识,学者们先后研究了Devaney混沌[2],Wiggins混沌[3],本质混沌[4],稠混沌,稠 δ-混沌[5],ω混沌[6],分布混沌[7],序列分布混沌[8]和Li-Yorke敏感[9]等多种类型的混沌.

Li-Yorke混沌的一个重要推广是文献[9]于2003年提出的Li-Yorke敏感.它与Li-Yorke混沌和初值敏感依赖性密切相关.近来,文献[10]研究了Li-Yorke混沌和Devaney混沌的有限乘积性质.

基于以上原因,本文致力于讨论Li-Yorke敏感的乘积性质以及它的迭代不变性.主要证明了Li-Yorke敏感在乘积运算下是保持的,以及在一致连续意义下,它的复合运算也是保持的.

贯穿全文,(X,ϱ)表示一度量空间,容易验证:

2 Li-Yorke敏感的乘积性质

类似于定理2.1的证明,易知下一定理是显然成立的:

3 Li-Yorke敏感的复合性质

上节讨论了Li-Yorke敏感性在乘积运算下的保持性,为了更加深入的研究Li-Yorke敏感性,本节重点考察其在复合运算下的不变性.

定理 3.1 设(X,ϱ)为一度量空间,映射f:X→X一致连续.则以下命题等价:

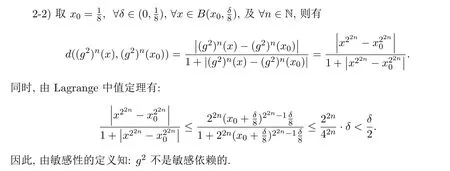
1-1)f是Li-Yorke敏感的;

[1]Li T Y,Yorke J A.Periodic three implies chaos[J],Amer.Math.Monthly,1975,82:985-992.

[2]Devaney R L.An Introduction to Chaotic Dynamical Systems[M].2nd ed.Redwood City,CA:Addison-Wesley Publishing Company,1989.

[3]Wiggins S.Introduction to Applied Nonlinear Dynamical Systems and Chaos[M].Berlin:Springer-Verlag, 1990.

[4]Pi´orek J.On the generic chaos in dynamical systems[J].Univ.Iagel.Acta.Math.,1985,25:293-298.

[5]Snoha L.Dense chaos[J].Comment.Math.Univ.Carolin.,1992,33(4):747-752.

[6]Li S.ω-Chaos and topological entropy[J].Trans.Amer.Math.Soc.,1993,399:243-249.

[7]Schweizer B,Sm´ıtal J.Measures of chaos and a spectral decomposition of dynamical systems on the interval[J]. Trans.Amer.Math.Soc.,1994,344:737-754.

[8]Wang L,Huang G,Huan S.Distributional chaos in a sequence[J].Nonlinear Anal.,2007,67:2131-2136.

[9]Akin E,Kolyada S.Li-Yorke sensitivity[J].Nonlinearity,2003,16:1421-1433.

[10]吴新星,朱培勇,关于两种混沌映射的有限乘积性质[J].纯粹数学与应用数学,2011,27(1):129-137.

Li-Yorke sensitivity in product and compositional systems

Yang Zhi,Zhu Peiyong,Wu Xinxing
(School of Applide Mathematics,University of Electronic Science and Technology,Chengdu 611731,China)

In this paper,we main discuss the properties of Li-Yorke sensitive product map and its iteration invariance,prove that the Li-Yorke sensitivity is always hold by product map operation,so its composite operation is under uniformly continuous sense.

Li-Yorke sensitivity,product map,compositional map

O189.11

A

1008-5513(2012)05-0649-06

2012-03-16.

国家自然科学基金(10671134).

杨智(1987-),硕士生,研究方向：混沌理论及其应用.

2010 MSC:54H20,58F03