时标上一阶脉冲方程的周期边值问题

尚仲平,金燕,鲁淑霞

(1.燕山大学职业技术学院,河北秦皇岛 066004;2.燕山大学理学院,河北秦皇岛 066004;3.河北大学数学与计算机学院,河北保定 071002)

时标上一阶脉冲方程的周期边值问题

尚仲平1,金燕2,鲁淑霞3

(1.燕山大学职业技术学院,河北秦皇岛 066004;2.燕山大学理学院,河北秦皇岛 066004;3.河北大学数学与计算机学院,河北保定 071002)

针对时标上一类一阶脉冲方程的周期边值问题,该论文基于算子不动点原理、上下解方法和单调迭代技巧,给出了周期边值问题解存在性的充分条件,并举例加以验证了主要结果.

时标;周期边值问题;脉冲方程;上下解方法;单调迭代技巧

MSC 2010:34K10;34B37;34B15

近年来,关于脉冲方程的周期边值问题的讨论备受广大学者的关注,但是在以往的研究过程中,大多是对微分方程的讨论,而针对时标上的一阶动力系统的讨论甚少[1-2],本文考虑如下时标上一阶脉冲方程

文中所用时标上的相关知识见文献[3],在此不再详述.记

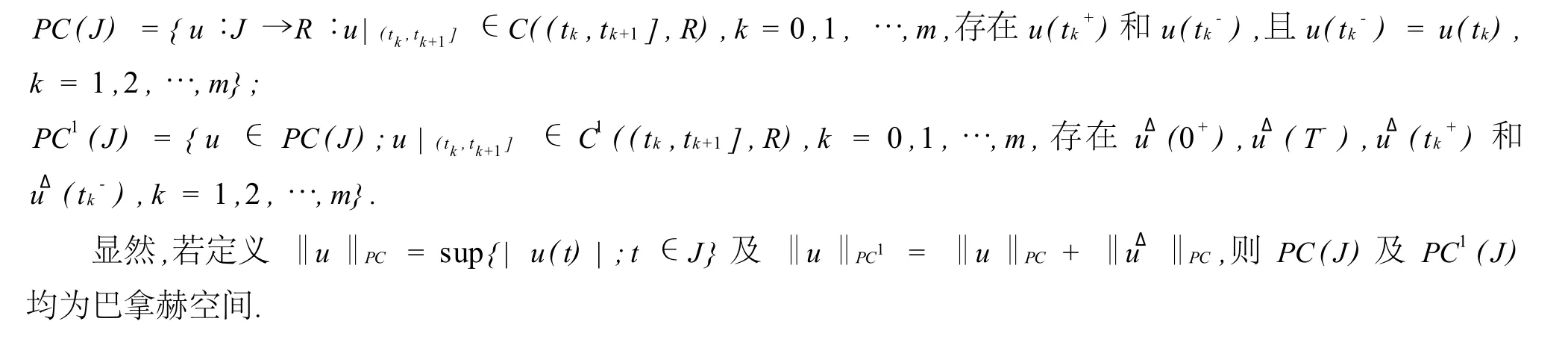
1 引理

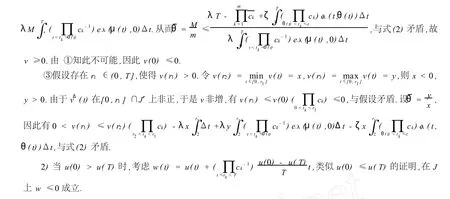
2 线性问题的解的存在性结果

3 主要结果

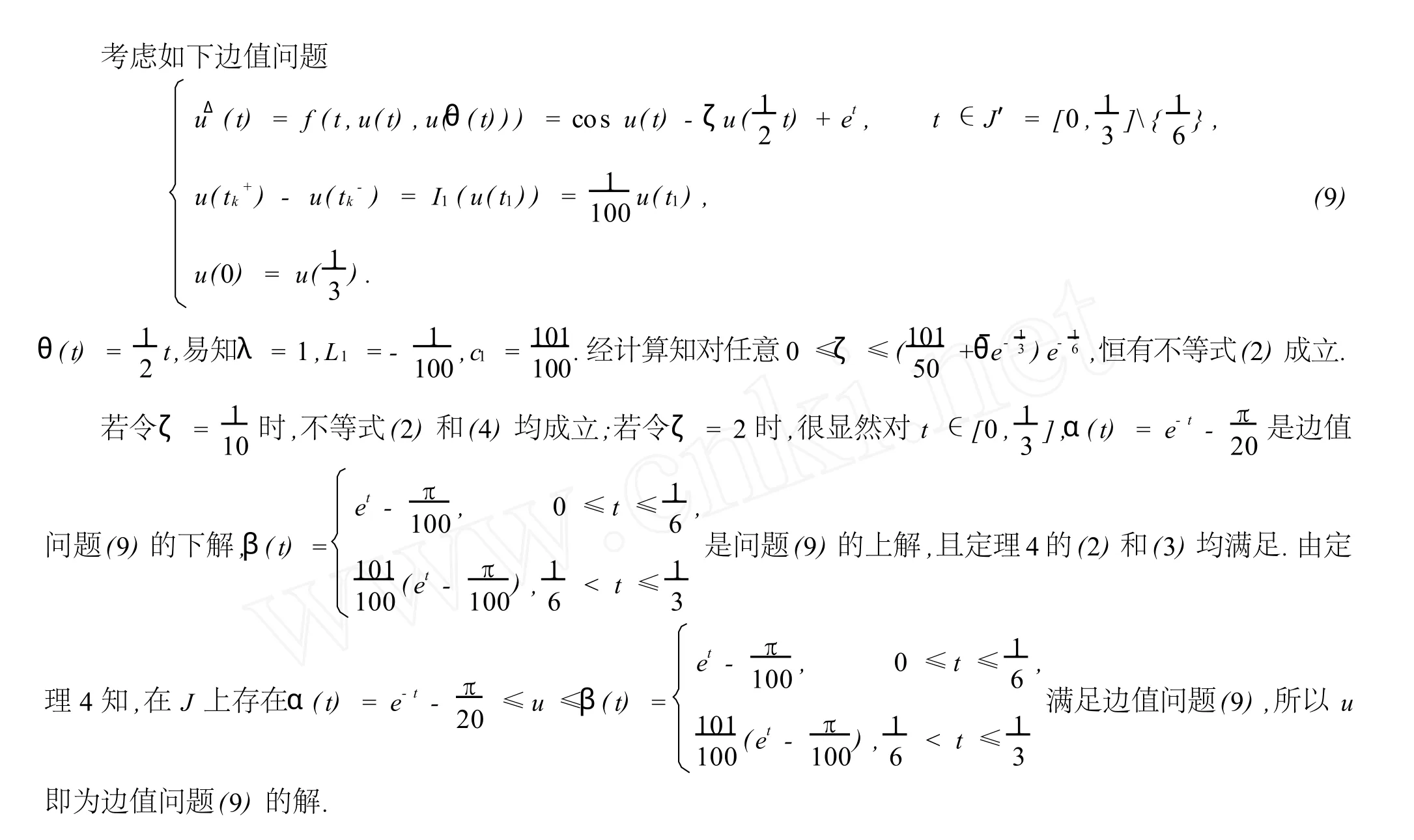
4 举例

[1]GENG Fengjie,XU Yancong,ZHU Deming.Periodic boundary value p roblems fo r first-order impulsive dynamic equations on time scales[J].Nonlinear Analysis,2008,69：4074-4087.

[2]WANGDabin.Positive solutions for nonlinear first-order periodic boundary value p roblem sof impulsive dynamic equations on time scales[J].Computers and Mathematics w ith Applications,2008,56：1496-1504.

[3]MARTIN Bohner,ALLAN Peterson.Dynamic equations on time scales,an introduction w ith app lications[M].Boston：Birkhauser,2001.

[4]LUO Zhiguo,JING Zhujun.Periodic boundary value p roblem fo r first-o rder impulsive functional differential equations[J].Computers and Mathematics w ith App lications,2008,55：2094-2107.

[5]李云红,吕文静,郭彦平.测度链上二阶边值问题多个正解的存在性[J].河北大学学报：自然科学版,2008,28(5)：462-465.

Periodic Boundary Value Problem for First-order Impulsive Functional Dynam ic Equations

SHANG Zhong-ping1,JIN Yan2,LU Shu-xia3
(1.Vocational and Technical College,Yanshan University,Qinhuangdao 066004,China;2.College of Science,Yanshan University,Qinhuangdao 066004,China;3.College of Mathematics and Computer Science,Hebei University,Baoding 071002,China)

Based on the know ledge of operato r fixed points,the upper and low er solution method and the monotone iterative technique,this paper p resents some sufficient conditions for the existence of the periodic boundary value p roblem fo r first-o rder impulsive functional dynamic equations on time scales,an examp le is also given to illustrate the main results.

time scales;Periodic boundary value p roblem;impulsive equations;lower and upper solution method;monotone iterative technique

O 175

A

1000-1565(2011)04-341-07

2010-12-05

河北省自然科学基金项目(F2011201063);河北省教育厅科学技术研究计划(2009107)

尚仲平(1964-),男,河北邯郸人,燕山大学讲师,主要从事微分方程理论的研究.

E-mail：shangzhongping1@163.com

王兰英)