多维正态分布函数的表示

周圣武， 张 艳， 韩 苗， 索新丽

（中国矿业大学理学院，江苏徐州 221116）

多维正态分布函数的表示

周圣武， 张 艳， 韩 苗， 索新丽

（中国矿业大学理学院，江苏徐州 221116）

在许多金融问题的研究中，如金融衍生产品定价、金融风险度量与管理等领域，经常用到多维正态分布函数.在概率论与数理统计文献中只给出了一维标准正态分布表，而多维正态分布函数的计算问题没有给出具体的算法.本文给出了多维正态分布函数与一维标准正态分布函数的关系式，从而解决了多维正态分布函数的计算问题.

正态分布；分布函数；协方差矩阵；正定矩阵

正态分布是概率统计中重要的分布.在许多金融问题的研究中，如金融衍生产品定价、金融风险度量与管理等领域，经常用到多维正态分布函数［1，2，3］.当标的资产价格服从对数正态分布时，其相应的金融衍生品的定价公式中通常就含有正态分布函数；特别是当金融衍生产品的价格由多个服从对数正态分布的标的变量共同驱动的情况下，该衍生产品的定价公式中就会出现多元正态分布函数［2，3，4］.在概率论与数理统计文献中只给出了一维标准正态分布表，而多维正态分布函数的计算问题没有给出具体的算法.本文研究如何用一维标准正态分布函数来表示多维正态分布函数，从而解决多维正态分布函数的计算问题.

1 多维标准正态分布函数的表示

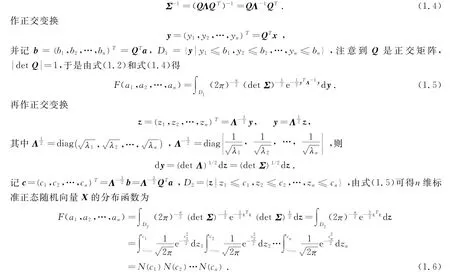
2 一般多维正态分布函数的表示

3 二维标准正态分布函数的表示

［1］ John C Hull.Options，futures and other derative securities［M］.北京：清华大学出版社，2009：507-550.

［2］ Manuel Ammann.信用风险评估—方法·模型·应用［M］.杨玉明译.北京：清华大学出版社，2004：163-198.

［3］ Peter Klein，Michael Inglis.Pricing vulnerable European options when the option’s payoff can increase the risk of financial distress［J］Journal of Banking ＆Finance.2001，25（5）：993-1012.

［4］ 陈松男.金融工程学［M］.上海：复旦大学出版社，2002：137-148.

［5］ 江龙，魏兵.线性代数［M］.徐州：中国矿业大学出版社，2004：187-205.

［6］ 周圣武，周长新，李金玉.概率论与数理统计［M］.北京：煤炭工业出版社，2007：61-87.

Expressions of Multi-dimensional Normal Distribution Function

ZHOU Sheng-wu， ZHANG Yan， HAN Miao， SUO Xin-li
（College of Science，China University of Mining ＆Technology，Xuzhou 221116，China）

In many researches of the financial problems，such as derivative securities pricing theory，financial risk measure and management and so on，multi-dimensional normal distribution functions are often used.But in the references of Probability and Statistics，the only one-dimensional standard normal distribution table was given and there’s no specific algorithm about multi-dimensional normal distribution functions.In this paper，the relational expression between multidimensional normal distribution function and one-dimensional standard normal distribution function was given.Thus the computational problems about multi-dimensional normal distribution function can be solved.

normal distribution；dsistribution function；covariance matrix；positive-definite matrix

O211.1

C

1672-1454（2011）04-0142-04

2008-11-11

中央高校基本科研业务费专项资金（JGK101677）；国家自然科学基金（61005089）