Investigation of Mg2+/Li+ Separation by Nanofiltration*

YANG Gang (杨刚)**, SHI Hong (史宏), LIU Wenqiang (刘文强), XING Weihong (邢卫红)and XU Nanping (徐南平)

State Key Laboratory of Materials-Oriented Chemical Engineering, Nanjing University of Technology, Nanjing 210009, China

1 INTRODUCTION

New technologies are urgently demanded in recent years for saving energy and reducing waste.Membrane separations have therefore been focused on,among which nanofiltration (NF) [1-3] is acknowledged as an efficient process. Such approaches were used for water softening in early days [4], and then were found to be a best choice for the treatment of process fluids. The mode is now being extended to the environmental protection area to recover valuable components. The interest herein is the NF rejections of lithium ions, which is often used for hygroscopic purpose or elsewhere (e.g. lithium battery). Actually membrane processes [5, 6] concerned this refrigeration agent, though a few ideas [7] did not seem practical yet. In nature the lithium element is basically stored in the brine. Its enrichment process is featured with evaporations, by which a variety of ions are removed through crystallizations. The work [8] on NF of the crude lithium brine was comprehensive, but the results almost led to a negative evaluation since the crude brine was too complicated. In the present industrial process it is able to evolve the lithium-containing stream into Mg2+/Li+mixture. A proper implementation of the NF technique might facilitate the removal of ions such as Mg2+with less energy consumption. In this paper, experimental investigations and theoretical predictions are given to elucidate the selective rejections of Mg2+/Li+.

2 EXPERIMENTAL

2.1 Chemicals and analysis

Analytical grade NaCl, MgSO4and glucose were used for the characterization of the DK membrane.Analytical grade LiCl and MgCl2were used to make the Mg2+/Li+/Cl-solutions shown in Table 1. Their mass ratios Mg2+/Li+are around that of the crude brine in the East Taijinaier Salt Lake [8], and the lithium ion concentrations change within a limited scope.All water used was pretreated with reverse osmosis membrane and ion exchange resin with the conductivity less than 0.5 μs·cm-1. Table 2 lists the bulk diffusion coefficients and Stokes radii of the solutes, which were incorporated in the mathematical computation.The data for lithium ion were from [9], while the rest were from [10].

Table 1 Brine compositions

Table 2 Bulk diffusion coefficient and Stokes radii

The cation concentrations were determined by Inductively Coupled Plasma (Shimdzu, Japan). The glucose concentrations were determined by TOC (total organic carbon) analyzer (Shimdzu, Japan).

2.2 Membrane and apparatus

The spiral-wound Desal DK membrane element(GE Osmonics) of nominally 8 m2membrane area was used. The parental solution was fed into the membrane element through a feeding pump and then a pressure-boosting pump. The later was equipped with a transducer for a smooth start-up and easy process adjustment. The inlet pressure and retentate flow rate were controlled with accuracy through the adjustment valve and the transducer. The retentate flow rate and the permeate flux were monitored with two electronic flowmeters, while the permeate flux records were calibrated to avoid the temperature, density and mechanical deviations. The storage tank jacket was circulated with cooling water to stabilize the feeding temperature at (35±1) °C.

2.3 Experimental procedure

All experiments were carried out at the cross-flow rate of 3 m3·h-1, at which the concentration polarization is negligible [11]. The temperature was kept constant at 35 °C. Both the retentate and permeate flew back to the feed tank. The constant process parameters and the relevant samples were available in the cycling. The permeate flux as well as the concentrations at both membrane sides were determined as the transmembrane pressure stepped up every 0.2 MPa from 0.8 MPa to 1.6 MPa. The membrane separation

factor (SF) is calculated as

3 SIMULATIONS

Modeling of nanofiltration based on the black-box treatment or the Nernst-Planck equations were reported [12-16]. A simplified Donnan steric pore model (DSPM) [17, 18] and its new version [10] were typically impressive. Much work was reported on the improvement [19]. The endeavors partly aimed at in-depth probing fundamental aspects of the mass transfer. Unfortunately, the prediction-oriented utilization is complicated, since it is difficult to obtain the physicochemical parameters such as dielectric constant or streaming potential [20]. And, the model parameters changes with process conditions. For a solution with components of relatively high concentrations,or a real wastewater that is apt to foul the membrane,the theoretical calculation seems deviated while the empirical or semi-empirical treatment works better.On the other hand, the dielectric exclusion is weakened at a high feed concentration, which is the case for the brines in [8, 21] and this paper. Therefore, only the simplified DSPM model is used herein for the process prediction and concise evaluation for rejections of the Mg2+/Li+system. The model parameters,i.e. effective membrane pore radius (rp), effective membrane thickness (Δx/Ak) and effective membrane charge density (Xd), are obtained through the characterization experiments. With the numerical treatment procedure [22], the extended prediction is available.

4 RESULTS AND DISCUSSION

4.1 Separations of Mg2+/Li+ mixture

Figure 1 Ion rejection and SF vs. permeation flux

The ion rejection and SF of Mg2+/Li+are shown in Fig. 1. For Feed A, the Mg2+rejection increases while the Li+rejection decreases with the increase of permeation flux. A strong Donnan effect is observed.In the permeation flux range, the SF decreases from 0.49 to 0.31. The Mg2+rejection for Feed B (as well as Feed C) is similar, but the Li+rejection is different,which increases slightly only at a higher flux. Negative rejections of -40%--20% are observed. The operations for the 3 mixtures started at the same working pressure, so their beginning permeation fluxes increased with their resistant osmotic pressures. The initiate values of SF for Feeds B and C decrease. It is interesting that the starting SF for Feed B is lower than that of Feed C. This is supposed to be caused by the permeation fluxes, the ion concentrations and the ratios. The trend is evident within the flux range, suggesting the phenomena are governed not only by the Donnan exclusion.

Normally a multi-valent anion is preferentially rejected by a negatively charged NF membrane if there exists a univalent anion. Herein the divalent and univalent cations are selectively rejected. This is encouraging from the perspective of field applications.The selection may be due to the electric properties and the geometric sizes of the ions. Other factors such as the dielectric properties, which are difficult to characterize, might also help lead to the above occurrences.

Figure 2 Variations of SF with retentate Li+ concentration and Mg2+/Li+ ratioΔp/MPa: □ 0.8; ○ 1.0; △ 1.2; ▽ 1.4; 1.6

The dependency of the Mg2+/Li+SFon the Li+concentration is shown in Fig. 2, where the parental Mg2+/Li+concentration ratio falls within 18-24. Under a given operating pressure,SFchanges within a narrow range with the Li+concentration or the Mg2+/Li+concentration ratio. Differently, the dependence of SF upon the operating pressure is manifest. For their single electrolyte solutions, the rejection decreases as the corresponding concentration increases, as shown in Fig. 3. It is interesting that their rejections are quite close and Li+rejection is even a little higher.The different dependencies very the electric functions that Mg2+and Li+exert at the membrane surface and inside the pore. This imply that an optimized operation is possible to obtain the Li+-enriched permeate.

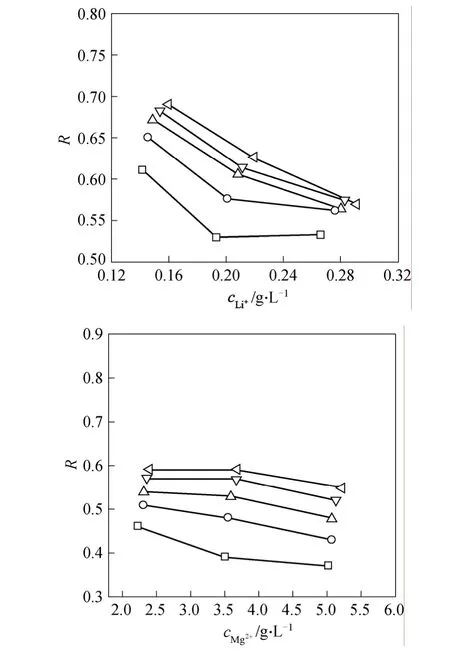
Figure 3 Rejection variations of Mg2+ and Li+Δp/MPa: □ 0.8; ○ 1.0; △ 1.2; ▽ 1.4; 1.6

4.2 Evaluation with a simulation model

With the retention data of the neutral solutes, the membrane pore radius (rp) and the effective membrane thickness (Δx/Ak) were calculated as 0.53 nm and 3.42 µm, respectively, through the best-fit method.This is slightly deviated from the data reported, which may be due to the different conditions that the membranes were produced and utilized. The effective membrane charge density (Xd) greatly relies on conditions such as pH and ionic strength [20, 23]. Several reports have used the adsorption isotherm of[20, 24] to relate it with the ionic strength. In this study, the constantsqandsare regressed from the rejections and fluxes and listed in Table 3. The results prove thatXdis greatly influenced by the solution composition, and even its sign is changed with Mg2+added.

Table 3 Xd fitted with the DSPM model as function of feed characteristics

Figure 4 gives the predicted relationship ofSFand the permeation flux. The trial withXdobtained from the other ionic systems leads to a complete failu

frroe

m.G

o

exo

pde

c

rio

mnef

on

r

tsmit

wyi tihs

ftoh

ue

n

dL

w

i+h/

Meng

2X+/dC i

ls-

r e

mgirxetsusreed.When extended to a moderately broader working pressure range, the predictedSFevolves forward smoothly.The factor decreases as the permeation increases, but the extent is gradually narrower. The factor seems to

apiTnprh epeftriehorre

ae d

cninhtf

iaf

aen

alr

o e

rlfneiimjlcetericat t itisivoo

antnlh u oa

eoft

fMaa

b tgpho2eeu+a t

0

.rs3ei

m1j

e

./ic+

ClTtailro

h

-n/

ewN

io

eat

nhf+c Ctoshol

ue-lr uaroetgfisiotunenlgnt.

emerged is not so clear here for Li (as instructed in F

noig

t.

a2p)

.p eSaurc

hs

iannc e

e

xMt

re

gm2+i t

y

is

o n f

otht

er eDj

eocntne

ad

n

ceof

fme

cp

tl e

d

teo

leys.Nevertheless the trend shown here suggests a te+chnically viable membrane approach for enriching Li.

Figure 4 DSPM predictions of SF○ experiment (Feed A); □ experiment (Feed B); △ experiment(Feed C); DSPM (Xd of Mg2+/Li+); DSPM (Xd of Na+)

The evaluated dependencies of SF upon the retentate Mg2+/Li+ratio as well as the retentate Li+concentration are given in Fig. 5. At the given working pressure,SFis basically not sensitive to the two parameters and maintains around 0.34. The rejections for two ions change, but are in the same trend. Apart from the membrane choice, the operation pressure seems to be a significant adjustment measure for an optimized purification of Li+component. The temperature factor is still left for investigation, but its influence on the membrane pore size and charge density is limited. In most cases room temperature is recommended due to the comprehensive consideration of the membrane service life, energy consumption and operation convenience.

Figure 5 Predicted SF vs. Mg2+/Li+ ratio and retentate Li+concentration

4.3 Dependency of effective membrane charge density

A typical treatment ofXdis the regression approach [10, 17, 19, 25-28], or it may be evaluated via the Gouy-Chapman double electric layer theory and experimental determination of the tangential membrane surface potential [20, 21, 29, 30]. However,Xdis an inenarrable variable [31] as it relates with membrane property, ionic adsorption and ambient pH condition.In this paper,Xdis also found dependent of the permeability, as shown in Fig. 6. An increase appears for the monovalent cation but a decrease occurs for the cation Mg2+. The contradicted trends seem to be affected by the cation valency other than the process phenomenon such as the concentration polarization.The permeate flux might affect the intrapore electrokinetic effect by its contribution to the slip plane movement of the adsorption layer. The absolute value ofXdtends to decrease as the permeate flux increases.This deserves attention in the future modeling. On the other hand,Xdvalue is intensively dependent on the cation pattern.Xdis negative for the monovalent ion but positive for the divalent ion. The trend is similar to the reports in literature [17, 28]. TheXdvariation is likely caused by the ionic adsorption that changes with the ion valency. And, theXdvalue for the Mg2+/Li+/Cl-mixture is between those for Mg2+and Li+systems. The component Mg2+preponderates for the charge density, but with the limited data, no evident linearity is found among them yet.

Figure 6 Dependency of intrapore charge density on flux○ Ni+; △ Li+; □ Mg2+; ▽ Mg2+/Li+

The Donnan potential at the pore entrance shown in Fig. 7 also changes with the permeation flux. The linearity is good but the slope and the intercept change with ion pattern. The positive slope for the monovalent ions and the negative one for the divalent cations show the diversion of the charge property. This is similar with the dependence of the streaming potential on the driving pressure, which is also linearly related [32].

5 CONCLUSIONS

A DK brand membrane was used to investigate the possibility of separating Li+from the Mg2+/Li+mixture. The prediction with the DSPM model was carried out for an extending analysis. Within the concerned concentration range, the Mg2+/Li+ratio and the Li+concentration were found basically not affecting their separation factor, while the working pressure, or the permeation flux, seemed significant. Higher driving pressure helped raising separation potential. The limitingSFof 0.31 was technically possible for richen Li+with membrane technologies. Actually, the integral membrane process design was able to facilitate a high Li+recovery at a relatively high purity. The data analysis disclosed the dependence of the intrapore membrane charge density on ion pattern, ion concentration and driving pressure force. The empirical expression ofXdfor the mixed electrolyte solution is still necessary for the probe of the separation possibility.

NOMENCLATURE

cconcentration, g·L-1

Di,∞bulk diffusion coefficient, m2·s-1

Jvvolumetric permeate flux, m·s-1

Δppressure on both sides of the membrane

qempirical parameter, mol·m-3

Rrejection

R2correlation coefficient

rpeffective membrane pore radius, m

rsstokes radius, m

sempirical parameter

SFseparation factor

Xdeffective membrane charge density, mol·m-3

zionic valence

Δx/Akeffective membrane thickness, m

0-feed side

1 Waypa, J.J., Elimelech, M., Hering, J.G., “Arsenic removal by RO and NF membranes”,J.Am.Water Works Assoc., 89, 102 (1997).

2 van der Bruggen, B., Vandecasteele, C., “Modelling of the retention of uncharged molecules with nanofiltration”,Water Res., 36, 1360-1368(2002).

3 Scarpello, J. T., Nair, D., Freitas dos Santos, L. M., White, L. S.,Livingston, A. G., “The separation of homogeneous organometallic catalysts using solvent resistant nanofiltration”,J.Membr.Sci., 203,71 (2002).

4 van der Bruggen, B., Vandecasteele, C., “Removal of pollutants from surface water and groundwater by nanofiltration: overview of possible applications in the drinking water industry”,Environ.Pollut.,122, 435 (2003).

5 Watari, K., Kobayashi, M., “Filtration method of absorptive liquid for freezer/cooling-heating device and filter cartridge”, JP. Pat.,09150040 (1997).

6 Riffat, S.B., Su, Y.H., “A novel absorption refrigeration cycle using centrifugal reverse osmosis”,J.Inst.Energ., 74, 66-69 (2001).

7 Xuan, B.M., “Lithium bromide absorption refrigerator with membrane separation unit for concentrating”, CN. Pat., 1645012 (2005).

8 Wen, X.M., Ma, P.H., Zhu, C.L., He, Q., Deng, X.C., “Preliminary study on recovering lithium chloride from lithium- containing waters by nanofiltration”,Sep.Purif.Technol., 49, 230-236 (2006).

9 Sabate, J., Labanda, J., Llorens, J., “Influence of coion and counterion size on multi-ionic solution nanofiltration”,J.Membr.Sci., 345,298-304 (2009).

10 Bowen, W.R., Welfoot, J.S., “Modeling the performance of membrane nanofiltration-critical assessment and model development”,Chem.Eng.Sci., 57, 1121-1137 (2002).

11 Yang, G., Xing, W.H., Xu, N.P., “Concentration polarization in spiral-wound nanofiltration membrane elements”,Desalination, 154,89-99 (2003).

12 Levenstein, R., Hasson, D., Semiat, R., “Utilization of the Donnan effect for improving electrolyte separation with nanofiltration membranes”, J. Membr. Sci., 116, 77-92 (1996).

13 Wang, X.L., Tsuru, T., Togoh, M., Nakao, S., Kimura, S., “The electrostatic and steric-hindrance model for the transport of charged solutes through nanofiltration membrane”, J. Membr. Sci., 135, 19-32(1997).

14 Ismail, A.F., Hassan, A.R., “The deduction of fine structural details of asymmetric nanofiltration membranes using theoretical models”,J. Membr. Sci., 231 (1/2), 25-36 (2004).

15 Ahmad, A.L., Chong, M.F., Bhatia, S., “Mathematical modeling and simulation of the multiple solutes system for nanofiltration process”,J. Membr. Sci., 253 (1-2), 103-115 (2005).

16 Sabatea, J., Labandab, J., Llorensb, J., “Influence of coion and counterion size on multi-ionic solution nanofiltration”, J. Membr.Sci., 345, 298-304 (2009).

17 Bowen, W.R., Mukhtar, H., “Characterisation and prediction of separation performance of nanofiltration membranes”, J. Membr.Sci., 112, 263-274 (1996).

18 Bowen, W. R., Mohammad, A. W., “Diafiltration by nanofiltration:Prediction and optimization”, AIChE J., 44,1799-1812(1998).

19 Bandini, S., Vezzani, D., “Nanofiltration modeling: the role of dielectric exclusion in membrane characterization”, Chem. Eng. Sci.,58, 3303-3326 (2003).

20 Szymczyk, A., Fatin-Rouge, N., Fievet, P., Ramseyer, C., Vidonne.,A., “Identification of dielectric effects in nanofiltration of metallic salts”, J. Membr. Sci., 287, 102-110 (2007).

21 Szymczyk, A., Fievet, P., “Investigating transport properties of nanofiltration membranes by means of a steric, electric and dielectric exclusion model”, J. Membr. Sci., 252, 77-88 (2005).

22 Mohammad, A.W., Takriff, M.S., “Predicting flux and rejection of multicomponent salts mixture in nanofiltration membranes”, Desalination, 157, 105-111 (2003).

23 Santafe-Moros, A., Gozálvez-Zafrilla, J.M., Lora-Garcia, J., “Applicability of the DSPM with dielectric exclusion to a high rejection nanofiltration membrane in the separation of nitrate solutions”, Desalination, 221, 268-276 (2008).

24 Childress, A.E., Elimelech, M., “Effect of solution chemistry on the surface charge of polymeric reverse osmosis and nanofiltration membranes”, J. Membr. Sci., 119, 253-268 (1996).

25 Schaep, J., Bowen, W.R., “Modelling the retention of ionic components for different nanofiltration membranes”, Sep. Purif. Technol.,22-23, 169-179 (2001).

26 Bowen, W.R., Cassey, B., Jones, P., Oatley, D.L., “Modelling the performance of membrane nanofiltration-application to an industrially relevant separation”, J. Membr. Sci., 242, 211-220 (2004).

27 Hussain, A.A., Nataraj, S.K., Abashar, M.E.E., Al-Mutaz, I.S., Aminabhavi, T.M., “Prediction of physical properties of nanofiltration membranes using experiment and theoretical models”, J. Membr.Sci., 310, 321-336 (2008).

28 Kovacsa, Z., Discacciati, M., Samhaber, W., “Modeling of batch and semi-batch membrane filtration processes”, J. Membr. Sci., 327,164-173 (2009).

29 Szymczyk, A., Sba, M., Fievet, P., Vidonne, A., “Transport properties and electrokinetic characterization of an amphoteric nanofilter”,Langmuir, 22, 3910-3919 (2006).

30 Szymczyk, A., Fatin-Rouge, N., Fievet, P., “Tangential streaming potential as a tool in modeling of ion transport through nanoporous membranes”, J. Colloid Interf. Sci., 309, 245-252 (2007).

31 Sharma, R. R., Chellam, S., “Temperature and concentration effects on electrolyte transport across porous thin-film composite nanofiltration membranes: Pore transport mechanisms and energetics of permeation”, J. Colloid Interf. Sci., 298, 327-340 (2006).

32 Fievet, P., Sba, M., Szymczyk, A., “Analysis of the pressure-induced potential arising across selective multilayer membranes”, J. Membr.Sci., 264, 1-12 (2005).