One-step quantum dialogue

Peng-Hui Zhu(朱鹏辉), Wei Zhong(钟伟), Ming-Ming Du(杜明明),Xi-Yun Li(李喜云), Lan Zhou(周澜),†, and Yu-Bo Sheng(盛宇波),,‡

1College of Science,Nanjing University of Posts and Telecommunications,Nanjing 210023,China

2College of Electronic and Optical Engineering&College of Flexible Electronics(Future Technology),Nanjing University of Posts and Telecommunications,Nanjing 210023,China

3Institute of Quantum Information and Technology,Nanjing University of Posts and Telecommunications,Nanjing 210003,China

Keywords: one-step quantum dialogue, hyperentanglement, hyperentanglement distribution, non-local Bellstate measurement

1.Introduction

Quantum secure communication can protect the security of transmitted messages based on the basic principles of quantum mechanics.Quantum secure communication has unconditional security, which is its most attractive advantage comparing with classical communication.There are some important branches in the quantum secure communication field,such as quantum key distribution (QKD),[1-9]quantum secret sharing(QSS),[10-14]quantum secure direct communication (QSDC),[15-18]and quantum dialogue (QD).[19,20]QKD and QSS can generate secret keys between two distant parties and among multiple parties, respectively.QSDC does not require keys.It enables the message sender to directly transmit messages to the message receiver through the quantum channel.QSDC has developed rapidly in theoretical and experimental aspects during the last few years.[21-42]In theory,in 2020, researchers introduced device-independent (DI) and measurement-device-independent(MDI)techniques in QSDC to enhance QSDC’s security under practical conditions.[22,23]Soon after, the masking (IN-CUM) technique was utilized in QSDC to increase its message capacity.[26]In 2023, the two-step QSDC scheme based on intermediate-basis was proposed,which used the intermediate-basis Einstein-Podolsky-Rosen(EPR)pairs to detect the channel security and help encode information.[32]Experimentally,the single-photon based QSDC experiment and entanglement-based QSDC experiment were realized in 2016 and 2017, respectively.[35,36]Recently,QSDC network experiment and a 100 km QSDC experiment were reported, which largely promoted the practicality of QSDC.[39,40]In 2023,the group of Paparelle realized the tabletop experimental demonstration of a CV-QSDC system.[42]

QD,which will be detailed here,enables two distant communication parties to exchange messages through quantum channels.[43-51]In this way, QD can play the role of bidirectional QSDC.In 2004, Nguyen proposed the first QD protocol based on quantum entanglement.[19]In 2005, Manet al.pointed out the security loophole of the QD protocol in Ref.[19] and proposed a modified secure QD protocol.[20]In 2007,a quasi-secure single-photon-based QD protocol was proposed,[43]which can guarantee the confidentiality and control of the QD content, and has certain anti-attack capabilities.In 2009, Shiet al.utilized Bell state to realize a secure QD.[45]In 2010, they proposed a secure single-photonbased QD protocol combining the idea of QSDC and BB84 protocol.[46]In 2014, a two-step QD protocol evolved from the two-step QSDC protocol was proposed,[49]whose security is assured by the two-step QSDC protocol.[16]In the same year, the entanglement swapping technique was introduced into the QD protocol.[50]Later, Gonget al.utilized the continuous-variable GHZ states to realize the quantum network dialogue.[51]

The conventional QSDC and QD protocols all require two rounds of photon transmission.We take the entanglementbased QSDC and QD protocols as examples.[15,16,19,20,45,49]Firstly, two communication parties firstly construct the longdistance entanglement channel by distributing a photon of each entangled photon pair in the quantum channel.Then,the photons are encoded by the parties.The encoded photons should be sent to one party for the Bell state measurement(BSM).However, in the photon transmission processes, the channel noise may cause photon transmission loss and quantum state decoherence, which may cause message loss and message error.Worse still, utilizing the channel noise, the eavesdropper (Eve) can intercept some messages without being detected.In 2022, the one-step QSDC protocol based on the hyperentanglement was put forward,[28]which reduces the photon transmission rounds from two to one.Compared with conventional two-step QSDC protocols,[15,16]one-step QSDC protocol can effectively simplify the experimental operation and reduce the message loss caused by the photon transmission loss.Later, to further enhance one-step QSDC’s security under practical imperfect conditions,researchers proposed DI and MDI one-step QSDC protocols.[29,30]Inspired by the one-step QSDC protocol, we propose the first one-step QD protocol.This one-step QD protocol first constructs the hyperentanglement channel between two distant communication parties.Then, the parties perform the hyperentanglementassisted complete BSM with the probability of 100%.In this way, the encoded photons are not required to transmit to one party,which can effectively reduce the message loss.To evaluate the one-step QD protocol’s performance under practical experimental conditions, we simulate its secret message capacity.Our one-step QD protocol has important applications in the quantum communication field.

The paper is organized as follows.In Section 2, we explain the one-step QD protocol based on hyperentanglement.In Section 3, an example of the one-step QD protocol is put forward.In Section 4, we analyze the theoretic security and the secret message capacity of the one-step QD protocol.In Section 5,we make some discussion and the summary elaboration.

2.One-step QD protocol

The one-step QD protocol utilizes the hyperentangled photon pairs as the sources.Hyperentanglement means entanglement in two or more DOFs of a system.Hyperentanglement has important applications in increasing the channel capacity,[52,53]realizing the high-efficient entanglement purification,[54-57]complete BSM,[58,59]and multi-DOF teleportation.[60,61]The generation of various hyperentanglement has been widely researched.[62-64]As shown in Fig.1,the one-step QD protocol can be described as follows.

Fig.1.The schematic diagram of the one-step QD protocol.The green circles linked by the dashed lines represent the initially prepared polarizationspatial-mode hyperentangled photon pairs in|φ+〉p ⊗|φ+〉s.The light blue circle pairs represent the randomly selected security checking photon pairs.The colorful circle pairs in steps (5) and (6) represent the encoded photon pairs.The light green rectangular box represents the non-local complete polarization Bell state measurement(BSM).

Step 1The communication party Alice requires to generateNpolarized-spatial-mode hyperentangled states in|φ+〉p⊗|φ+〉s(Nis large).Here,we use|H〉and|V〉to represent the horizontal polarization and vertical polarization, respectively.The four polarization Bell states can be described as

In the spatial-mode DOF,a1,a2,a′1,a′2represent different spatial modes in Alice’s location.The four spatial-mode Bell states can be described as

We divide theNcopies of photon pairs into two photon sequences S1 and S2.

Step 2Alice randomly selectsMphoton pairs for subsequent security checking and records their positions (Mis a large number andM ＜N).

Step 3The photons in sequence S2 are sent from Alice to Bob through two quantum channels, and the photons in sequence S1 are stored in Alice’s quantum memory (QM)devices.The spatial modesa′1anda′2in Alice’s location correspond to the spatial modesb1andb2in Bob’s location,respectively.Bob stores all the received photons in his QM devices.

Step 4For each security checking photon, Alice randomly selects the rectilinear (Z) basis or diagonal (X) basis in each DOF.Then, Alice announces the position and measurement bases in both DOFs of each security checking photon pair through a classical channel.Alice and Bob extract the security checking photons from the QM devices and measure them in both DOFs with the announced bases.In Fig.2,we show four linear optical apparatuses for Alice(Bob)measuring the photon inXsZp,XsXp,ZsXp,ZsZpbases and the corresponding measurement results.[65]Then,Alice(Bob)announces her (his) measurement results through the classical channel.Under the case that they both chooseZbasis in a DOF, if their measurement results are different, it indicates that a bit-flip error occurs in this DOF.Under the case that they both chooseXbasis in a DOF, if their measurement results are different, it indicates that a phase-flip error occurs.After the security checking, Alice and Bob estimate the bitflip error rate(eB)and phase-flip error rate(eP)in both DOFs.IfeBorePin any DOF exceeds a tolerable threshold,Alice determines that the photon transmission is unsafe and aborts the communication.Only when botheBandePin each DOF are lower than the tolerable thresholds, Alice and Bob continue the communication.

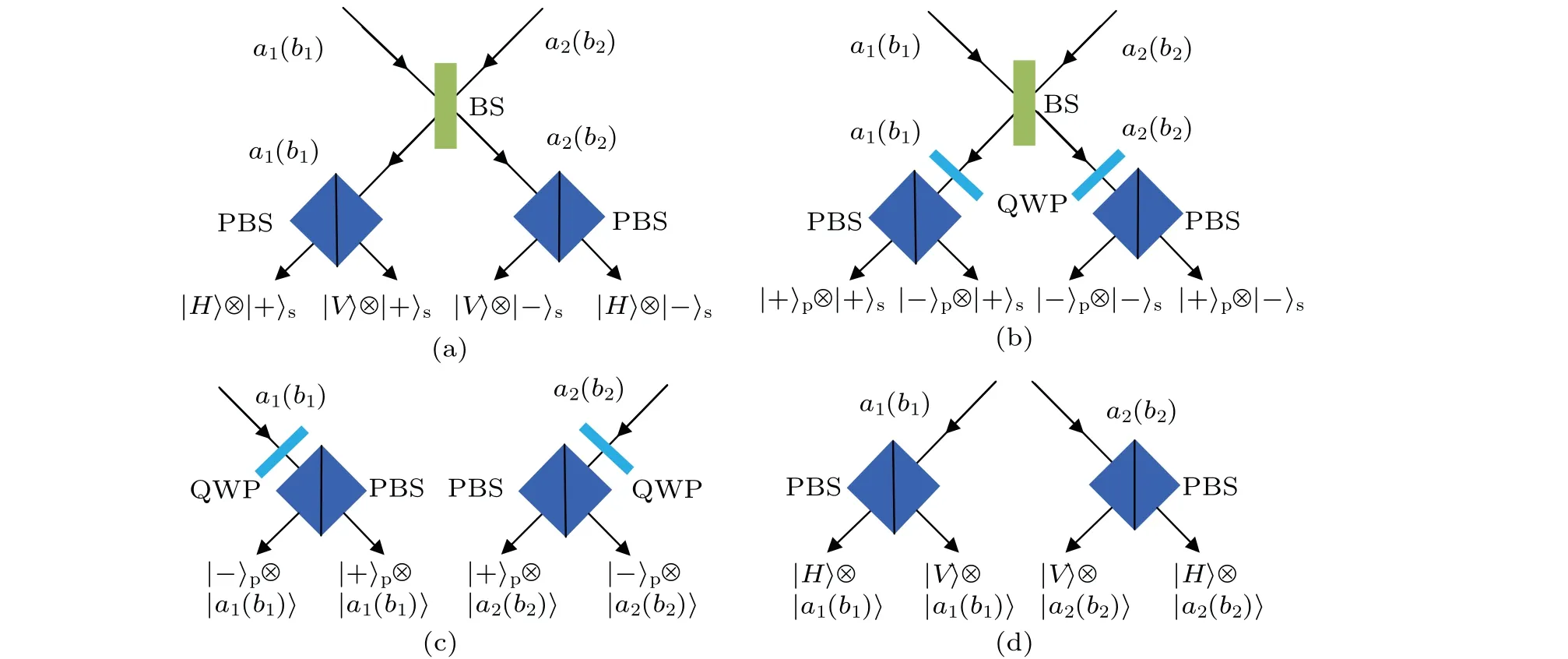
Fig.2.Four linear optical apparatuses for the parties measuring the photons in(a)XsZp, (b)XsXp, (c)ZsXp, (d)ZsZp, and the corresponding measurement results.[65] PBS and BS represent the polarization beam splitter and 50:50 beam splitter, respectively.PBS can totally transmit the photon in|H〉and totally reflect the photon in|V〉photon.BS plays the role of the Hadamard(H)gate in the spatial-mode DOF,leading|i1〉→(|i1〉+|i2〉)and|i2〉→(|i1〉-|i2〉)(i=a,b).The quarter wave plate(QWP)can be treated as the H gate in the polarization DOF,leading|H〉→(|H〉+|V〉)and|V〉→(|H〉-|V〉).

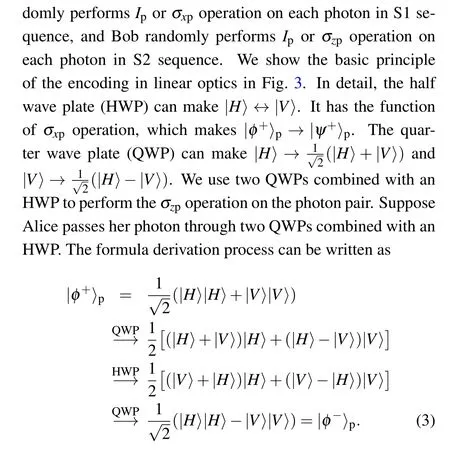
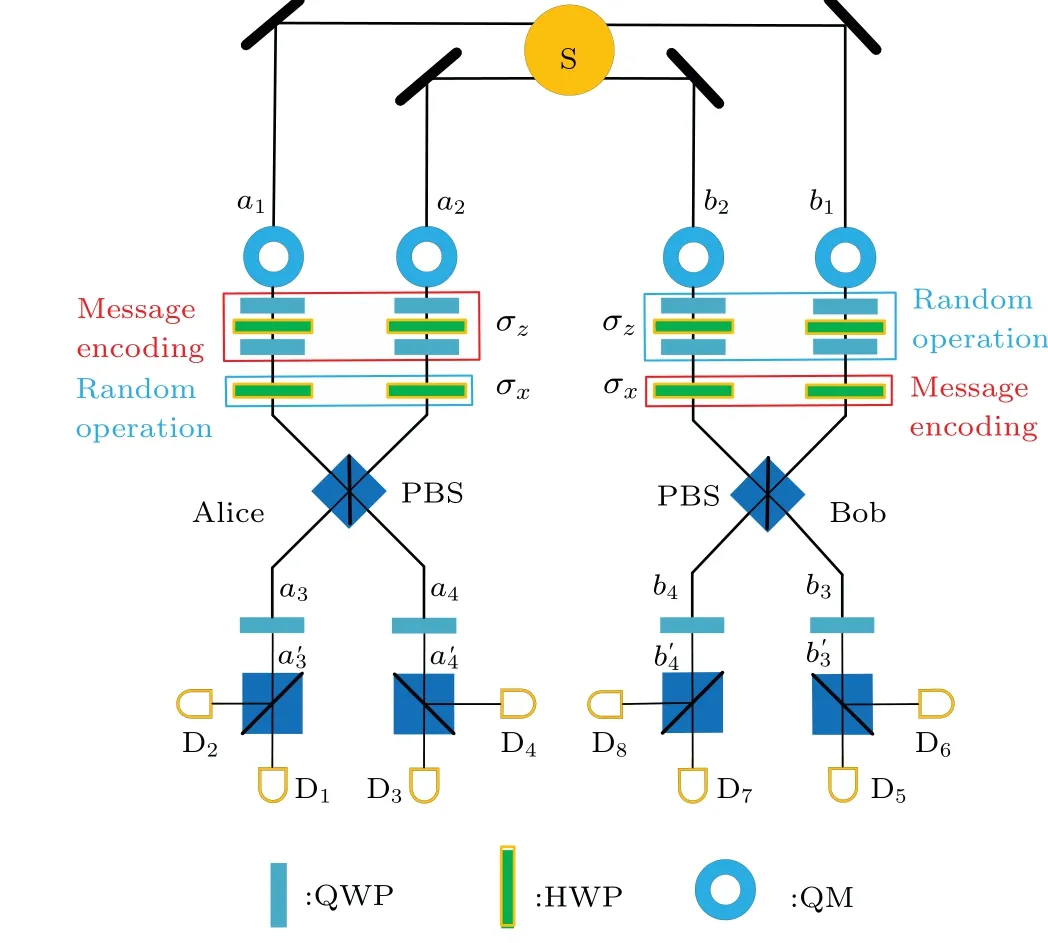
Fig.3.Schematic principle of the encoding and non-local hyperentanglement-assisted polarization BSM in our one-step QD protocol.The parties can perform the σxp operation by passing the photon through the half wave plate(HWP).Combining two QWPs with an HWP, the parties can perform the σzp operation.After the encoding,the parties perform the non-local hyperentanglement-assisted polarization BSM.[28,58] Four polarization Bell states can be distinguished by the responses of eight photon detectors D1-D8.

Step 6 Both parties perform the non-local hyperentanglement-assisted polarization BSM, which is also shown in Fig.3.After the measurement, both parties announce the detector responses through a classical channel.From the detector responses, both parties can deduce the encoded polarization Bell state.The detector responses and the corresponding polarization Bell states are shown in Table 1.Then, combined with the detector responses and their own random operations, they can deduce the encoded operations from each other and realize the bidirectional communication.As the random operations are private,anyone except the communication parties cannot read out the exchanged messages.

Table 1.The non-local complete polarization BSM results corresponding to the detector responses with the spatial Bell state being |φ+〉s.DiDj means both the photon detectors Di and Dj response.

It is worth noting that if the Bell state in the spatial-mode DOF degrades to one of the other three Bell states in Eq.(2),the parties will obtain wrong polarization BSM result from the detector responses.We list the polarization Bell states corresponding to the detector responses with the spatial-mode entanglement in|ψ+〉sin Table 2.We take a specific example.From Table 2,if the polarization is|φ+〉p,the detectors D1D7,D2D8,D3D5,or D4D6will click.However,the parties deduce the polarization Bell state from Table 1, so that they will deduce that the encoded polarization Bell state is|ψ+〉p.

Table 2.The non-local complete polarization BSM results corresponding to the detector responses with the spatial Bell state being|ψ+〉s.DiDj means both the photon detectors Di and D j response.

3.A specific example of the one-step QD protocol

To enhance the understanding of the one-step QD protocol,we provide a specific example of this one-step QD protocol.After the hyperentanglement distribution,Alice and Bob share the initial hyperentangled state in|φ+〉p⊗|φ+〉s.Suppose that Alice aims to transmit the message 1,and Bob aims to transmit the message 0.In this way, Alice performsσzpon the photon in S1 sequence, and Bob performsIpon the corresponding photon in S2 sequence.In addition,Alice also performsσxpon her photon and Bob performsσzpon his photon.After the encoding,the initial state|φ+〉pis converted to|ψ+〉p, while the state|φ+〉sis unchanged.The specific process is as follows:

which corresponds to the responses of D1D7, D2D8, D3D5,or D4D6.Based on the detector responses, the parties obtain the encoded polarization Bell state is|ψ+〉pfrom Table 1.Then, each party combined|ψ+〉pwith his/her random operation.Alice can obtain that Bob’s encoding operation isIpcorresponding to the transmitted message of 0.Bob can obtain that Alice’s encoding operation isσzpcorresponding to the message of 1.

4.Security analysis and the secret message capacity

We first analyze the theoretic security of our one-step QD protocol against the most common attack, say, the interceptresend attack.During the photon transmission process, Eve can intercept some photons.To avoid being discovered, Eve prepares some new hyperentangled photon pairs in|φ+〉p⊗|φ+〉s.He distributes one photon of each hyperentangled photon pair to Bob through a perfect quantum channel and randomly encodes the photons in his location.After the parties’encodings, Eve performs the non-local complete polarization BSM with the parties,respectively,and he can deduce Alice’s and Bob’s message according to the announced detector responses from Alice and Bob.Meanwhile,the parties can only obtain Eve’s randomly encoded messages.However, this attack can be resisted by the security checking.As Alice randomly selects a large number of security checking photon pairs in the photon sequences.It is unavoidable for Eve to intercept some security checking photons.Eve’s newly generated photons in Bob’s location are not entangled with Alice’s corresponding photons.As a result,their measurement results in each DOF may be different with a probability of 50%.In this way, Eve’s intercept-resend attack can increaseeBandePin each DOF.Under ideal conditions, if there is no eavesdropping,eBandePin each DOF are strictly equal to 0.IfeBorePin any DOF is higher than 0,Alice can detect the existence of Eve.Under practical noisy conditions,Alice sets the tolerable thresholds ofeBandePin both DOFs.If the any error rate exceeds the tolerable threshold,Alice ensures that the photon distribution is unsafe and discards the communication.

Actually,in the practical noisy channel condition,Eve can intercept a part of photons from the photon transmission process.The total error rates caused by Eve’s interception can be concealed by that caused by the channel noise,so that Eve will not be detected by the parties.As a result,Eve can obtain a part of encoded messages.As each hyperentangled photon pair carries 2 bits of messages,the message leakage rate of our one-step QD protocol is twice the photon interception rate.

For evaluating the performance of our one-step QD protocol in the practical scenario,we numerically simulate its secret message capacity(Cs).Similar to QSDC,we define the secret message capacity of QD as the ratio of the total exchanged secure and correct qubits to the overall number of encoded photon pairs.Here, we consider the symmetric scenario, where the hyperentanglement source is at the midpoint between the communication parties.Under this circumstance, the generated photon pairs hyperentangled in two DOFs pass through a quantum channel with the transmission distance ofLto reach both parties, and the communication distanced=2L.Consequently, this configuration allows Eve to intercept photons intended for each party.According to Wyner’s wiretap channel theory,[66]we can calculateCsas[38,67,68]

Here,I(A:B)(I(B:A))represents the message capacity from Alice to Bob (Bob to Alice).Similarly,I(B:E) (I(A:E))denotes the mutual message capacity between Bob and Eve(Alice and Eve).Theoretically, for a hyperentangled photon pair,Alice and Bob can exchange two bits of messages in total.With this framework, we can obtain the sum ofI(A:B)andI(B:A)as

whereH(x)represents the binary Shannon entropy asH(x)=-xlog2x-(1-x)log2(1-x)andCrawdenotes the raw message capacity.eQDrepresents the total error rate of the protocol.

Referring to the principles of the entanglement-based QKD and one-step QSDC protocols,[28,69,70]on can obtain the sum ofI(A:E)andI(B:E)as

Thus,Csof the one-step QD protocol can be calculated as

Next,we estimate the values ofCrawandeQD.We utilize the spontaneous parametric down-conversion (SPDC) source to generate the original two-photon hyperentangled states.The SPDC source can generate a hyperentangled photon pair with a probability ofP(whereP～10-3).[57]GivenP ≪1, our protocol only focuses on the vacuum state,one-pair,two-pair and three-pair emissions,while ignores the higher-order terms.Consequently, the practical photon stateρgenerated by the SPDC source can be expressed as

Following the hyperentanglement distribution, the communication parties perform the non-local complete polarization BSM.The non-local polarization BSM protocol requires eight photon detectors D1-D8,four in Alice’s location and four in Bob’s location.As illustrated in Table 1, there are four kinds of detector responses corresponding to each polarized Bell state, each with an equal probability.In this simulation,we account for the practical photon detector, which is unable to distinguish the number of incident photons.Meanwhile,the photon detector has a dark count rate denoted asY0.The detection probability of detector Dj(j=1,2,...,8)whenkphotons are incident is represented asDkj.Here,we introduce the concept of collection efficiencyα,encompassing the coupling efficiencyηcbetween the photon source and fiber, the quantum memory efficiencyηm,the photon transmission efficiencyηt=10-0.2L/10,and the detection efficiency of the detectorηd(α=ηcηmηtηd).AsαandY0are far less than 1,Dkjcan be written as

Here,we introduce a simplification by definingα′=α/4,enabling us to expressDkj ≃kα′+Y0.

The calculation ofCrawin our one-step QD protocol is similar as that in the one-step QSDC protocol.[28]First, we consider the vacuum state with the probability of 1-P-P2-P3, the clicks of all detectors are attributed to dark counts.Consequently,Craw1can be calculated as

Second,we focus on the one hyperentangled-photon-pair component in Eq.(10)with a probability ofP.After the nonlocal BSM,this photon pair can cause the click of one detector pair,while the clicks of the other detector pairs are due to dark counts.Here,we suppose the encoded hyperentangled state to be|Φ+〉=|φ+〉p⊗|φ+〉s.It may lead the response of D1D5,D2D6,D3D7,or D4D8.In this case,Craw2can be calculated as

Third, we consider the two hyperentangled-photon-pair components in Eq.(10) with a probability ofP2.The detection results can be categorized into the following two scenarios.First, only one detector pair clicks by the incident of the two photon pairs,such as D1D5.Secondly,two detector pairs click by the incident of the two photon pairs, such as D1D5and D2D6.Combining the two categories,we can obtain

Fourth, we focus on the three hyperentangled-photonpair components with a probability ofP3.This situation can be classified into three categories, say, the incident of three photon pairs may cause the clicks of one detector pair, two detector pairs, and three detector pairs, respectively.In all the three categories, we can calculateD1+D2+D3+D4=D5+D6+D7+D8=3α′+4Y0.As a result, we can obtainCraw4 as

On the other hand,we have to consider the multiple coincidences,say,three or more photon pairs click simultaneously at any one party’s side.It is obvious that the multiple coincidence will cause the failure of the non-local BSM.As the probability of four or more detectors clicking simultaneously is significantly lower than that of the threefold click,we only focus on the threefold click case.Referring to the calculations in Ref.[28], the threefold coincidence rate in the above four cases can be calculated as

Then,we focus on the total error rateeQD.The error may be caused by both the imperfect experimental devices and the decoherence during the photon transmission.We first consider the error caused by the imperfect experimental devices.We also take|Φ+〉=|φ+〉p⊗|φ+〉sas an example.Only the clicks from D1D5, D2D6, D3D7, or D4D8are correct BSM results,while other types of clicks caused by dark counts would lead to error.Therefore,the correct message capacity corresponding to the vacuum state,one hyperentangled photon pair,two hyperentangled photon pairs,and three hyperentangled photon pairs can be calculated as

In the above expression,Fp(Fs) represents the fidelity of target state in the polarization(spatial-mode)DOF.Decoherence may affect the non-local BSM results and cause errors,which may make Alice and Bob deduce incorrect messages.It is important to note that if the same kind of error occurs in both DOFs, the BSM can still obtain the right detector response.We take the case that the bit-flip error occurs in both DOFs as an example.In this case, the initial hyperentangled state will be converted to|ψ+〉p⊗|ψ+〉s.Suppose that Alice aims to transmit the message 1 and Bob aims to transmit the message 0,and their random operations are bothIp.After encoding,the hyperentangled state will evolve to|φ+〉p⊗|ψ+〉s.The BSM process can be written as

As a result, the output photons will be detected by D3D5,D4D6,D1D7,or D2D8.From Table 1,Alice and Bob can infer the encoded polarization Bell state to be|ψ+〉p, and thus can exchange correct messages 1 and 0.Similarly, if the phaseflip error or bit-phase-flip error occurs in both DOFs, Alice and Bob can also obtain the correct polarization BSM results from the detector responses.Therefore,if the same kind of error occurs in both DOFs,the parties can still exchange correct messages based on the BSM results.

Thus,the total correct rateCcorrecttis

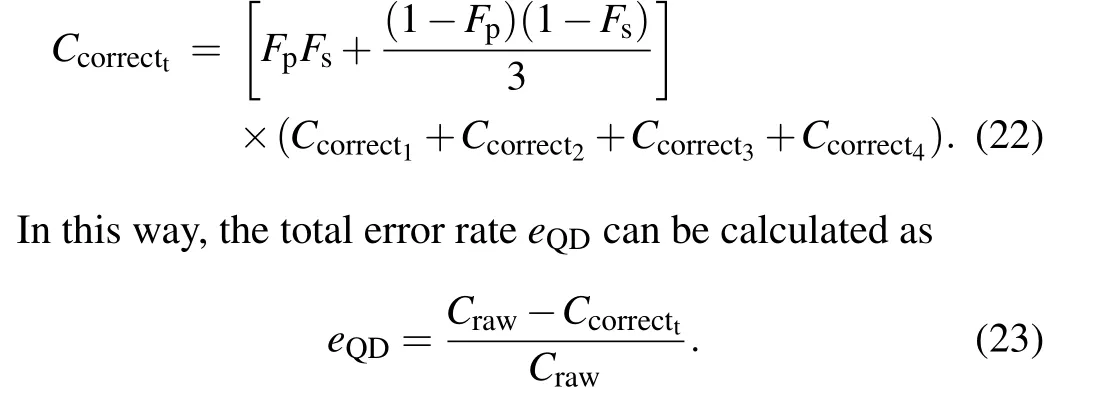
Taking the values ofCrawandeQDin Eqs.(18) and (23)into Eq.(9),we calculate the value ofCs.In Fig.4,we showCsof the one-step QD protocol altered with the communication distancedbetween the communication parties.We fixY0=6.02×10-6,ηm=ηc=0.95,ηd=0.9.For calculatingηt, we chooseα=0.2 dB/km.The parametersFpandFsare adjusted to be 1, 0.98, 0.96, respectively.It can be seen that whenFp=Fs=0.98, the maximum communication distance achieves approximately 211 km.With the repetition rate of the SPDC source being 10 GHz[71]andFp=Fs=0.98,Csis around 1435 bit/s at a communication distance of 100 km.

Fig.4.The secret message capacity Cs alters with the communication distance d between the communication parties.Here, we fix Y0 =6.02×10-6, ηm =ηc =0.95, ηd =0.9, and adjust Fp =Fs =1,0.98,0.96,respectively.

5.Discussion and conclusion

QD enables two communication parties to directly exchange secret messages simultaneously,realizing real-time secure bidirectional communication.Similar to conventional QSDC protocols,[15-18]previous QD protocols require to transmit photons in the quantum channel twice.[19,20,43-47]The channel noise may cause photon transmission loss and quantum state decoherence in each photon transmission process.The photon transmission loss and quantum state decoherence can largely limit the maximal secure communication distance and reduce the secret message capacity.Worse still, they may cause message loss and message error, which makes the transmitted messages incomplete and incorrect.In our QD protocol, the parties only require to distribute photons in the quantum channel once, which can simplify the experimental operation and reduce the photon transmission loss.Most entanglement-based QD requires the complete BSM.[19,20,45,49]However, the linear-optical BSM only has a success probability of 50%.The complete BSM often relies on nonlinear optical elements,[72-76]which are difficult to realize under current experimental conditions.In contrast, our onestep QD protocol adopts the hyperentanglement-assisted nonlocal complete polarization BSM with a probability of 100%,which is feasible with current linear optical devices.

QM is an important element of our one-step QD protocol.In the protocol, the parties should store the photons in the quantum memory until they ensure the photon transmission being secure.During recent few years,QM has achieved great experimental progresses.[77-83]In 2017, a high-fidelity nanophotonic QM with＞95% spin polarization for efficient initialization of the atomic frequency comb memory and time bin-selective readout was experimentally demonstrated.[78]In 2018, Hsiaoet al.achieved a storage efficiency of 92.0%for a coherent optical memory based on the electromagnetically induced transparency (EIT) scheme in optically dense cold atomic media.[79]In 2019,a high-performance atomic Raman memory in87Rb vapor with the memory efficiency of above 82.0%for 6 ns-20 ns optical pulses and the unconditional fidelity of up to 98.0% was achieved.[80]Later, the coherent storage of light in an atomic frequency comb memory over 1 hour was realized by the group of Ma.[82]Recently, Zhanget al.reported their achievement on the fiber-integrated multimode quantum storage of single photon at telecom band with 330 temporal modes on a laser-written chip.[83]Based on these attractive progresses,our one-step QD protocol may be experimentally realized in the near future.

From the security analysis, the channel noise makes it possible for Eve to intercept some photons without being detected,and increases message loss rate and message error rate.Similar to QSDC, in QD, the communication parties cannot perform the post-processing technique to resist message loss and message errors.In this way, the message loss and message error are two big obstacles in the practicality of QD.Actually, we can adopt the polarization-spatial-mode hyperentanglement purification and heralded amplification to modify our one-step QD protocol.In detail, Bob can perform the hyperentanglement amplification to herald the arrival of each transmitted photon.[84]After that,both parties can repeat the hyperentanglement purification[85]to improve the fidelity of the hyperentanglement channel.In theory, Alice and Bob can construct the nearly perfect hyperentanglement channel,where the parties can detect any eavesdropping behavior from Eve.As a result, the message leakage loophole can be eliminated.Meanwhile, the message loss and message error can be also nearly eliminated, thus guaranteeing the correctness and integrity of the transmitted messages.In contrast, in the previous entanglement-based QD protocols,[19,20]the parties cannot perform the EPP after the second photon transmission round, for the EPP may change the encoded messages.As a result, the decoherence caused by the second photon transmission round cannot be eliminated,which can bring security loophole and message errors.Moreover,our one-step QD can be combined with the quantum repeater and drone to construct the long-distance hyperentanglement channel,and thus realize the long-distance one-step QD.

In summary, we propose the first one-step QD protocol with the help of hyperentanglement and non-local complete BSM.In the protocol, two communication parties first construct the hyperentanglement channel.After checking the security of the photon transmission process, they encode their messages in the polarization DOF of each hyperentangled photon pair.Then, by performing the non-local hyperentanglement-assisted complete polarization BSM,they can finally obtain the exchanged messages.This one-step QD protocol is theoretically secure and two parties can exchange 2 bits of messages by using a hyperentangled photon pair.The secret message capacity of the one-step QD protocol is numerically simulated.We obtain that with the fidelities in both DOFs ofFp=Fs=0.98,the one-step QD protocol can achieve the maximal communication distance of about 211 km.Compared with previous QD protocols, our one-step QD protocol has some attractive advantages.First, photons only need to transmit in the quantum channel once, which can simplify the experiment operations and reduce the photon transmission loss.Second, the non-local complete polarization BSM can completely distinguish four polarization Bell states and is feasible with current technique.Third, combined with the heralded amplification and purification,the nearly perfect hyperentanglement channel can be constructed between two parties,which can nearly eliminate the message leakage loophole,the message loss and message error.Moreover, combined with quantum repeater and drone,our one-step QD is possible to realize long-distance QD.In this way,our one-step QD protocol is an important development of QD and will have important applications in future quantum communication field.

Acknowledgement

Project supported by the National Natural Science Foundation of China(Grant Nos.12175106 and 92365110).