内含k个H-点且边界H-点数为3k+5的H-三角形

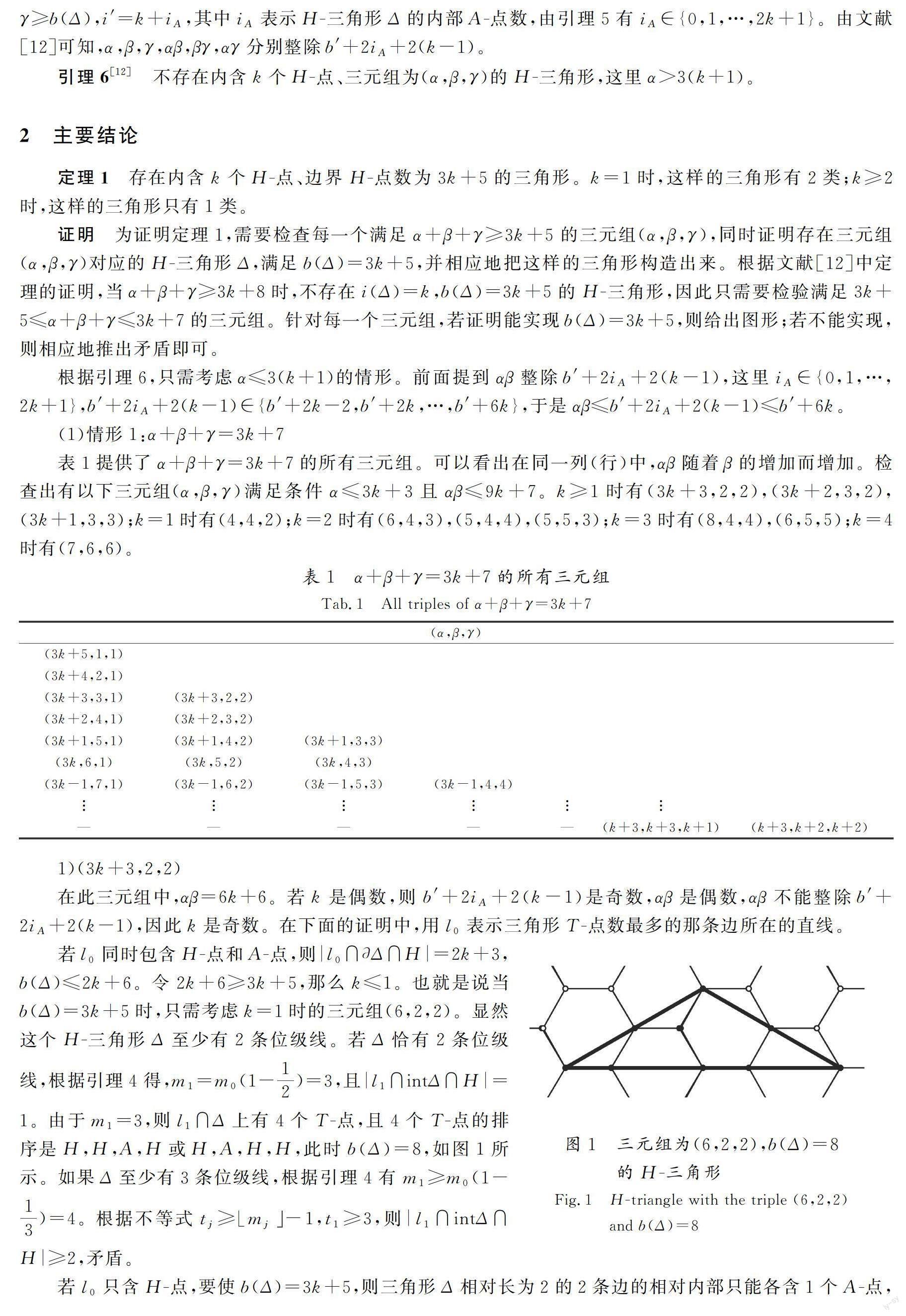
朱伟丽 魏祥林

摘 要：为了研究内含k个H-点的H-多边形的边界特性和几何结构，针对正六边形阿基米德铺砌，研究铺砌上的H-三角形内部H-点和边界H-点的关系。首先，通过分析H-三角形的三元组（α，β，γ），确定所有可能满足要求的三元组；其次，利用位级线理论和铺砌点分布特性，排除不能实现的三元组；最后，证明内含k个H-点且边界H-点数为3k+5的H-三角形存在，且只有2种构图，并给出这2种构图的具体构造。结果表明，在能够确定三角形所有可能的三元组条件下，H-三角形满足给定边界点数的图形结构是确定的。研究结果丰富了阿基米德铺砌的相关理论，也为阿基米德铺砌相关问题的研究提供了重要的理论依据。

关键词：离散数学；离散几何；阿基米德铺砌；正六边形铺砌；H-三角形；H-点

H-triangle with k interior H-points and 3k+5 boundary H-points

ZHU Weili，WEI Xianglin

（School of Sciences， Hebei University of Science and Technology， Shijiazhuang， Hebei 050018， China）

Abstract：In order to study the boundary characteristics and geometric structure of an H-polygon with k interior  H-points，the relationship between the interior H-points and boundary H-points of the H-triangle in a regular hexagonal Archimedean tiling was studied. Firstly， by analyzing the triple （α，β，γ）  of H-triangle， the triples that may meet the requirements were determined. Then， the impossible triples were excluded by using the theory of level and the distribution characteristics of tiling points. Finally， H-triangle with k interior H-points and 3k+5 boundary H-points was obtained. Considering there were only two types of configurations， the specific constructions of these two configurations were given. The results show that the configurations of the H-triangle which satisfies the given number of boundary H-points are certain under the condition that all possible triples of triangle can be determined. The research results enrich the related theories of Archimedean tiling， and provide an important theoretical basis for the research of related problems of Archimedean tiling.

Keywords：discrete mathematics；discrete geometry；Archimedean tiling; regular hexagonal tiling; H-triangle; H-point

離散与组合几何^［1-2］中的一个重要研究问题是阿基米德铺砌^［3］问题，阿基米德铺砌是指用一种或多种正多边形铺砌全平面，且要求铺砌的每个顶点的特征相同。如果阿基米德铺砌中围绕一顶点的铺砌元按环形循序是n1-边形、n2-边形等，则称该铺砌属［n1，n2，…］型，阿基米德铺砌共有11种^［4-8］。正六边形阿基米德铺砌，即［6.6.6］铺砌，是由边长为单位长度的正六边形构成的平面铺砌。设H是［6.6.6］铺砌上正六边形的顶点集，H中的点称做H-点，顶点落在H上的多边形称为H-多边形。1988年，DING等^［9］针对［6.6.6］铺砌给出了新的PICK型定理，为研究［6.6.6］铺砌上的H-多边形问题打下了基础。

3 结 语

本文运用H-三角形三元组（α，β，γ）的性质和位级线理论，结合铺砌点的分布特性，研究了［6.6.6］阿基米德铺砌上H-三角形内部H-点和边界H-点的关系。证明了当k取任意值时，内含k个H-点、边界H-点数为3k+5的H-三角形存在，并且只有2种构图。

研究结论丰富了正六边形阿基米德铺砌的相关理论。2种构图的获得对H-四边形的同类问题研究至关重要，能够提供有效的论证方法和分类技巧。但是，关于H-多边形的研究仍不是很全面，在今后的研究中，将利用铺砌理论、分划理论、凸集理论等方法，深入研究H-多边形的边界点问题及相关应用。

参考文献/References：

［1］ GRUBER P M.Convex and Discrete Geometry［M］.Berlin：Springer，2007.

［2］ PACH J，AGARWAL P K.Combinatorial Geometry［M］.New York：Wiley，1995.

［3］ GR?NBAUM B，SHEPHARD G C.Tilings and Patterns［M］.New York：W.H. Freeman，1986.

［4］ WEI Xianglin，WANG Jianjun，GAO Feixing.A note on area of lattice polygons in an Archimedean tiling［J］.Journal of Applied Mathematics and Computing，2015，48（1）：573-584.

［5］ VASSALLO S F.Buffon type problems in Archimedean tilings［J］.Universal Journal of Mathematics and Mathematical Sciences，2013，4：201-219.

［6］ EGGLETON R B.Tiling the plane with triangles［J］.Discrete Mathematics，1974，7（1/2）：53-65.

［7］ 徐倩.阿基米德铺砌图相关性质的研究［D］.石家庄：河北师范大学，2014.

XU Qian.Some Properties on Archimedean Tiling Graphs［D］.Shijiazhuang：Hebei Normal University，2014.

［8］ 林松.阿基米德铺砌相关性质的研究［D］.石家庄：河北师范大学，2013.

LIN Song.On Some Related Properties of Archimedean Tilings［D］.Shijiazhuang：Hebei Normal University，2013.

［9］ DING REN，KO?ODZIEJCZYK K，REAY J.A new pick-type theorem on the hexagonal lattice［J］.Discrete Mathematics，1988，68（2/3）：171-177.

［10］KOLODZIEJCZYK K.Hex-triangles with one interior H-point［J］.Ars Combinatoria，2004，70：33-45.

［11］WEI Xianglin，DING Ren.H-triangles with 3 interior H-points［J］.Journal of Applied Mathematics and Computing，2008，27（1）：117-123.

［12］WEI Xianglin，DING Ren.H-triangles with k interior H-points［J］.Discrete Mathematics，2008，308（24）：6015-6021.

［13］WEI Xianglin，GUO Zihuan.H-quadrilateral with one interior H-point［J］.Ars Combinatoria，2020，152：257-262.

［14］WEI Xianglin，GAO Feixing.A disproof of the conjecture about boundary H-points of H-triangles［J］.Journal of Applied Mathematics and Computing，2016，51（1）：299-313.

［15］高飞星.正六边形铺砌上H-三角形边界H-点数的研究［D］.石家庄：河北科技大学，2015.

GAO Feixing.The Study about the Number of the Boundary H-points of H-triangle on the Regular Hexagonal Tiling［D］.Shijiazhuang：Hebei University of Science and Technology，2015.

［16］王华宁.正六边形阿基米德铺砌上H-多边形边界H-点的研究［D］.石家庄：河北科技大学，2020.

WANG Huaning.The Study about the Boundary H-points of H-polygon on Regular Hexagonal Archimedean Tiling［D］.Shijiazhuang：Hebei University of Science and Technology，2020.

［17］KO?ODZIEJCZYK K，OLSZEWSKA D.A proof of Coleman′s conjecture［J］.Discrete Mathematics，2007，307（15）：1865-1872.

［18］WANG Qi，GAO Feixing，WEI Xianglin.The number of boundary H-points of H-triangles［J］.Ars Combinatoria，2017，132：3-9.

［19］DING Ren，REAY J R，ZHANG Jianguo.Areas of generalizedH-polygons［J］.Journal of Combinatorial Theory，Series A，1997，77（2）：304-317.

［20］曹鵬浩.关于H-点相关问题的研究［D］.石家庄：河北师范大学，2010.

CAO Penghao.On Some Problems Related to H-points［D］.Shijiazhuang：Hebei Normal University，2010.

［21］魏祥林，王卫琪.关于［4.8.8］铺砌中椭圆上D-点数的研究［J］.河北科技大学学报，2017，38（2）：143-150.

WEI Xianglin，WANG Weiqi.Research about the number of D-points of ［4.8.8］-tiling in given ellipse［J］.Journal of Hebei University of Science and Technology，2017，38（2）：143-150.

［22］KO?ODZIEJCZYK K.Realizable quadruples for Hex-polygons［J］.Graphs and Combinatorics，2007，23（1）：61-72.