坐标变换下平面解析系统单值轨道的不变性

郭春　黄土森

摘 要： 为了研究平面解析系统在何种坐标变换下单值轨道具有不变性，首先给出平面解析系统的轨线沿固定方向进入奇点的两个定义，并证明了它们是等价的；其次引入判别轨线沿固定方向进入奇点的一个充要条件，得到平面解析系统在非正则变换下系统轨道可以具有不同的单值性；最后通过对平面解析系统做正则变换，证明了变换前后的系统轨线具有相同的单值性。该结果对研究平面解析系统单值轨道的不变性具有参考价值。

关键词： 平面解析系统；轨线；奇点；单值性问题；正则坐标变换

中图分类号： O175.14

文献标志码： A

文章编号： 1673-3851 （2023） 11-0775-09

引文格式：郭春，黃土森.坐标变换下平面解析系统单值轨道的不变性［J］. 浙江理工大学学报（自然科学），2023，49（6）：775-783.

Reference Format： GUO Chun， HUANG Tusen. Invariance of monodromic orbits of planar analytic system under coordinate transformation［J］. Journal of Zhejiang Sci-Tech University，2023，49（6）：775-783.

Invariance of monodromic orbits of planar analytic system under coordinate transformation

GUO Chun， HUANG Tusen

（School of Science， Zhejiang Sci-Tech University， Hangzhou 310018， China）

Abstract：  In order to study the invariance of monodromic orbits under which coordinate transformation of planar analytic system， firstly， two definitions of orbits of planar analytic system entering singular point along a fixed direction are given， and they are proved to be equivalent. Secondly， a necessary and sufficient condition for discriminating that orbits enter singular point along a fixed direction is introduced， and it is obtained that the orbits for the planar analytic system and the associated system under non-regular coordinate transformation have a different monodromy. Finally， by making a regular coordinate transformation on the planar analytic system， it is proved that the orbits for the planar analytic system and the associated system have the same monodromy. The result can provide a reference for studying the invariance of monodomic orbits of planar analytic system.

Key words： planar analytic system; orbit; singular point; monodromy problem; regular coordinate transformation

0 引 言

非线性微分方程出现在应用科学的许多分支中，而中心与焦点的区分问题（简称中心问题或稳定性问题）是平面微分系统定性理论中尚未完全解决的经典问题之一［1］。

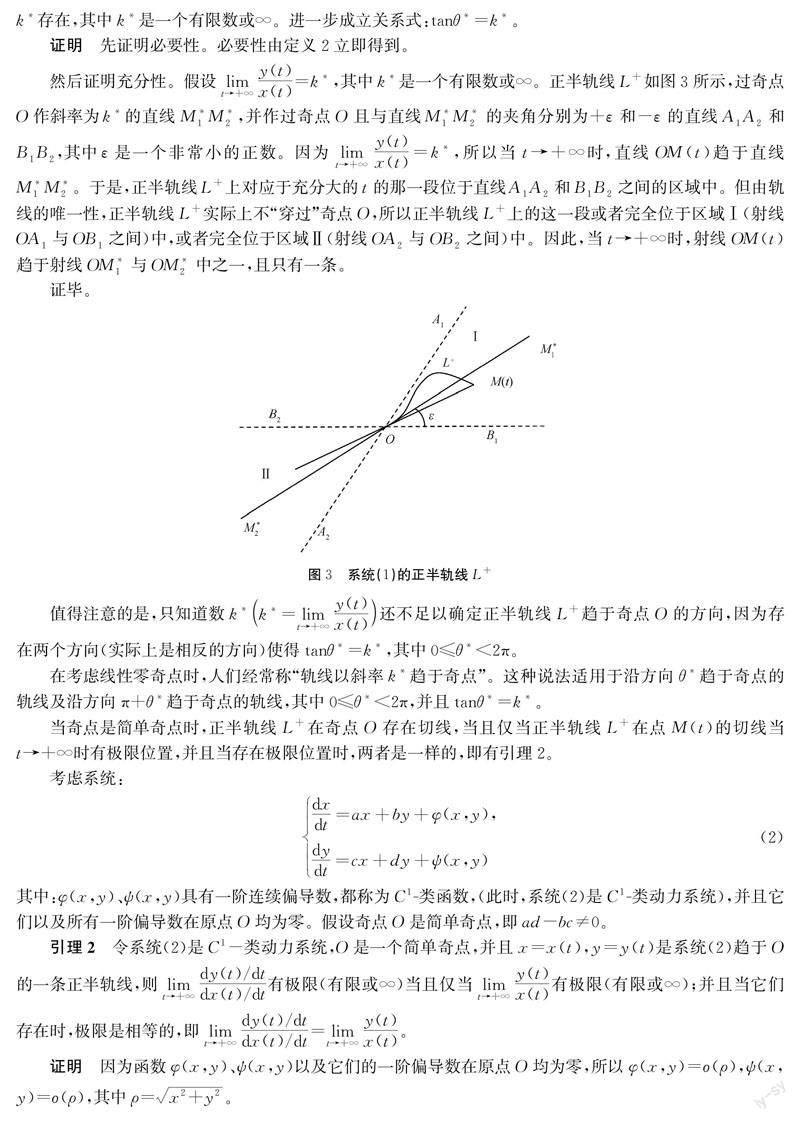
在中心问题中，首先要确定系统的奇点是否为单值的，即在该点的某邻域中是否可以定义Poincar第一返回映射［2］；然后进一步确定它是中心还是焦点。对平面解析系统而言，如果有轨线进入奇点，那么只能螺旋形进入或沿固定方向进入［3］，当系统没有轨线沿固定方向进入奇点，则该奇点只能是单值奇点。因此人们可以根据系统有无轨线沿固定方向进入奇点研究单值性问题。

当奇点的线性化矩阵不恒为零时，单值性问题已经完全解决；当线性化矩阵的特征值是一对共轭复数（本文称这样的奇点为简单奇点）时，单值性问题由Poincar［4］解决；当线性化矩阵是幂零矩阵（本文称这样的奇点为幂零奇点）时，单值性问题由Andreev［5］解决；当线性化矩阵恒等于零（本文称这样的奇点为线性零奇点）时，单值性问题的研究要困难很多，目前学界仍然没有完全解决。目前判别中心和焦点的一种常用方法是Blow-up技术［6］，它通过对这样的系统在线性零奇点附近做一系列变量变换，把奇点变为一个不可定向曲面上的一条封闭曲线，进而只需研究其上的简单奇点或幂零奇点，再通过反变换得到原系统线性零奇点的单值性。然而，为确定参数比较多的平面线性零奇点单值性，一方面Blow-up技术过程就会变得十分繁杂；另一方面，每一次变量变换前后两个系统的单值性有可能发生了改变（见例1）。正因为如此，使用Blow-up技术研究线性零奇点单值性仅仅取得了部分结果［7-9］。较好的结果是Algaba等［10］给出的一种新算法，这种算法虽然繁杂，但还是能有效地确定形式比较简单的线性零奇点的单值性。

坐标变换是研究系统奇点单值性的另外一个有力工具，其基本思想是通过坐标变换，将平面解析系统化简为更简单的形式，特别是可以减少系统中一些不起作用的参数的项，使得变换后的系统在形式上更为简单，从而能更好地解决问题。例如正规形理论［11］就是一种典型的工具，它通过寻找合适的近恒等变量变换，在形式上尽可能多地消去一些不影响系统定性结构的参数，简化系统以方便研究［12-15］。针对平面解析系统奇点单值性的判定，人们自然希望经过坐标变换，不但要把系统变得简单，而且还应保持变换前后两个系统的对应奇点具有相同的单值性。然而，事实并非如此：连续且可逆的坐标变换（即拓扑变换）或可微但不可逆的坐标變换（比如Blow-up技术中的变换），都可以把一个非单值奇点变为单值奇点，或反之。

本文研究平面解析系统在不同坐标变换下单值轨道的不变性。首先，用两个实例说明系统在非正则变换下，变换前后系统的轨道的单值性是不同的；其次，在正则变换下，将变换表达式的非线性部分表示为积分形式，结合微分定义得到轨线函数的极限存在，从而证明了坐标变换是正则时，能够实现经过坐标变换，使得变换前与变换后的两个系统的对应奇点具有相同的单值性，这为中心焦点的判别问题提供了一定的理论依据。

3 结 语

本文研究了平面解析系统的单值轨道的不变性问题。若系统是解析的但坐标变换是拓扑的或是（非可逆）可微的，则这样的坐标变换不能保证变换前后的系统有相同的单值性。本文证明：如果坐标变换是正则的，则能保证变换前后系统有相同的单值性。正规型理论中所做的近恒等变换显然是正则变换，因此任何解析系统与它的正规型具有相同的单值性。这个结果在平面解析系统经典的中心问题的研究中具有重要的理论意义与应用价值。因为一方面，在研究中心问题前，需要先解决奇点的单值性问题；另一方面，研究奇点的单值性问题，可以利用正规型理论把系统中那些不重要的项通过近恒等坐标变换消去，从而得到形式更简单的系统以易于研究。尤其对含线性零奇点的系统，有例子表明，通过Blow-up技术是难以实现的，尽管任何解析系统可以通过做有限次的可微但不可逆的Blow-up变换，把线性零奇点化为一般是不可定向曲面上的一条封闭曲线，并且可进一步通过时间尺度变换化为有限个简单奇点去研究。

由于可逆的可微坐标变换一般未必是正则变换，对于这种变换能否保证变换前后系统有相同的单值性仍有待继续研究。另外，如果系统不是解析的，何种坐标变换能保证变换前后系统有相同的单值性也是一个有待研究的问题。

参考文献：

［1］Algaba A， Freire E， Gamero E， et al. Monodromy， center-focus and integrability problems for quasi-homogeneous polynomial systems［J］. Nonlinear Analysis： Theory， Methods & Applications， 2010， 72（3/4）： 1726-1736.

［2］Arnold V I， Ilyashenko Y S. Ordinary Differential Equations［M］. Berlin： Springer-Verlag， 1988： 1-148.

［3］张芷芬， 丁同仁， 黄文灶， 等. 微分方程定性理论［M］. 北京： 科学出版社， 1985： 19-96.

［4］Poincar H. Mmoire Sur Les Courbes Dfinies Par Une quation Diffrentielle Oeuvres［M］. Paris： Gauthier-Villars， 1957： 251-290.

［5］Andreev A F. Investigation of the behaviour of the integral curves of a system of two differential equations in the neighborhood of a singular point［J］. Transactions of the American Mathematical Society， 1958， 8（2）：183-207.

［6］lvarez M J， Ferragut A， Jarque X. A survey on the blow up technique［J］. International Journal of Bifurcation and Chaos， 2011， 21（11）： 3103-3118.

［7］Algaba A， García C， Reyes M. Characterization of a monodromic singular point of a planar vector field［J］. Nonlinear Analysis： Theory， Methods & Applications， 2011， 74（16）： 5402-5414.

［8］Maosa V. On the center problem for degenerate singular points of planar vector fields［J］. International Journal of Bifurcation and Chaos， 2002， 12（4）： 687-707.

［9］García I A， Gin J， Grau M. A necessary condition in the monodromy problem for analytic differential equations on the plane［J］. Journal of Symbolic Computation， 2006， 41（9）： 943-958.

［10］Algaba A， García C， Reyes M. A new algorithm for determining the monodromy of a planar differential system［J］. Applied Mathematics and Computation， 2014， 237： 419-429.

［11］Ashkenazi M， Chow S N. Normal forms near critical points for differential equations and maps［J］.IEEE Transactions on Circuits and Systems， 1988， 35（7）： 850-862.

［12］Algaba A， García C， Gin J. Analytic integrability around a nilpotent singularity［J］. Journal of Differential Equations， 2019， 267（1）： 443-467.

［13］Algaba A， García C， Reyes M. Algebraic integrability of nilpotent planar vector fields［J］. Chaos， Solitons &  Fractals， 2021， 145： 110765.

［14］Queiroz L， Pessoa C. Nilpotent centers in R3［EB/OL］. （2021-10-05）［2022-10-22］. https：//arxiv.org/abs/2110.02383v1.

［15］Pessoa C， Queiroz L. Monodromic nilpotent singular points with odd Andreev number and the center problem［J］. Qualitative Theory of Dynamical Systems， 2022， 21（4）： 1-24.

［16］唐敏， 黄土森. 关于平面解析系统的定性结构［J］. 浙江理工大学学报， 2015， 33（1）： 140-145.

［17］陆启韶， 彭临平， 杨卓琴. 常微分方程与动力系统［M］. 北京： 北京航空航天大学出版社， 2010： 74-103.

（责任编辑：康 锋）

收稿日期： 2022-09-15网络出版日期：2022-12-05

基金项目： 国家自然科学基金项目（11671359，11672270）

作者简介： 郭 春（1998— ），女，河南南阳人，硕士研究生，主要從事微分方程定性理论方面的研究。

通信作者： 黄土森，E-mail：huangtusen@sina.com