一种适用于线路两侧电势幅值不相等的实时功角计算方法

黄少锋，李 慧，李轶凡

一种适用于线路两侧电势幅值不相等的实时功角计算方法

黄少锋，李 慧，李轶凡

(新能源电力系统国家重点实验室(华北电力大学)，北京 102206)

功角实时计算通常应用于电力系统稳定分析与振荡识别，但是现有的线路功角计算方法在两侧电势幅值不相等时存在较大误差，为此提出了一种新的实时功角计算方法。针对计算电压最低点位于电势连线内、外两种情况进行讨论，得到不同状态下的功角计算公式，并对两种功角计算公式进行判别。考虑到以稳态母线电压来近似电势幅值，会给功角计算带来一定误差，为此对电势幅值进行修正，并将修正后的功角计算误差与现有方法的误差进行对比。基于PSCAD的仿真验证证明了新方法的可靠性和有效性。结果表明，相比于现有功角计算方法而言，新方法有效降低了两侧电势幅值不相等时功角计算的误差，具有良好的计算精度和实用效果。

系统振荡；等效双电源系统；功角计算；两侧电势幅值比；误差分析

0 引言

功角是表征电力系统运行状态的重要参数，线路两侧功角在失步解列、在线稳定分析与控制、距离保护振荡闭锁等方面起着重要作用[1-5]，因此，寻求可靠、准确的方法实时计算功角，对于电力系统监视、预测、控制与保护具有重要意义[6-9]。近年来，基于相量测量单元(phasor measurement unit, PMU)的广域测量系统日趋完善[10-14]，实现了量测信息的全局性与实时性，为功角实时计算提供了技术支撑。

通常，线路两侧功角可以基于等效双电源系统模型进行计算，其方法主要分为两种。(1) 测量送、受端系统内每台发电机的电势相角，通过等值变换将各发电机电势相角等效为双电源系统功角[15]。文献[16-17]分别基于观测器与非线性估计器估计发电机电势相角，基于此可以等值得到双电源系统功角；文献[18]介绍了一种发电机电势相角的直接测量方法，进一步可以求出系统功角；文献[19]利用PMU测量发电机电势相角，并总结了现有的电势相角测量方法，进而可以计算系统功角。但由于发电机相角难以准确测量，通过文献[16-19]所述方法实时测量功角，在工程实际中难以实现。(2) 利用线路两侧母线可测的电气量，通过振荡中各电气量与功角间的变化规律间接求取功角。文献[1,20]分析了等效双电源系统功角、电势及电势连线垂线之间的相关关系，文献[21]根据上述相关关系，计算振荡过程中的功角值，在两侧电势幅值相等时具有良好的实用价值，但是未考虑两侧电势幅值不相等的情况。

针对文献[21]的不足之处，本文提出了一种新的实时功角计算方法。对于两侧电势幅值比的不同情况进行讨论，得到不同电势比的功角表达式；此外，为了减小功角计算的误差，对功角表达式中的电势幅值进行修正，保证了功角计算的可靠性。

1 功角计算原理

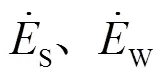
图1 等效双电源系统示意图

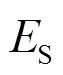
下面对两侧电势幅值比的不同情况进行讨论，得到不同电势比的功角表达式，提高功角计算的有效性、实用性。

1.1 两侧电势幅值相等

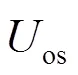
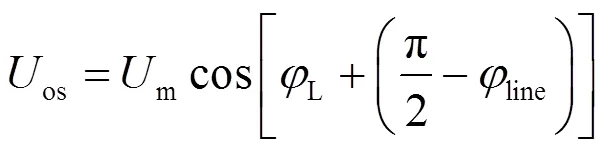
式中：为PMU实测M点电压；为M点测量电压超前测量电流的角度；为系统综合阻抗角。电力系统通常将式(1)简化为[1]。

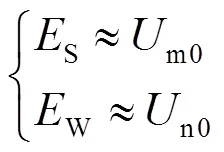
因此，式(3)即为现有的功角计算方法。

1.2 两侧电势幅值不相等

1.2.1原理分析

下面分别对图3中两种O点位置对应的功角进行分析。

1) O点位于电势连线内对应的功角

2) O点位于电势连线外对应的功角

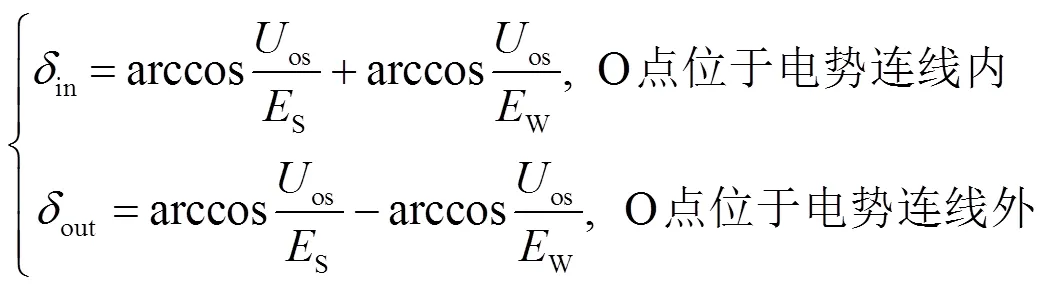
式(6)中，O点位于电势连线内、外对应的功角取值分别为[25]

1.2.2功角表达式的判别方法

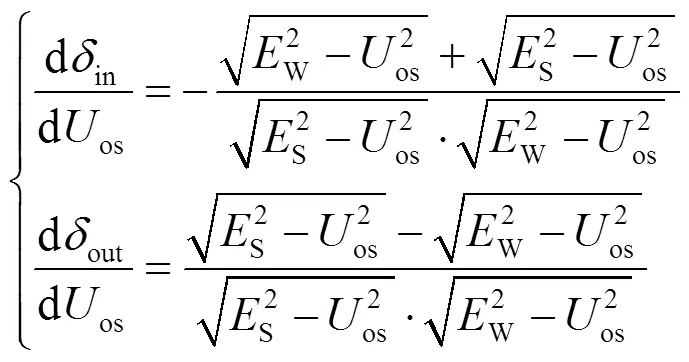
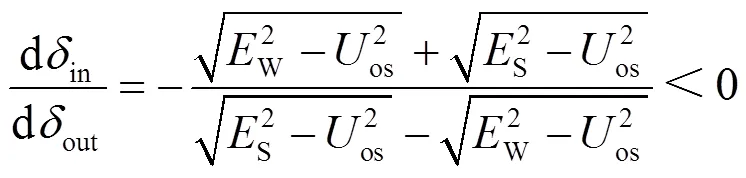
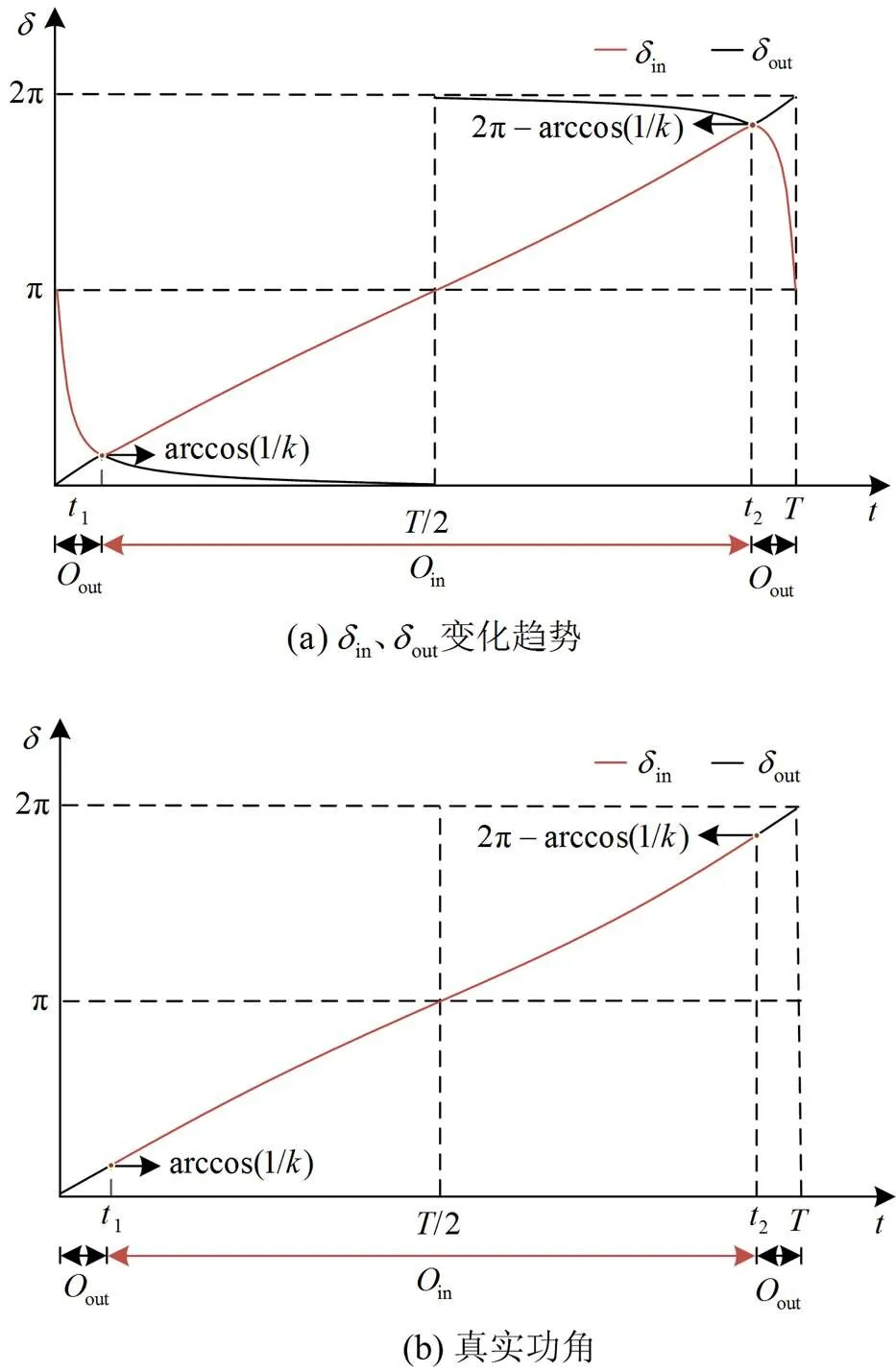
图4 一个振荡周期内功角

上述功角表达式判别方法需要真实功角的变化趋势，然而真实功角的变化趋势难以直接得到，故通过振荡电流与两侧母线电压夹角的变化规律间接反映功角变化趋势。

将式(11)代入式(10)得

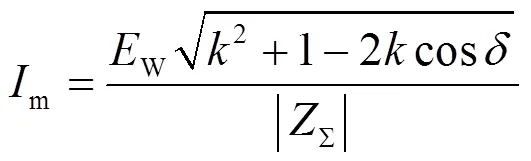
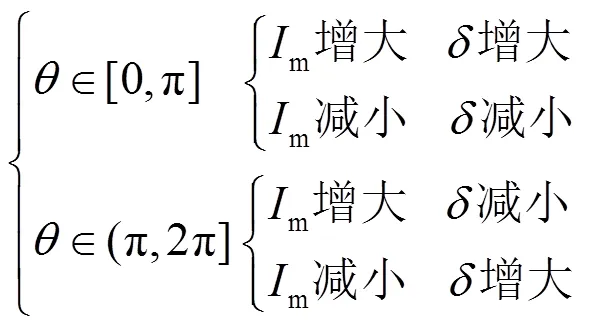
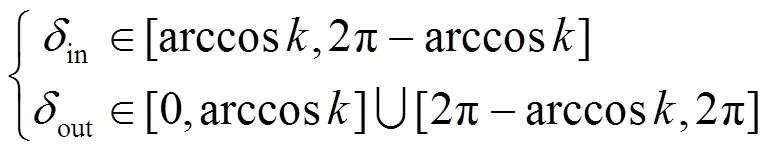
2 电势幅值修正及误差分析

基于上述分析，对功角表达式中的电势幅值进行修正，推导了最优幅值修正系数，并将修正后的功角计算误差与现有方法的误差进行对比。

2.1 现有方法误差分析

为了证明新方法有效提高了功角计算的精确度，从理论误差的角度进行对比分析。因此，对现有功角计算方法的误差进行分析，以便与下文新方法的误差进行对比。

2.1.1误差表达式推导

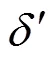
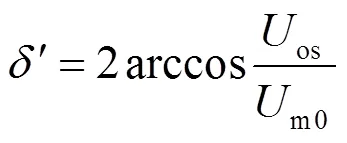
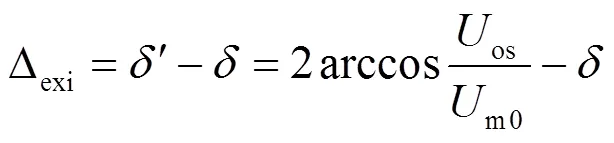
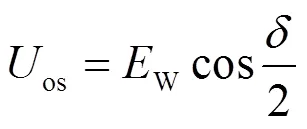
从而有

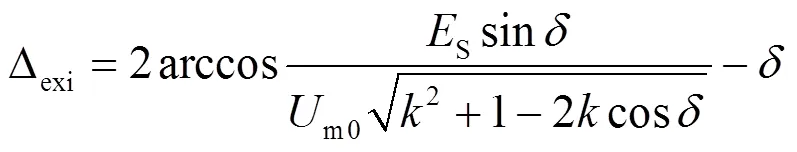
2.1.2取值范围分析

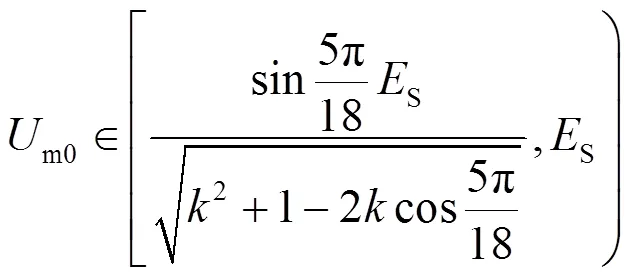
2.1.3误差边界分析

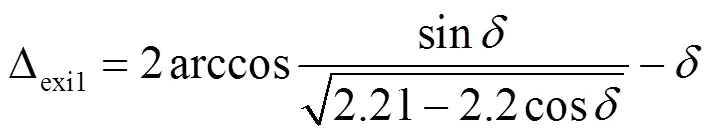
式(26)、式(27)正是第2.4节中误差对比分析的基础。

2.2 新方法的电势幅值修正

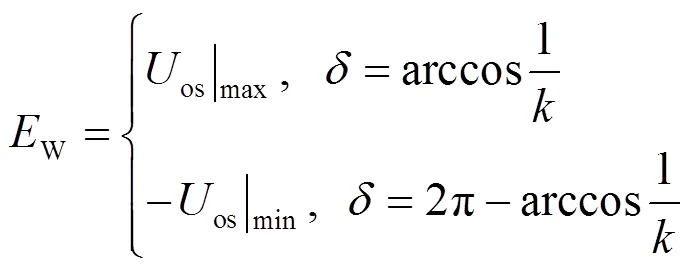
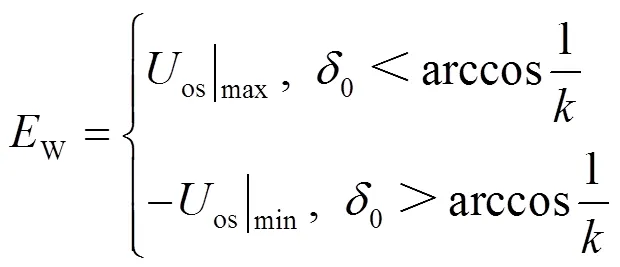
图5 曲线图

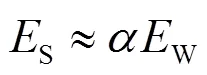
2.3 新方法误差及最优幅值修正系数

下面推导误差表达式，作为最优幅值修正系数分析的基础。

2.3.1误差表达式推导

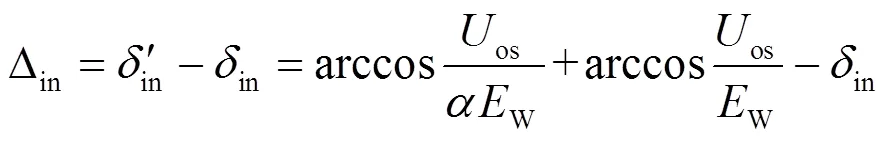
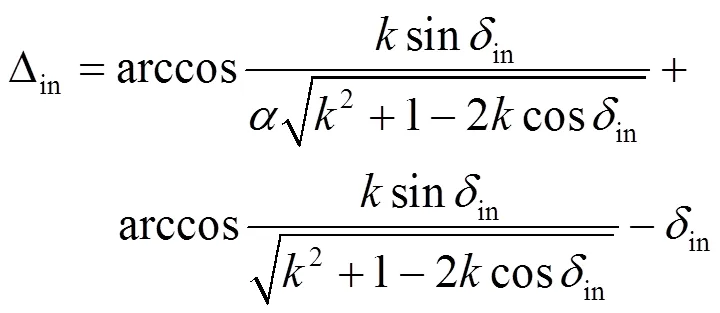
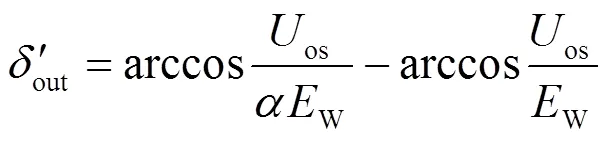
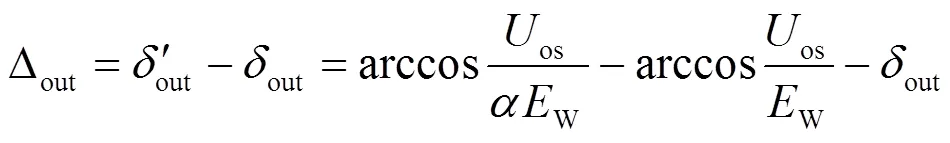
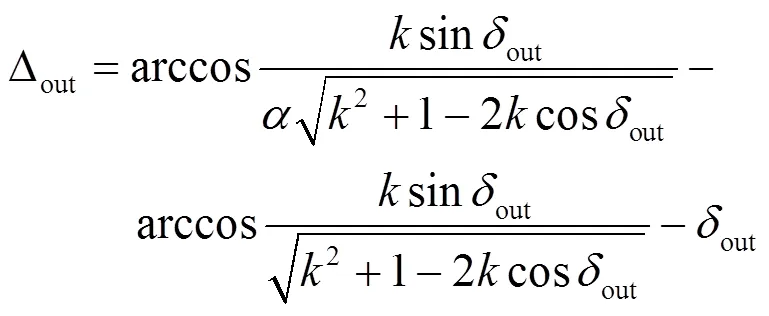
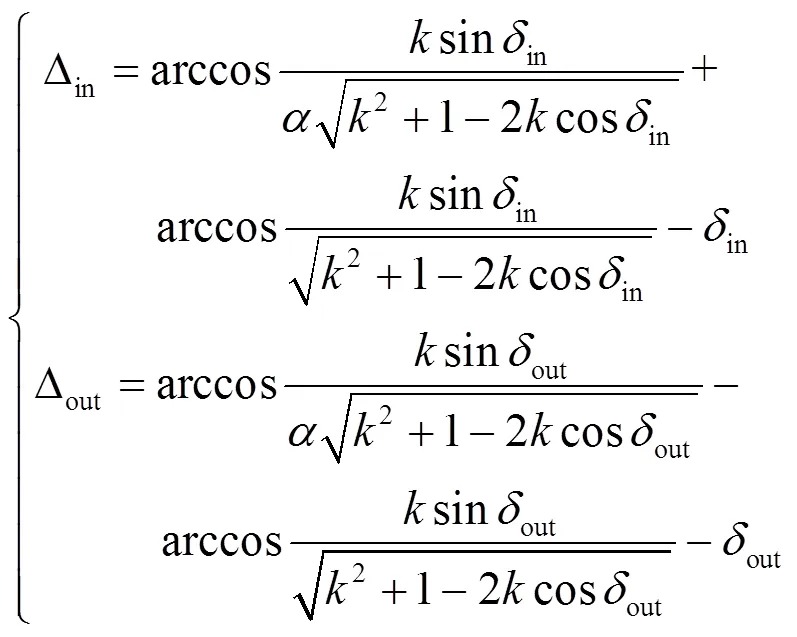
2.3.2最优幅值修正系数

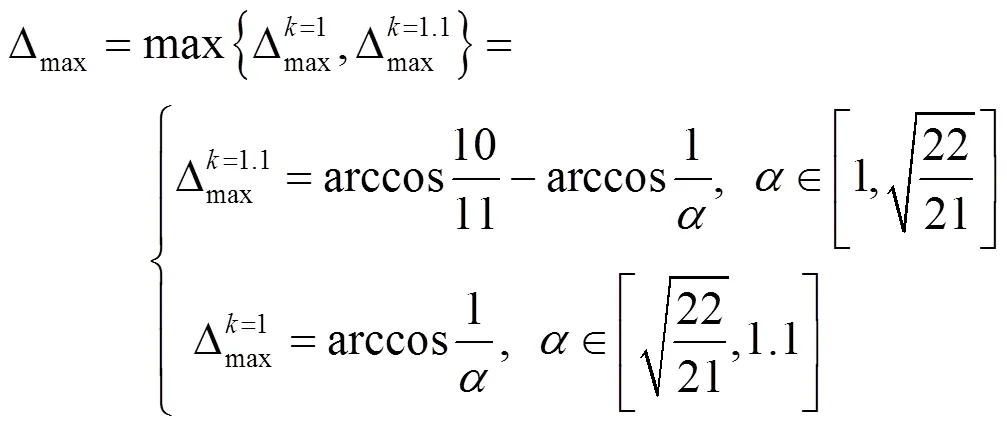
2.3.3误差边界分析

基于上述结论，给出新方法的误差边界，作为下文误差对比分析的基础。

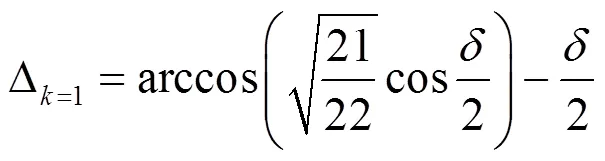
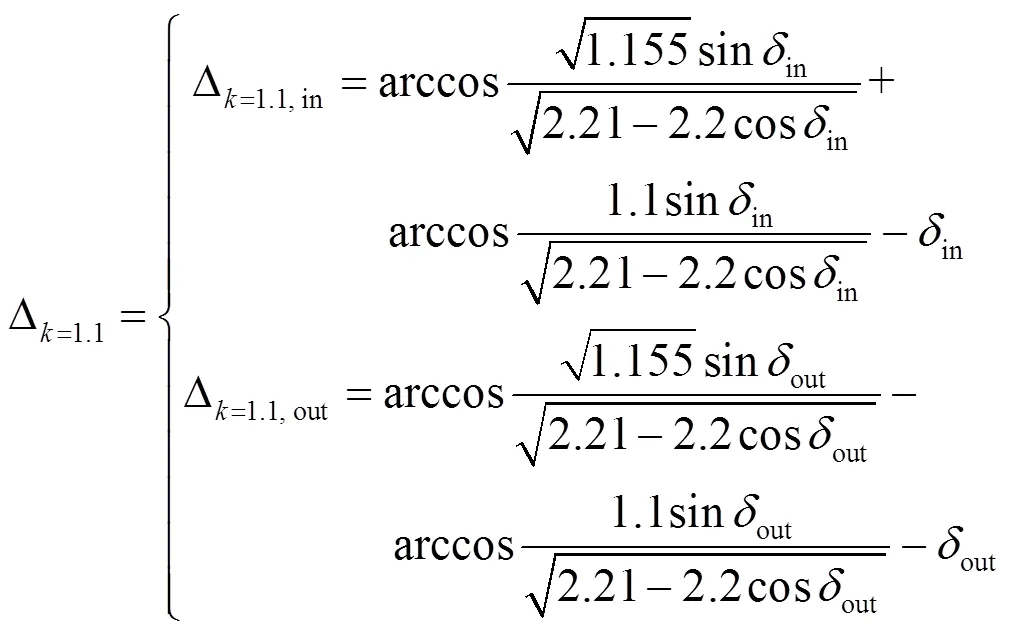
2.4 误差对比与分析

为了进一步验证新方法的可靠性，从以下两种角度进行误差分析。

1) 与现有方法对比分析

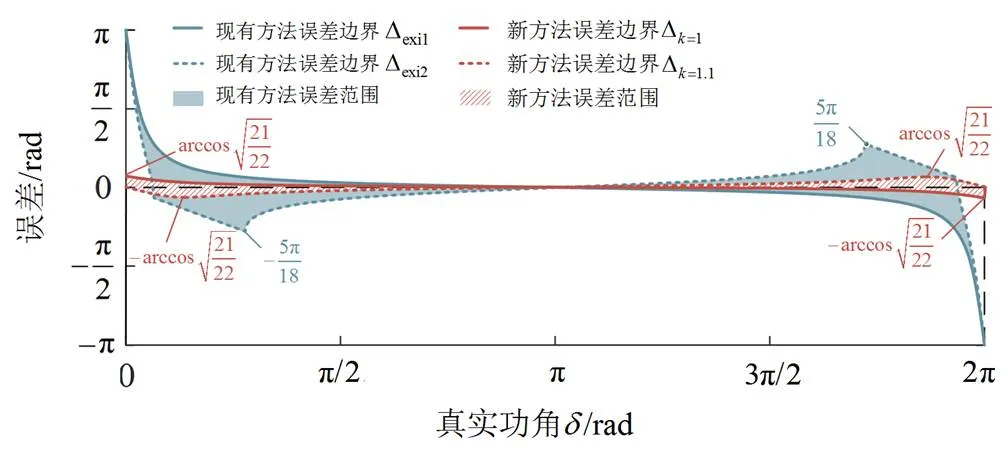
图7 误差边界曲线

2) 结合实际分析

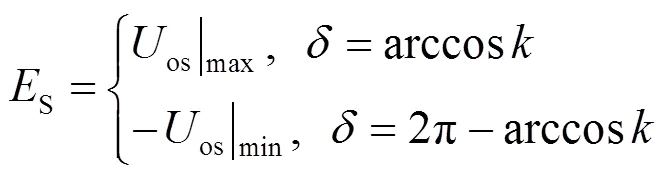
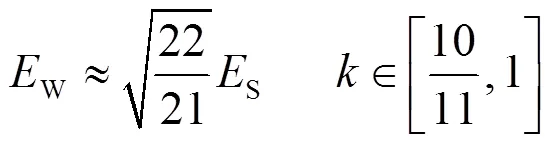
此时，可以通过式(44)、式(45)对功角表达式(15)中的电势幅值进行修正。

3 仿真验证

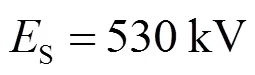
根据上述分析，新的功角计算方法误差在允许范围内，提高了两侧电势幅值不相等时功角计算的精确度。

此外，还验证了多种情况，均证明了上述理论的正确性，不再赘述。

4 结论

本文基于等效双电源系统两侧电势幅值比的不同情况进行讨论，提出了一种新的实时功角计算方法。在两侧电势幅值不相等的情况下，针对振荡过程中计算电压最低点的不同状态，给出了不同的功角计算公式，并通过判断真实功角变化趋势对两种功角计算公式进行判别。为了减小功角计算误差，进一步对电势幅值进行修正，推导了最优幅值修正系数，并将修正后的功角计算误差与现有方法的误差进行对比。理论分析和仿真结果均表明，新方法提高了两侧电势幅值不相等时功角计算的精确度，保证了功角计算的可靠性，具有更普遍的意义和更为实用的价值。

为了突出重点、避免繁复，有关分布电容及系统等值阻抗突变对本文功角计算方法的影响将在以后的论文中进行分析。

[1] 黄少锋. 电力系统继电保护[M]. 北京: 中国电力出版社, 2015.

[2] 瞿艳霞, 时伯年, 刘志超, 等. 基于视在阻抗角的失步解列判据改进研究[J]. 电力系统保护与控制, 2020, 48(5): 181-187.

QU Yanxia, SHI Bonian, LIU Zhichao, et al. Improvement of out-of-step separation criterion based on apparentimpedance angle[J]. Power System Protection and Control, 2020, 48(5): 181-187.

[3] 冯双, 崔昊, 吴熙, 等. 基于对角化LDPC压缩感知和k-近邻算法的广域系统宽频振荡监测方法[J]. 电网技术, 2021, 45(8): 3025-3033.

FENG Shuang, CUI Hao, WU Xi, et al. Monitoring method of wide-band oscillation based on compressed sensing of diagonalizable LDPC and k-nearest neighbour algorithm[J]. Power System Technology, 2021, 45(8): 3025-3033.

[4] 相禹维, 王彤, 李聪聪, 等. 面向大容量直流闭锁的暂态稳定紧急切机控制策略研究[J]. 电力系统保护与控制, 2021, 49(15): 84-92.

XIANG Yuwei, WANG Tong, LI Congcong, et al. Strategy of emergency generator tripping control for transient stability after a large capacity HVDC blocking fault[J]. Power System Protection and Control, 2021, 49(15): 84-92.

[5] 田芳, 周孝信, 史东宇, 等. 基于卷积神经网络的电力系统暂态稳定预防控制方法[J]. 电力系统保护与控制, 2020, 48(18): 1-8.

TIAN Fang, ZHOU Xiaoxin, SHI Dongyu, et al. A preventive control method of power system transient stability based on a convolutional neural network[J]. Power System Protection and Control, 2020, 48(18): 1-8.

[6] 朱劭璇, 王彤, 王增平, 等. 考虑主导不稳定平衡点变化的电力系统暂态稳定切机控制策略[J]. 电力系统保护与控制, 2021, 49(5): 20-28.

ZHU Shaoxuan, WANG Tong, WANG Zengping, et al. Generator tripping strategy in transient stability control of a power system considering the change of the controlling unstable equilibrium point[J]. Power System Protection and Control, 2021, 49(5): 20-28.

[7] CHEN Y, MAZHARI S M, CHUNG C Y, et al. Rotor angle stability prediction of power systems with high wind power penetration using a stability index vector[J]. IEEE Transactions on Power Systems, 2020, 35(6): 4632-4643.

[8] YUAN H, XU Y. Preventive-corrective coordinated transient stability dispatch of power systems with uncertain wind power[J]. IEEE Transactions on Power Systems, 2020, 35(5): 3616-3626.

[9] 蒋越梅, 卢敏, 周浩涵, 等. 大电网事故预案推演系统的设计与实现[J]. 电力系统保护与控制, 2021, 49(3): 142-149.

JIANG Yuemei, LU Min, ZHOU Haohan, et al. Design and realization of an anticipated accident plan simulation system for a bulk power system[J]. Power System Protection and Control, 2021, 49(3): 142-149.

[10] 徐飞阳, 薛安成, 常乃超, 等. 电力系统同步相量异常数据检测与修复研究现状与展望[J]. 中国电机工程学报, 2021, 41(20): 6869-6886.

XU Feiyang, XUE Ancheng, CHANG Naichao, et al. Research status and prospects of detection, correction and recovery for abnormal synchrophasor data in power system[J]. Proceedings of the CSEE, 2021, 41(20): 6869-6886.

[11] AGUSTONI M, CASTELLO P, FRIGO G. Phasor measurement unit with digital inputs: synchronization and interoperability issues[J]. IEEE Transactions on Instrumentation and Measurement, 2022, 71: 1-10.

[12] APPASANI B, JHA A V, MISHRA S K, et al. Communication infrastructure for situational awareness enhancement in WAMS with optimal PMU placement[J]. Protection and Control of Modern Power Systems, 2021, 6(1): 124-135.

[13] 戴锋, 叶昱媛, 刘贞瑶, 等. 基于S变换及同步相量测量的输电线路故障定位研究[J]. 电测与仪表, 2020, 57(8): 13-19.

DAI Feng, YE Yuyuan, LIU Zhenyao, et al. Research on fault location of transmission line based on S-transform and synchronized phasor measurement[J]. Electrical Measurement & Instrumentation, 2020, 57(8): 13-19.

[14] 赵震宇, 邓高峰, 朱亮, 等. 用于反窃电的实时相量测量算法及装置硬件设计[J]. 电测与仪表, 2021, 58(2): 101-106.

ZHAO Zhenyu, DENG Gaofeng, ZHU Liang, et al. Real-time phase measurement algorithm and device hardware design for reverse power theft[J]. Electrical Measurement & Instrumentation, 2021, 58(2): 101-106.

[15] 薛禹胜. 运动稳定性量化理论－非自治非线性多刚体系统的稳定性分析[M]. 南京: 江苏科学技术出版社, 1999.

[16] 杨凌霄, 杨松林. 基于观测器的发电机功角和未知输入估计[J]. 电力系统保护与控制, 2016, 44(15): 128-134.

YANG Lingxiao, YANG Songlin. Observer-based power angle and unknown input estimations for generator system[J]. Power System Protection and Control, 2016, 44(15): 128-134.

[17] TRIPATHY P, SRIVASTAVA S C, SINGH S N. A divide-by-difference-filter based algorithm for estimation of generator rotor angle utilizing synchrophasor measurements[J]. IEEE Transactions on Instrumentation and Measurement, 2010, 59(6): 1562-1570.

[18] 李刚, 王少荣, 夏涛, 等. 电力系统广域动态监测中的功角直接测量技术[J]. 电力系统自动化, 2005, 29(3): 45-50.

LI Gang, WANG Shaorong, XIA Tao, et al. Direct measurement of power angles in wide-area power system dynamic supervisory[J]. Automation of Electric Power Systems, 2005, 29(3): 45-50.

[19] 伍双喜, 吴文传, 张伯明, 等. 用PMU实测数据辨识同步发电机参数的关键问题[J]. 电力系统自动化, 2012, 36(17): 50-55.

WU Shuangxi, WU Wenchuan, ZHANG Boming, et al. Key issues of synchronous generator parameter identification using PMU measurements[J]. Automation of Electric Power Systems, 2012, 36(17): 50-55.

[20] 邓华, 高鹏, 王建全. 关于振荡角的振荡中心电压和ucosφ的变化特征[J]. 电力系统及其自动化学报, 2007, 19(1): 68-73.

DENG Hua, GAO Peng, WANG Jianquan. Oscillation center voltage of oscillation-angle and the characteristics of ucosφ[J]. Proceedings of the CSU-EPSA, 2007, 19(1): 68-73.

[21] 黄少锋, 赵远, 申洪明, 等. 一种基于功角计算的振荡闭锁方案[J]. 电力系统自动化, 2015, 39(2): 87-91.

HUANG Shaofeng, ZHAO Yuan, SHEN Hongming, et al. A scheme for swing blocking based on calculation of power angle[J]. Automation of Electric Power Systems, 2015, 39(2): 89-91.

[22] 刘福锁, 方勇杰, 吴雪莲, 等. 导致振荡中心迁移的基本因素及其敏感性分析[J]. 中国电机工程学报, 2017, 37(6): 1695-1702.

LIU Fusuo, FANG Yongjie, WU Xuelian, et al. Basic factors leading to the migration of the oscillation center and its sensitivity[J]. Proceedings of the CSEE, 2017, 37(6): 1695-1702.

[23] 李宗翰, 刘道伟, 张东霞, 等. 计及动作时序的电网在线快速主动解列策略计算[J]. 中国电机工程学报, 2021, 41(14): 4740-4752.

LI Zonghan, LIU Daowei, ZHANG Dongxia, et al. Rapid active controlled islanding strategy considering action sequence for power grid[J]. Proceedings of the CSEE, 2021, 41(14): 4740-4752.

[24] 张艳霞, 蒙高鹏, 赵冉, 等. 复杂场景下失步中心定位及振荡中心漂移规律[J]. 电网技术, 2015, 39(8): 2264-2269.

ZHANG Yanxia, MENG Gaopeng, ZHAO Ran, et al. Misstep center location and oscillation center drift in complex scenes[J]. Power System Technology, 2015, 39(8): 2264-2269.

[25] 高鹏, 王建全, 周文平, 等. 关于振荡中心的研究[J]. 电力系统自动化学报, 2005, 17(2): 48-53.

GAO Peng, WANG Jianquan, ZHOU Wenping, et al. Study on oscillation center[J]. Proceedings of the CSU-EPSA, 2005, 17(2): 48-53.

A real-time power angle calculation method suitable for unequal potential amplitudes on both sides of the line

HUANG Shaofeng, LI Hui, LI Yifan

(State Key Laboratory of Alternate Electrical Power System with Renewable Energy Sources,North China Electric Power University, Beijing 102206, China)

Real-time calculation of power angle is usually used in power system stability analysis and oscillation identification,but the existing line power angle calculation method has a large error when the potential amplitudes on both sides are not equal. For this reason, a new real-time power angle calculation method is proposed. Two situations where the lowest point of the calculated voltage is located inside and outside the potential connection are discussed, and the power angle calculation formulas under different conditions are obtained and distinguished. The steady-state bus voltage is used to approximate the potential amplitude. This will bring a certain error to the power angle calculation. Therefore, the potential amplitude is corrected and the corrected power angle calculation error is compared with the error of the existing method. Simulation verifications based on PSCAD prove the reliability and effectiveness of the new method. The results show that compared with the existing power angle calculation method, the new method effectively reduces the power angle calculation error when the potential amplitudes on both sides are not equal and has good calculation accuracy and practical effect.

system oscillation; equivalent dual power system; power angle calculation; potential amplitude ratio on both sides; error analysis

10.19783/j.cnki.pspc.220567

国家重点研发计划项目资助(2021YFB2401000)

This work is supported by the National Key Research and Development Program of China (No. 2021YFB2401000).

2022-04-20；

2022-06-20

黄少锋(1958—)，男，教授，博士生导师，研究方向为电力系统继电保护；E-mail: huangsf@sf-auto.com

李 慧(1994—)，女，博士研究生，研究方向为电力系统稳定与控制；E-mail: student089@163.com

李轶凡(1994—)，男，通信作者，博士，讲师，研究方向为电力系统稳定与控制。E-mail: ivanlee_lyf@163.com

(编辑 许 威)