Beam dynamics optimization of very-high-frequency gun photoinjector
2022-11-21HanChenLianMinZhengBinGaoZiZhengLiYingChaoDuRenKaiLiWenHuiHuangChuanXiangTangDuanGuZhengQiMengZhangHaiXiaoDengQiangGuDongWang
Han Chen• Lian-Min Zheng • Bin Gao • Zi-Zheng Li • Ying-Chao Du •Ren-Kai Li • Wen-Hui Huang • Chuan-Xiang Tang • Duan Gu•Zheng Qi • Meng Zhang• Hai-Xiao Deng• Qiang Gu•Dong Wang
Abstract Very-high-frequency (VHF) gun photoinjectors,capable of producing high-brightness and high-repetitionrate electron bunches,are some of the best electron sources for driving MHz-class repetition-rate free-electron lasers.In this study, the beam dynamics optimization of a VHF gun photoinjector for Shanghai HIgh Repetition Rate X-ray Free Electron Laser and Extreme Light Facility(SHINE)is systematically demonstrated using a genetic algorithm.Through the inclusion of the solenoid geometry as an optimization variable into the genetic algorithm, the optimum projected normalized emittance for 100 pC bunches with bunch length of 1 mm rms is reduced to 0.1 mm mrad for 100% of the particles and 0.075 mm mrad for 95% of the particles, proving that sub-100 nm emittance can be achieved in the SHINE injector using a single-cell Tsinghua University (THU) VHF gun. This emittance fulfills the requirements not only of SHINE and Linac Coherent Light Source (LCLS)-II but also of LCLS-II-High Energy(LCLS-II-HE). We demonstrate that the optimal emittance in the VHF gun injector is reduced via the optimization of the solenoid geometry,thereby reducing solenoid spherical aberration. Through the inclusion of high-order (H.O.)energy spread among the optimization objectives,the H.O.energy spread can be reduced by a factor of nearly six using a high-harmonic cavity despite a 38% emittance growth. Finally, the beam dynamics in the SHINE main accelerator show that reducing the H.O. energy spread in the injector is of great significance to improving compression efficiency and reducing bunch current spike.
Keywords VHF gun photoinjector ∙Multi-objective optimization ∙Emittance ∙High-order energy spread
1 introduction
X-ray free-electron lasers (FELs) operating at MHzclass repetition rates are expected to significantly improve the average power of lasers and benefit many kinds of scientific research.In recent years,a number of MHz-class repetition-rate free-electron laser facilities, such as Linac Coherent Light Source (LCLS)-II [1] in Stanford Linear Accelerator Center (SLAC) and Shanghai HIgh repetitioN rate X-ray free electron laser and Extreme light facility(SHINE)[2]in Shanghai,have been constructed.A higherenergy upgrade of LCLS-II, known as the LCLS-II-HE facility [3], has also been approved by SLAC. For successful operation, these hard X-ray facilities require electron beams with high peak brightnesses, i.e., low normalized transverse emittances and high bunch currents.For example, LCLS-II and SHINE require normalized transverse emittances lower than 0.4 mm mrad at bunch charges of 100 pC and bunch lengths of 1 mm rms at the ends of their photoinjectors. On the other hand, to enable LCLS-II-HE with wider X-ray energy ranges, emittances lower than 0.1 mm mrad at 100 pC and 1 mm rms are preferred [3–5].
Beam dynamics optimization is important for achieving electron beams with low transverse emittances at certain bunch lengths in the photoinjectors of FEL facilities. For this purpose,start-to-end simulations of beam transmission through a photoinjector can be conducted using beam dynamics simulation codes,such as ASTRA[6],OPAL[7],IMPACT-T [8], and GPT [9]. In such simulations, the key parameters of the injector elements, such as field strengths and element positions, are optimized simultaneously to obtain the best beam quality.
In this paper,we present the results of a beam dynamics optimization of the SHINE photoinjector. A parallel-processing genetic algorithm was employed to simultaneously optimize several variables directly relevant to the injector.The SHINE facility employs a 216.667 MHz very-highfrequency (VHF) gun [10], inspired by the Berkeley VHF gun [11], and operates in continuous-wave (CW) mode as an electron source to produce electron bunches with 1 MHz repetition rates. The designed cathode electric gradient is 30 MV/m, with an input power of 90.4 kW. The beam energy at the gun exit is 868 keV. The fabrication of the gun is already complete, whereas the high-power conditioning is in progress at Tsinghua University (THU). The VHF gun has a lower cathode gradient than those of the commonly used S-band or L-band guns [12, 13]; thus, the expansion of the transverse beam size after emission on the cathode is large, and the influence of solenoid spherical aberration on the emittance growth is relatively severe[14]. Conventional genetic-algorithm optimization of photoinjectors usually considers only the intensity of the electromagnetic field and the positions of beamline elements as the optimization variables [15–18]. However, we have observed that a lower emittance in the VHF gun injector can be obtained when the solenoid geometry is employed as an optimization variable because the spherical aberration of the solenoid becomes the main source of emittance growth in the VHF gun injector.Through the use of a genetic algorithm that includes the solenoid geometry as a variable,we achieve a normalized transverse emittance lower than 0.1 mm mrad for 100 pC bunches with a bunch length of 1 mm rms and thermal emittance of 0.5 mm mrad/mm, typical of multi-alkali antimonide high-quantum-efficiency photocathodes [19, 20]. This emittance fulfills the requirements not only of SHINE and LCLS-II but also of LCLS-II-HE.

The remainder of this paper is organized as follows: In Sect. 2, the genetic algorithm employed in the beam dynamics optimization is introduced, and the results,including for the two optimization objectives for beam emittance and bunch length, are illustrated. In Sect. 3,solenoid geometry optimization is added to the algorithm to reduce the influence of solenoid spherical aberration and to obtain a lower emittance. In Sect. 4, the optimization results for different gun cathode fields,thermal emittances,and bunch charges are presented. In Sect. 5, the H.O.energy spread is set as one of the optimization objectives,and a 3.9 GHz high-harmonic cavity is added to the injector to optimize the H.O.energy spread.Three injectoroptimized cases involving decreases in the H.O. energy spread are then presented. In Sect. 6,for these three cases,we demonstrate the final current distributions at the exit of the main accelerator in FEL. With a lower H.O. energy spread, the current distribution is closer to a flattop distribution.
2 Injector layout and basic settings in optimization
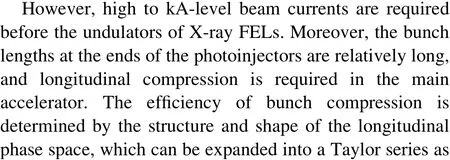
For a VHF gun injector, a drive laser with a long duration, typically 30–60 ps, is usually used to reduce space-charge-induced emittance; therefore, a buncher is needed to compress the bunch length. The injector layout design is illustrated in Fig. 1. The injector consists mainly of a VHF gun,two solenoids(labeled as SOL1 and SOL2),a 2-cell normal conducting CW 1.3 GHz buncher, a single 9-cell 1.3 GHz cavity accelerating unit(labeled as CAV0),and an accelerating cryomodule [26, 27], which includes eight 9-cell 1.3 GHz cavities (labeled as CAV1–CAV8).An example of the axial field distribution of the injector is shown in Fig. 2.
A parallel processing non-dominated sorting genetic algorithm (NSGA)-II [28], written in Python and using a message passing interface (MPI) for communication, is implemented on the Sunway TaihuLight supercomputer to drive ASTRA [6] simulations in the optimization. A laser beam with uniform transverse and temporal flattop distributions is employed in the simulation. The rising time of the longitudinal distribution is fixed at 2 ps. The thermal emittance is determined based on the performance of the photocathode.We set the thermal emittance to be from 0.5 mm mrad/mm to 1 mm mrad/mm. Of this range, 0.5 mm mrad/mm is considered an optimistic case, whereas 1 mm mrad/mm is a conservative case. The maximum cathode field is fixed at 30 MV/m. The heat dissipation in the CW mode and the possibility of RF breakdown in the buncher are taken into account,and thus the maximum on-axis field of the buncher is limited to less than 3 MV/m, corresponding to a bunch voltage of 343 kV.The maximum onaxis field of CAV3–CAV6 is fixed at 30 MV/m, corresponding to an energy gain of 16.3 MeV. The phase of CAV3–CAV6 is fixed at 0 deg (maximum energy gain phase). The gradients of CAV7 and CAV8 are set to zero,which can be adjusted to match the requirements of the laser heater.
Eighteen parameters of the beamline are selected as the optimization variables, as listed in Table 1. The optimized values of the 18 variables for three specific cases, labeled as Cases 1, 2, and 3, are listed in the table. Case 1 is an optimization with only two objectives:emittance and beam length, and the solenoid geometry is optimized. Case 2 involves H.O. energy spread as an objective in addition to the emittance and beam length. Based on Case 2, Case 3 adds a third harmonic cavity into the optimization.Detailed descriptions of these three cases are provided in the following sections.
First, the dual-objective (i.e., emittance and bunch length at the end of the long cryomodule shown in Fig. 1)optimization is conducted using a genetic algorithm. The thermal emittance is assumed to be 1 mm mrad/mm. The method described in Refs. [15] is adopted to reduce the computing time. First, the optimization runs for 300 generations with a small number of macroparticles, given that the CPU time consumption in ASTRA simulation is approximately proportional to the number of macroparticles. The population generated in this run is used as the initial population of the new run, where a large number of macroparticles are used in ASTRA (50000 in our simulation). With this method, approximately only 100 generations are required in the new run to produce satisfactory optimization results. Figure 3a illustrates the Pareto front of the transverse emittance ∈and rms bunch length σzfor different generations. The emittance is calculated statistically from the coordinates of 100%of the particles.After a total of 400 generations,the optimal emittance is 0.15 mm mrad at a bunch length of 1 mm rms, which for a 100 pC bunch roughly corresponds to a peak current of 12 A.
To evaluate the optimization results, we usually compare the optimal emittance at a specific bunch length,such as 1 mm rms. In this case, the efficiency of the dual-objective optimization is low because a large number of individuals are used to optimize the emittances at other bunch lengths. The algorithm is improved to solve this problem, using a single-objective algorithm in the optimization. The single-objective algorithm is based on the penalty function method[29].With the bunch length aimed at 1 mm rms, the single objective f can be expressed as

where max()is a function that returns the larger one of two values, ∈is the value of emittance in mm mrad, and σzis the bunch length in mm. The value-0.1 in the expression indicates that a bunch length between 0.9 and 1.1 mm rms is considered acceptable in the optimization.Meanwhile,α is the penalty coefficient,which is generally an experiential value.We set α=4 for the optimization.It should be noted that there are some other constraint-handling techniques,such as the feasibility-first approach [28], which can also be used in optimization,where no penalty value is needed.
Fig. 1 (Color online) Injector layout design for SHINE

Fig. 2 (Color online) Example of axial field distribution of injector. Two out of eight cavities in the cryomodule are off

Table 1 Eighteen optimization variables for beamline and optimized values for three cases
The advantage of single-objective optimization is its rapid convergence. During the optimization process, the number of individuals in each generation for single-objective optimization is only half that for dual-objective optimization; however, after 400 generations of optimization, single-objective optimization still obtains better optimization results. Figure 3b shows the evolution of the best f for each generation in single-objective optimization.After 400 generations, the best f is equal to the emittance for a bunch length of approximately 1 mm rms, i.e., 0.147 mm mrad.

Fig.3 (Color online)a Pareto front of transverse emittance ∈and rms bunch length σz for different generations.b Evolution of best f in each generation in single-objective optimization
3 Optimization of solenoid geometry
The solenoid spherical aberration resulting from the 3rdorder term of the magnetic field is considered to be one of the sources causing emittance growth [30]. This is especially true for VHF gun injectors, where the reduction in spherical aberration is more important than in S-or L-band gun injectors because of the large beam size and low beam energy in the solenoid.The spherical aberration coefficient C1, which is determined only by the solenoid geometry, is an important factor that describes the emittance growth due to spherical aberration. The value of C1can be calculated using the longitudinal on-axis magnetic field Bz,0[31]:

For our VHF gun injector, optimization of the solenoid geometry is focused on SOL1 because simulations have shown that the emittance growth due to solenoid spherical aberration occurs mainly in SOL1 rather than in SOL2[14]. Figure 4 depicts the basic design of SOL1. The single-side width of the iron yoke is fixed at 1 cm. The outer radius of SOL1 is fixed at 15 cm because of the limitations of the structural dimensions. The inner radius R of SOL1 and the width W of the current region are selected as the optimization variables for our genetic algorithm. A singleobjective optimization is employed. We determine that the genetic algorithm has difficulty converging when these two solenoid variables change continuously along with other beamline variables in the optimization. As a compromise,we perform a two-dimensional discrete scan where R=0.2,2,4,6, and 8 cm and W =5,10,15,20, and 25 cm. The value of R must be less than 8 cm to achieve the required solenoid ampere turns, while W must be less than 25 cm because of the limitation of the beamline element layout. The solenoid field profiles are calculated for all combinations of R and W, and these field profiles are employed as the optimization variables in the genetic algorithm. For example, the field profiles for different R values at W =10 cm are shown in Fig. 5a, and the field profiles for different W values at R=6 cm are shown in Fig. 5b]: During optimization, the position of SOL1 is fixed. The inner radius and current-region width of SOL2 are fixed at 8 and 15 cm, respectively. The ranges of the other beamline element variables are kept the same as those for the optimizations described in Sect. 2.
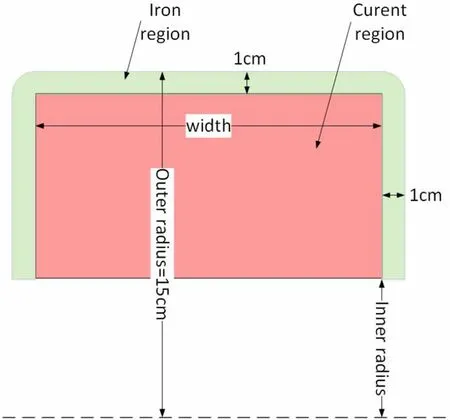
Fig. 4 Basic design of SOL1

Fig. 5 (Color online) a On-axis magnetic field profiles for different inner radii R. Width of current region is fixed at 10 cm. b On-axis magnetic field profiles for different widths W of the current region.Inner radius of solenoid is fixed at 6 cm
First, a conservative thermal emittance of 1 mm mrad/mm is employed in the optimization. The optimal emittances at a bunch length of 1 mm rms for different solenoid geometries are calculated using the genetic algorithm. For example, the optimal emittances for different inner radii R of SOL1 at W =10 cm are shown in Fig. 6a, and the optimal emittances for different current-region widths W at R=6 cm are shown in Fig. 6b. The solenoid spherical aberrations C1for different R and W are also shown in the figure. We determine that C1decreases as the inner radius R is increased;similarly,C1decreases as the current-region width W is increased. Correspondingly, the optimal emittance decreases when C1decreases, indicating that it is important to reduce the solenoid spherical aberration to reduce the final emittance. After optimization of the solenoid geometry,the optimal emittance is 0.138 mm mrad at a bunch length of 1.05 mm rms,where R=8 cm and W =25 cm. In this part of the study, the case that results in the lowest emittance is referred to as Case 1. Figure 7 visualizes the beam dynamics results for Case 1 from ASTRA simulation, including the evolution of the transverse normalized emittance,beam energy,rms beam size,and bunch length in the injector. In addition, the longitudinal phase space and current distribution at the end of the injector are illustrated. The H.O. longitudinal phase space is shown in Fig. 7d with the removal of the first- and second-order energy chirps. For Case 1, the H.O. energy spread is calculated to be 3.46 keV rms. Compared with those for the design of the LCLS-II injector, a higher cathode field and careful solenoid geometry optimization are key to improving the performance of the SHINE injector.

Fig. 6 (Color online) a Optimal emittance and solenoid spherical aberration coefficient C1 change as functions of SOL1 inner radius with current-region width fixed at 10 cm. b Optimal emittance and solenoid spherical aberration coefficient C1 change as functions of current-region width with inner radius fixed at 6 cm
Second,all the beamline parameters are kept the same as in Case 1, but this time, we use the optimistic thermal emittance, i.e., 0.5 mm mrad/mm, in the simulation. The lowest emittance becomes 0.1 mm mrad. Note that, unless mentioned otherwise, the emittances mentioned in this paper involve 100% of the particles. The final projected normalized emittance for 95%of the particles is reduced to 0.075 mm mrad,proving that sub-100 nm emittance can be achieved in the SHINE injector using a single-cell THU VHF gun. The emittance also fulfills the requirements of LCLS-II-HE for the electron beam quality of the lasing spectrum in the FEL [3, 5].
4 optimization results with different cathode
fields, thermal emittances, and bunch charges
With the uncertainty of the achievable cathode field and thermal emittance taken into account, the optimal emittances for different cathode fields and thermal emittances are obtained using the genetic algorithm, as illustrated in Fig. 8a and b, respectively. Dual-objective optimization is employed.The optimal emittance corresponding to a bunch length of 1 mm rms is indicated in the figure by red dashed lines.As shown in Fig. 8a,the optimal emittance for 100%of the particles at a bunch length of 1 mm rms for thermal emittances of 0.5 mm mrad/mm,0.75 mm mrad/mm,and 1 mm mrad/mm are 0.102 mm mrad, 0.129 mm mrad, and 0.150 mm mrad, respectively. As shown in Fig. 8b, the optimal emittance for 100% of the particles at a bunch length of 1 mm rms increases from 0.15 mm mrad to 0.194 mm mrad as the cathode field is decreased from 30 MV/m to 20 MV/m. The beam energies at the gun exit for different cathode fields are also given in the figure legend.
Similarly, the optimal emittances for different bunch charges are calculated using the genetic algorithm, as illustrated in Fig. 8c, considering the possible high-charge mode during the actual operation of the injector. The optimal emittances for 100% of the particles at a bunch length of 1 mm rms for bunch charges of 100 pC, 200 pC,and 300 pC are 0.150 mm mrad, 0.258 mm mrad, and 0.407 mm mrad, respectively.

Fig. 7 (Color online) Beam dynamics results for Case 1 (lowest emittance).a Evolution of transverse normalized emittance and beam energy in injector.b Evolution of rms beam size and bunch length in injector.c Longitudinal phase space and current distribution at end of injector. d H.O. longitudinal phase space at end of injector
5 Optimization of H.O. energy spread
In this part of the study,the H.O.energy spread is set as one of the optimization objectives in addition to the beam emittance and bunch length. Single-objective optimization is employed, and the objective is expressed as f =∈+max(abs(σz-1)-0.1,0)×4+σpHO/20. The value of the H.O. energy spread in keV is σpHO. The thermal emittance is assumed to be 1 mm mrad/mm. First,a beamline that does not include the high-harmonic cavity is used in the optimization. After optimization, the beam emittance is 0.179 mm mrad at a bunch length of 1.081 mm rms, and the H.O. energy spread is 0.92 keV rms. We refer to this case as Case 2. Figure 9a shows the H.O.longitudinal phase space and current distribution at the end of the injector for Case 2. The H.O. energy spread originates mainly from the space charge forces and nonlinearity of velocity bunching in the VHF gun injector [32].
Second,a 3.9 GHz high-harmonic cavity is added to the beamline (downstream of the buncher) to offset the nonlinearity of velocity bunching. The high-harmonic cavity used in the simulation is a 3-cell 3.9 GHz structure with a voltage of 70 kV. Note that this cavity, which operates in the CW mode with such a low voltage,does not need to be superconductive. Single-objective optimization is also employed, and the optimization objective is the same as that in Case 2. After optimization, the beam emittance is 0.193 mm mrad at a bunch length of 1.03 mm rms,and the H.O.energy spread is 0.6 keV rms.We refer to this case as Case 3.Figure 9b shows the H.O.longitudinal phase space and current distribution at the end of the injector for Case 3. It is determined that the H.O. energy spread is close to the slice energy spread after optimization.
The beam emittances, bunch lengths, and H.O. energy spreads for Cases 1, 2, and 3 are summarized in Table 2.Unlike Case 1, Case 3 uses a high-harmonic cavity and adds the H.O. energy spread as an optimization objective,which reduces the H.O.energy spread by a factor of nearly 6, despite a 38% increase in beam emittance. Comparing the current distributions at the end of the injector for Cases 1(Fig. 7c),2(Fig. 9a),and 3(Fig. 9b),we observe that the current distributions for Cases 1 and 2 are very similar,whereas the current distribution for Case 3 is much more symmetrical than those for Cases 1 and 2. This is because Case 3 has benefited from the suppression of the nonlinearity in velocity bunching because of the high-harmonic cavity.It should be noted that a current distribution similar to that for Case 3 cannot be achieved without the highharmonic cavity, even if the symmetry of the current distribution is considered as one of the optimization objectives in Cases 1 and 2.In the following section,the longitudinal beam dynamics in the main accelerator downstream of the injector are evaluated to demonstrate the significance of reducing the H.O. energy spread in the injector.

Fig.8 (Color online)Pareto fronts of emittance and bunch length for different parameters.a Thermal emittances of 0.5 mm mrad/mm,0.75 mm mrad/mm, and 1 mm mrad/mm, with cathode field of 30 MV/m and bunch charge of 100 pC;b cathode fields of 30 MV/m,27 MV/m,25 MV/m,23 MV/m,and 20 MV/m,with thermal emittance of 1 mm mrad/mm and bunch charge of 100 pC; c bunch charges of 100 pC,200 pC, and 300 pC, with thermal emittance of 1 mm mrad/mm and cathode field of 30 MV/m

Fig. 9 (Color online) a H.O. longitudinal phase space and current distribution at end of injector for Case 2 (with optimization of H.O.energy spread). b H.O. longitudinal phase space and current distribution at end of injector for Case 3 (with inclusion of third harmonic cavity)

Table 2 Optimized beam parameters for the three cases
6 Main accelerator simulation results
Bunch longitudinal current spikes in FEL accelerators reduce the laser signal-to-noise ratio and enhance the coherent synchrotron radiation (CSR) effect, further deteriorating the beam emittance [33–35]. A more uniform current distribution is helpful for increasing the FEL output power and achieving a better controllable spectral bandwidth.In seeded FEL,especially in seeded cascade FEL,a flattop current distribution is desired to overcome the influence of time jitter on lasing performance.
To verify the benefits of reducing the H.O. energy spread on improving the current distribution after compression, simulations of the beam dynamics in the SHINE main accelerator are implemented using the beam parameters at the end of the injector for Cases 1, 2, and 3(Table 2) at the beginning of the simulations. Figure 10 shows the layout of the main accelerator at the SHINE facility. LH denotes the laser heater. L1, L2, L3, and L4 denote the four linac boosters composed of superconducting cryomodules. HL is the third harmonic cavity [36].BC1 and BC2 indicate two chicanes. The dechirper is a corrugated wakefield structure[37,38]that is employed to eliminate the linear energy chirp. The bunch length is compressed in two stages in the main accelerator, and the final peak current is above 1 kA. The beam energy is increased to approximately 8 GeV.
Litrack [39] is used to simulate the longitudinal beam dynamics of the main accelerator. Wakefields, nonlinear terms of the RF fields, and chicane compressions are included in the simulation. A genetic algorithm is employed to optimize the final current distribution at the exit of the main accelerator.The optimization variables are the voltage and phase of L1 and HL, the phase of L2, and R56 of BC1 and BC2. The optimization objective is quantified as follows:An asymmetric flattop distribution is used to fit the final current distribution, and the fitting function f can be written as

where H(x) is the Heaviside function, and Itop is the top current of the flattop distribution. Meanwhile, r,t1,t2,L,and d are the fitting parameters.
A fitting example is presented in Fig. 11.We choose the interval where f >Itop×0.9 and mark the boundaries with red dashed lines. The data points in this interval are selected ( green dots in Fig. 11), and the current at each data point is denoted as I.Then,the rms value of I-Itop is calculated as the optimization objective.
After the optimization, the current distributions at the exit of the main accelerator for Cases 1, 2, and 3 are obtained.First,Itop is set to be 1.8 kA for the optimization objective,and the optimum current distributions are shown in Fig. 12. We already know that the H.O. energy spreads at the injector exit for Cases 1,2,and 3 decrease in turn.It can be observed that the current distribution at the exit of the main accelerator is more uniform when the H.O.energy spread at the injector exit is smaller.The top of the current distribution for Case 3 is rather flat.Second,Itop is set to 4 kA for the optimization objective,and the optimum current distributions are shown in Fig. 13.We determine that Case 3 remains to have the most uniform current distribution,although the double horns have started to appear.
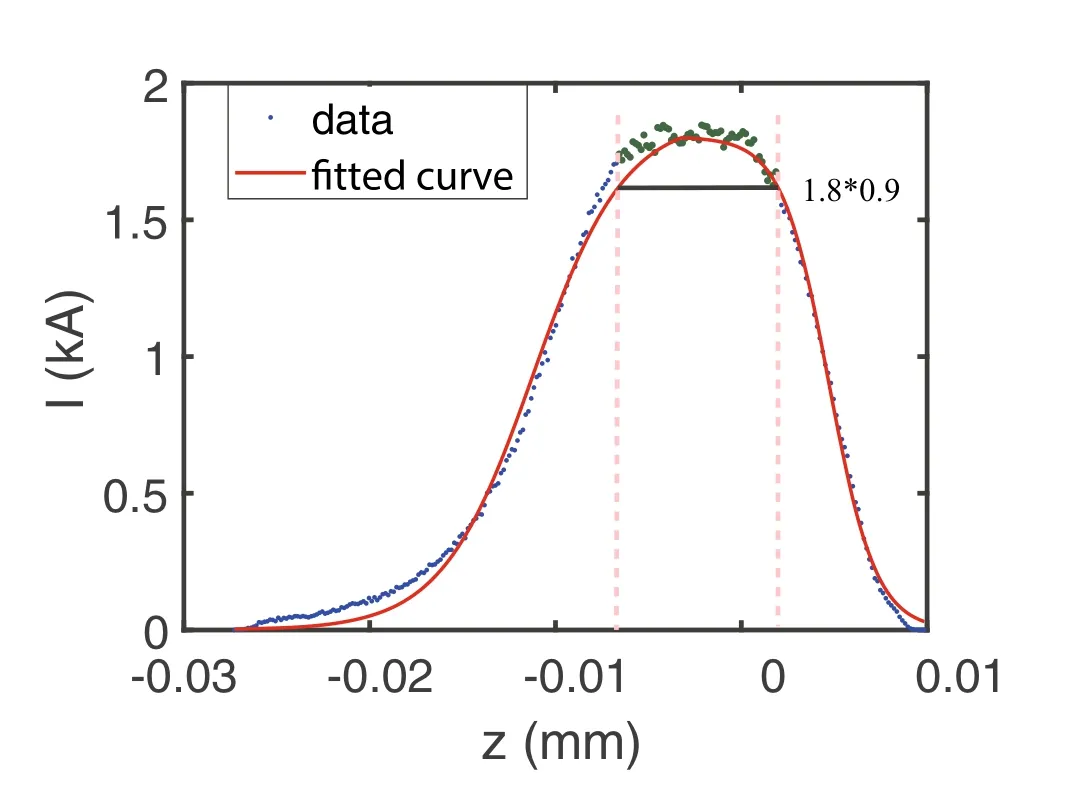
Fig. 11 (Color online) Illustration of method for calculating optimization objective of current distribution in main accelerator

Fig. 10 (Color online) Layout of main accelerator in SHINE facility
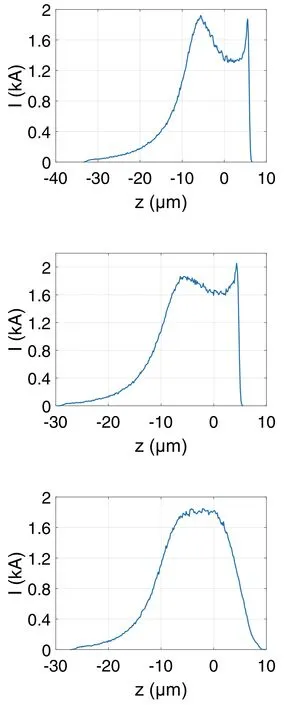
Fig. 12 a, b, and c are the optimum current distributions at exit of main accelerator for Cases 1, 2, and 3, respectively. Itop = 1.8 kA

Fig. 13 a, b and c are the optimum current distributions at exit of main accelerator for Cases 1, 2, and 3, respectively. Itop = 4 kA

Fig. 14 (Color online) Evolutions of longitudinal phase space in main accelerator.Itop =1.8 kA.Three rows correspond to Cases 1,2,and 3, respectively. Phase space of first column is at exit of laser heater. Phase space of second column is at entrance of BC1. Phase space of third column is at exit of main accelerator
The laser heater at the start of the main accelerator is used to increase the beam slice energy spread to prevent microbunch instability.Here,an rms slice energy spread of 3.5 keV is added after the laser heater, based on the longitudinal phase space at the exit of the injector. The dechirper at the end of the main accelerator is used to eliminate the linear chirp in the longitudinal phase space.The evolution of the longitudinal phase-space in the main accelerator is shown in Fig. 14. Itop is set to be 1.8 kA.The three rows correspond to Cases 1, 2, and 3, respectively.The phase space of the first column is located at the exit of the laser heater. The phase space of the second column is at the entrance of BC1. The phase space of the third column is located at the exit of the main accelerator.It can be determined that the final phase space at the exit of the main accelerator maintains the main characteristics of the H.O.phase space at the end of the injector,and that the H.O. energy spread at the end of the injector is greatly amplified during the compression process in the main accelerator. Unlike for the S-band main accelerator in the LCLS, where the nonlinear energy spread is induced mainly by the resistive wall wakefield of the RF structures[40], the nonlinear energy spread in the SHINE VHF gun injector dominates the nonlinear terms in the phase space after the main accelerator. Therefore, reducing the H.O.energy spread at the end of the injector improves compression efficiency and reduces current spike,which are of great significance to FEL operation.
7 Conclusion
In this study, beam dynamics simulation and optimization of a VHF gun photoinjector for SHINE is systematically studied using a parallel processing genetic algorithm.We determine that a lower emittance can be obtained through the application of the solenoid geometry as an optimization variable in the design of the VHF gun injector because the spherical aberration of the solenoid becomes the main source of emittance growth in the VHF gun injector. Based on an assumption that the thermal emittance is 0.5 mm mrad/mm, the optimum projected normalized emittance after the optimization for 100 pC bunches with a bunch length of 1 mm rms is 0.1 mm mrad for 100% of the particles and 0.075 mm mrad for 95% of the particles, proving that sub-100 nm emittance can be achieved in the SHINE injector using a single-cell THU VHF gun.This emittance fulfills the requirements not only of SHINE and LCLS-II but also of LCLS-II-HE.
Moreover, optimizations of the beam emittance and bunch length for different cathode fields, thermal emittances, and bunch charges are demonstrated in this study.
Finally, we emphasize that the H.O. energy spread should be set as one of the optimization objectives in addition to emittance and bunch length. The H.O. energy spread can be reduced by a factor of nearly six using a high-harmonic cavity, despite a 38% emittance growth.The beam dynamics in the SHINE main accelerator show that reducing the H.O. energy spread at the end of the injector improves compression efficiency and reduces current spike, which are of great significance to FEL performance.
Author ContributionsAll authors contributed to the study conception and design. Material preparation, data collection and analysis were performed by Han Chen, Lian-Min Zheng and Ying-Chao Du.The first draft of the manuscript was written by Han Chen and all authors commented on previous versions of the manuscript. All authors read and approved the final manuscript.
杂志排行
Nuclear Science and Techniques的其它文章
- Hard X-ray focusing resolution and efficiency test with a thickness correction multilayer Laue lens
- Monte Carlo study of the neutron ambient dose equivalent at the heavy ion medical machine in Wuwei
- Experimental investigation on the characteristics of molten lead–bismuth non-eutectic alloy fragmentation in water
- Configurational information entropy analysis of fragment mass cross distributions to determine the neutron skin thickness of projectile nuclei
- Differences in MBUs induced by high-energy and medium-energy heavy ions in 28 nm FPGAs
- Thin-film approximate point scattered function and its application to neutron radiography
